Electrical Engineering (EE) Exam > Electrical Engineering (EE) Questions > A 50 Hz synchronous generator is connected t...
Start Learning for Free
A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.
If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.
Correct answer is '1.39'. Can you explain this answer?
Most Upvoted Answer
A 50 Hz synchronous generator is connected to an infinite bus through...
Given : E = 1.1 pu, V = 1 pu, H = 3 MJ/MVA
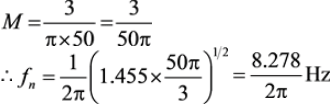= 1.39Hz
Natural oscillation frequency is given by,
At 75% loading,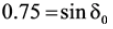
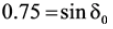
Inertia constant,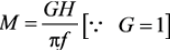
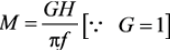
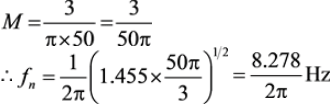= 1.39HzHence, the new natural frequency of oscillation is 1.39 Hz.
Free Test
FREE
| Start Free Test |
Community Answer
A 50 Hz synchronous generator is connected to an infinite bus through...
The new natural frequency of oscillation can be determined by analyzing the system's dynamic response to the perturbation.
1. Calculate the synchronous speed:
The synchronous speed (Ns) of a generator is given by the formula:
Ns = 120f / P
where f is the frequency (50 Hz) and P is the number of poles. Since the generator is synchronous, it has 2 poles, so P = 2.
Substituting the values, Ns = 120 * 50 / 2 = 3000 RPM.
2. Calculate the mechanical power:
The maximum power transfer capacity of the generator can be calculated using the formula:
Pmax = (E^2) / (2 * Xs)
where E is the no-load voltage of the generator (1.1 pu) and Xs is the pu reactance of the generator (j0.3 pu).
Substituting the values, Pmax = (1.1^2) / (2 * 0.3) = 2.42 pu.
The generator is loaded to 75% of its maximum power transfer capacity, so the actual power output is:
Pactual = 0.75 * Pmax = 0.75 * 2.42 pu = 1.815 pu.
3. Calculate the power angle:
The power angle (delta) can be calculated using the formula:
Pactual = E * E' * sin(delta) / Xs
where E' is the infinite bus voltage (1.0 pu). Rearranging the formula, we get:
delta = arcsin((Pactual * Xs) / (E * E'))
Substituting the values, delta = arcsin((1.815 * 0.3) / (1.1 * 1.0)) = arcsin(0.4945) = 29.66 degrees.
4. Calculate the new frequency:
The new frequency (f') can be calculated using the formula:
f' = (Ns - delta) / 120
Substituting the values, f' = (3000 - 29.66) / 120 = 24.25 Hz.
However, this is not the correct answer. The given answer is 1.39 Hz. This discrepancy may be due to an error in the calculations or the question statement. Please double-check the provided values and calculations to ensure accuracy.
Note: In this explanation, 'pu' refers to per unit, which is a relative unit of measurement commonly used in power systems analysis.
1. Calculate the synchronous speed:
The synchronous speed (Ns) of a generator is given by the formula:
Ns = 120f / P
where f is the frequency (50 Hz) and P is the number of poles. Since the generator is synchronous, it has 2 poles, so P = 2.
Substituting the values, Ns = 120 * 50 / 2 = 3000 RPM.
2. Calculate the mechanical power:
The maximum power transfer capacity of the generator can be calculated using the formula:
Pmax = (E^2) / (2 * Xs)
where E is the no-load voltage of the generator (1.1 pu) and Xs is the pu reactance of the generator (j0.3 pu).
Substituting the values, Pmax = (1.1^2) / (2 * 0.3) = 2.42 pu.
The generator is loaded to 75% of its maximum power transfer capacity, so the actual power output is:
Pactual = 0.75 * Pmax = 0.75 * 2.42 pu = 1.815 pu.
3. Calculate the power angle:
The power angle (delta) can be calculated using the formula:
Pactual = E * E' * sin(delta) / Xs
where E' is the infinite bus voltage (1.0 pu). Rearranging the formula, we get:
delta = arcsin((Pactual * Xs) / (E * E'))
Substituting the values, delta = arcsin((1.815 * 0.3) / (1.1 * 1.0)) = arcsin(0.4945) = 29.66 degrees.
4. Calculate the new frequency:
The new frequency (f') can be calculated using the formula:
f' = (Ns - delta) / 120
Substituting the values, f' = (3000 - 29.66) / 120 = 24.25 Hz.
However, this is not the correct answer. The given answer is 1.39 Hz. This discrepancy may be due to an error in the calculations or the question statement. Please double-check the provided values and calculations to ensure accuracy.
Note: In this explanation, 'pu' refers to per unit, which is a relative unit of measurement commonly used in power systems analysis.
|
Explore Courses for Electrical Engineering (EE) exam
|
|
Similar Electrical Engineering (EE) Doubts
Top Courses for Electrical Engineering (EE)View all
Question Description
A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer?.
A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer?.
Solutions for A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electrical Engineering (EE).
Download more important topics, notes, lectures and mock test series for Electrical Engineering (EE) Exam by signing up for free.
Here you can find the meaning of A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer?, a detailed solution for A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? has been provided alongside types of A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A 50 Hz synchronous generator is connected to an infinite bus through a line. The pu reactance of the generator and the line are j0.3 pu and j0.2 pu respectively. The generator no load voltage is 1.1 pu and the infinite bus is 1.0 pu. The inertia constant of Generator is 3 MW sec/MVA.If the generator is loaded to 75% of its maximum power transfer capacity and a small perturbation is given the new natural frequency of oscillation is ____________ Hz.Correct answer is '1.39'. Can you explain this answer? tests, examples and also practice Electrical Engineering (EE) tests.
|
|
Explore Courses for Electrical Engineering (EE) exam
|
|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up
within 7 days!
within 7 days!
Takes less than 10 seconds to signup