JEE Exam > JEE Questions > In an ellipse the locus of point of intersect...
Start Learning for Free
In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, is
- a)corresponding directrix
- b)latus rectum
- c)director circle
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
In an ellipse the locus of point of intersection of the perpendicular ...
Any point on the ellipse 
can be taken as P(a cosθ, b sinθ)
The equation of tangent at point P is
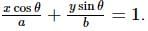
The equation of line perpendicular to tangent is
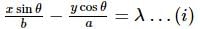
Since, the equation (i) passes through the focus (ae,0), then we get,
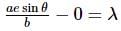
Thus, the equation (i) becomes
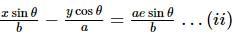
Now, the equation of line joining centre and point of contact (a cos θ, b sin θ) is
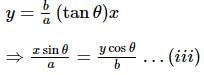
Solving the equation (ii) & (iii), we get the point of intersection Q whose abscissa is a/e.
Hence, Q lies on the corresponding directrix x = a/e.
can be taken as P(a cosθ, b sinθ)The equation of tangent at point P is
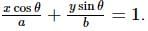
The equation of line perpendicular to tangent is
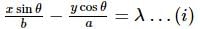
Since, the equation (i) passes through the focus (ae,0), then we get,
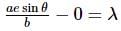
Thus, the equation (i) becomes
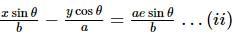
Now, the equation of line joining centre and point of contact (a cos θ, b sin θ) is
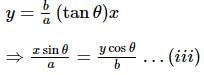
Solving the equation (ii) & (iii), we get the point of intersection Q whose abscissa is a/e.
Hence, Q lies on the corresponding directrix x = a/e.
Free Test
FREE
| Start Free Test |
Community Answer
In an ellipse the locus of point of intersection of the perpendicular ...
Understanding the Locus in an Ellipse
In an ellipse, certain geometric properties lead to interesting loci of points related to its foci and tangents. Let's explore why the locus of the intersection of the perpendicular from a focus to any tangent and the line connecting the center to the point of contact corresponds to the directrix.
Key Concepts:
- Ellipse Definition: An ellipse is defined as the set of all points where the sum of the distances from two fixed points (foci) is constant.
- Focus and Directrix: Each focus has an associated directrix. For an ellipse, the directrix serves as a guiding line that helps define the shape and properties of the ellipse.
Geometric Construction:
- Tangent Line: At any point on the ellipse, you can draw a tangent line.
- Perpendicular from Focus: From a focus, you can drop a perpendicular line to the tangent.
- Connecting Line: The line that connects the center of the ellipse (the midpoint between the foci) to the point where the tangent touches the ellipse is also drawn.
Locus of Intersection:
- Intersection Point: The intersection of the perpendicular from the focus and the line from the center to the point of contact is of interest.
- Resulting Locus: As the tangent moves around the ellipse, the intersection point traces out a straight line, which is precisely the directrix associated with the corresponding focus.
Conclusion:
- The intersection point's trajectory aligns with the directrix, confirming that the correct answer to the question is indeed option 'A', the corresponding directrix. This relationship showcases the elegant symmetry and properties inherent in conic sections, particularly ellipses.
In an ellipse, certain geometric properties lead to interesting loci of points related to its foci and tangents. Let's explore why the locus of the intersection of the perpendicular from a focus to any tangent and the line connecting the center to the point of contact corresponds to the directrix.
Key Concepts:
- Ellipse Definition: An ellipse is defined as the set of all points where the sum of the distances from two fixed points (foci) is constant.
- Focus and Directrix: Each focus has an associated directrix. For an ellipse, the directrix serves as a guiding line that helps define the shape and properties of the ellipse.
Geometric Construction:
- Tangent Line: At any point on the ellipse, you can draw a tangent line.
- Perpendicular from Focus: From a focus, you can drop a perpendicular line to the tangent.
- Connecting Line: The line that connects the center of the ellipse (the midpoint between the foci) to the point where the tangent touches the ellipse is also drawn.
Locus of Intersection:
- Intersection Point: The intersection of the perpendicular from the focus and the line from the center to the point of contact is of interest.
- Resulting Locus: As the tangent moves around the ellipse, the intersection point traces out a straight line, which is precisely the directrix associated with the corresponding focus.
Conclusion:
- The intersection point's trajectory aligns with the directrix, confirming that the correct answer to the question is indeed option 'A', the corresponding directrix. This relationship showcases the elegant symmetry and properties inherent in conic sections, particularly ellipses.
Attention JEE Students!
To make sure you are not studying endlessly, EduRev has designed JEE study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in JEE.
|
Explore Courses for JEE exam
|
|
Top Courses for JEEView all
In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer?
Question Description
In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer?.
In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer?.
Solutions for In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In an ellipse the locus of point of intersection of the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact, isa)corresponding directrixb)latus rectumc)director circled)none of theseCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice JEE tests.
|
|
Explore Courses for JEE exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup