Class 7 Exam > Class 7 Questions > A scooterist travels at 30 km/h along straigh...
Start Learning for Free
A scooterist travels at 30 km/h along straight path for 20 min. What is distance?
- a)10 km
- b)1.5 km
- c)6 km
- d)90 km
Correct answer is option 'A'. Can you explain this answer?
Verified Answer
A scooterist travels at 30 km/h along straight path for 20 min. What i...
If the scooter is travelling at precisely 30km/h in a perfectly straight line.
We know 1 hour=60 minutes
Therefore to calculate the distance traveled in the started fraction of the hour.
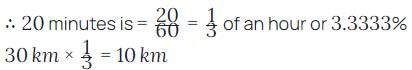
Therefore 10km is the distance traveled in 20 minutes of travelling at a speed of 30km/h
We know 1 hour=60 minutes
Therefore to calculate the distance traveled in the started fraction of the hour.
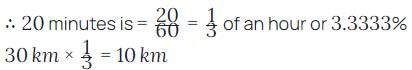
Therefore 10km is the distance traveled in 20 minutes of travelling at a speed of 30km/h
Most Upvoted Answer
A scooterist travels at 30 km/h along straight path for 20 min. What i...
Explanation:
20m = 20/60hr = 1/3hr
3. Use the formula:
Distance = Speed x Time = 30km/h x 1/3hr
Distance = 30km x 1/3 = 10km
To solve this problem, we'll use the formula for distance:
Distance = Speed x Time
Step-by-Step Explanation:
1. - Speed = 30 km/h
- Time = 20 minutes
- Time = 20 minutes
2. Convert time to hours:
Since speed is in kilometers per hour (km/h), we need to convert the time from minutes to hours.
20m = 20/60hr = 1/3hr
3. Use the formula:
Distance = Speed x Time = 30km/h x 1/3hr
4. Calculate the distance:
Distance = 30km x 1/3 = 10km
Final Answer:
The scooterist travels a distance of 10 kilometers.
Free Test
FREE
| Start Free Test |
Community Answer
A scooterist travels at 30 km/h along straight path for 20 min. What i...
To determine the distance traveled by the scooterist, we can use the formula distance = speed × time.
Given:
Speed = 30 km/h
Time = 20 min
Converting the time to hours:
20 min = 20/60 = 1/3 hours
The formula for distance can be modified to:
Distance = Speed × Time
Substituting the given values:
Distance = 30 km/h × 1/3 hours
Simplifying:
Distance = (30 × 1/3) km
Distance = 10 km
Therefore, the distance traveled by the scooterist is 10 km.
Explanation:
The formula distance = speed × time is commonly used to calculate the distance traveled when the speed and time are known. In this case, the scooterist's speed is given as 30 km/h, and the time is given as 20 minutes.
To use the formula, we convert the time to hours by dividing it by 60 (since there are 60 minutes in an hour). In this case, 20 minutes is equal to 1/3 hours.
Substituting the values into the formula, we multiply the speed (30 km/h) by the time (1/3 hours) to find the distance.
After simplifying the equation, we find that the distance traveled by the scooterist is 10 km.
Therefore, the correct answer is option A) 10 km.
Given:
Speed = 30 km/h
Time = 20 min
Converting the time to hours:
20 min = 20/60 = 1/3 hours
The formula for distance can be modified to:
Distance = Speed × Time
Substituting the given values:
Distance = 30 km/h × 1/3 hours
Simplifying:
Distance = (30 × 1/3) km
Distance = 10 km
Therefore, the distance traveled by the scooterist is 10 km.
Explanation:
The formula distance = speed × time is commonly used to calculate the distance traveled when the speed and time are known. In this case, the scooterist's speed is given as 30 km/h, and the time is given as 20 minutes.
To use the formula, we convert the time to hours by dividing it by 60 (since there are 60 minutes in an hour). In this case, 20 minutes is equal to 1/3 hours.
Substituting the values into the formula, we multiply the speed (30 km/h) by the time (1/3 hours) to find the distance.
After simplifying the equation, we find that the distance traveled by the scooterist is 10 km.
Therefore, the correct answer is option A) 10 km.
|
Explore Courses for Class 7 exam
|
|
Top Courses for Class 7View all
A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer?
Question Description
A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? for Class 7 2025 is part of Class 7 preparation. The Question and answers have been prepared according to the Class 7 exam syllabus. Information about A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Class 7 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer?.
A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? for Class 7 2025 is part of Class 7 preparation. The Question and answers have been prepared according to the Class 7 exam syllabus. Information about A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Class 7 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer?.
Solutions for A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 7.
Download more important topics, notes, lectures and mock test series for Class 7 Exam by signing up for free.
Here you can find the meaning of A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A scooterist travels at 30 km/h along straight path for 20 min. What is distance?a)10 kmb)1.5 kmc)6 kmd)90 kmCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice Class 7 tests.
|
|
Explore Courses for Class 7 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up
within 7 days!
within 7 days!
Takes less than 10 seconds to signup