UGC NET Exam > UGC NET Questions > A one-dimensional potential has the following...
Start Learning for Free
A one-dimensional potential has the following form:
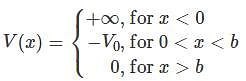
where V0 and b are positive constants.
Find V0 as a function of b such that there is just one bound state, of about zero binding energy, for a particle of mass M.
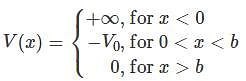
where V0 and b are positive constants.
Find V0 as a function of b such that there is just one bound state, of about zero binding energy, for a particle of mass M.
- a)
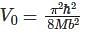
- b)
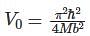
- c)
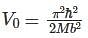
- d)
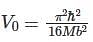
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
A one-dimensional potential has the following form:whereV0and bare pos...
To solve the bound state problem, we require that −V0<E<0. The relevant boundary conditions are ψ(0)=0, due to the infinitely high barrier at x=0 and limx→∞ψ(x)=0. The solution to the time-independent Schrödinger equation is:
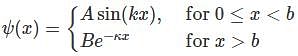
where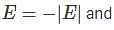
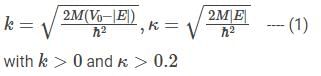
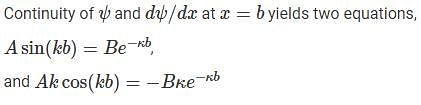
Diving these two equations, we obtain,
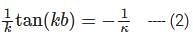
Note that equation (1) implies that,
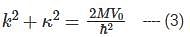
We can also rewrite equations (2) and (3) as,
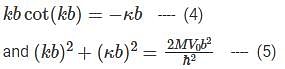
We require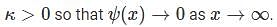
The requirement of k > 0 is conventional, since the sign of k can be absorbed into the definition of the constant A.
Equations (4) and (5) can be solved graphically by looking for intersection of the function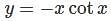
with the circle of radius 
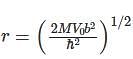in the first quadrant of the xy plane (where x=kb and y=kb ).
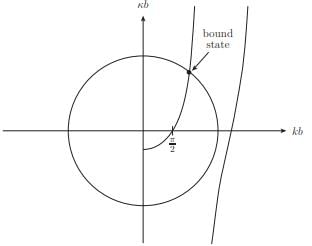
It is clear from the figure above that if V0 is less than some critical value, then the radius of the circle,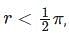
in which case there are no intersections in the first quadrant, and therefore no bound states. The critical value of of V0 is obtained by setting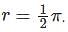 
That is,
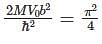
which yields,
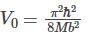
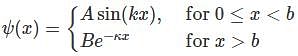
where
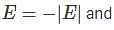
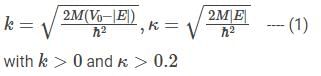
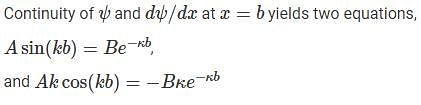
Diving these two equations, we obtain,
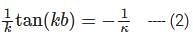
Note that equation (1) implies that,
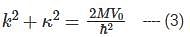
We can also rewrite equations (2) and (3) as,
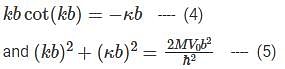
We require
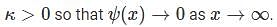The requirement of k > 0 is conventional, since the sign of k can be absorbed into the definition of the constant A.Equations (4) and (5) can be solved graphically by looking for intersection of the function
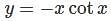with the circle of radius 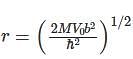in the first quadrant of the xy plane (where x=kb and y=kb ).It is clear from the figure above that if V0 is less than some critical value, then the radius of the circle,
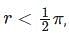in which case there are no intersections in the first quadrant, and therefore no bound states. The critical value of of V0 is obtained by setting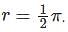 That is,
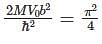
which yields,
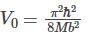
Attention UGC NET Students!
To make sure you are not studying endlessly, EduRev has designed UGC NET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in UGC NET.
|
Explore Courses for UGC NET exam
|
|
Top Courses for UGC NETView all
A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer?
Question Description
A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? for UGC NET 2024 is part of UGC NET preparation. The Question and answers have been prepared according to the UGC NET exam syllabus. Information about A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for UGC NET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer?.
A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? for UGC NET 2024 is part of UGC NET preparation. The Question and answers have been prepared according to the UGC NET exam syllabus. Information about A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for UGC NET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer?.
Solutions for A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for UGC NET.
Download more important topics, notes, lectures and mock test series for UGC NET Exam by signing up for free.
Here you can find the meaning of A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer?, a detailed solution for A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A one-dimensional potential has the following form:whereV0and bare positive constants.Find V0as a function of bsuch that there is just one bound state, of about zero binding energy, for a particle of mass M.a)b)c)d)Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice UGC NET tests.
|
|
Explore Courses for UGC NET exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup