Class 12 Exam > Class 12 Questions > Can you explain the answer of this question b...
Start Learning for Free
Can you explain the answer of this question below:
In hexagonal close packing of sphere in three dimensions.
- A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.
- B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.
- C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.
- D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.
The answer is b.
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
Can you explain the answer of this question below:In hexagonal close p...
(B)
HCP = AB AB AB . pattem repeat For calculating voids between two layers A and B. Total tetrahedral voids = 12 (represented by dots) out of which 8 are completely inside but rest are shared by other unit cells. Total octahedral voids = 6 (represented by cross). All are completely inside.
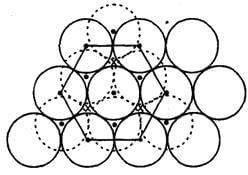
HCP = AB AB AB . pattem repeat For calculating voids between two layers A and B. Total tetrahedral voids = 12 (represented by dots) out of which 8 are completely inside but rest are shared by other unit cells. Total octahedral voids = 6 (represented by cross). All are completely inside.
Free Test
FREE
| Start Free Test |
Community Answer
Can you explain the answer of this question below:In hexagonal close p...
Hexagonal Close Packing (HCP)
Hexagonal close packing is a type of crystal structure found in some metals and non-metals, where the spheres (atoms or ions) are arranged in a closely packed hexagonal lattice. In HCP, each sphere has 12 closest neighbors, forming a regular pattern. Understanding the arrangement of voids within the unit cell is crucial in studying the crystal structure.
Explanation of the Answer
Statement A: In one unit cell, there are 12 octahedral voids, and all are completely inside the unit cell.
This statement is incorrect because the number of octahedral voids in a unit cell of HCP is not 12. Therefore, option A is not the correct answer.
Statement B: In one unit cell, there are six octahedral voids, and all are completely inside the unit cell.
This statement is correct. The HCP crystal structure consists of a close-packed array of spheres, where each sphere is in contact with six neighboring spheres. Between these spheres, there are voids known as octahedral voids. In HCP, there are six octahedral voids per unit cell, and all of them are completely inside the unit cell. Therefore, option B is the correct answer.
Statement C: In one unit cell, there are six octahedral voids, of which three are completely inside the unit cell, and the other three are partially inside the unit cell.
This statement is incorrect because all six octahedral voids in HCP are completely inside the unit cell, not partially. Thus, option C is not the correct answer.
Statement D: In one unit cell, there are 12 tetrahedral voids, all of which are completely inside the unit cell.
This statement is incorrect because HCP does not have tetrahedral voids. Tetrahedral voids are found in a different crystal structure called face-centered cubic (FCC). Therefore, option D is not the correct answer.
Conclusion
Based on the given statements, the correct answer is option B. In one unit cell of hexagonal close packing, there are six octahedral voids, and all of them are completely inside the unit cell.
Hexagonal close packing is a type of crystal structure found in some metals and non-metals, where the spheres (atoms or ions) are arranged in a closely packed hexagonal lattice. In HCP, each sphere has 12 closest neighbors, forming a regular pattern. Understanding the arrangement of voids within the unit cell is crucial in studying the crystal structure.
Explanation of the Answer
Statement A: In one unit cell, there are 12 octahedral voids, and all are completely inside the unit cell.
This statement is incorrect because the number of octahedral voids in a unit cell of HCP is not 12. Therefore, option A is not the correct answer.
Statement B: In one unit cell, there are six octahedral voids, and all are completely inside the unit cell.
This statement is correct. The HCP crystal structure consists of a close-packed array of spheres, where each sphere is in contact with six neighboring spheres. Between these spheres, there are voids known as octahedral voids. In HCP, there are six octahedral voids per unit cell, and all of them are completely inside the unit cell. Therefore, option B is the correct answer.
Statement C: In one unit cell, there are six octahedral voids, of which three are completely inside the unit cell, and the other three are partially inside the unit cell.
This statement is incorrect because all six octahedral voids in HCP are completely inside the unit cell, not partially. Thus, option C is not the correct answer.
Statement D: In one unit cell, there are 12 tetrahedral voids, all of which are completely inside the unit cell.
This statement is incorrect because HCP does not have tetrahedral voids. Tetrahedral voids are found in a different crystal structure called face-centered cubic (FCC). Therefore, option D is not the correct answer.
Conclusion
Based on the given statements, the correct answer is option B. In one unit cell of hexagonal close packing, there are six octahedral voids, and all of them are completely inside the unit cell.
|
Explore Courses for Class 12 exam
|
|
Similar Class 12 Doubts
Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b.
Question Description
Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b..
Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b..
Solutions for Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. defined & explained in the simplest way possible. Besides giving the explanation of
Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b., a detailed solution for Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. has been provided alongside types of Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. theory, EduRev gives you an
ample number of questions to practice Can you explain the answer of this question below:In hexagonal close packing of sphere in three dimensions.A:In one unit cell there are 12 octahedral voids and all are completely inside the unit cell.B:In one unit cell there are six octahedral voids and all are completely inside the unit cell.C:In one unit cell there are six octahedral void and of which three are completely inside the unit cell and other three are partially inside the unit cell.D:In one unit cell there are 12 tetrahedral voids, all are completely inside the unit cell.The answer is b. tests, examples and also practice Class 12 tests.
|
|
Explore Courses for Class 12 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup