CA Foundation Exam > CA Foundation Questions > The regression coefficients remain unchanged ...
Start Learning for Free
The regression coefficients remain unchanged due to a
- a)Shift of origin
- b)Shift of scale
- c)Both (a) and (b)
- d)(a) or (b)
Correct answer is option 'A'. Can you explain this answer?
Verified Answer
The regression coefficients remain unchanged due to aa)Shift of origin...
The regression coefficients remain unchanged due to a shift of origin but change due to a shift of scale. This property states that if the original pair of variables is (x, y) and if they are changed to the pair (u, v) where
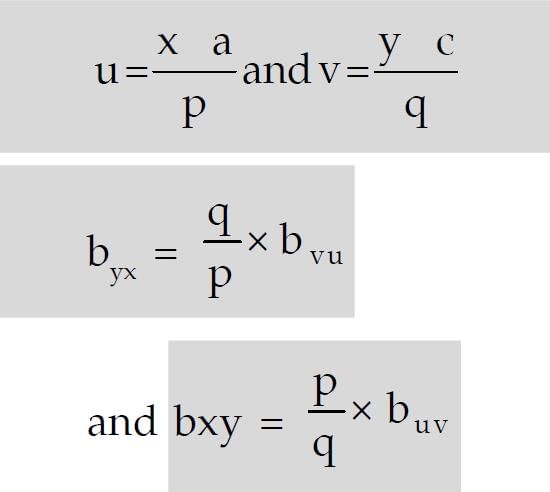
View all questions of this test
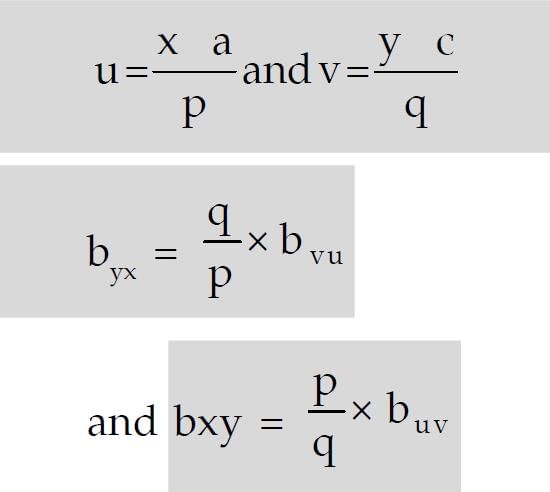
(ii) The two lines of regression intersect at the point
(mea of "x", mean of "y"),
where x and y are the variables under consideration.
(iii) The coefficient of correlation between two variables x and y in the simple geometric mean of the two regression coefficients. The sign of the correlation coefficient would be the common sign of the two regression coefficients.
Most Upvoted Answer
The regression coefficients remain unchanged due to aa)Shift of origin...
The correct answer is option 'A', which states that the regression coefficients remain unchanged due to a shift of origin. In this explanation, we will discuss why the other options are incorrect and provide a detailed understanding of why the regression coefficients remain unaffected by a shift of origin.
Explanation:
1. Shift of Origin:
- A shift of origin refers to a change in the position of the coordinate system used to represent the data.
- When a shift of origin occurs, the entire dataset is moved by a fixed amount in both the x-axis and y-axis directions.
- However, the regression line represents the relationship between the variables, and the coefficients of the regression equation capture this relationship.
- The regression coefficients are calculated based on the relative positions and relationships of the data points, rather than their absolute positions.
- Therefore, a shift of origin does not affect the slope or intercept of the regression line, and the regression coefficients remain unchanged.
2. Shift of Scale:
- A shift of scale refers to a change in the units or measurement scale of the variables.
- For example, converting the variable from inches to centimeters or from meters to kilometers.
- When a shift of scale occurs, the regression coefficients will be affected as they are based on the units of measurement.
- The slope of the regression line represents the change in the dependent variable for a one-unit change in the independent variable.
- If the scale of the independent variable changes, the slope will also change proportionally, and hence, the regression coefficients will be affected.
3. Both (a) and (b):
- This option suggests that both a shift of origin and a shift of scale can affect the regression coefficients.
- However, as explained above, a shift of origin does not affect the regression coefficients, only a shift of scale does.
- Therefore, this option is incorrect.
4. (a) or (b):
- This option suggests that either a shift of origin or a shift of scale can affect the regression coefficients.
- However, as discussed earlier, a shift of origin does not affect the regression coefficients.
- Therefore, this option is also incorrect.
In conclusion, the correct answer is option 'A' because the regression coefficients remain unchanged due to a shift of origin.
Explanation:
1. Shift of Origin:
- A shift of origin refers to a change in the position of the coordinate system used to represent the data.
- When a shift of origin occurs, the entire dataset is moved by a fixed amount in both the x-axis and y-axis directions.
- However, the regression line represents the relationship between the variables, and the coefficients of the regression equation capture this relationship.
- The regression coefficients are calculated based on the relative positions and relationships of the data points, rather than their absolute positions.
- Therefore, a shift of origin does not affect the slope or intercept of the regression line, and the regression coefficients remain unchanged.
2. Shift of Scale:
- A shift of scale refers to a change in the units or measurement scale of the variables.
- For example, converting the variable from inches to centimeters or from meters to kilometers.
- When a shift of scale occurs, the regression coefficients will be affected as they are based on the units of measurement.
- The slope of the regression line represents the change in the dependent variable for a one-unit change in the independent variable.
- If the scale of the independent variable changes, the slope will also change proportionally, and hence, the regression coefficients will be affected.
3. Both (a) and (b):
- This option suggests that both a shift of origin and a shift of scale can affect the regression coefficients.
- However, as explained above, a shift of origin does not affect the regression coefficients, only a shift of scale does.
- Therefore, this option is incorrect.
4. (a) or (b):
- This option suggests that either a shift of origin or a shift of scale can affect the regression coefficients.
- However, as discussed earlier, a shift of origin does not affect the regression coefficients.
- Therefore, this option is also incorrect.
In conclusion, the correct answer is option 'A' because the regression coefficients remain unchanged due to a shift of origin.
Free Test
FREE
| Start Free Test |
Community Answer
The regression coefficients remain unchanged due to aa)Shift of origin...
C

|
Explore Courses for CA Foundation exam
|

|
Similar CA Foundation Doubts
The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer?
Question Description
The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? for CA Foundation 2025 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for CA Foundation 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer?.
The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? for CA Foundation 2025 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for CA Foundation 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer?.
Solutions for The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for CA Foundation.
Download more important topics, notes, lectures and mock test series for CA Foundation Exam by signing up for free.
Here you can find the meaning of The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer?, a detailed solution for The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The regression coefficients remain unchanged due to aa)Shift of originb)Shift of scalec)Both (a) and (b)d)(a) or (b)Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice CA Foundation tests.

|
Explore Courses for CA Foundation exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























