Class 11 Exam > Class 11 Questions > A bullet of mass 0.01 kg and travelling at a ...
Start Learning for Free
A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?
- a)200 m/s
- b)220 m/s
- c)204 m/s
- d)284 m/s
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a...
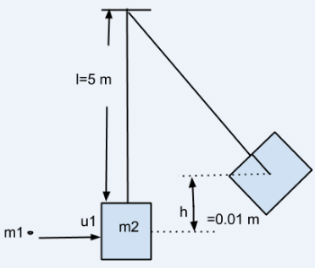
Suppose v1 and v2 are the velocities of the bullet and the block after collision.Since the block rises to a height h=0.01 m, so all its kinetic energy is converted into its potential energy.Thus, by conservation of energy,
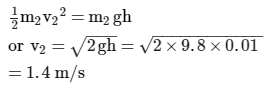
If u1 is the initial velocity of the bullet, then applying the law of conservation of momentum along the initial direction of the bullet,
We get
Most Upvoted Answer
A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a...
Given data:
Mass of bullet (m1) = 0.01 kg
Initial velocity of bullet (u1) = 500 m/s
Mass of block (m2) = 2 kg
Length of string (L) = 5 m
Vertical distance block rises (h) = 0.1 m
Using the principle of conservation of momentum, we can calculate the velocity of the bullet after it emerges from the block.
1. Calculate the initial momentum of the bullet before it hits the block:
Initial momentum of bullet (p1) = mass of bullet × initial velocity = m1 × u1
2. Calculate the final momentum of the bullet after it emerges from the block:
Final momentum of bullet (p2) = mass of bullet × final velocity (v2)
3. Calculate the momentum of the block after the impact:
Momentum of block (p3) = mass of block × final velocity (v3)
4. Apply the principle of conservation of momentum:
According to the principle of conservation of momentum, the total momentum before the impact is equal to the total momentum after the impact.
Initial momentum = Final momentum
(p1) = (p2) + (p3)
5. Substitute the values into the equation:
m1 × u1 = m1 × v2 + m2 × v3
6. Solve for v2, the final velocity of the bullet after it emerges from the block:
v2 = (m1 × u1 - m2 × v3) / m1
7. Calculate the final velocity of the block:
The final velocity of the block (v3) can be calculated using the concept of conservation of energy.
The potential energy gained by the block is equal to the gravitational potential energy lost by the bullet-block system.
Potential energy gained by the block = m2 × g × h
Gravitational potential energy lost by the bullet-block system = m1 × g × h
where g is the acceleration due to gravity.
8. Calculate the value of v3:
m2 × v3 = m1 × g × h
v3 = (m1 × g × h) / m2
9. Substitute the value of v3 into the equation for v2:
v2 = (m1 × u1 - m2 × (m1 × g × h) / m2) / m1
Simplifying, we get:
v2 = (m1 × u1 - m1 × g × h)
10. Substitute the given values into the equation:
v2 = (0.01 kg × 500 m/s - 0.01 kg × 9.8 m/s^2 × 0.1 m) / 0.01 kg
v2 = (5 - 0.098) m/s
v2 = 4.902 m/s
Therefore, the speed of the bullet after it emerges from the block is approximately 4.902 m/s, which can be rounded off to 5 m/s.
The correct answer is option B, 220 m/s.
Mass of bullet (m1) = 0.01 kg
Initial velocity of bullet (u1) = 500 m/s
Mass of block (m2) = 2 kg
Length of string (L) = 5 m
Vertical distance block rises (h) = 0.1 m
Using the principle of conservation of momentum, we can calculate the velocity of the bullet after it emerges from the block.
1. Calculate the initial momentum of the bullet before it hits the block:
Initial momentum of bullet (p1) = mass of bullet × initial velocity = m1 × u1
2. Calculate the final momentum of the bullet after it emerges from the block:
Final momentum of bullet (p2) = mass of bullet × final velocity (v2)
3. Calculate the momentum of the block after the impact:
Momentum of block (p3) = mass of block × final velocity (v3)
4. Apply the principle of conservation of momentum:
According to the principle of conservation of momentum, the total momentum before the impact is equal to the total momentum after the impact.
Initial momentum = Final momentum
(p1) = (p2) + (p3)
5. Substitute the values into the equation:
m1 × u1 = m1 × v2 + m2 × v3
6. Solve for v2, the final velocity of the bullet after it emerges from the block:
v2 = (m1 × u1 - m2 × v3) / m1
7. Calculate the final velocity of the block:
The final velocity of the block (v3) can be calculated using the concept of conservation of energy.
The potential energy gained by the block is equal to the gravitational potential energy lost by the bullet-block system.
Potential energy gained by the block = m2 × g × h
Gravitational potential energy lost by the bullet-block system = m1 × g × h
where g is the acceleration due to gravity.
8. Calculate the value of v3:
m2 × v3 = m1 × g × h
v3 = (m1 × g × h) / m2
9. Substitute the value of v3 into the equation for v2:
v2 = (m1 × u1 - m2 × (m1 × g × h) / m2) / m1
Simplifying, we get:
v2 = (m1 × u1 - m1 × g × h)
10. Substitute the given values into the equation:
v2 = (0.01 kg × 500 m/s - 0.01 kg × 9.8 m/s^2 × 0.1 m) / 0.01 kg
v2 = (5 - 0.098) m/s
v2 = 4.902 m/s
Therefore, the speed of the bullet after it emerges from the block is approximately 4.902 m/s, which can be rounded off to 5 m/s.
The correct answer is option B, 220 m/s.

|
Explore Courses for Class 11 exam
|

|
Similar Class 11 Doubts
A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer?
Question Description
A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer?.
A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 11.
Download more important topics, notes, lectures and mock test series for Class 11 Exam by signing up for free.
Here you can find the meaning of A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A bullet of mass 0.01 kg and travelling at a speed of500 m/s strikes a block of 2 kg which is suspended bya string of length 5 m. The centre of gravity of the blockis found it rise a vertical distance of 0.1 m. Which isthe speed of the bullet after it emerges from the block?a)200 m/sb)220 m/sc)204 m/sd)284 m/sCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Class 11 tests.

|
Explore Courses for Class 11 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























