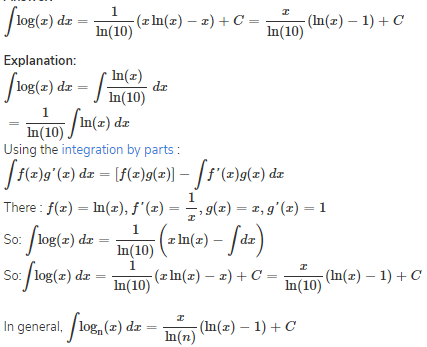CA Foundation Exam > CA Foundation Questions > The integration of log x DX=?
Start Learning for Free
The integration of log x DX=?
Community Answer
The integration of log x DX=?
Integration of log x
Integration is a fundamental concept in calculus that deals with finding the antiderivative of a given function. When it comes to integrating logarithmic functions, we need to use specific integration techniques based on the properties of logarithms.
Logarithmic properties
Before diving into the integration of log x, let's briefly review some important properties of logarithms:
1. Product rule: log(xy) = log(x) + log(y)
2. Quotient rule: log(x/y) = log(x) - log(y)
3. Power rule: log(x^n) = n*log(x)
4. Change of base formula: log_a(x) = log_b(x)/log_b(a)
These properties will come in handy when integrating logarithmic functions.
Integration of log x
The integral of log x, denoted as ∫log(x) dx, can be evaluated using a technique called integration by parts. This technique involves splitting the original function into two parts and applying a specific formula.
Integration by parts formula: ∫u dv = uv - ∫v du
Let's apply this formula to the integral of log x:
We choose:
u = log(x) (to differentiate)
dv = dx (to integrate)
Differentiating u:
du/dx = 1/x
Integrating dv:
v = x
Applying the integration by parts formula:
∫log(x) dx = x*log(x) - ∫x*(1/x) dx
= x*log(x) - ∫dx
= x*log(x) - x + C
Therefore, the integral of log x is x*log(x) - x + C, where C is the constant of integration.
Conclusion
The integration of log x can be evaluated using the integration by parts technique. By applying the formula and simplifying the resulting expression, we find that the integral of log x is x*log(x) - x + C. Understanding the properties of logarithms and the techniques of integration allows us to solve more complex problems involving logarithmic functions.
Integration is a fundamental concept in calculus that deals with finding the antiderivative of a given function. When it comes to integrating logarithmic functions, we need to use specific integration techniques based on the properties of logarithms.
Logarithmic properties
Before diving into the integration of log x, let's briefly review some important properties of logarithms:
1. Product rule: log(xy) = log(x) + log(y)
2. Quotient rule: log(x/y) = log(x) - log(y)
3. Power rule: log(x^n) = n*log(x)
4. Change of base formula: log_a(x) = log_b(x)/log_b(a)
These properties will come in handy when integrating logarithmic functions.
Integration of log x
The integral of log x, denoted as ∫log(x) dx, can be evaluated using a technique called integration by parts. This technique involves splitting the original function into two parts and applying a specific formula.
Integration by parts formula: ∫u dv = uv - ∫v du
Let's apply this formula to the integral of log x:
We choose:
u = log(x) (to differentiate)
dv = dx (to integrate)
Differentiating u:
du/dx = 1/x
Integrating dv:
v = x
Applying the integration by parts formula:
∫log(x) dx = x*log(x) - ∫x*(1/x) dx
= x*log(x) - ∫dx
= x*log(x) - x + C
Therefore, the integral of log x is x*log(x) - x + C, where C is the constant of integration.
Conclusion
The integration of log x can be evaluated using the integration by parts technique. By applying the formula and simplifying the resulting expression, we find that the integral of log x is x*log(x) - x + C. Understanding the properties of logarithms and the techniques of integration allows us to solve more complex problems involving logarithmic functions.

|
Explore Courses for CA Foundation exam
|

|
Similar CA Foundation Doubts
The integration of log x DX=?
Question Description
The integration of log x DX=? for CA Foundation 2025 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about The integration of log x DX=? covers all topics & solutions for CA Foundation 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The integration of log x DX=?.
The integration of log x DX=? for CA Foundation 2025 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about The integration of log x DX=? covers all topics & solutions for CA Foundation 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The integration of log x DX=?.
Solutions for The integration of log x DX=? in English & in Hindi are available as part of our courses for CA Foundation.
Download more important topics, notes, lectures and mock test series for CA Foundation Exam by signing up for free.
Here you can find the meaning of The integration of log x DX=? defined & explained in the simplest way possible. Besides giving the explanation of
The integration of log x DX=?, a detailed solution for The integration of log x DX=? has been provided alongside types of The integration of log x DX=? theory, EduRev gives you an
ample number of questions to practice The integration of log x DX=? tests, examples and also practice CA Foundation tests.

|
Explore Courses for CA Foundation exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.