Class 10 Maths Chapter 11 Case Based Questions - Area Related to Circles
Study Case - 1
A farmer has a rectangular field of length 30m and breadth 15m. By the farmer a pit of diameter 7m is dug 12m deep for rain water harvesting. The earth taken out is spread in the field.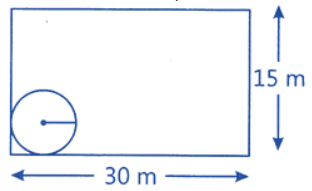 Based on the above information, answer the following questions:
Based on the above information, answer the following questions:
Q1: Find the volume of the earth taken out.
(a) 460m3
(b) 462m3
(c) 465m3
(d) 468m3
Ans: (b)
Explanation: Volume of the earth taken out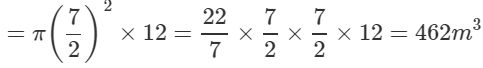
Q2: The area of the rectangular field is
(a) 420m2
(b) 430m2
(c) 440m2
(d) 450m2
Ans: (b)
Explanation: Volume of the earth taken out
Area of the rectangular field = 30 × 15 = 450m2
Q3: Find the area of the top of the pit.
(a) 38.5m2
(b) 40.5m2
(c) 41.5m2
(d) None of these
Ans: (a)
Explanation: Area of top of the pit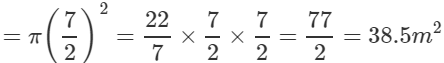
Q4: The area of the remaining field is
(a) 402.3m2
(b) 405m2
(c) 410m2
(d) 411.5m2
Ans: (d)
Explanation: Area of the remaining field = Area of rectangular field - area of top of pit = 450 − 38.5 − 411.5m2.
Q5: Find the level rise in the field.
(a) 0.5m
(b) 3m
(c) 1.12 m
(d) 2.12 m
Ans: (c)
Explanation: The rise in the level of field = 462/4115 = 112m.
Study Case - 2
Kritika bought a pendulum clock for her living room. The clock contains a small pendulum of length 15 cm. The minute hand and hour hand of the clock are 9 cm and 6 cm long respectively.  Based on the above information, answer the following questions,
Based on the above information, answer the following questions,
Q1: Find the area swept by the minute hand in 10 minutes.
(a) 22.24 cm2
(b) 42.42 cm2
(c) 44 cm2
(d) 44.42 cm2
Ans: (b)
Explanation: Angles made by minutes hand in 60 minutes = 360∘
∴ Area made by minute hand in 10 minutes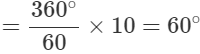
Length of minute hand = 9cm
∴ Area swept by minute hand in 10 minutes
= Area of sector having central angles 60∘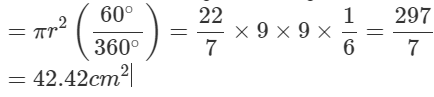
Q2: If the pendulum covers a distance of 22cm in the complete oscillation, then find the angles described by pendulum at the centre.
(a) 40∘
(b) 42∘
(c) 45∘
(d) 48∘
Ans: (b)
Explanation: 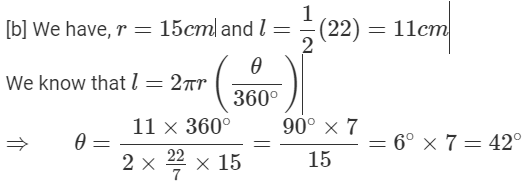
Q3: Find the angles described by hour hand in 10 minutes.
(a) 5∘
(b) 10∘
(c) 15∘
(d) 20∘
Ans: (a)
Explanation: Angle made by hour in 12 hours
∴ Angle made by hour hand in 10 minutes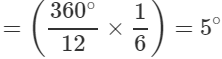
Q4: Find the area swept by the hour hand in 1 hour.
(a) 7.68 cm2
(b) 8.2 cm2
(c) 8.86 cm2
(d) 9.428 cm2
Ans: (d)
Explanation: Angle made by hour hand in 1 hour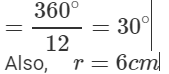
∴ Area swept made by hour hand in 1hour
= Area of sector having central angle 30∘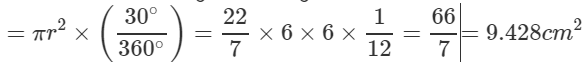
Q5: Find the area swept by the hour hand between11 a.m.and 5 p.m.
(a) 56.568 cm2
(b) 62 cm2
(c) 70 cm2
(d) 72 cm2
Ans: (a)
Explanation: Number of hours from 11 a.m. to 5 p.m. = 6
Area swept by hour hand in 1 hour = 9.428cm2
∴ Area swept by hour hand in 6 hours
= 9.428 × 6 = 56.568cm2
Study Case - 3
A man wanted attractive design on the front door of his house. So, he asked a painter to paint the design as per specifications provided by him. Four equal circles were inscribed inside a square PQRS of side 28 cm as shown in the figure below: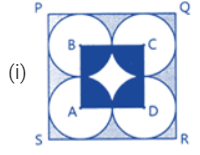
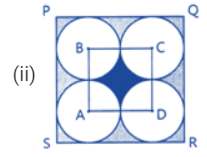
Q1: The diameter of each circle is:
(a) 3.5 cm
(b) 7 cm
(c) 12 cm
(d) 14 cm
Ans: (d)
Explanation: Side of square PQRS = 28cm.
∴ Diameter of each circle 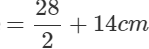
Q2: Area of smaller square ABCD formed by joining the centres A, B, C and D of the four circles, is
(a) 49 cm2
(b) 196 cm2
(c) 824 cm2
(d) 12.25 cm2
Ans: (b)
Explanation: We have. AB = radius of circle with centre A + Radius of circle with centre B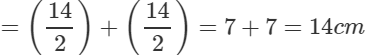
Length of each side of square ABCD = 14cm
∴ Area of square ABCD = (14)2 = 196cm2.
Q3: Refer to figure I area of the shaded region is:
(a) 154 cm2
(b) 308 cm2
(c) 616 cm2
(d) 77 cm2
Ans: (c)
Explanation: Area of the shaded portion region
= Area of four quadrants with centres A, B, C and D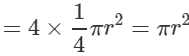
Q4: Area of the shaded portion in figure II, is:
(a) 154 cm2
(b) 66 cm2
(c) 42 cm2
(d) 21 cm2
Ans: (c)
Explanation: Area of shaded region A = Area of square ABCD
= 4 × Area of quadrants with radius 7cm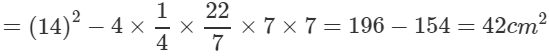
Q5: Cost of painting of the circular regions with centre A, B, C and D at Rs.5.00 per sq. cm.
(a) Rs.12320.00
(b) Rs.3080.00
(c) Rs.6160.00
(d) Rs.1540.00
Ans: (b)
Explanation: Radius of each circle =7cm
∴ Area of one circle
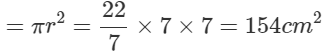
∴ Area of four such circles 4 × 154 = 616cm2
So, cost of painting the circles
= Rs.(5.00 × 616) = Rs.3080.00
|
29 videos|254 docs|56 tests
|
















