Chapter 3 - Pair Of Linear Equations In Two Variables, RD Sharma Solutions - (Part-18) | RD Sharma Solutions for Class 10 Mathematics PDF Download
Page No 3.79
Q.12. One says, "Give me a hundred, friend! I shall then become twice as rich as you." The other replies, "If you give me ten, I shall be six times as rich as you." Tell me what is the amount of their respective capital?
Ans. To find:
(1) Total amount of A.
(2) Total amount of B.
Suppose A has Rs x and B has Rs y
According to the given conditions,
x + 100 = 2(y − 100)
x + 100 = 2y − 200
x − 2y = −300 ....(1)
and
y + 10 = 6(x − 10)
y + 10 = 6x − 60
6x − y = 70 ....(2)
Multiplying equation (2) by 2 we get
12x − 2y = 140 ....(3)
Subtracting (1) from (3), we get
11x = 440
x = 40
Substituting the value of x in equation (1), we get
40 − 2y = −300
−2y = −340
y = 170
Hence A has x = Rs 40 and B has y = Rs 170
Q.13. A and B each have a certain number of mangoes. A says to B, "if you give 30 of your mangoes, I will have twice as many as left with you." B replies, "if you give me 10, I will have thrice as many as left with you." How many mangoes does each have?
Ans. To find:
(1) Total mangoes of A.
(2) Total mangoes of B.
Suppose A has x mangoes and B has y mangoes,
According to the given conditions,
x + 30 = 2(y - 30)
x + 30 = 2y - 60
x - 2y + 30 + 60 = 0
x - 2y + 90 = 0 ....(1)
y + 10 = 3(x - 10)
y + 0 = 3x - 30
y - 3x + 10 + 30 = 0
y - 3x + 40 = 0 ....(2)
Multiplying eq. 1 by (3),
3x + 6y + 270 = 0 …… (3) and
Now adding eq.2 and eq.3
5y = 310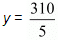
y = 62
x - 2 x 62 + 90 = 0
x - 124 + 90 = 0
x - 34 = 0
x = 34
Hence A has 34 mangoes and B has 62 mangoes.
Q.14. Vijay had some bananas , and he divided them into two lots A and B . He sold first lot at the rate of Rs. 2 for 3 bananas and the second lot at the rate of Rs.1 per banana and got a total of Rs. 400 . If he had sold the first lot at the rate of Rs.1 per banana and the second lot at the rate of Rs. 4 per five bananas, his total collection would have been Rs. 460 . Find the total number of bananas he had .
Ans. Let the bananas in lot A be x and that in lot B be y.
Vijay sold 3 bananas for Rs 2 in lot A.
So, the cost of x bananas in lot A =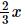
Now,
Cost of x bananas in lot A + Cost of y bananas in lot B = Rs. 400
∴ 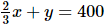
⇒ 2x + 3y = 1200 .....(i)
Now, if he sells the first lot at the rate of Rs 1 per banana and second for Rs 4 for 5 bananas, then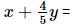 Rs. 460
Rs. 460
⇒ 5x + 4y = 2300 .....(ii)
Solving (i) and (ii), we get
x = 300 and y = 200
So, the total number of bananas = x + y = 300 + 200 = 500.
Page No 3.79
Q.15. On selling a T.V. at 5% gain and a fridge at 10% gain, a shopkeeper gains Rs 2000. But if he sells the T.V. at 10% gain the fridge at 5% loss. He gains Rs 1500 on the transaction. Find the actual prices of T.V. and fridge.
Ans. Given:
(i) On selling of a T.V. at 5% gain and a fridge at 10% gain, shopkeeper gain Rs.2000.
(ii) Selling T.V. at 10% gain and fridge at 5% loss. He gains Rs. 1500.
To find: Actual price of T.V. and fridge.
S.P. of T.V at 5% gain = 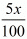
S.P. of T.V at 10% gain = 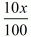
S.P. of Fridge at 5% gain =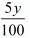
S.P. of Fridge at 10% gain = 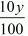
According to the question: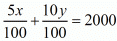
5x + 10y = 200000
x + 2y = 40000
x + 2y - 40000 = 0 .....(1)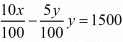
10x - 5y = 15000
2x - 1y = 30000
2x - 1y = 30000 = 0 .....(2)
Hence we got the pair of equations
1x + 2y − 40000 = 0 …… (1)
2x − 1y − 30000 = 0 …… (2)
Solving the equation by cross multiplication method;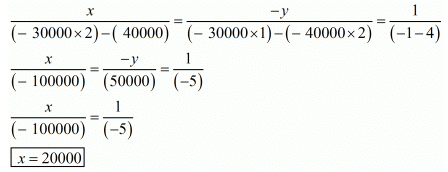
Cost of T.V. = 20000
Cost of fridge = 10000
Hence the cost of T.V. is Rs. 20000 and that of fridge is Rs. 10000
Page No 3.85
Q.1. The sum of two numbers is 8. If their sum is four times their difference, find the numbers.
Ans. Let the numbers are x and y. One of them must be greater than or equal to the other. Let us assume that x is greater than or equal to y.
The sum of the two numbers is 8. Thus, we have x + y = 8
The sum of the two numbers is four times their difference. Thus, we have
x + y = 4(x - y)
⇒ x + y = 4x - 4y
⇒ 4x - 4y - x - y = 0
⇒ 3x - 5y = 0
So, we have two equations
x + y = 8
3x - 5y = 0
Here x and y are unknowns. We have to solve the above equations for x and y.
Multiplying the first equation by 5 and then adding with the second equation, we have
5(x + y) + (3x - 5y) = 5 x 8 + 0
⇒ 5x + 5y + 3x - 5y = 40
⇒ 8x = 40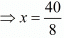
⇒ x = 5
Substituting the value of x in the first equation, we have
5 + y = 8
⇒ y = 8 - 5
⇒ y = 3
Hence, the numbers are 5 and 3.
Page No 3.85.
Q.2. The sum of digits of a two digit number is 13. If the number is subtracted from the one obtained by interchanging the digits, the result is 45. What is the number?
Ans. Let the digits at units and tens place of the given number be x and y respectively. Thus, the number is 10 y + x.
The sum of the digits of the number is 13. Thus, we have x + y = 13
After interchanging the digits, the number becomes 10 x + y.
The difference between the number obtained by interchanging the digits and the original number is 45. Thus, we have
(10x + y) - (10y + x) = 45
⇒ 0x + y - 10y + x = 45
⇒ 9x - 9y = 45
⇒ 9(x - y) = 45
So, we have two equations
x + y = 13
x - y = 5
Here x and y are unknowns. We have to solve the above equations for x and y.
Adding the two equations, we have
(x + y) + (x - y) = 13 + 5
⇒ x + y + x - y = 18
⇒ 2x = 18
⇒ x = 18/2
⇒ x = 9
Substituting the value of x in the first equation, we have
9 + y = 13
⇒ y = 13 - 9
⇒ y = 4
Hence, the number is 10 x 4 + 9 = 49.
Page No 3.86
Q.3. A number consist of two digits whose sum is five. When the digits are reversed, the number becomes greater by nine. Find the number.
Ans. Let the digits at units and tens place of the given number be x and y respectively. Thus, the number is 10y + x.
The sum of the digits of the number is 5. Thus, we have x + y = 5
After interchanging the digits, the number becomes 10x + y
The number obtained by interchanging the digits is greater by 9 from the original number. Thus, we have
10x + y = 10y + x + 9
⇒ 10x + y - 10y - x = 9
⇒ 9x - 9y = 9
⇒ 9(x - y) = 9
⇒ 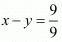
⇒ x - y = 1
So, we have two equations
x + y = 5
x - y = 1
Here x and y are unknowns. We have to solve the above equations for x and y.
Adding the two equations, we have
(x + y) + (x - y) = 5 + 1
⇒ x + y + x - y = 6
⇒ 2x = 6
⇒ x = 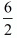
⇒ x = 3
Substituting the value of x in the first equation, we have
3 + y = 5
⇒ y = 5 - 3
⇒ y = 2
Hence, the number is 10 x 2 + 3 = 23
Page No 3.86.
Q.4. The sum of digits of a two number is 15. The number obtained by reversing the order of digits of the given number exceeds the given number by 9. Find the given number.
Ans. Let the digits at units and tens place of the given number be x and y respectively. Thus, the number is 10y + x.
The sum of the digits of the number is 15. Thus, we have x + y = 15
After interchanging the digits, the number becomes 10x + y.
The number obtained by interchanging the digits is exceeding by 9 from the original number. Thus, we have
10x + y = 10y + x + 9
⇒ 10x + y - 10y - x = 9
⇒ 9x - 9y = 9
⇒ 9(x - y) = 9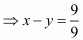
⇒ x - y = 1
So, we have two equations
x + y = 15
x - y = 1
Here x and y are unknowns. We have to solve the above equations for x and y.
Adding the two equations, we have
(x + y) + (x - y) = 15 + 1
⇒ x + y + x - y = 16
⇒ 2x = 16
⇒ x =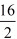
⇒ x = 8
Substituting the value of x in the first equation, we have
8 + y = 15
⇒ y = 15 - 8
⇒ y = 7
Hence, the number is 10 x 7 + 8 = 78
Page No 3.86
Q.5. The sum of a two-digit number and the number formed by reversing the order of digit is 66. If the two digits differ by 2, find the number. How many such numbers are there?
Ans. Let the digits at units and tens place of the given number be x and y respectively. Thus, the number is 10y + x.
The two digits of the number are differing by 2. Thus, we have x - y = ± 2
After interchanging the digits, the number becomes 10x + y.
The sum of the numbers obtained by interchanging the digits and the original number is 66. Thus, we have
(10x + y) + (10y + x) = 66
⇒ 10x + y + 10y + x = 66
⇒ 11x + 11y = 66
⇒ 11(x + y) = 66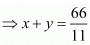
⇒ x + y = 6
So, we have two systems of simultaneous equations
x - y = 2
x + y = 6
x - y = - 2
x + y = 6
Here x and y are unknowns. We have to solve the above systems of equations for x and y.
(i) First, we solve the system
x - y = 2,
x + y = 6
Adding the two equations, we have
(x - y) + (x + y) = 2 + 6
⇒ x - y + x + y = 8
⇒ 2x = 8
⇒ 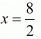
⇒ x = 4
Substituting the value of x in the first equation, we have
4 - y = 2
⇒ y = 4 - 2
⇒ y = 2
Hence, the number is 10 x 2 + 4 = 24
(ii) Now, we solve the system
x - y = - 2,
x + y = 6
Adding the two equations, we have
(x - y) + (x + y) = -2 + 6
⇒ x - y + x + y = 4
⇒ 2x = 4
⇒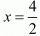
⇒ x = 2
Substituting the value of x in the first equation, we have
2 - y = - 2
⇒ y = 2 + 2
⇒ y = 4
Hence, the number is 10 x 4 + 2 = 42
There are two such numbers.
FAQs on Chapter 3 - Pair Of Linear Equations In Two Variables, RD Sharma Solutions - (Part-18) - RD Sharma Solutions for Class 10 Mathematics
| 1. What are the properties of a pair of linear equations in two variables? |  |
| 2. How can we solve a pair of linear equations in two variables using the substitution method? |  |
| 3. Can a pair of linear equations in two variables have no solution? |  |
| 4. How do we determine the number of solutions for a pair of linear equations in two variables graphically? |  |
| 5. What is the importance of solving a pair of linear equations in two variables in real-life scenarios? |  |






















