Some Applications of Trigonometry Class 10 Notes Maths Chapter 9
| Table of contents |

|
| Introduction |

|
| Heights and Distances |

|
| Solved Examples |

|
| (i) Angle of Elevation |

|
| (ii) Calculating heights |

|
| (iii) Calculating lengths |

|
Introduction
Trigonometry, the branch of mathematics dealing with the relationships between the angles and sides of triangles, has several practical applications in various fields.
This chapter discusses the practical applications of trigonometry in everyday life. In trigonometry, we measure sides of triangle, when particular angle is given.
Heights and Distances
Most the buildings, walls, towers we see around are perpendicular to ground. This chapter deals with measurement of heights and distance from certain points with the help of trigonometry if particular angles are known.
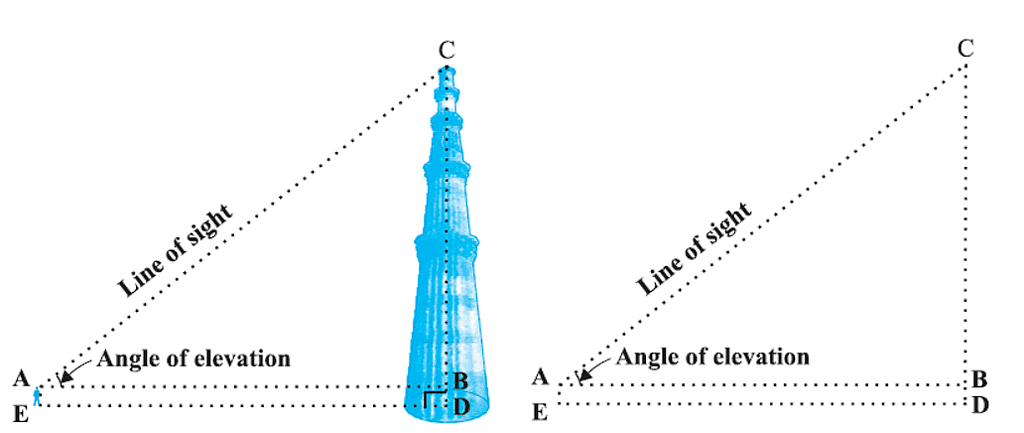
- In the diagram, the line AC from the student's eye to the top of a Minar is the line of sight. The angle BAC between the line of sight and the horizontal is the angle of elevation.
- The line of sight is drawn from an observer's eye to the object they're looking at.

- Angle of elevation is the angle between the line of sight and the horizontal when viewing an object above eye level.
- Angle of depression is the angle between the line of sight and the horizontal when viewing an object below eye level.
Applications of Trigonometry Concepts
(i) Height and Distance Calculations :- Finding the height of a building using its shadow and the angle of elevation of the sun.
(ii) Navigation and Surveying:-Calculating distances between points or the height of landmarks using angles measured from different locations.
(iii) Architecture and Engineering:-Determining structural angles or dimensions in construction projects.
(iv) Astronomy:-Calculating the distance between celestial bodies or their angles in the sky.
(v) Physics Problems:-Analyzing projectile motion, where trigonometric functions help in resolving velocity components.
(vi) Computer Graphics:-Using trigonometry to rotate or scale images in graphics programming.
Solved Examples
(i) Angle of Elevation
Q1: If a tower 30m high casts a shadow 10/√3m long on the ground then, what is the angle of elevation of sun?Solution:
Q2: An observer 1.5 metres tall is 20.5 metres away from a tower 22 metres high. Determine the angle of elevation of the top of the tower from the eye of the observer.
Solution:
Let AB be the height of the observer and PR be the height of the tower.
Also, PB is the distance between the foot of the tower and the observer.
Consider θ as the angle of elevation of the top of the tower from the eye of the observer.
(ii) Calculating heights
Q 3: A ladder is placed along a wall of a house such that its upper end is touching the top of the wall. The foot of the ladder is 2 m away from the wall and the ladder is making an angle of 60° with the level of the ground. Determine the height of the wall.
Solution:
Given,
Distance between the wall and the foot of the ladder = 2m = BC
Angle made by ladder with ground (θ) = 60°
Height of the wall (H) = AB
Now, the fig. of ABC forms a right angle triangle.
So, tan θ =Perpendicular/hypotenuse
Q4: A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30°.
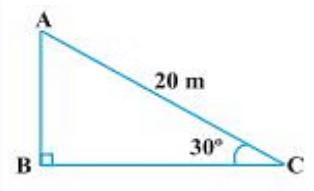
Solution:
Length of the rope is 20 m and angle made by the rope with the ground level is 30°.
Given: AC = 20 m and angle C = 30°
To Find: Height of the pole
Let AB be the vertical pole
In right ΔABC, using sine formula
sin 30° = AB/AC
Using value of sin 30 degrees is ½, we have
1/2 = AB/20
AB = 20/2
AB = 10
Therefore, the height of the pole is 10 m.
Q5: A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8 m. Find the height of the tree.
Solution:
Using given instructions, draw a figure. Let AC be the broken part of the tree. Angle C = 30°
BC = 8 m
To Find: Height of the tree, which was originally BD or AB+AC
AD=AC as [ tree fall due to storm ]
From figure: Total height of the tree is the sum of AB and AC i.e. AB+AC
In right ΔABC,
Using Cosine and tangent angles,
cos 30° = BC/AC
We know that, cos 30° = √3/2
√3/2 = 8/AC
AC = 16/√3 …(1)
Also,
tan 30° = AB/BC
1/√3 = AB/8
AB = 8/√3 ….(2)
Therefore, total height of the tree = AB + AC = 16/√3 + 8/√3 = 24/√3 = 8√3 m.
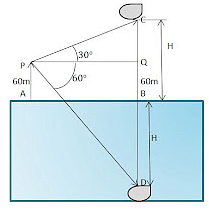
Q6: The angle of elevation of a cloud 60m above a lake is 30° and the angle of reflection of the cloud in the lake is 60°. Find height of the cloud.
Solution: The situation is depicted by the following diagram.
Let AB be the surface of the lake and let P be the point vertically above A such that AP=60m
Let C be the position of cloud and let D be its reflection in the lake.
Let the height of Cloud be H meters.
Then BC=BD=H (Let)
Draw PQ Perpendicular to CD
⇒ ∠QPC=30°, ∠QPD=60°
BQ=AP=60m
CQ=(H-60)m, DQ=(H+60)m
From right triangle ∆ CQP, we have
Let AB be the surface of the lake and let P be the point vertically above A such that AP=60m
Let C be the position of cloud and let D be its reflection in the lake.
Let the height of Cloud be H meters.Then BC=BD=H (Let)
Draw PQ Perpendicular to CD
⇒∠QPC=30°,∠QPD=60°
BQ=AP=60m
CQ=(H-60)m, DQ=(H+60)mFrom right triangle ∆ CQP, we have
PQ/CQ=cot30°
PQ/(H-60) = √3
PQ⇒(H-60) √3 m (i)From the right triangle ∆ DQP
PQ/DQ=cot60°
⇒PQ=(H+60)/√3 (ii)From (i) and (ii), we get
(H-60) √3=(H+60)/√3
⇒3H-180=H+60
⇒H=240
So, height of the cloud is H=240m.
(iii) Calculating lengths
Q7:The angle of elevation of a ladder leaning against a wall is 45° and foot of the ladder is 10m away from the wall. Find the length of ladder. The following diagram depicts the situation

Solution:Let OY is the ladder. Let OY=l. YX is the wall on which ladder is leaning on. It is given, ladder is leaned at 45°. Also, distance from the wall to foot of ladder is 10m, OX=d=10m. It is given √2=1.414
From the diagram,
cos 45°= OX/OY = d/l
⇒ l=d/cos 45°
We know that cos 45° = 1/√2
So, l = d/(1/√2) = √2 d
l = √2×10 m = 10√2 m = 10×1.414 = 14.14m
So, length of ladder is 14.14m
Q8 :- Two poles of equal heights are standing opposite each other on either side of the road, which is 80 m wide. From a point between them on the road, the angles of elevation of the top of the poles are 60° and 30°, respectively. Find the height of the poles and the distances of the point from the poles.
Solution:
Let AB and CD be the poles of equal height.
O is the point between them from where the height of elevation is taken. BD is the distance between the poles.
Therefore, the height of the poles are 20√3 m and the distance from the point of elevation are 20 m and 60 m respectively.
|
126 videos|457 docs|75 tests
|
FAQs on Some Applications of Trigonometry Class 10 Notes Maths Chapter 9
| 1. How can trigonometry be used to calculate heights and distances? |  |
| 2. How can one calculate heights using trigonometry? |  |
| 3. Can trigonometry help in calculating lengths of objects? |  |
| 4. What are some real-life applications of trigonometry in calculating heights and distances? |  |
| 5. How can one improve their trigonometry skills to better calculate heights and distances? |  |