Earth Satellites & Energy of a Satellite | Physics Class 11 - NEET PDF Download
| Table of contents |

|
| What is a Satellite? |

|
| Energies of a Satellite in a Circular Orbit |

|
| Motion of a Satellite in Elliptical Path |

|
| Satellite Motion and Angular Momentum Conservation |

|
What is a Satellite?
- An object orbiting around the sun, earth or any other colossal body is known as a satellite. There are two major types of categorization when it comes down to satellites, one is natural and the other is man-made.
- Some examples of natural satellites are planets, moons, and comets. Jupiter has 67 natural satellites. The earth has one permanent natural satellite, the moon we know, which causes the tides in the sea. Sometimes other objects (like asteroids) can enter into temporary orbits of the earth and become a natural satellite for a span.
- Apart from these, the earth has many man-made satellites that are placed in the orbit and are used for different applications in communications and information gathering. As the term itself states, an artificial satellite is one that is put in our space by human efforts and follows the orbit of natural satellites.
- Since they have a very large view field, they can collect data a lot faster than instruments that can be used at ground level. Apart from this, their view into space beyond earth is not blocked by clouds, dust, and other obscurities, due to which a satellite can view space a lot more efficiently than telescopes on earth.
 Satellites
Satellites
- The satellites are launched with a specific objective in mind pertaining to several uses such as communications, research in scientific areas, forecasting the weather, and intelligence. Once out in the space, all the different types of satellites follow similar physics principles and are governed by the same math equations.
Based on their purpose, there are two kinds of artificial satellites. They are geostationary satellites and polar satellites.
Types of Satellites
- Geostationary Satellite: These satellites are placed into orbit at a distance of around 35,800 km from the earth’s surface. They rotate in the same direction as the earth and one revolution of such satellites is the same as one day on earth (roughly 24 hours). This means that, as seen from earth, these satellites will appear to be at the same spot throughout. Hence, the name “geostationary” satellites. These satellites are used as communication satellites and for weather-based applications.
- Polar Satellite: Polar satellites revolve around the earth in a north-south direction around the earth as opposed to east-west like the geostationary satellites. They are very useful in applications where the field vision of the entire earth is required in a single day. Since the entire earth moves below them, this can be done easily. They are used in weather applications where predicting weather and climate-based disasters can be done in a short time. They are also used as relay stations.
The International Space Station (ISS) was launched into orbit in 1998. It is a habitable artificial satellite and sometimes can be seen on nights with a clear sky. It functions as a lab, observatory, and a landing base for possible expeditions.
Projectile Nature of a Satellite
- The main thing one can understand about a satellite is that at the end of the day, they are projectiles. Any object, that only has the force of gravity acting upon it, is known as a satellite. The gravity’s force is the only thing that affects a satellite once it is launched into the orbit.
- To understand this concept clearly, we will use the example of launching a satellite from the top of Newton’s Mountain which is a hypothetical location well above the influence of the drag force of the air. Newton was the first scientist to give the concept that if an object is launched with the adequate speed it will start orbiting the earth. This object would experience a gravitational pull that would try to pull it downwards when it travels in a horizontal direction tangentially to the earth.
- If the launch speed is slower than the escape velocity it will fall back to the earth. The lines A and B of the diagram represent those types of projectiles.
- If a projectile is shot off at an escape velocity with the perfect speed it will fall into an orbit outside the earth and will start revolving around the earth; the dotted line C represents such an object. If launched at a higher speed, the object will still revolve around the earth but will now have an elliptical orbit; the dotted line D represents such an object.
- It can also be possible that the object is shot at such a speed that it escapes the gravitational pull of the earth and become a free body; the solid line E represents such an object. The objects C and D never fall back to the earth even though being pulled towards it continuously, as our earth is a circular body.
Velocity Needed for an Object to Orbit the Earth in a Circular Pattern
This entire observation raises a very basic question, that how much velocity is necessary for shooting a body out of the earth’s lower atmosphere and establishing it into the outer one still in the range of the gravitational force. We get the answer by observing the most basic aspect of the earth, measuring its curvature.
It has been measured that for every 8000 meters that one goes along the horizon of the earth, the surface dips down by about 5 meters. Thus, applying basic mathematics we get the assumption that if a projectile wants to orbit around the sun, it will have to be at such a speed that it travels 8000 m for every 5 m of downward fall.
It was observed that if an object is launched horizontally it will fall by around 5 meters in the first second. Thus, we get to the conclusion that an object that is launched with a velocity of around 8000 m/s will orbit the earth in a circular pattern. This is only applicable when the object experiences an insignificant amount of atmospheric drag. The launched object will travel at a speed of around 8000 meters in a second and will drop around 5 meters but as the earth is spherical and has a curvature that drops 5 meters every 8000 meters, the object will never touch the ground.
Energies of a Satellite in a Circular Orbit
When there is a satellite revolving in a stable circular orbit of radius r around the earth, its speed is given by the above equation. During its motion, the kinetic energy of the satellite can be given as
As the gravitational force on the satellite due to the earth is the only force it experiences during motion, it has gravitational interaction energy in the field of the earth, which is given as
Thus the total energy of a satellite in an orbit of radius r can be given as
Total energy E = Kinetic energy + K Potential Energy U
or
We can see that,
The above relation in the magnitude of total, kinetic and potential energies of a satellite is very useful in numerical problems so it is advised to keep this relation in mind while handling satellite problems related to energy.
Now to understand satellite and planetary motion in detail, we take a few examples.
Example 1: Estimate the mass of the sun, assuming the orbit of the earth round the sun to be a circle. The distance between the sun and earth is 1.49 × 1011 m and G = 6.66 × 10-11 Nm2/kg2.
Solution:
Here the revolving speed of the earth can be given as
[Orbital speed]
Where M is the mass of the sun and r is the orbit radius of the earth.
We know time period of Earth around the sun is T = 365 days, thus we have
T =
or or
M = =
= 1.972 × 1022 kg
Example 2: If the earth is one-half of its present distance from the sun, how many days will be in one year ?
Solution: If the orbit of earth's radius is R, in the previous example we've discussed that time period is given as,
If the radius changes or r' = , the new time period becomes
From the above equations, we have
or
=
Example 3: A satellite revolving in a circular equatorial orbit of radius r = 2.00 × 104 km from west to east appears over a certain point at the equator every t = 11.6 hours. Using this data, calculate the mass of the earth. The gravitational constant is supposed to be known.
Solution: Here the absolute angular velocity of the satellite is given by
ω = ωs + ωE
Where wE is the angular velocity of the earth, which is from west to east.
or [Where t = 11.6 hr. and T = 24 hr.]
From Kepler's III law, we have
Thus we have
or = 6.0 × 1024 kg
Example 4: A satellite of mass m is moving in a circular orbit of radius r. Calculate its angular momentum with respect to the centre of the orbit in terms of the mass of the earth.
Solution: The situation is shown in the figure
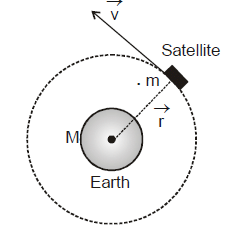
The angular momentum of the satellite with respect to the centre of orbit is given by
where is the position vector of the satellite with respect to the centre of orbit and
is its velocity vector of the satellite.
In the case of a circular orbit, the angle between and
is 90°. Hence
L = m v r sin 90° = m v r
The direction is perpendicular to the plane of the orbit.
We know the orbital speed of the satellite is
⇒
Now we will understand the concept of the double star system through an example.
Example 5: In a double star, two stars of masses m1 and m2. distance d apart revolves around their common centre of mass under the influence of their mutual gravitational attraction. Find an expression for the period T in terms of masses m1, m2 and d. Find the ratio of their angular momenta about the centre of mass and also the ratio of their kinetic energies.
Sol. The centre of mass of the double star from mass m1 is given by
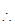 The distance of the centre of mass from m2 is
The distance of the centre of mass from m2 is
Both the stars rotate around the centre of mass in their circular orbits with the same angular speed ω.
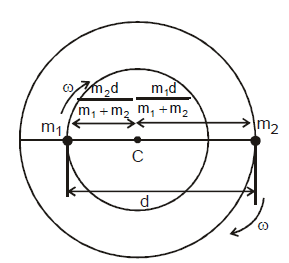
the gravitational force acting on each star provides the necessary centripetal force. if we consider the rotation of mass m1, then
or
This gives w = =
or Period of revolution
The ratio of Angular Momenta is
The ratio of kinetic energies is
Motion of a Satellite in Elliptical Path
- Whenever a satellite is in a circular or elliptical path, these orbits are called bounded orbits as the satellite is moving in an orbit bounded to Earth. The bound nature of orbit means that the kinetic energy of the satellite is not enough at any point in the orbit to take the satellite to infinity. In equation shows the negative total energy of a revolving satellite shows its boundness to Earth. Even when a body is in an elliptical path around the earth, its total energy must be negative. Let's first discuss how a satellite or a body can be in an elliptical path.
- Consider a body (satellite) of mass m in a circular path of radius r around the earth as shown in the figure. we've discussed that in a circular path, the net gravitational frame on the body is exactly balancing the centrifugal force on it in a radial direction relative to a rotating frame with the body.
- If suddenly the velocity of the body decreases then the centrifugal force on it becomes less than the gravitational force acting on it and due to this it can not continue in the circular orbit and will come inward from the circular orbit due to unbalanced force. Mathematical analysis shows that this path-I along which the body is now moving is an ellipse. The analytical calculations of the laws for this path are beyond the scope of this book. But it should be kept in mind that if the velocity of a body at a distance r from the earth's centre tangential to the circular orbit is less than
then its path will be elliptical with earth's centre at one of the foci of the ellipse.
- Similarly, if the speed of the body exceeds
then it must move out of the circular path due to unbalancing of forces again but this time Fe > Fg. Due to this if the speed of the body is not increased by such a value that its kinetic energy can take the particle to infinity then it will follow in a bigger elliptical orbit as shown in figure in path-II, with earth's at one of the foci of the orbit.
- In the above case when the speed of the body was decreased and its value is lesser than
and the speed is decreased to such a value that the elliptical orbit will intersect the earth's surface as shown in the figure, then the body will follow an arc of the ellipse and will fall back to earth.
Satellite Motion and Angular Momentum Conservation
- We've discussed that when a body is in bounded orbit around a planet it can be in a circular or elliptical path depending on its kinetic energy at the time of launching. Let's consider a case when a satellite is launched in an orbit around the earth.
- A satellite S is first fired away from the earth's source in the vertical direction to penetrate the earth's atmosphere. When it reaches point A, it is imparted a velocity in the tangential direction to start its revolution around the earth in its orbit.
- This velocity is termed insertion velocity, if the velocity imparted to the satellite is
then it starts following the circular path shown in the figure. If the velocity imparted is v1 > v0 then it will trace the elliptical path shown. During this motion, the only force acting on the satellite is the gravitational force due to Earth which is acting along the line joining the satellite and the centre of earth.
- As the force on the satellite always passes through the centre of the earth during motion, we can say that on a satellite there is no torque acting about the centre of the earth thus total angular momentum of the satellite during orbital motion remains constant about the earth's centre.
- As no external force is involved for the earth-satellite system, no external work is being done here so we can also state that the total mechanical energy of the system also remains conserved.
- In the elliptical path of the satellite shown in the figure if r1 and r2 are the shortest distance (perigee) and farthest distance (apogee) of the satellite from Earth and at the points, velocities of the satellite are v1 and v2 then we have according to the conservation of angular momentum, the angular momentum of the satellite at a general point is given as
- L = mv1r1 = mv2r2 = mvr sinθ
- During motion, the total mechanical energy of a satellite (kinetic potential) also remains conserved. Thus the total energy of the satellite can be given as
- Using the above relations in the equation written above we can find velocities v1 and v2 of satellites at nearest and farthest locations in terms of r1 and r2.
|
122 videos|481 docs|98 tests
|
FAQs on Earth Satellites & Energy of a Satellite - Physics Class 11 - NEET
| 1. What is the energy of a satellite in a circular orbit? |  |
| 2. How does a satellite move in an elliptical path? |  |
| 3. How is angular momentum conserved in satellite motion? |  |
| 4. What is the significance of Earth satellites in studying the energy of a satellite? |  |
| 5. What are some frequently asked questions about the motion of satellites in relation to energy conservation? |  |




















