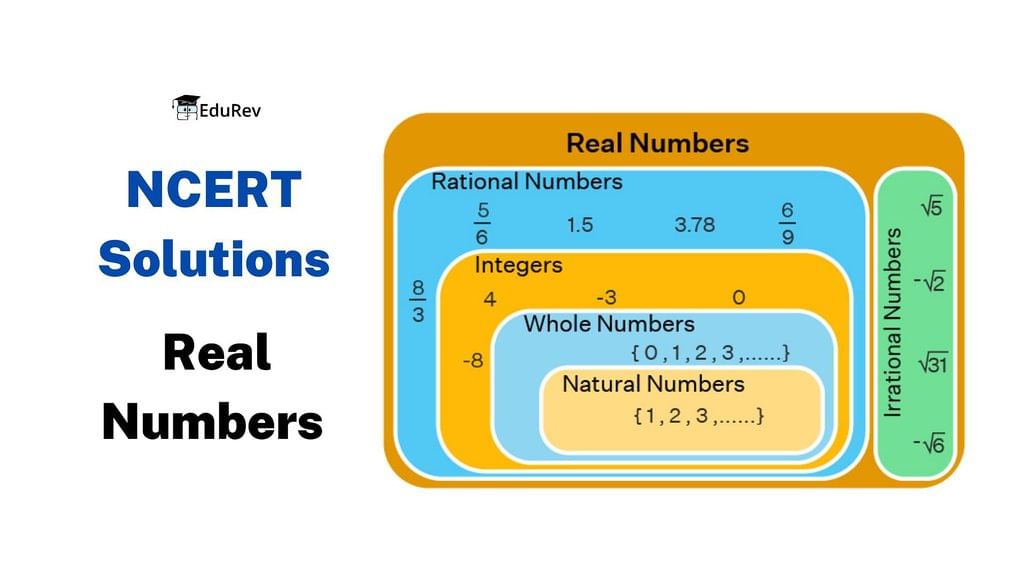
NCERT Solutions for Class 10 Maths Chapter 1 - Real Numbers (Exercise 1.1)
Q1. Express each number as a product of its prime factors:
(i) 140
(ii) 156
(iii) 3825
(iv) 5005
(v) 7429
Solutions:
(i) 140
Prime factors of 140 = 2, 2, 5, 7
= 22 x 5 x 7
(ii) 156
Prime factors of 156 = 2 x 2 x 3 x 13
= 22 x 3 x 13
(iii) 3825
Prime factors of 3825 = 3 x 3 x 5 x 5 x 17
= 32 x 52 x 17
(iv) 5005
Prime factors of 5005 = 5 x 7 x 11 x 13
(v) 7429
Prime factors of 7429 = 17 x 19 x 23
Q2. Find the LCM and HCF of the following pairs of integers and verify that LCM × HCF = product of the two numbers.
(i) 26 and 91
(ii) 510 and 92
(iii) 336 and 54
Solutions:
(i) 26 and 91
Prime factors of 26 = 2 x 13
Prime factors of 91 = 7 x 13
HCF of 26 and 91 = 13
LCM of 26 and 91 = 2 x 7 x 13
= 14 x 17
= 182
Product of two numbers = 26 x 91
= 2,366
LCM x HCF = 182 x13 = 2,366
So, product of two numbers = LCM x HCF
(ii) 510 and 92
Prime factors of 510 = 2 x 3 x 5 x17
Prime factors of 92 = 2x 2 x 23
HCF of two numbers = 2
LCM of two numbers = 2 x 2 x 3 x 5 x 17 x 23
= 23460
Product of two numbers = 510x92
= 46920
LCM x HCF = 2 x 23460
=46920
Product of two numbers = LCM x HCF
(iii) 336 and 54
Prime factors of 336 = 2 x 2 x 2 x 2 x 3 x 7
Prime factors of 54 = 2 x 3 x 3 x 3
HCF of two numbers = 6
LCM of two numbers = 2 x 2 x 2 x 2 x 3 x 3 x 3 x 7
= 24 x 33 x 7 = 3024
Product of two numbers = 336 x 54
=18144
LCM x HCF = 3024 x 6 =18144
Product duct of two numbers = LCM x HCF
Q3. Find the LCM and HCF of the following integers by applying the prime factorisation method.
(i) 12, 15 and 21
(ii) 17, 23 and 29
(iii) 8, 9 and 25
Solutions:
(i) 12, 15 and 21
Prime factors of 12 = 2 x 2 x 3
= 22 x 3
Prime factors of 15 = 3 x 5
Prime factors of 21 = 2 x 2 x 3
HCF of 12,15 and 21 = 3
LCM of 12,15 and 21 = 22 x 3 x 5 x 7
= 420
(ii) 17, 23 and 29
Prime factors of 17 = 17 x 1
Prime factors of 23 = 23 x 1
Prime factors of 29 = 29 x 1
HCF of 17, 23 and 29 = 1
LCM of 17, 23 and 29 = 17 x 23 x 29
= 11339
(iii) 8, 9 and 25
Prime factors of 8 = 2 x 2 x 2 x1
= 23 x 1
Prime factors of 9 = 3 x 3 x1
= 32 x 1
Prime factors of 25 = 5 x 5 x 1
= 52 x 1
HCF of 8, 9 and 25 = 1
LCM of 8,9 and 25 = 2 x 2 x 2 x 3 x 3 x 5 x 5
= 1800
Q4. Given that HCF (306, 657) = 9, find LCM (306, 657).
Solution:
Given, HCF (306, 657) = 9.
We have to find,
LCM (306, 657) = ?
We know that
LCM x HCF = Product of two numbers
LCM x 9 = 306x 657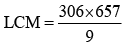
LCM = 34 x 657
LCM = 22338
Q5. Check whether 6n can end with the digit 0 for any natural number n.
Solution:
Prime factors of 6n = (2 x 3)n = (2)n (3)n
You can observe clearly, 5 is not in the prime factors of 6n.
That means 6n will not be divisible by 5.
Therefore, 6n cannot end with the digit 0 for any natural number n.
Q6. Explain why 7 × 11 × 13 + 13 and 7 × 6 × 5 × 4 × 3 × 2 × 1 + 5 are composite numbers.
Solution:
It can be observed that,
7 x 11 x 13 + 13 = 13 (7 x11 +1)
= 13( 77 + 1)
= 13 x 78
= 13 x13 x 6 x1
= 13 x13 x 2 x 3 x1
The given number has 2,3,13 and 1 as its factors. Therefore, it is a composite number.
Q7. There is a circular path around a sports field. Sonia takes 18 minutes to drive one round of the field, while Ravi takes 12 minutes for the same. Suppose they both start at the same point and at the same time, and go in the same direction. After how many minutes will they meet again at the starting point?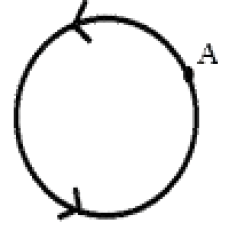 Solution:
Solution:
LCM of 18 and 12,
18 = 2 x 3 x 3
12 = 2 x 2 x 3
LCM of 12 and 18 = 2 x 2 x 3 x 3 = 36
Therefore, Ravi and Sonia will meet together at starting point after 36 minutes.
Check out the NCERT Solutions of all the exercises of Real Numbers:
|
127 videos|550 docs|75 tests
|
FAQs on NCERT Solutions for Class 10 Maths Chapter 1 - Real Numbers (Exercise 1.1)
| 1. What are real numbers and how are they classified? |  |
| 2. How do you find the LCM and HCF of real numbers? |  |
| 3. What is the significance of the Euclidean algorithm in finding HCF? |  |
| 4. Can you provide examples of rational and irrational numbers? |  |
| 5. How do real numbers relate to the number system? |  |























