Ionic Bonding & Characteristics of Ionic Compounds | Chemistry Optional Notes for UPSC PDF Download
Introduction
- Ions are atoms or molecules which are electrically charged. Cations are positively charged and anions carry a negative charge. Ions form when atoms gain or lose electrons. Since electrons are negatively charged, an atom that loses one or more electrons will become positively charged; an atom that gains one or more electrons becomes negatively charged. Ionic bonding is the attraction between positively- and negatively charged ions. These oppositely charged ions attract each other to form ionic networks (or lattices).
- Electrostatics explains why this happens: opposite charges attract and like charges repel. When many ions attract each other, they form large, ordered, crystal lattices in which each ion is surrounded by ions of the opposite charge. Generally, when metals react with non-metals, electrons are transferred from the metals to the non-metals. The metals form positively-charged ions and the non-metals form negatively-charged ions.
Generating Ionic Bonds
- Ionic bonds form when metals and non-metals chemically react. By definition, a metal is relatively stable if it loses electrons to form a complete valence shell and becomes positively charged. Likewise, a non-metal becomes stable by gaining electrons to complete its valence shell and become negatively charged. When metals and non-metals react, the metals lose electrons by transferring them to the non-metals, which gain them. Consequently, ions are formed, which instantly attract each other—ionic bonding.
- In the overall ionic compound, positive and negative charges must be balanced, because electrons cannot be created or destroyed, only transferred. Thus, the total number of electrons lost by the cationic species must equal the total number of electrons gained by the anionic species.
For example, in the reaction of Na (sodium) and Cl (chlorine), each Cl atom takes one electron from a Na atom. Therefore each Na becomes a Na+ cation and each Cl atom becomes a Cl- anion. Due to their opposite charges, they attract each other to form an ionic lattice. The formula (ratio of positive to negative ions) in the lattice is NaCl.
2Na(s) + Cl2(g) → 2NaCl(s) (9.2.1)
These ions are arranged in solid NaCl in a regular three-dimensional arrangement (or lattice):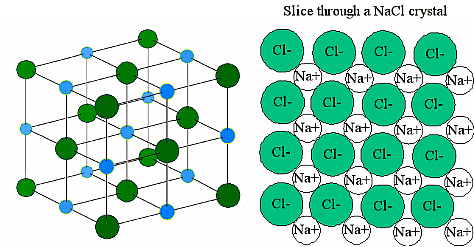
NaCl lattice. (left) 3-D structure and (right) simple 2D slice through lattes. Images used with permission from Wikipedia and Mike Blaber.
The chlorine has a high affinity for electrons, and the sodium has a low ionization potential. Thus the chlorine gains an electron from the sodium atom. This can be represented using electron-dot symbols (here we will consider one chlorine atom, rather than Cl2):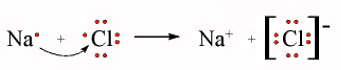
The arrow indicates the transfer of the electron from sodium to chlorine to form the Na+ metal ion and the Cl- chloride ion. Each ion now has an octet of electrons in its valence shell:
- Na+: 2s22p6
- Cl-: 3s23p6
Energetics of Ionic Bond Formation
Ionic bonds are formed when positively and negatively charged ions are held together by electrostatic forces. Consider a single pair of ions, one cation and one anion. How strong will the force of their attraction be? According to Coulomb's Law, the energy of the electrostatic attraction (E) between two charged particles is proportional to the magnitude of the charges and inversely proportional to the internuclear distance between the particles (r):
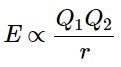 (10.4.1)
(10.4.1)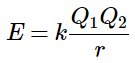 (10.4.2)
(10.4.2)
where each ion’s charge is represented by the symbol Q. The proportionality constant k is equal to 2.31 × 10−28 J·m. This value of k includes the charge of a single electron (1.6022 × 10−19 C) for each ion. The equation can also be written using the charge of each ion, expressed in coulombs (C), incorporated in the constant. In this case, the proportionality constant, k, equals 8.999 × 109 J·m/C2. In the example given, Q1 = +1(1.6022 × 10−19 C) and Q2 = −1(1.6022 × 10−19 C). If Q1 and Q2 have opposite signs (as in NaCl, for example, where Q1 is +1 for Na+ and Q2 is −1 for Cl−), then E is negative, which means that energy is released when oppositely charged ions are brought together from an infinite distance to form an isolated ion pair.
- Energy is always released when a bond is formed and correspondingly, it always requires energy to break a bond.
As shown by the green curve in the lower half of Figure 10.4.1, the maximum energy would be released when the ions are infinitely close to each other, at r = 0. Because ions occupy space and have a structure with the positive nucleus being surrounded by electrons, however, they cannot be infinitely close together. At very short distances, repulsive electron–electron interactions between electrons on adjacent ions become stronger than the attractive interactions between ions with opposite charges, as shown by the red curve in the upper half of Figure 10.4.1. The total energy of the system is a balance between the attractive and repulsive interactions. The purple curve in Figure 10.4.1 shows that the total energy of the system reaches a minimum at r0, the point where the electrostatic repulsions and attractions are exactly balanced. This distance is the same as the experimentally measured bond distance.
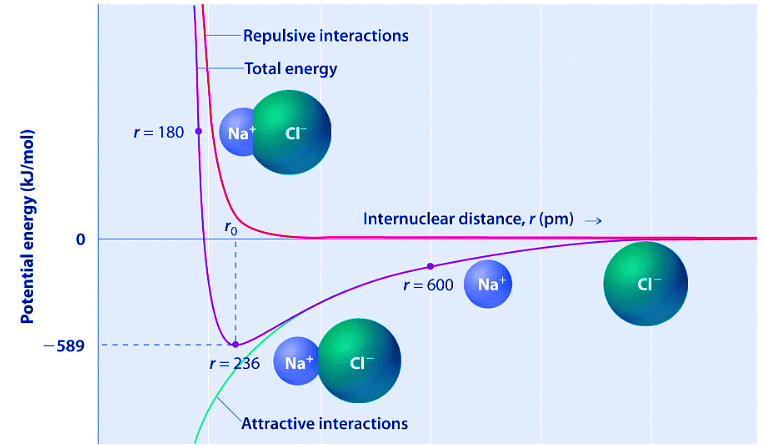
Figure 10.4.1: A Plot of Potential Energy versus Internuclear Distance for the Interaction between a Gaseous Na+ Ion and a Gaseous Cl− Ion. The energy of the system reaches a minimum at a particular distance (r0) when the attractive and repulsive interactions are balanced.
Consider the energy released when a gaseous Na+ ion and a gaseous Cl− ion are brought together from r = ∞ to r = r0. Given that the observed gas-phase internuclear distance is 236 pm, the energy change associated with the formation of an ion pair from an 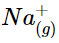 ion and a
ion and a 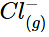 ion is as follows:
ion is as follows: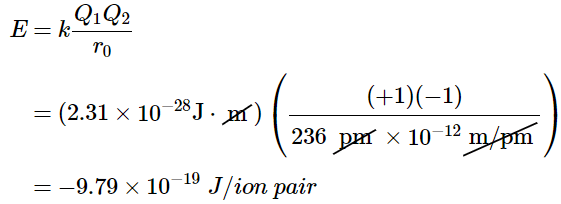
The negative value indicates that energy is released. Our convention is that if a chemical process provides energy to the outside world, the energy change is negative. If it requires energy, the energy change is positive. To calculate the energy change in the formation of a mole of NaCl pairs, we need to multiply the energy per ion pair by Avogadro’s number: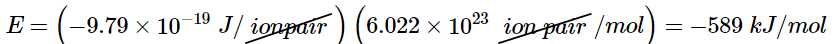 (10.4.3)
(10.4.3)
This is the energy released when 1 mol of gaseous ion pairs is formed, not when 1 mol of positive and negative ions condenses to form a crystalline lattice. Because of long-range interactions in the lattice structure, this energy does not correspond directly to the lattice energy of the crystalline solid. However, the large negative value indicates that bringing positive and negative ions together is energetically very favorable, whether an ion pair or a crystalline lattice is formed.
We summarize the important points about ionic bonding:
- At r0, the ions are more stable (have a lower potential energy) than they are at an infinite internuclear distance. When oppositely charged ions are brought together from r = ∞ to r = r0, the energy of the system is lowered (energy is released).
- Because of the low potential energy at r0, energy must be added to the system to separate the ions. The amount of energy needed is the bond energy.
- The energy of the system reaches a minimum at a particular internuclear distance (the bond distance).
Solved Examples
Example 1: Calculate the amount of energy released when 1 mol of gaseous Li+F− ion pairs is formed from the separated ions. The observed internuclear distance in the gas phase is 156 pm.
Given: cation and anion, amount, and internuclear distance
Asked for: energy released from formation of gaseous ion pairs
Strategy: Substitute the appropriate values into Equation 10.4.2 to obtain the energy released in the formation of a single ion pair and then multiply this value by Avogadro’s number to obtain the energy released per mole.
Ans: Inserting the values for Li+F− into Equation 10.4.2 (where Q1 = +1, Q2 = −1, and r = 156 pm), we find that the energy associated with the formation of a single pair of Li+F− ions is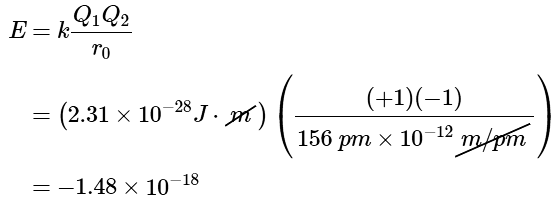
Then the energy released per mole of Li+F− ion pairs is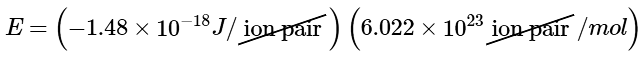
−891kJ/mol
Because Li+ and F− are smaller than Na+ and Cl− (see Section 7.3), the internuclear distance in LiF is shorter than in NaCl. Consequently, by Equation 10.4.2, much more energy is released when 1 mol of gaseous Li+F− ion pairs is formed (−891 kJ/mol) than when 1 mol of gaseous Na+Cl− ion pairs is formed (−589 kJ/mol).
Example 2: Calculate the amount of energy released when 1 mol of gaseous MgO ion pairs is formed from the separated ions. The internuclear distance in the gas phase is 175 pm.
Ans: −3180 kJ/mol = −3.18 × 103 kJ/mol
Electron Configuration of Ions
How does the energy released in lattice formation compare to the energy required to strip away a second electron from the Na+ ion? Since the Na+ ion has a noble gas electron configuration, stripping away the next electron from this stable arrangement would require more energy than what is released during lattice formation (Sodium I2 = 4,560 kJ/mol). Thus, sodium is present in ionic compounds as Na+ and not Na2+. Likewise, adding an electron to fill a valence shell (and achieve noble gas electron configuration) is exothermic or only slightly endothermic. To add an additional electron into a new subshell requires tremendous energy - more than the lattice energy. Thus, we find Cl- in ionic compounds, but not Cl2-.
Table 10.4.1: Lattice energies range from around 700 kJ/mol to 4000 kJ/mol:
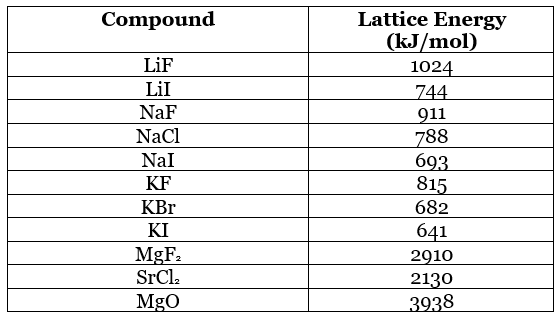
This amount of energy can compensate for values as large as I3 for valence electrons (i.e. can strip away up to 3 valence electrons). Because most transition metals would require the removal of more than 3 electrons to attain a noble gas core, they are not found in ionic compounds with a noble gas core. A transition metal always loses electrons first from the higher 's' subshell, before losing from the underlying 'd' subshell. (The remaining electrons in the unfilled d subshell are the reason for the bright colors observed in many transition metal compounds!) For example, iron ions will not form a noble gas core:
- Fe: [Ar]4s23d6
- Fe2+: [Ar] 3d6
- Fe3+: [Ar] 3d5
Some metal ions can form a pseudo noble gas core (and be colorless), for example:
- Ag: [Kr]5s14d10 Ag+ [Kr]4d10 Compound: AgCl
- Cd: [Kr]5s24d10 Cd2+ [Kr]4d10 Compound: CdS
The valence electrons do not adhere to the "octet rule" in this case (a limitation of the usefulness of this rule).
Note: The silver and cadmium atoms lost the 5s electrons in achieving the ionic state.
- When a positive ion is formed from an atom, electrons are always lost first from the subshell with the largest principle quantum number
Polyatomic Ions
Not all ionic compounds are formed from only two elements. Many polyatomic ions exist, in which two or more atoms are bound together by covalent bonds. They form a stable grouping which carries a charge (positive or negative). The group of atoms as a whole acts as a charged species in forming an ionic compound with an oppositely charged ion. Polyatomic ions may be either positive or negative, for example:
- NH4+ (ammonium) = cation
- SO42- (sulfate) = anion
The principles of ionic bonding with polyatomic ions are the same as those with monatomic ions. Oppositely charged ions come together to form a crystalline lattice, releasing a lattice energy. Based on the shapes and charges of the polyatomic ions, these compounds may form crystalline lattices with interesting and complex structures.
Summary
The amount of energy needed to separate a gaseous ion pair is its bond energy. The formation of ionic compounds are usually extremely exothermic. The strength of the electrostatic attraction between ions with opposite charges is directly proportional to the magnitude of the charges on the ions and inversely proportional to the internuclear distance. The total energy of the system is a balance between the repulsive interactions between electrons on adjacent ions and the attractive interactions between ions with opposite charges.
Characteristics of Ionic Compounds
The figure below shows just a few examples of the color and brilliance of naturally occurring ionic crystals. The regular and orderly arrangement of ions in the crystal lattice is responsible for the various shapes of these crystals, while transition metal ions give rise to the colors.
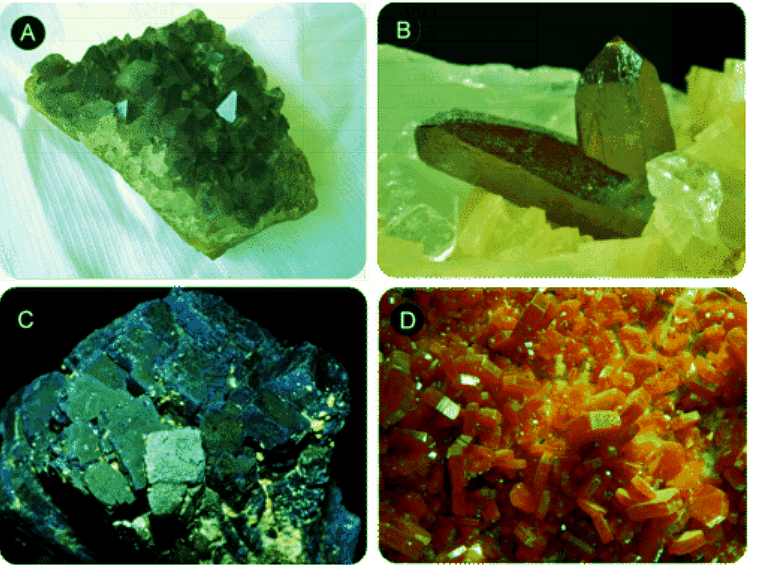
Figure 4.7.1: In nature, the ordered arrangement of ionic solids gives rise to beautiful crystals. (A) Amethyst - a form of quartz, SiO2, whose purple color comes from iron ions. (B) Cinnabar - the primary ore of mercury is mercury (II) sulfide, HgS (C) Azurite - a copper mineral, Cu3(CO3)2(OH)2. (D) Vanadinite - the primary ore of vanadium, Pb3(VO4)3Cl.
Physical Properties of Ionic Compounds
- Melting Points: Because of the many simultaneous attractions between cations and anions that occur, ionic crystal lattices are very strong. The process of melting an ionic compound requires the addition of large amounts of energy in order to break all of the ionic bonds in the crystal. For example, sodium chloride has a melting temperature of about 800oC. As a comparison, the molecular compound water melts at 0 °C.
- Shattering: Ionic compounds are generally hard, but brittle. Why? It takes a large amount of mechanical force, such as striking a crystal with a hammer, to force one layer of ions to shift relative to its neighbor. However, when that happens, it brings ions of the same charge next to each other (see below). The repulsive forces between like-charged ions cause the crystal to shatter. When an ionic crystal breaks, it tends to do so along smooth planes because of the regular arrangement of the ions.
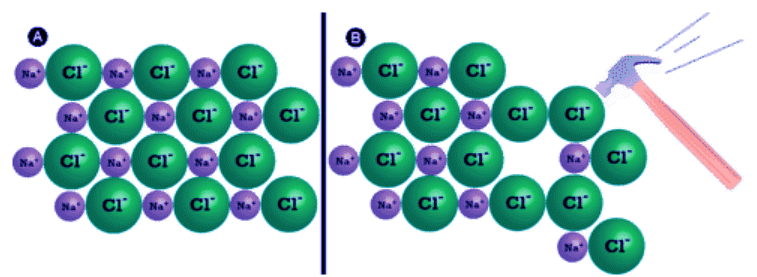
Figure 4.7.2: (A) The sodium chloride crystal is shown in two dimensions. (B) When struck by a hammer, the negatively-charged chloride ions are forced near each other and the repulsive force causes the crystal to shatter.
- Solubility in water A considerable high number of ionic compounds are soluble in water. Table salt, or sodium chloride (NaCl), the most common ionic compound, is soluble in water (360 g/L). Recall that NaCl is a salt crystal composed not of discrete NaCl molecules, but rather of an extended array of Na+ and Cl- ions bound together in three dimensions through electrostatic interactions. When a crystal of NaCl comes into contact with water, the water molecules interact with the Na+ and Cl- ions on the crystal’s surface, as shown in the figure 4.7.3 . As a consequence of the interaction between the Na+ and Cl- ions and the water molecules, the electrostatic interactions within the crystal are broken.
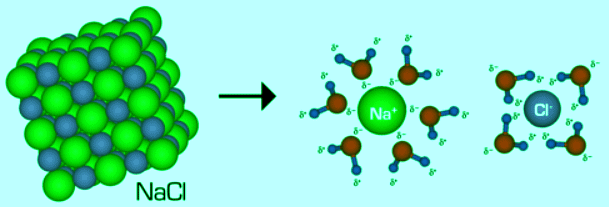
Figure 4.7.3: Dosilution of sodium chloride in water. Image by Ahazard.
- Conductivity: Another characteristic property of ionic compounds is their electrical conductivity. The figure below shows three experiments in which two electrodes that are connected to a light bulb are placed in beakers containing three different substances.
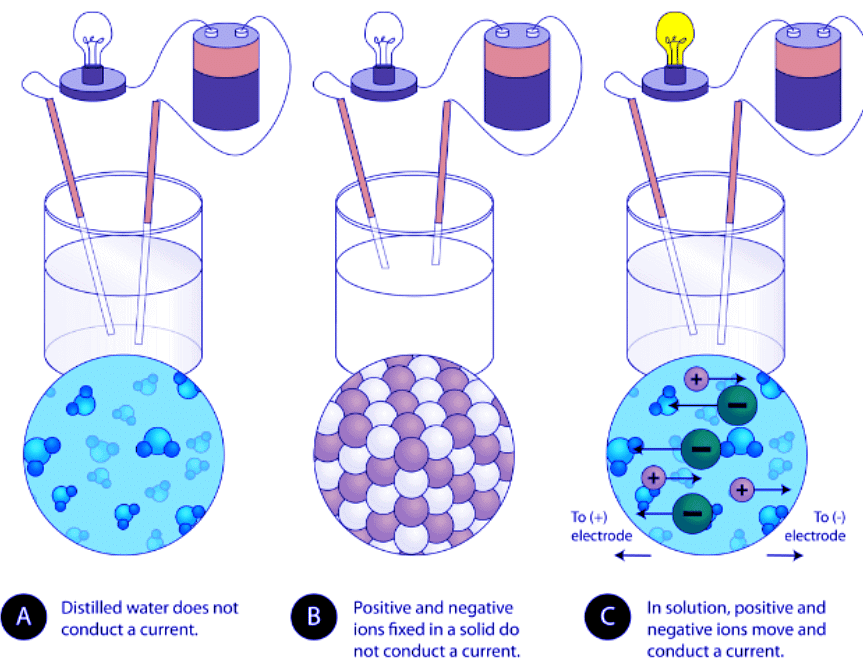
Figure 4.7.4. (A) Distilled water does not conduct electricity. (B) A solid ionic compound also does not conduct. (C) A water solution of an ionic compound conducts electricity well.
- In the first beaker, distilled water does not conduct a current because water is a molecular compound. In the second beaker, solid sodium chloride also does not conduct a current. Despite being ionic and thus composed of charged particles, the solid crystal lattice does not allow the ions to move between the electrodes.
- Mobile charged particles are required for the circuit to be complete and the light bulb to light up. In the third beaker, the NaCl has been dissolved into the distilled water. Now the crystal lattice has been broken apart and the individual positive and negative ions can move. Cations move to one electrode, while anions move to the other, allowing electricity to flow (see figure below). Melting an ionic compound also frees the ions to conduct a current. Ionic compounds conduct an electric current when melted or dissolved in water.
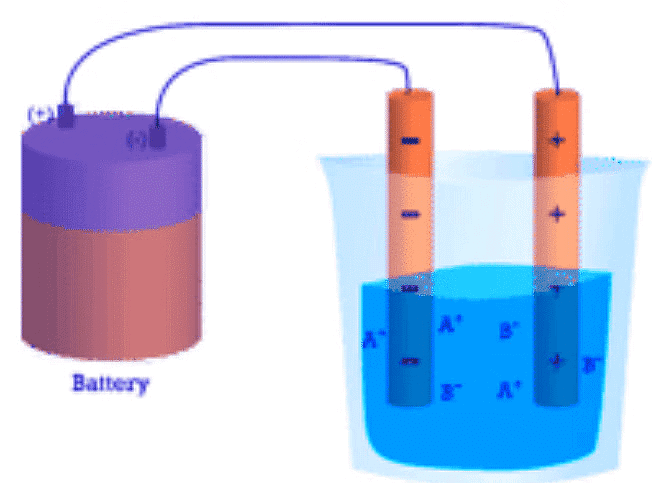
Figure 4.7.5: In an ionic solution, the A+ ions migrate toward the negative electrode, while the B− ions migrate toward the positive electrode.
Solved Examples
Example 1: Write the dissociation equation of solid NaCl in water.
Ans: NaCl(s) → Na+(aq) + Cl–(aq)
Example 2: Write the dissociation equation of solid NH4NO3 in water.
Ans: NH4NO3(s) → NH4+(aq) + NO3–(aq)
Key Takeaways
- Ionic compounds have high melting points.
- Ionic compounds are hard and brittle.
- Ionic compounds dissociate into ions when dissolved in water.
- Solutions of ionic compounds and melted ionic compounds conduct electricity, but solid materials do not.
- An ionic compound can be identified by its chemical formula: metal + nonmetal or polyatomic ions.
FAQs on Ionic Bonding & Characteristics of Ionic Compounds - Chemistry Optional Notes for UPSC
| 1. What is an ionic bond? |  |
| 2. How are ionic bonds formed? |  |
| 3. What is the role of energy in the formation of ionic bonds? |  |
| 4. What are the physical properties of ionic compounds? |  |
| 5. What is the electron configuration of ions? |  |

|
Explore Courses for UPSC exam
|

|
















