Overview: Number Series, Letter Series, Alpha-Numeric Series | CSAT Preparation - UPSC PDF Download
| Table of contents |

|
| Importance in CSAT |

|
| Introduction |

|
| Type I Number Series |

|
| Type II Letter Series |

|
| Type III Alpha-Numeric Series |

|
| CSAT Solved Examples |

|
Importance in CSAT
The chapter 'Series' carries immense importance for CSAT exam. Questions are asked frequently from this chapter. Based on the analysis of Previous Years' Papers, it has been concluded that in the year 2023, three questions and in the year 2022 one question was asked. In the years 2021-2018, almost every year one question was asked from this chapter. Basically, the questions that are asked from this topic are based on finding the missing term or wrong term of a number/letter/mixed series.
Introduction
The series is the systematic arrangement of letters, words, numbers, objects, etc., in a particular order or sequence. The series follows a certain pattern, which is based on rules comprising numerical, alphabetical, or the combination of both alphabets and numbers.
Let us look at various types of series:
Type I Number Series
These are the systematic arrangement of numbers following a predefined pattern. These patterns can be of any type like possessing an arithmetic operation (division, multiplication, subtraction, and addition), consisting of prime numbers, even numbers, odd numbers, etc.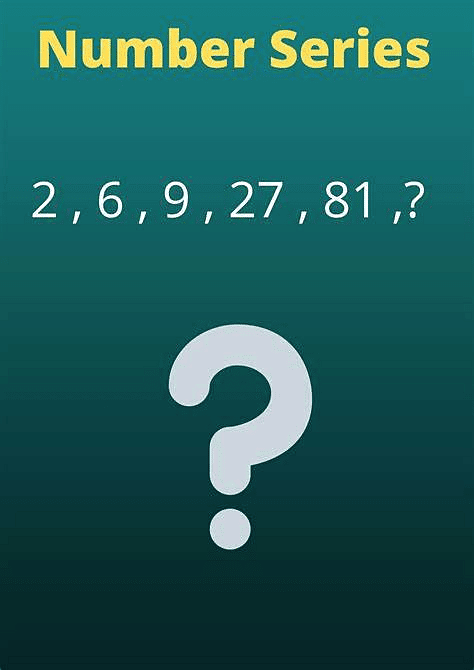 Generally, two types of questions are asked from this chapter:1. Finding the Missing Term: In these types of questions, it is asked to find the next missing term or term missing in between. For example: 4, 8, ?, 32, 64
Generally, two types of questions are asked from this chapter:1. Finding the Missing Term: In these types of questions, it is asked to find the next missing term or term missing in between. For example: 4, 8, ?, 32, 64
Here, the given series is a multiplication series, i.e., × 2. So, the missing term is:
8 × 2 = 16
2. Find the Wrong Term: In these types of questions, it is asked to find the wrong term in the given series following a certain pattern. For example: 5, 10, 15, 19, 25
Here, this series is a multiple series having the multiples of 5, i.e., 5, 10, 15,… So, the wrong term is 19, which should be replaced by 20.
Some of the series are explained as under:
Prime Number Series
These types of series are formed with prime numbers. In such series, operations on prime numbers are performed to form a series such as addition, subtraction, or multiplication of prime numbers. They can also include squares of prime numbers or alternate prime numbers to form a series.
Example: Find the wrong term in the series:
7, 11, 13, 15, 19, 23
(a) 13
(b) 15
(c) 19
(d) 23
Ans: (b)
Clearly, it is a series of consecutive prime numbers, so after 13, the next prime number is 17. So, 15 must be replaced by 17.
Addition Series
In such series, a particular number or a particular sequence is added to a term to form the next consecutive term.
Example: Find the missing term in the series: 3, 6, 9, 12, 15, ..., 21.
(a) 16
(b) 17
(c) 20
(d) 18
Ans:: In the given series, 3 is added to each term to form the next consecutive term, so the missing term is 15 + 3 = 18. Therefore, the correct option is (d).
Find the missing term in the series: 3, 6, 9, 12, 15, ..., 21.
Subtraction Series
In these types of series, a particular number or a number in a certain pattern is subtracted from each term to form the next consecutive term.
Example: Find the missing term in the series:
105, 104, 101, 96, ?, 80.
(a) 81
(b) 91
(c) 89
(d) 88
Ans:
The pattern of the given series is -1, -3, -5, -7, …
i.e. subtraction of consecutive odd numbers. So, the missing term is 96 - 7, i.e., 89.
Therefore, the correct answer is (c) 89
Multiplication Series
In these types of series, each term is multiplied by a number under a specific pattern to obtain the next consecutive term.
Example: Find the value of x in the series: 2, 6, 30, 210, x, 30030, ... UPSC (CSAT) 2012
(a) 2310
(b) 1890
(c) 2520
(d) 2730
Ans: (a)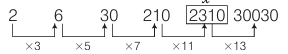
Here, number are multiplied by prime numbers.
Division Series
Like multiplication series, here each term is divided by ular number or number in a specified pattern to form the consecutive termExample: Find the missing term in the series: 5040, 720, 120, 24, …, 2, 1.
(a) 8
(b) 7
(c) 6
(d) 5
Ans: (c)
Here, 5040/7= 720, 720/6 =120
120/5= 24, 24/4 = 6
6/3 = 2, 2/2= 1
Mixed Series
These types of series can be formed by using any mathematical operation or by any other means having two or three series mixed together. Except it, series can have square, square root, cube, cube root, etc., in ascending or descending order.Example: Find the missing term in the series . UPSC(CSAT) 2012
48, 24,72, 36, 108,?
(a) 115
(b) 216
(c) 121
(d) 54
Ans: (d)
Solution: The pattern of the series is
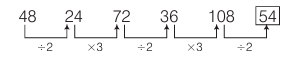
Series of Series
In these types of series, a number series is given in ascending or descending order. The terms by which the original terms increase or decrease also follow a series, and so on. The questions based on this type are comparatively difficult.
Example: Find the missing term of the givenseries
0, 19, 45, 85, 153, 277 ?
(a) 433
(b) 513
(c) 497
(d) 555
Ans: (b)
Solution:The pattern of the series is
Type II Letter Series
These type of series are formed with the help of the English alphabet. In these type of series, the letters of the English alphabet are arranged in some pre-defined patterns like skipping of letters between them, in pairs of opposite letters, continuous sequences of letters, etc. As in number series, in letter series also we may have been asked about missing term or wrong term but once we have known about the pattern, we may answer the question.
Single Letter or Group of Letters Series
These series are formed by skipping a pre-defined number of letters between the consecutive terms. This skipping can be in forward order or in backward order or can be in both directions in a group of letter series.
Example: Find the next term in the series.
A, D, G, J, ?
(a) K
(b) L
(c) M
(d) N
Ans: (c)
Solution:
Each next term of the series is the next third letter of the alphabet (according to position).
Continuous Series
In this type of series, usually small letters of the alphabet are used to make a set of letters, which are repeated. The candidate has to find the set of letters which are fit in the blanks left in the series in such a manner that follow a certain pattern. This pattern may be repeated exactly or an increasing order in every block may be there.
Example: In the following series, some letters are missing. From the choices, select the choice that gives the letters that can fill the blanks in the given sequence.
a_c_b_ab_a_ca_c
Ans: (d)
Solution:
First of all, notice that there are 6 blanks in the given sequence and each choice gives six letters to fill up the six blanks in that order. Now, we have to select an alternative which, if placed in the blanks of the series in that order, we get a complete series of letters which follow some particular pattern.
The best way is to try with each option. Inserting the letters of option (d) in place of the blanks, we get a series like 'abcabcabcabcabc', which is a repetition of the group of letters 'abc'.
Important Points
While attempting the questions based on continuous series:
- In continuous series, firstly count the total given letters and blanks and secondly, find out how many multiples of numbers are exist in the series. Like in the above example, there are 15 places which have letters and blanks, so 15 could be the multiple of: =3 × 5= 15 × 1
Here, we prefer 15 as 5×3 because the series is divided into 5 parts and continues accordingly: abc/abc/abc/abc/abc.
Opposite Letter Series
In these type of series, a term is formed with grouping the opposite letters in the English alphabet and then terms are skipped between them.
Example: Find the missing term in the series: LO, TG, ..., MN, BY, UF.
Ans: (c)
Solution:
Every element of the series has two opposite letters and their number position addition is equivalent to 27. So, only option (c) having the letters whose number position addition is equivalent to 27.
Type III Alpha-Numeric Series
These type of series are the combination of letter and number series and follow any of the above patterns.
Example: Find the missing term in the series. 5 G 7, 7 H 10, 10 I 14, 14 J 19,?
(a) 16 K 20
(b) 17 K 21
(c) 18 K 21
(d) 19 K 25
Ans: (d)
The pattern of the series is
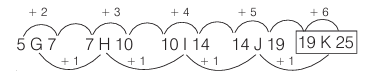
CSAT Solved Examples
Directions (Question 1 and 2) : In the given mixed series, find the value of missing term.
1. D23F, H19J, L17N, …, T11V
(a) P15R
(b) P14R
(c) P13R
(d) P12R
Ans: (c)
Solution: The pattern of the series is
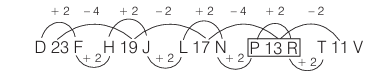
2. Z70B, D65F, H60J, …, P50R
(a) K55L
(b) L55M
(c) L55N
(d) L55P
Ans: (c)
Solution: The pattern of the series is
3. In the series AABABCABCDABCDE… which letter occupies the 100th position? UPSC (Civil Services) 2008
(a) H
(b) I
(c) J
(d) K
Ans: (b)
Solution: In this series, it contains 1, 2, 3, 4,… alphabets from beginning. In this sequence up to 13, it will be 91 letters. After that, 14 letters of the English alphabet will begin, in which the 9th letter, which will make the 100th (91+9th) from beginning, will be I.
4. Which number is not suitable in the following series? 1 9 36 81 99 121 (CGPSC 2014)
(a) 1
(b) 121
(c) 36
(d) 99
Ans: (d)
Solution: Given series is the series of square numbers. Thus, 99 is not suitable in the series.
5. What is the missing number ‘X’ of the series.
7, X, 21, 31, 43, ? UPSC (CSAT) 2015
(a) 11
(b) 12
(c) 13
(d) 14
Ans: (c)
Solution:
The pattern of the series is as follows:
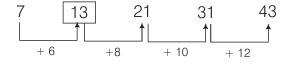
|
208 videos|272 docs|138 tests
|
FAQs on Overview: Number Series, Letter Series, Alpha-Numeric Series - CSAT Preparation - UPSC
| 1. What is a Number Series? |  |
| 2. How can one solve a Letter Series? |  |
| 3. What is an Alpha-Numeric Series? |  |
| 4. How can one approach solving a Type I Number Series question in a competitive exam like CSAT? |  |
| 5. What are some tips for improving performance in solving Alpha-Numeric Series questions in CSAT? |  |
















