Partial Molar Quantities | Chemistry Optional Notes for UPSC PDF Download
| Table of contents |

|
| Introduction |

|
| Partial molar volume |

|
| The total differential of the volume in an open system |

|
| Evaluation of partial molar volumes in binary mixtures |

|
Introduction
- The symbol Xi, where X is an extensive property of a homogeneous mixture and the subscript i identifies a constituent species of the mixture, denotes the partial molar quantity of species i defined by
 (9.2.1)(mixture)
(9.2.1)(mixture) - This is the rate at which property X changes with the amount of species i added to the mixture as the temperature, the pressure, and the amounts of all other species are kept constant. A partial molar quantity is an intensive state function. Its value depends on the temperature, pressure, and composition of the mixture.
- Keep in mind that as a practical matter, a macroscopic amount of a charged species (i.e., an ion) cannot be added by itself to a phase because of the huge electric charge that would result. Thus if species i is charged, Xi as defined by Eq. 9.2.1 is a theoretical concept whose value cannot be determined experimentally.
- An older notation for a partial molar quantity uses an overbar:
 . The notation X′i was suggested in the first edition of the IUPAC Green Book (Ian Mills et al, Quantities, Units and Symbols in Physical Chemistry, Blackwell, Oxford, 1988, p. 44), but is not mentioned in later editions.
. The notation X′i was suggested in the first edition of the IUPAC Green Book (Ian Mills et al, Quantities, Units and Symbols in Physical Chemistry, Blackwell, Oxford, 1988, p. 44), but is not mentioned in later editions.
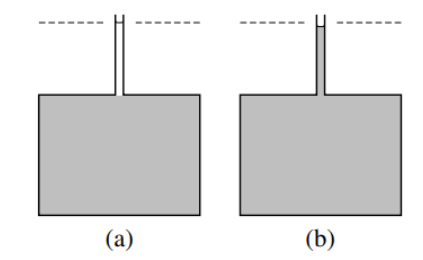
Figure 9.2.1: Addition of pure methanol (substance B) to a water-methanol mixture at constant T and p.
(a) 40.75 cm3 (one mole) of methanol is placed in a narrow tube above a much greater volume of a mixture (shaded) of composition xB = 0. The dashed line indicates the level of the upper meniscus.
(b) After the two liquid phases have mixed by diffusion, the volume of the mixture has increased by only 38.8 cm3.
Partial molar volume
- In order to gain insight into the significance of a partial molar quantity as defined by Eq. 9.2.1, let us first apply the concept to the volume of an open single-phase system. Volume has the advantage for our example of being an extensive property that is easily visualized. Let the system be a binary mixture of water (substance A) and methanol (substance B), two liquids that mix in all proportions. The partial molar volume of the methanol, then, is the rate at which the system volume changes with the amount of methanol added to the mixture at constant temperature and pressure: VB=(∂V/∂nB)T,p,nA
- At 25∘C and 1 bar, the molar volume of pure water is V∗m,A = 18.07cm3 mol−1 and that of pure methanol is V∗m,B = 40.75cm3 mol−1. If we mix 100.0cm3 of water at 25∘C with 100.0cm3 of methanol at 25∘C , we find the volume of the resulting mixture at 25∘C is not the sum of the separate volumes, 200.0cm3, but rather the slightly smaller value 193.1cm3. The difference is due to new intermolecular interactions in the mixture compared to the pure liquids.
Let us calculate the mole fraction composition of this mixture: (9.2.2), (9.2.3), (9.2.4)
(9.2.2), (9.2.3), (9.2.4) - Now suppose we prepare a large volume of a mixture of this composition (xB=0.307) and add an additional 40.75 cm3 (one mole) of pure methanol, as shown in Fig. 9.1(a). If the initial volume of the mixture at 25∘C was 10,000.0 cm3, we find the volume of the new mixture at the same temperature is 10,038.8 cm3, an increase of 38.8 cm3- see Fig. 9.1(b). The amount of methanol added is not infinitesimal, but it is small enough compared to the amount of initial mixture to cause very little change in the mixture composition: xB increases by only 0.5%. Treating the mixture as an open system, we see that the addition of one mole of methanol to the system at constant T,p, and nA causes the system volume to increase by 38.8 cm3. To a good approximation, then, the partial molar volume of methanol in the mixture, VB=(∂V/∂nB)T,p,nA, is given by ΔV/ΔnB=38.8 cm3 mol−1 .
- The volume of the mixture to which we add the methanol does not matter as long as it is large. We would have observed practically the same volume increase, 38.8 cm3, if we had mixed one mole of pure methanol with 100,000.0 cm3 of the mixture instead of only 10,000.0 cm3.
- Thus, we may interpret the partial molar volume of B as the volume change per amount of B added at constant T and p when B is mixed with such a large volume of mixture that the composition is not appreciably affected. We may also interpret the partial molar volume as the volume change per amount when an infinitesimal amount is mixed with a finite volume of mixture..
- The partial molar volume of B is an intensive property that is a function of the composition of the mixture, as well as of T and p. The limiting value of VB as xB approaches 1 (pure B) is V∗m,B , the molar volume of pure B . We can see this by writing V=nBV∗m,B for pure B, giving us

- If the mixture is a binary mixture of A and B , and xB is small, we may treat the mixture as a dilute solution of solvent A and solute B. As xB approaches 0 in this solution, VB approaches a certain limiting value that is the volume increase per amount of B mixed with a large amount of pure A. In the resulting mixture, each solute molecule is surrounded only by solvent molecules. We denote this limiting value of VB by V∞B, the partial molar volume of solute B at infinite dilution.
It is possible for a partial molar volume to be negative. Magnesium sulfate, in aqueous solutions of molality less than 0.07 mol kg−1, has a negative partial molar volume. Physically, this means that when a small amount of crystalline MgSO4 dissolves at constant temperature in water, the liquid phase contracts. This unusual behavior is due to strong attractive water-ion interactions.
The total differential of the volume in an open system
Consider an open single-phase system consisting of a mixture of nonreacting substances. How many independent variables does this system have?
- We can prepare the mixture with various amounts of each substance, and we are able to adjust the temperature and pressure to whatever values we wish (within certain limits that prevent the formation of a second phase). Each choice of temperature, pressure, and amounts results in a definite value of every other property, such as volume, density, and mole fraction composition. Thus, an open single-phase system of C substances has 2+C independent variables. 3
Footnote
3. C in this kind of system is actually the number of components. The number of components is usually the same as the number of substances, but is less if certain constraints exist, such as reaction equilibrium or a fixed mixture composition. The general meaning of C will be discussed in Sec. 13.
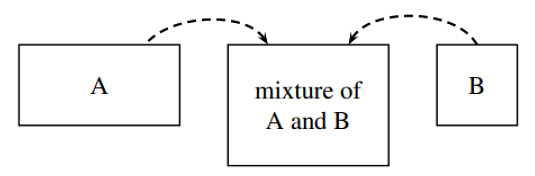
Figure 9.2.2 : Mixing of water (A) and methanol (B) in a 2:1 ratio of volumes to form a mixture of increasing volume and constant composition. The system is the mixture.
For a binary mixture (C=2) , the number of independent variables is four. We may choose these variables to be T,p,nA, and nB , and write the total differential of V in the general form
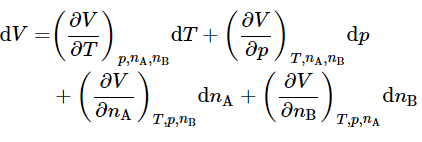 (binary mixture)
(binary mixture)
We know the first two partial derivatives on the right side are given by4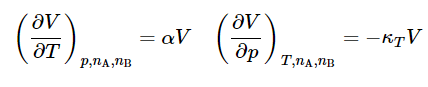
We identify the last two partial derivatives on the right side of Eq. 9.2.5 as the partial molar volumes VA and VB . Thus, we may write the total differential of V for this open system in the compact form
dV = αV dT−κTV dp + VAdnA+VBdnB (9.2.2)
(binary mixture)
If we compare this equation with the total differential of V for a one-component closed system, dV=αV dT−κTV dp (Eq. 7.1.6), we see that an additional term is required for each constituent of the mixture to allow the system to be open and the composition to vary.
When T and p are held constant, Eq. 9.2.7 becomes
dV = VAdnA+VBdnB (9.2.3)
(binary mixture, constant T and p)
We obtain an important relation between the mixture volume and the partial molar volumes by imagining the following process. Suppose we continuously pour pure water and pure methanol at constant but not necessarily equal volume rates into a stirred, thermostatted container to form a mixture of increasing volume and constant composition, as shown schematically in Fig. 9.2. If this mixture remains at constant T and p as it is formed, none of its intensive properties change during the process, and the partial molar volumes VA and VB remain constant. Under these conditions, we can integrate Eq. 9.2.8 to obtain the additivity rule for volume: 5
V = VAnA + VBnB (9.2.4)
(binary mixture)
This equation allows us to calculate the mixture volume from the amounts of the constituents and the appropriate partial molar volumes for the particular temperature, pressure, and composition.
For example, given that the partial molar volumes in a water-methanol mixture of composition xB = 0.307 are VA=17.74 cm3 mol−1 and VB = 38.76 cm3 mol−1, we calculate the volume of the water-methanol mixture described at the beginning of Sec. 9.2.1 as follows:
V=(17.74 cm3 mol−1)(5.53 mol)+(38.76 cm3 mol−1)(2.45 mol)=193.1 cm3
We can differentiate Eq. 9.2.9 to obtain a general expression for dV under conditions of constant T and p:
dV = VAdnA+VBdnB+nAdVA+nBdVB (9.2.5)
But this expression for dV is consistent with Eq. 9.2.8 only if the sum of the last two terms on the right is zero:
nAdVA+nBdVB=0 (9.2.6)
(binary mixture, constant T and p)
Equation 9.2.12 is the Gibbs-Duhem equation for a binary mixture, applied to partial molar volumes. (Section 9.2.4 will give a general version of this equation.) Dividing both sides of the equation by nA+nB gives the equivalent form
xAdVA + xBdVB = 0 (9.2.7)
(binary mixture, constant T and p)
Equation 9.2.12 shows that changes in the values of VA and VB are related when the composition changes at constant T and p. If we rearrange the equation to the form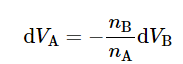 (9.2.8)
(9.2.8)
(binary mixture, constant T and p)
we see that a composition change that increases VB (so that dVB is positive) must make VA decrease.
Evaluation of partial molar volumes in binary mixtures
The partial molar volumes VA and VB in a binary mixture can be evaluated by the method of intercepts. To use this method, we plot experimental values of the quantity V/n (where n is nA+nB) versus the mole fraction xB.V/n is called the mean molar volume.
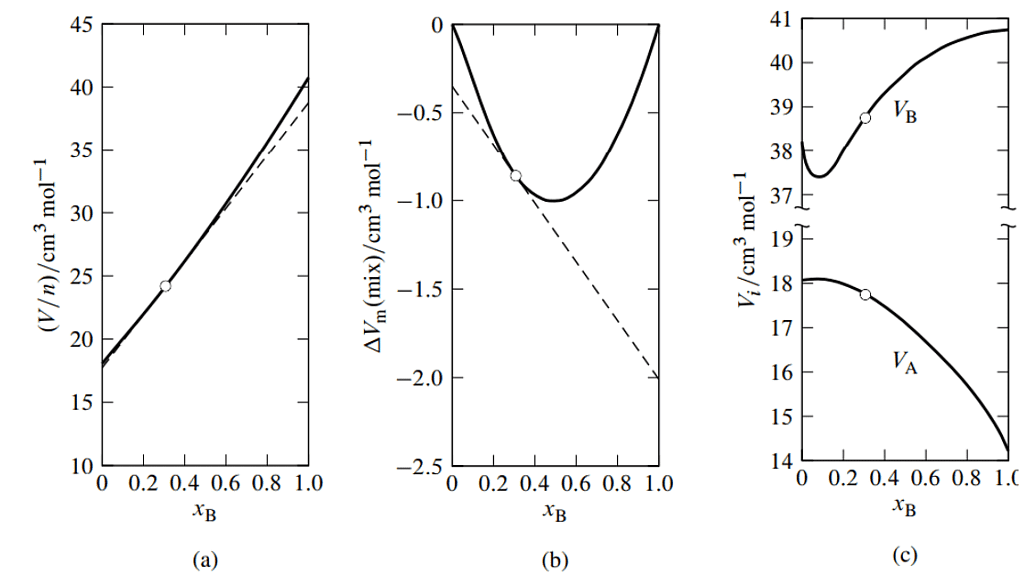
Figure 9.2.3: Figure 9.3 Mixtures of water (A) and methanol (B) at 25∘C and 1 bar. a
- Mean molar volume as a function of xB. The dashed line is the tangent to the curve at xB=0.307.
- Molar volume of mixing as a function of xB. The dashed line is the tangent to the curve at xB=0.307.
- Partial molar volumes as functions of xB. The points at xB=0.307 (open circles) are obtained from the intercepts of the dashed line in either (a) or (b).
Fig. 9.3(a) for an example. In this figure, the tangent to the curve drawn at the point on the curve at the composition of interest (the composition used as an illustration in Sec. 9.2.1) intercepts the vertical line where xB equals 0 at V/n=VA=17.7cm3 mol−1 , and intercepts the vertical line where xB equals 1 at V/n = VB = 38.8cm3 mol−1.
To derive this property of a tangent line for the plot of V/n versus xB, we use Eq. 9.2.9 to write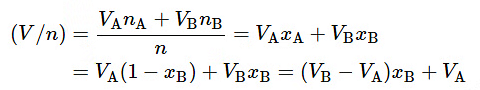 (9.2.15)
(9.2.15)
5The equation is an example of the result of applying Euler's theorem on homogeneous functions to V treated as a function of nA and nB.
When we differentiate this expression for V/n with respect to xB, keeping in mind that VA and VB are functions of xB , we obtain
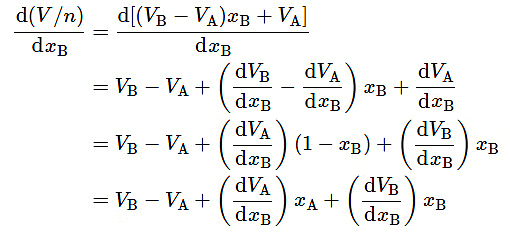 (9.2.16)
(9.2.16)
The differentials dVA and dVB are related to one another by the Gibbs–Duhem equation (Eq. 9.2.13): xAdVA + xBdVB = 0 . We divide both sides of this equation by dxB to obtain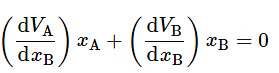 (9.2.17)
(9.2.17)
and substitute in Eq. 9.2.16 to obtain
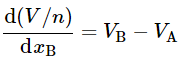 (9.2.18)
(9.2.18)
Let the partial molar volumes of the constituents of a binary mixture of arbitrary composition x′B′ be V′A′ and V′B′ . Equation 9.2.15 shows that the value of V/n at the point on the curve of V/n versus xB where the composition is x′B′ is (V′B−V′A)x′B+V′A. Equation 9.2.18 shows that the tangent to the curve at this point has a slope of V′B−V′A . The equation of the line that passes through this point and has this slope, and thus is the tangent to the curve at this point, is y=(V′B−V′A)xB+V′A, where y is the vertical ordinate on the plot of (V/n) versus xB. The line has intercepts y=V′A′ at xB=0 and y=V′B′ at xB=1 .
A variant of the method of intercepts is to plot the molar integral volume of mixing given by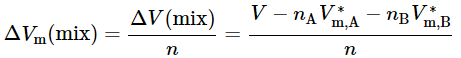 (9.2.19)
(9.2.19)
versus xB, as illustrated in Fig. 9.3(b). ΔV(mix) is the integral volume of mixing—the volume change at constant T and p when solvent and solute are mixed to form a mixture of volume V and total amount n (see Sec. 11.1.1). The tangent to the curve at the composition of interest has intercepts VA−V∗m,A at xB = 0 and VB−V∗m,B at xB=1.
To see this, we write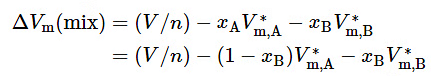 (9.2.20)
(9.2.20)
We make the substitution (V/n)=(VB−VA)xB+VA from Eq. 9.2.15 and rearrange: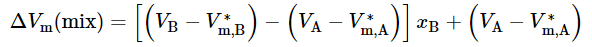 (9.2.21)
(9.2.21)
Differentiation with respect to xB yields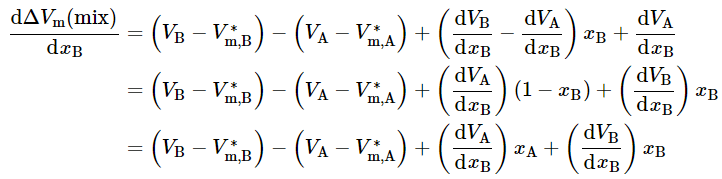
(9.2.22)
With a substitution from Eq. 9.2.17, this becomes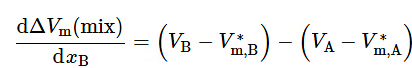 (9.2.23)
(9.2.23)
Equations 9.2.21 and 9.2.23 are analogous to Eqs. 9.2.15 and 9.2.18, with V/n replaced by ΔVm(mix) , VA by (VA−V∗m,A) , and VB by (VB−V∗m,B). Using the same reasoning as for a plot of V/n versus xB, we find the intercepts of the tangent to a point on the curve of ΔVm(mix) versus xB are at VA−V∗m,A and VB−V∗m,B .
- Figure 9.3 shows smoothed experimental data for water–methanol mixtures plotted in both kinds of graphs, and the resulting partial molar volumes as functions of composition. Note in Fig. 9.3(c) how the VA curve mirrors the VB curve as xB varies, as predicted by the Gibbs–Duhem equation. The minimum in VB at xB≈0.09 is mirrored by a maximum in VA in agreement with Eq. 9.2.14; the maximum is much attenuated because nB/nA is much less than unity.
- Macroscopic measurements are unable to provide unambiguous information about molecular structure. Nevertheless, it is interesting to speculate on the implications of the minimum observed for the partial molar volume of methanol. One interpretation is that in a mostly aqueous environment, there is association of methanol molecules, perhaps involving the formation of dimers.
General relations
- The discussion above of partial molar volumes used the notation V∗m,A and V∗m,B for the molar volumes of pure A and B. The partial molar volume of a pure substance is the same as the molar volume, so we can simplify the notation by using V∗A and V∗B instead. Hereafter, this e-book will denote molar quantities of pure substances by such symbols as V∗A , H∗B , and S∗i.
- The relations derived above for the volume of a binary mixture may be generalized for any extensive property X of a mixture of any number of constituents. The partial molar quantity of species i, defined by
 (9.2.24)
(9.2.24)
is an intensive property that depends on T, p, and the composition of the mixture. The additivity rule for property X is (9.2.25)(mixture)
(9.2.25)(mixture) - and the Gibbs–Duhem equation applied to X can be written in the equivalent forms
 (9.2.26)(constant T and p)
(9.2.26)(constant T and p)
and
- These relations can be applied to a mixture in which each species i is a nonelectrolyte substance, an electrolyte substance that is dissociated into ions, or an individual ionic species. In Eq. 9.2.27, the mole fraction xi must be based on the different species considered to be present in the mixture. For example, an aqueous solution of NaCl could be treated as a mixture of components A=H2O and B=NaCl, with xB equal to nB/(nA+nB) ; or the constituents could be taken as H2O, Na +, and Cl −, in which case the mole fraction of Na+ + would be x+ = n+/(nA+ n+ + n−).
- A general method to evaluate the partial molar quantities XA and XB in a binary mixture is based on the variant of the method of intercepts described in Sec. 9.2.3. The molar mixing quantity ΔX(mix)/n/ is plotted versus xB, where ΔX(mix) is (X−nAX∗A−nBX∗B) . On this plot, the tangent to the curve at the composition of interest has intercepts equal to XA−X∗A at xB=0 and XB−X∗B at xB=1.
- We can obtain experimental values of such partial molar quantities of an uncharged species as Vi , Cp,i , and Si. It is not possible, however, to evaluate the partial molar quantities Ui, Hi , Ai , and Gi because these quantities involve the internal energy brought into the system by the species, and we cannot evaluate the absolute value of internal energy (Sec. 2.6.2). For example, while we can evaluate the difference Hi−H∗i from calorimetric measurements of enthalpies of mixing, we cannot evaluate the partial molar enthalpy Hi itself. We can, however, include such quantities as Hi in useful theoretical relations.
- A partial molar quantity of a charged species is something else we cannot evaluate. It is possible, however, to obtain values relative to a reference ion. Consider an aqueous solution of a fully-dissociated electrolyte solute with the formula Mν+Xν−, where ν+ and ν− are the numbers of cations and anions per solute formula unit. The partial molar volume VB of the solute, which can be determined experimentally, is related to the (unmeasurable) partial molar volumes V+ and V− of the constituent ions by
VB = ν+V+ + ν−V− (9.2.28) - For aqueous solutions, the usual reference ion is H + , and the partial molar volume of this ion at infinite dilution is arbitrarily set equal to zero:

For example, given the value (at 298.15K and 1bar) of the partial molar volume at infinite dilution of aqueous hydrogen chloride (9.2.29)
(9.2.29)
we can find the so-called “conventional” partial molar volume of Cl− ion: (9.2.30)
(9.2.30)
Going one step further, the measured value gives, for Na + ion, the conventional value
gives, for Na + ion, the conventional value (9.2.31)
(9.2.31)
FAQs on Partial Molar Quantities - Chemistry Optional Notes for UPSC
| 1. What is partial molar volume? |  |
| 2. How is the total differential of volume defined in an open system? |  |
| 3. How can we evaluate partial molar volumes in binary mixtures? |  |
| 4. Why are partial molar volumes important in studying mixtures? |  |
| 5. What applications can be derived from the evaluation of partial molar volumes? |  |

|
Explore Courses for UPSC exam
|

|
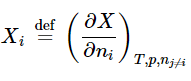 (9.2.1)(mixture)
(9.2.1)(mixture)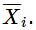 . The notation X′i was suggested in the first edition of the IUPAC Green Book (Ian Mills et al, Quantities, Units and Symbols in Physical Chemistry, Blackwell, Oxford, 1988, p. 44), but is not mentioned in later editions.
. The notation X′i was suggested in the first edition of the IUPAC Green Book (Ian Mills et al, Quantities, Units and Symbols in Physical Chemistry, Blackwell, Oxford, 1988, p. 44), but is not mentioned in later editions.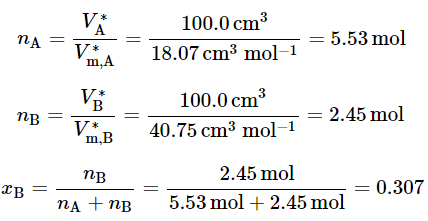 (9.2.2), (9.2.3), (9.2.4)
(9.2.2), (9.2.3), (9.2.4)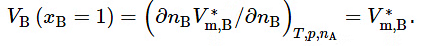
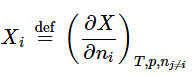 (9.2.24)
(9.2.24)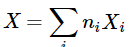 (9.2.25)(mixture)
(9.2.25)(mixture)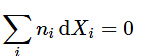 (9.2.26)(constant T and p)
(9.2.26)(constant T and p)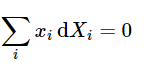
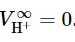
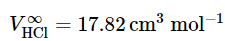 (9.2.29)
(9.2.29)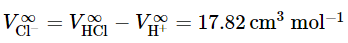 (9.2.30)
(9.2.30)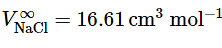 gives, for Na + ion, the conventional value
gives, for Na + ion, the conventional value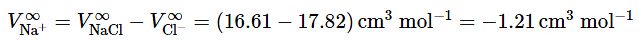 (9.2.31)
(9.2.31)















