Ex-2.1 Polynomials, Class 10, Maths RD Sharma Solutions PDF Download
Q.1: Find the zeroes of each of the following quadratic polynomials and verify the relationship between the zeroes and their coefficients:
(i) f(x) = x2 – 2x – 8
(ii) g(s) = 4s2 – 4s + 1
(iii) 6x2 – 3 – 7x
(iv) h(t) = t2 – 15
(v) p(x) = x2 + 2√2x – 6
(vi) q(x) = √3x2 + 10x + 7√3
(vii) f(x) = x2 – (√3 + 1)x + √3
(viii) g(x) = a(x2 + 1) – x(a2 + 1)
Solution: (i) f(x) = x2 – 2x – 8
We have,
f(x) = x2 – 2x – 8
= x2 – 4x + 2x – 8
= x(x – 4) + 2(x – 4)
= (x + 2)(x – 4)
Zeroes of the polynomials are -2 and 4.
Now,
Sum of the zeroes = 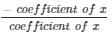
-2 + 4 = −(−2)/1
2 = 2
Product of the zeroes = 
-8 = −8/1
-8 = -8
Hence, the relationship is verified.
(ii) g(s) = 4s2 – 4s + 1
We have,
g(s) = 4s2 – 4s + 1
= 4s2 – 2s – 2s + 1
= 2s(2s – 1)−1(2s – 1)
= (2s – 1)(2s – 1)
Zeroes of the polynomials are 1/2 and 1/2.
Sum of zeroes = 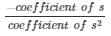
1/2 + 1/2 = −(−4)/4
1 = 1
Product of zeroes = 
1/2 × 1/2 = 1/4
1/4 = 1/4
Hence, the relationship is verified.
(iii) 6s2−3−7x
= 6s2−7x−3 = (3x + 11)(2x – 3)
Zeros of the polynomials are 3/2 and −1/3
Sum of the zeros = 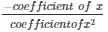
−1/3 + 3/2 = −(−7)/6
7/6 = 7/6
Product of the zeroes = 
−1/3 × 3/2 = −3/6
−3/6 = −3/6
Hence, the relationship is verified.
(iv) h(t) = t2 – 15
We have,
h(t) = t2 – 15
= t2 – 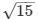
= (t + 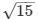 )(t –
)(t – 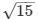 )
)
Zeroes of the polynomials are −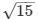 and
and 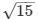
Sum of the zeroes = 0
−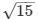 +
+ 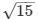 = 0
= 0
0 = 0
Product of zeroes = 
−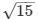 ×
×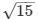 = −15
= −15
-15 = -15
Hence, the relationship verified.
(v) p(x) = x2 + 2√2x – 6
We have,
p(x) = x2 + 2√2x – 6
= x2 + 3√2x + 3√2x – 6
= x(x + 3√2) – √2(x + 3√2)
= (x + 3√2)(x – √2)
Zeroes of the polynomials are 3√2 and −3√2
Sum of the zeroes = −2√2/1
√2 – 3√2 = −2√2
– 2√2 = −2√2
Product of the zeroes = 
√2×−3√2 = −6/1
-6 = -6
Hence, the relationship is verified.
(vi) q(x) = √3x2 + 10x + 7√3
= √3x2 + 7x + 3x + 7√3
= √3x(x + √3)7(x + sqrt3)
= (x + √3)(7 + sqrt3)
Zeros of the polynomials are −√3 and −7/3√
Sum of zeros = −10√3
−√3−7/√3 = −10/√3
−10/√3 = −10/√3
Product of the polynomials are −√3,−7/√3
7 = 7
Hence, the relationship is verified.
(vii) h(x) = x2−(√3 + 1)x + √3
= x2−√3−x + √3
= x(x – √3)−1(x−√3)
= (x – √3)(x−1)
Zeros of the polynomials are 1 and √3
Sum of zeros = 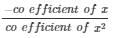 = −[−√3−1]
= −[−√3−1]
1 + √3 = √3 + 1
Product of zeros = 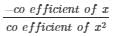 = √3
= √3
√3 = √3
Hence, the relationship is verified
(viii) g(x) = a[(x2 + 1) – x(a2 + 1)]2
= ax2 + a−a2x−x
= ax2−[(a2x + 1)] + a
= ax2−a2x – x + a
= ax(x−a)−1(x – a) = (x – a)(ax – 1)
Zeros of the polynomials are 1/a and 1
Sum of the zeros = 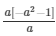

Product of zeros = a/a
1/a×a = a/a
1 = 1
Hence, the relationship is verified.
Q.2: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – 5x + 4, find the value of 1/α + 1/β – 2αβ.
Solution: We have,
α and β are the roots of the quadratic polynomial.
f(x) = x2 – 5x + 4
Sum of the roots = α + β = 5
Product of the roots = αβ = 4
So,
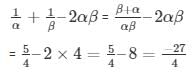
Q.3: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – 5x + 4, find the value of 1/α + 1/β – 2αβ.
Solution: Since, α and β are the zeroes of the quadratic polynomial.
p(y) = x2 – 5x + 4
Sum of the zeroes = α + β = 5
Product of the roots = αβ = 4
So,
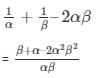
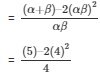
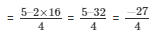
Q.4: If α and β are the zeroes of the quadratic polynomial p(y) = 5y2 – 7y + 1, find the value of 1/α + 1/β.
Solution: Since, α and β are the zeroes of the quadratic polynomial.
p(y) = 5y2 – 7y + 1
Sum of the zeroes = α + β = 7
Product of the roots = αβ = 1
So,
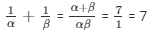
Q.5: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – x – 4, find the value of 1/α + 1/β – αβ.
Solution: Since, α and β are the zeroes of the quadratic polynomial.
We have,
f(x) = x2 – x – 4
Sum of zeroes = α + β = 1
Product of the zeroes = αβ = -4
So,
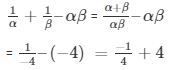
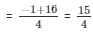
Q.6: If α and β are the zeroes of the quadratic polynomial f(x) = x2 + x – 2, find the value of 1/α – 1/β.
Solution: Since, α and β are the zeroes of the quadratic polynomial.
We have,
f(x) = x2 + x – 2
Sum of zeroes = α + β = 1
Product of the zeroes = αβ = -2
So,
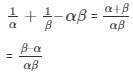
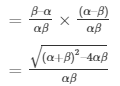
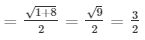
Q.7: If one of the zero of the quadratic polynomial f(x) = 4x2 – 8kx – 9 is negative of the other, then find the value of k.
Solution: Let, the two zeroes of the polynomial f(x) = 4x2 – 8kx – 9 be α and −α.
Product of the zeroes = α x −α = -9
Sum of the zeroes = α + (−α) = -8k = 0 Since,α – α = 0
⇒ 8k = 0
⇒ k = 0
Q.8: If the sum of the zeroes of the quadratic polynomial f(t) = kt2 + 2t + 3k is equal to their product, then find the value of k.
Solution: Let the two zeroes of the polynomial f(t) = kt2 + 2t + 3k be α and β.
Sum of the zeroes = α + β = 2
Product of the zeroes = α × β = 3k
Now,
−2/k = 3k/k
⇒ 3k = −2
⇒ k = −2/3
So, k = 0 and ⇒ k = −2/3
Q.9: If α and β are the zeroes of the quadratic polynomial p(x) = 4x2 – 5x – 1, find the value of α2β + αβ2.
Solution: Since, α and β are the zeroes of the quadratic polynomial p(x) = 4x2 – 5x – 1
So, Sum of the zeroes = α + β = 5/4
Product of the zeroes = α × β = −1/4
Now,
α2β + αβ2 = αβ(α + β)
= 5/4(−1/4)
= −5/16
Q.10: If α and β are the zeroes of the quadratic polynomial f(t) = t2 – 4t + 3, find the value of α4β3 + α3β4.
Solution: Since, α and β are the zeroes of the quadratic polynomial f(t) = t2 – 4t + 3
So, Sum of the zeroes = α + β = 4
Product of the zeroes = α × β = 3
Now,
α4β3 + α3β4 = α3β3(α + β)
= (3)3(4) = 108
Q.11: If α and β are the zeroes of the quadratic polynomial f(x) = 6x2 + x – 2, find the value of α/β + β/α.
Solution: Since, α and β are the zeroes of the quadratic polynomial f(x) = 6x2 + x – 2.
Sum of the zeroes = α + β = −1/6
Product of the zeroes = α × β = −1/3
Now,
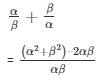
By substitution the values of the sum of zeroes and products of the zeroes, we will get
= −25/12
Q.12: If α and β are the zeroes of the quadratic polynomial f(x) = 6x2 + x – 2, find the value of 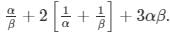
Solution: Since, α and β are the zeroes of the quadratic polynomial f(x) = 6x2 + x – 2.
Sum of the zeroes = α + β = 6/3
Product of the zeroes = α × β = 4/3
Now,
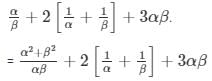
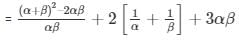
By substituting the values of sum and product of the zeroes, we will get
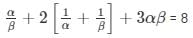
Q.13: If the squared difference of the zeroes of the quadratic polynomial f(x) = x2 + px + 45 is equal to 144, find the value of p.
Solution: Let the two zeroes of the polynomial be α and β.
We have,
f(x) = x2 + px + 45
Now,
Sum of the zeroes = α + β = -p
Product of the zeroes = α × β = 45
So,
(α + β)2 – 4αβ = 144
(p)2 – 4 × 45 = 144
(p)2 = 144 + 180
(p)2 = 324
p = 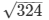
p = ±18
Thus, in the given equation, p will be either 18 or -18.
Q.14: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – px + q, prove that 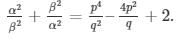
Solution: Since, α and β are the roots of the quadratic polynomial given in the question.
f(x) = x2 – px + q
Now,
Sum of the zeroes = p = α + β
Product of the zeroes = q = α × β
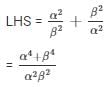
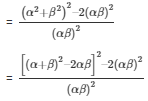
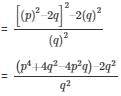
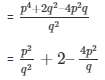
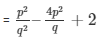
LHS = RHS
Hence, proved.
Q.15: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – p(x + 1) – c, show that (α + 1)(β + 1) = 1 – c.
Solution: Since, α and β are the zeroes of the quadratic polynomial
f(x) = x2 – p(x + 1) – c
Now,
Sum of the zeroes = α + β = p
Product of the zeroes = α × β = (- p – c)
So,
(α + 1)(β + 1)
= αβ + α + β + 1
= αβ + (α + β) + 1
= (−p – c) + p + 1
= 1 – c = RHS
So, LHS = RHS
Hence, proved.
Q.16: If α and β are the zeroes of the quadratic polynomial such that α + β = 24 and α – β = 8, find a quadratic polynomial having α and β as its zeroes.
Solution: We have,
α + β = 24 …………E-1
α – β = 8 ………….E-2
By solving the above two equations accordingly, we will get
2α = 32
α = 16
Substitute the value of α, in any of the equation. Let we substitute it in E-2, we will get
β = 16 – 8
β = 8
Now,
Sum of the zeroes of the new polynomial = α + β = 16 + 8 = 24
Product of the zeroes = αβ = 16 × 8 = 128
Then, the quadratic polynomial is-
K x2 – (sumofthezeroes)x + (productofthezeroes) = x2 – 24x + 128
Hence, the required quadratic polynomial is f(x) = x2 + 24x + 128
Q.17: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – 1, find a quadratic polynomial whose zeroes are 2α/β and 2β/α.
Solution: We have,
f(x) = x2 – 1
Sum of the zeroes = α + β = 0
Product of the zeroes = αβ = -1
From the question,
Sum of the zeroes of the new polynomial = 2α/β and 2β/α
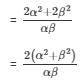
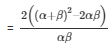
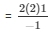 { By substituting the value of the sum and products of the zeroes }
{ By substituting the value of the sum and products of the zeroes }
As given in the question,
Product of the zeroes = 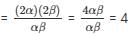
Hence, the quadratic polynomial is
x2 – (sumofthezeroes)x + (productofthezeroes)
= kx2 – (−4)x + 4 = x2 + 4x + 4
Hence, the required quadratic polynomial is f(x) = x2 + 4x + 4
Q.18: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – 3x – 2, find a quadratic polynomial whose zeroes are 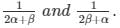
Solution: We have,
f(x) = x2 – 3x – 2
Sum of the zeroes = α + β = 3
Product of the zeroes = αβ = -2
From the question,
Sum of the zeroes of the new polynomial 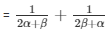
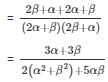
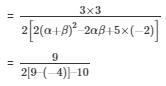
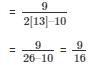
Product of the zeroes = 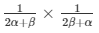
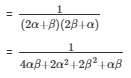
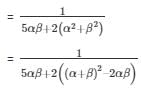
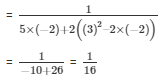
So, the quadratic polynomial is,
x2 – (sumofthezeroes)x + (productofthezeroes)
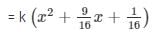
Hence, the required quadratic polynomial is k 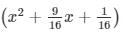
Q.19: If α and β are the zeroes of the quadratic polynomial f(x) = x2 + px + q, form a polynomial whose zeroes are (α + β)2 and (α – β)2.
Solution: We have,
f(x) = x2 + px + q
Sum of the zeroes = α + β = -p
Product of the zeroes = αβ = q
From the question,
Sum of the zeroes of new polynomial = (α + β)2 + (α – β)2
= (α + β)2 + α2 + β2 – 2αβ
= (α + β)2 + (α + β)2 – 2αβ – 2αβ
= (−p)2 + (−p)2 – 2×q – 2×q
= p2 + p2 – 4q
= 2p2 – 4q
Product of the zeroes of new polynomial = (α + β)2(α – β)2
= (−p)2((−p)2 – 4q)
= p2(p2 – 4q)
So, the quadratic polynomial is ,
x2 – (sumofthezeroes)x + (productofthezeroes)
= x2 – (2p2 – 4q)x + p2(p2 – 4q)
Hence, the required quadratic polynomial is f(x) = k(x2 – (2p2 – 4q)x + p2(p2 – 4q)).
Q.20: If α and β are the zeroes of the quadratic polynomial f(x) = x2 – 2x + 3, find a polynomial whose roots are:
(i) α + 2,β + 2
(ii) 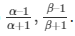
Solution: We have,
f(x) = x2 – 2x + 3
Sum of the zeroes = α + β = 2
Product of the zeroes = αβ = 3
(i) Sum of the zeroes of new polynomial = (α + 2) + (β + 2)
= α + β + 4
= 2 + 4 = 6
Product of the zeroes of new polynomial = (α + 1)(β + 1)
= αβ + 2α + 2β + 4
= αβ + 2(α + β) + 4 = 3 + 2(2) + 4 = 11
So, quadratic polynomial is:
x2 – (sumofthezeroes)x + (productofthezeroes)
= x2 – 6x + 11
Hence, the required quadratic polynomial is f(x) = k(x2 – 6x + 11)
(ii) Sum of the zeroes of new polynomial = 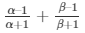
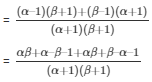
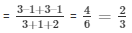
Product of the zeroes of new polynomial = 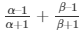
= 2/6 = 1/3
So, the quadratic polynomial is,
x2 – (sumofthezeroes)x + (productofthezeroes)
= 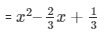
Thus, the required quadratic polynomial is f(x) = 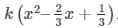
Q.21: If α and β are the zeroes of the quadratic polynomial f(x) = ax2 + bx + c, then evaluate:
(i) α – β
(ii) 1/α – 1/β
(ii) 1/α + 1/β – 2αβ
(iv) α2β + αβ2
(v) α4 + β4
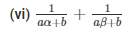
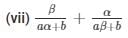
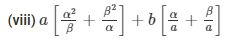
Solution:
f(x) = ax2 + bx + c
Here,
Sum of the zeroes of polynomial = α + β = −b/a
Product of zeroes of polynomial = αβ = c/a
Since, α + β are the roots (or) zeroes of the given polynomial, so
(i) α – β
The two zeroes of the polynomials are-
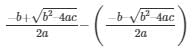
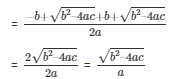
(ii) 1/α – 1/β
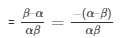 ….. E.1
….. E.1
From previous question we know that,
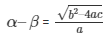
Also,
αβ = c/a
Putting the values in E.1, we will get
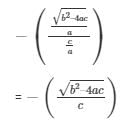
(iii) 1/α + 1/β – 2αβ
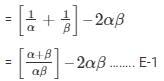
Since,
Sum of the zeroes of polynomial = α + β = −b/a
Product of zeroes of polynomial = αβ = c/a
After substituting it in E-1, we will get
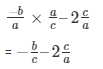
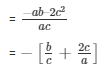
(iv) α2β + αβ2
= αβ(α + β) …….. E-1.
Since,
Sum of the zeroes of polynomial = α + β = −b/a
Product of zeroes of polynomial = αβ = c/a
After substituting it in E-1, we will get
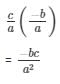
(v) α4 + β4
= (α2 + β2)2 – 2α2β2
= ((α + β)2 – 2αβ)2 – (2αβ)2 ……. E- 1
Since,
Sum of the zeroes of polynomial = α + β = −b/a
Product of zeroes of polynomial = αβ = c/a
After substituting it in E-1, we will get
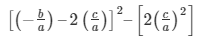
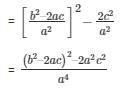
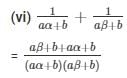
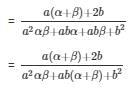
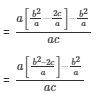
Since,
Sum of the zeroes of polynomial = α + β = −b/a
Product of zeroes of polynomial = αβ = c/a
After substituting it , we will get
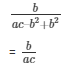
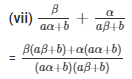
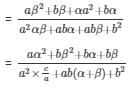
Since,
Sum of the zeroes of polynomial = α + β = −b/a
Product of zeroes of polynomial = αβ = c/a
After substituting it , we will get
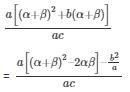
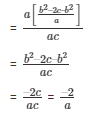
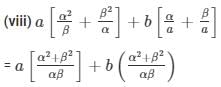
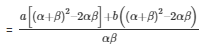
Since,
Sum of the zeroes of polynomial = α + β = −b/a
Product of zeroes of polynomial = αβ = c/a
After substituting it , we will get
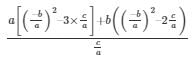
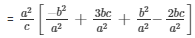
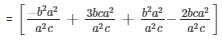
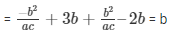
FAQs on Ex-2.1 Polynomials, Class 10, Maths RD Sharma Solutions
| 1. What is the importance of studying polynomials in Class 10 Mathematics? |  |
| 2. How can I identify a polynomial expression? |  |
| 3. What are the different types of polynomials? |  |
| 4. How do I add or subtract polynomials? |  |
| 5. What is the degree of a polynomial and how is it determined? |  |





















