Ratio And Proportion | Quantitative Techniques for CLAT PDF Download
| Table of contents |

|
| Ratio |

|
| Proportion |

|
| Ratio Formula |

|
| Proportion Formula |

|
| Difference Between Ratio and Proportion |

|
Ratio
In certain situations, the comparison of two quantities by the method of division is very efficient. We can say that the comparison or simplified form of two quantities of the same kind is referred to as a ratio. This relation gives us how many times one quantity is equal to the other quantity. In simple words, the ratio is the number that can be used to express one quantity as a fraction of the other ones.
The two numbers in a ratio can only be compared when they have the same unit. We make use of ratios to compare two things. The sign used to denote a ratio is ‘:’.

Ratios can be represented in three different forms, such as:
- a to b
- a : b
- a/b
Notes
- The ratio should exist between the quantities of the same kind.
- While comparing two things, the units should be similar.
- There should be significant order of terms.
- The comparison of two ratios can be performed, if the ratios are equivalent like the fractions.
Proportion
Proportion is an equation that defines that the two given ratios are equivalent to each other. In other words, the proportion states the equality of the two fractions or the ratios. In proportion, if two sets of given numbers are increasing or decreasing in the same ratio, then the ratios are said to be directly proportional to each other.
 For example, The speed of a train covering 100 km in 1 hour is equal to the speed when covering 500 km in 5 hours, as both yield 100 km/hr.
For example, The speed of a train covering 100 km in 1 hour is equal to the speed when covering 500 km in 5 hours, as both yield 100 km/hr.
Ratio and proportions are said to be faces of the same coin. When two ratios are equal in value, then they are said to be in proportion. In simple words, it compares two ratios. Proportions are denoted by the symbol ‘::’ or ‘=’.
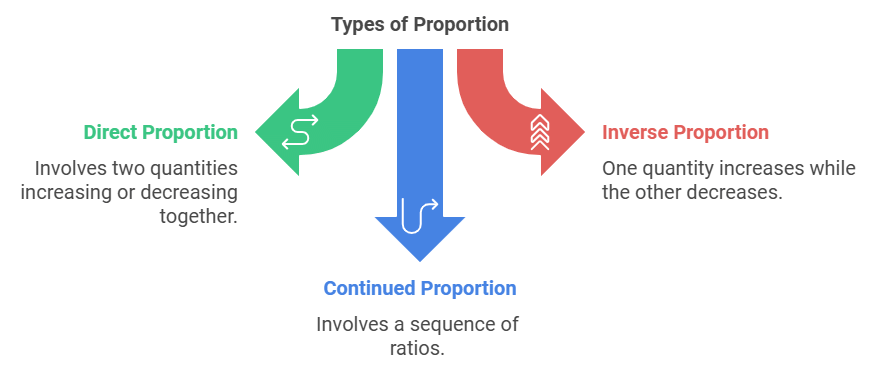
The proportion can be classified into the following categories, such as:
- Direct Proportion
- Inverse Proportion
- Continued Proportion
Direct Proportion
The direct proportion describes the relationship between two quantities, in which the increase in one quantity leads to an increase in the other quantity also. Similarly, if one quantity decreases, the other quantity also decreases. Hence, if “a” and “b” are two quantities, then the direction proportion is written as a ∝ b.
Inverse Proportion
The inverse proportion describes the relationship between two quantities in which an increase in one quantity leads to a decrease in the other quantity. Similarly, if there is a decrease in one quantity, there is an increase in the other quantity. Therefore, the inverse proportion of two quantities, say “a” and “b” is represented by a ∝ (1/b).
Continued Proportion
In a continued proportion, for three quantities a, b, and c, the ratio a : b = b : c. For two ratios a : b and c : d, a continued proportion can be formed by finding a common middle term, often using the LCM of the means (b and c)
Ratio Formula
Assume that, we have two quantities (or two numbers or two entities) and we have to find the ratio of these two, then the formula for ratio is defined as;
a : b ⇒ a/b
where a and b could be any two quantities.
Here, “a” is called the first term or antecedent, and “b” is called the second term or consequent.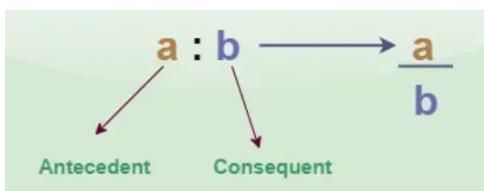 Example: In ratio 4 : 3, is represented by 4/9, where 4 is the antecedent and 3 is the consequent.
Example: In ratio 4 : 3, is represented by 4/9, where 4 is the antecedent and 3 is the consequent.
If we multiply and divide each term of ratio by the same number (non-zero), it doesn’t affect the ratio.
Example: 4 : 9 = 8 : 18 = 12 : 27
Proportion Formula
Now, let us assume that, in proportion, the two ratios are a:b & c:d. The two terms ‘b’ and ‘c’ are called ‘means or mean term,’ whereas the terms ‘a’ and ‘d’ are known as ‘extremes or extreme terms.’
a/b = c/d or a : b :: c : d
Example: Let us consider one more example of a number of students in a classroom. Our first ratio of the number of girls to boys is 3:5 and that of the other is 4:8, then the proportion can be written as:
3 : 5 :: 4 : 8 or 3/5 = 4/8
Here, 3 & 8 are the extremes, while 5 & 4 are the means.
Note: The ratio value does not affect when the same non-zero number is multiplied or divided on each term.
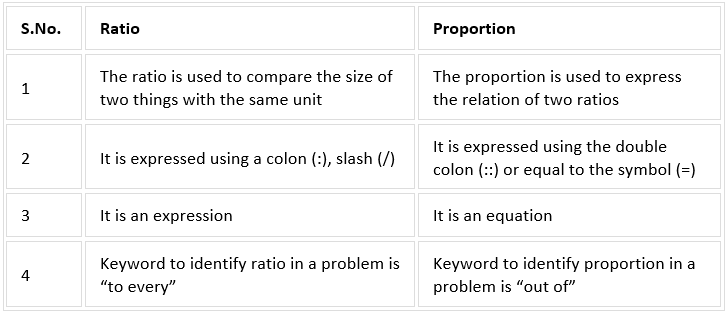
Difference Between Ratio and Proportion
Some Useful Results on Proportion
If a, b, c and d are four quantities. then,
(i) a:b :: c:d means b:a :: d:c (invertendo)
(ii) a:b :: c:d means a:c :: b:d (Alternando)
(iii) a:b :: c:d means (a+b) : b :: (c+d) : d (Componendo)
(iv) a:b :: c:d means (a - b) : b :: (c - d) :d (dividendo)
(v) If a:b :: c:d then (a + b) : (a – b) :: (c + d) : (c – d) (componendo and dividendo)
(vi) If a:b :: c:d then a: (a – b) :: c : (c – d) (convertendo)
Some Useful Result for Proportional Division
- The process of dividing a given quantity into parts which are proportional to a given ratio is called proportional division and the resultant quantities obtained are called proportional parts.
- For example: If 240 is to be divided in the ratio 3:4:5 60, 80 and 100 will be the proportional part since the ratio of these quantities is 3:4:5 and their sum is 240.
Method of Finding Proportional Part
If a quantity P is to be divided in the ratio of a:b:c and the resultant proportional parts are x, y and z then,
x = ka, y = kb and z = kc
Now, P = x + y +z
or ka + kb + kc = P
or
Proportional part
So, to calculate the proportional parts:
(a) Given quantity is divided by the sum of ratios.
(b) The quotient so obtained is multiplied by the respective numbers of the given ratio.
(c) The resultants will be the required proportional parts.
For example: Divide 2700 in the ratio 2:3:4
Sum of the ratio = 2 + 3 + 4 = 9
First part = = 300 x 2 = 600
Second part = = 300 x 3 = 900
Third part = = 300 x 4 = 1200
Example 1: If 38% of A = 52% of B then A : B = ?
Solution: It means
or 38 A = 52 B or . So A : B = 26:19
Example 2: The salaries of A, B and C are in the ratio 3:5:7. If their salaries are increased by 50%, 60% and 50% respectively, the ratio between new salaries is
Solution: After increase of 50% Salary of A is 3 X
After increase of 60% of salary of B is =
After increase of 50% salary of C is
Ratio between the new salaries of A, B and C is
or 45:80:105 or 9:16:21
Example 3: The speed of three Cars is in the ratio of 3:4:5. The ratio between the time taken by them to travel a fixed distance is
Solution: Let fixed distance be d
Time taken by first car is
Time taken by second car is
Time taken by 3rd car is =
So ratio of time between three cars is
or ratio is
or
20 : 15 : 12
Example 4: A mixture contains alcohol and water in the ratio 3:2. On adding 5 litre of water in it, the quantities of alcohol and water become equal. What is the quantity of alcohol in the mixture.
Solution: Let alcohol = 3x and water = 2x.
After adding 5 litres of water, 3x = 2x + 5.
Solve: x = 5, so alcohol = 3x = 15 litres.
Example 5:Two metals contain zinc and copper in the ratio 2:1 and 4:1 respectively. In what ratio these two must be mixed to get a new metal containing zinc and copper in the ratio 3 : 1
Solution: By the method of allegation and mixture
First metal: Zinc = 2/3, Copper = 1/3.
Second metal: Zinc = 4/5, Copper = 1/5.
Desired mixture: Zinc = 3/4, Copper = 1/4.
Using alligation for zinc:
- Difference between first metal (2/3) and desired (3/4): 3/4 - 2/3 = 9/12 - 8/12 = 1/12.
- Difference between second metal (4/5) and desired (3/4): 4/5 - 3/4 = 16/20 - 15/20 = 1/20.
- Ratio of first metal to second metal = (1/20) : (1/12) = 12 : 20 = 3 : 5.
Example 6: Mean proportional between 104 and 234 is
Solution: Mean proportional is
= 2 x 2 x 3 x 13 = 156
Example 7: There are three partners A, B and c in a firm. A’s capital is equal to thrice of B’s and B’s capital is 4 times C’s capital. Find the ratio of the capital of A, B and C
Solution: Given is A = 3B
and B = 4c
so A = 3B = 3 x 4c
So
So Ratio of A : B : C is 12 : 4 : 1
Example 8: In a mixture of 75 litres, the ratio of milk to water is 2:1. How much water should be added in the mixture so that the ratio of milk to water is 1 : 2
Solution: Ratio of milk to water is 2 : 1
In 75 litres milk is 50 and water is 25 i.e. 50:25
We want to make the ratio as 1 : 2 i.e 50 : 100.
Since the milk in two ratios is 50 each and we want to convert 25 water to 100 water. So, 75 litres of water should be added.
|
49 videos|179 docs|73 tests
|
FAQs on Ratio And Proportion - Quantitative Techniques for CLAT
| 1. What is the definition of a ratio? |  |
| 2. How do you calculate a proportion? |  |
| 3. What is the formula for calculating a ratio? |  |
| 4. What is the difference between ratio and proportion? |  |
| 5. How are ratio and proportion relevant to the CLAT exam? |  |
















