Speed, Time & Distance: Solved Examples - 1 | CSAT Preparation - UPSC PDF Download
Q1: A person travelled a distance of 50 km in 8 h. He covered a part of the distance on foot at the rate of 4 km/h and a part on a bicycle at the rate of 10 km/h. How much distance did he travel on foot?
(a) 10 km
(b) 20 km
(c) 30 km
(d) 40 km
Ans: (b)
Sol: Let he travels by on foot be x h.
Then, according to the question,
4x + 10 (8 − x) = 50 ⇒ 80 − 6x = 50
⇒ 6x = 30 ⇒ x = 5 h
∴ Distance travelled on the foot = 4 × 5 = 20 km
Q2: Two cities A and B 360 km apart. A car goes form A to B with a speed of 40 km/h and returns to A with a speed of 60 km/h. What is the average speed of the car?
(a) 45 km/h
(b) 48 km/h
(c) 50 km/h
(d) 55 km/h
Ans: (b)
Sol: Distance between the cities A and B = 360 km
∴ Average speed,
Q3: A man in car notices that he can count 25 telephone posts in 1 min. If they are known to be 40 m apart, then at what speed is the car travelling?
(a) 52.4 km/h
(b) 57.6 km/h
(c) 48.2 km/h
(d) 44.9 km/h
Ans: (b)
Sol: ∵ Number of gaps between 25 telephone posts = 24 and distance travelled in 1 min = 40 × 24 = 960 m
∴ Required speed 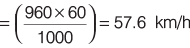
Q4: QA man walks 7.5 km at a speed of 3 km/h. At what speed would the man need to walk during the next 2 h to have an average of 4 km/h for the entire session?
(a) 3.65 km/h
(b) 4.75 km/h
(c) 5.25 km/h
(d) 6.50 km/h
Ans: (c)
Sol: Let speed of walking be x km/h.
Total time taken 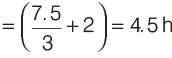
Total distance covered = (7. 5 + 2x) km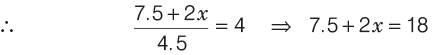
⇒ 2 x = 10.5 ⇒ x = 5.25
Hence, the speed of walking is 5.25 km/h.
Q5: Ram and Sita are walking towards each other with the speed of 8 km/h and 2 km/h respectively over a road 160 km long. How soon will they meet?
(a) 16 h
(b) 8 h
(c) 10 h
(d) 20 h
Ans: (a)
Sol: ∵ Distance = 160 km/h and relative speed = 8 + 2 = 10 km/h
Q6: If a person travels 39 km at a speed of 26 km/h, another 39 km at a speed of 39 km/h and 39 km again at a speed of 52 km/h. Then, find the average speed of entire journey.
(a) 35 km/h
(b) 35 km/h
(c) 36 km/h
(d) 40 km/h
Ans: (c)
Sol: Average speed of entire journey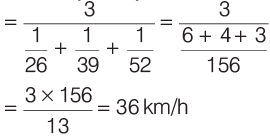
Q7: Total time taken by a person in going to a place by walking and returning on cycle is 5 h 45 min. He would have gained 2 h by cycling both ways. The time taken by him to walk both ways, is
(a) 6 h 45 min
(b) 7 h 45 min
(c) 8 h 15 min
(d) 8 h 30 min
Ans: (b)
Sol: Walking time + Cycling time = 5 h 45 min = 345 min ...(i)
If he had cycled both way he would have gained 2h (120 min).
Then, 2 × Cycling time = 345 − 120 = 225 min ...(ii)
⇒ Walking time = 2 × 345 − 225 = 690 − 225 = 465 min
∴ Time taken by him to walk both ways = 7 h 45 min
Q8: Amit starts from a point A and walks to another point B and then returns from B to A by his car and thus takes a total time of 6 h and 45 min. If he had driven both ways in his car, then he would have taken 2 h less. How long would it take for him to walk both ways?
(a) 7 h 45 min
(b) 8 h 15 min
(c) 8 h 30 min
(d) 8 h 45 min
Ans: (d)
Sol: Let w be the time taken in one way by walking and c be the time taken in one way by car.
Then, according to the question,
In first case, w + c = 6 h 45 min
⇒ 2 w + 2c = 13 h 30 min ...(i)
In second case, 2c = 4 h 45 min ...(ii)
From Eqs. (i) and (ii),
2 w + 2 c = 13 h 30 min
⇒ 2 w + 4h 45 min = 13 h 30 min
⇒ 2 w = 13 h 30 min − 4 h 45 min
⇒ 2 w = 8 h 45 min
Hence, if he walks both ways, then time taken is 8 h 45 min.
Q9: A student reaches his school 15 min before time by going there from his house at a speed of 5 km/h and reaches late by 9 min by going with a speed of 3 km/h. Accordingly distance between his house and school is
(a) 5 km
(b) 8 km
(c) 3 km
(d) 2 km
Ans: (c)
Sol: Let the distance between the house and the school be d.
Then, according to the question,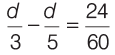
⇒ 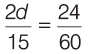
∴ d = 12/4 = 3
Hence, the distance between the house and the school is 3 km.
Q10: A car during its journey travels 30 min at a speed of 40 km/h, another 45 min at a speed of 60 km/h and 2 h at a speed of 70 km/h. Find its average speed.
(a) 58 km/h
(b) 63 km/h
(c) 67 km/h
(d) 71 km/h
Ans: (b)
Sol: Average speed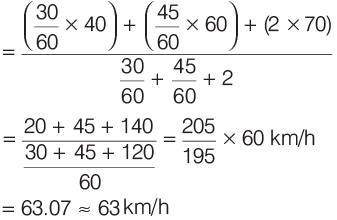
Q11: Mohan walking at a speed of 20 km/h reaches his office 1h late. Next time he increases his speed by 5 km/h, then he reaches his office 30 min early. What is the distance of his office from his house?
(a) 100 km
(b) 125 km
(c) 150 km
(d) None of these
Ans: (c)
Sol: Here, a = 20 km/h, t1 = 1 h, b = 20 + 5 = 25 km/h and t2 = 30 min = 0.5 h
Distance of his office from his house 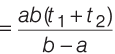
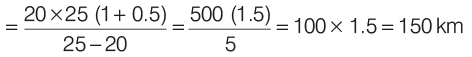
Q12: Starting from his house, one day a student walks at a speed of 3 km/h and reaches his school 5 min late. Next day he increases his speed by 1 km/h and reaches the school 3 min early. How far is the school from his house?
(a) 1.6 km
(b) 1.24 km
(c) 1.36 km
(d) 1.8 km
Ans: (a)
Sol: Let the distance be x km.
Then, the difference in timings 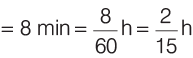
According to the question,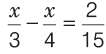
⇒ 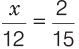
∴ 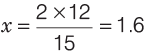
Hence, the required distance is 1.6 km.
Q13: Anurag travelled a distance of 45 km in 8 h 45 min. He travelled partly on foot at 3 km/h and partly by bicycle at 8 km/h. The distance travelled on the bicycle, is
(a) 25 km
(b) 15 km
(c) 30 km
(d) 20 km
Ans: (c)
Sol: Let the distance travelled on foot be x km.
Then, the distance covered by bicycle = (45 − x) km
According to the question,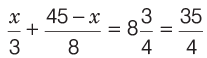
⇒ 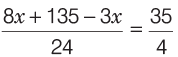
⇒ 5x + 135 = 210
⇒ 5x = 75 ⇒ x = 15
∴ Distance covered by bicycle = (45 − 15) = 30 km
Q14: A student goes to his school from his house walking at 4 km/h and reaches his school 10 min late. Next day, starting at the same time he walks as 6 km/h and reaches his school 5 min earlier than the scheduled time. Find the distance between school and home.
(a) 2 km
(b) 3 km
(c) 4 km
(d) 5 km
Ans: (b)
Sol: Let the distance be x km.
Then, time = Distance/Speed
1st day time taken = x/4 h
2nd day time taken = x/6 h
Difference in time = (x/4 - x/6) h
Actual difference between these two times = 15 min = 1/4 h
According to the question,
x/4 - x/6 = 1/4
⇒ 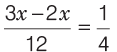
∴ x = 3
Hence, the required distance is 3 km.
Q15: Aryan runs at a speed of 40 m/min. Rahul follows him after an interval of 5 min and runs at a speed of 50 m/min. Rahul’s dog runs at a speed of 60 m/min and starts along with Rahul. The dog reaches Aryan and then comes back to Rahul and continues to do so till Rahul reaches Aryan. What is the total distance covered by the dog?
(a) 600 m
(b) 750 m
(c) 980 m
(d) 1200 m
Ans: (d)
Sol: Let they meet at a distance of y from start, after time t of Rahul start.
Then, according to the question,
40 × (5 + t ) = 50 t
⇒ 200 + 40 t = 50 t
⇒ t = 200/10 = 20 Min
∴ y = 50 × t = 50 × 20 = 1000 m
From the options, it is evident that all options except 1200 m are smaller than 1000 m and dog in any case has to move more than 1000 m.
|
205 videos|265 docs|136 tests
|
FAQs on Speed, Time & Distance: Solved Examples - 1 - CSAT Preparation - UPSC
| 1. What is the formula to calculate speed, time, and distance? |  |
| 2. How do you solve problems involving speed, time, and distance? |  |
| 3. What are some common units used in speed, time, and distance problems? |  |
| 4. How can I convert speed from km/h to m/s? |  |
| 5. What types of word problems can involve speed, time, and distance calculations? |  |





















