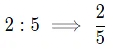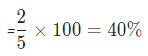Percentage Tips and Tricks for Government Exams
| Table of contents |

|
| What are Percentages? |

|
| How to Calculate a Percentage |

|
| Concept of Percentage Change |

|
| Increase/Decrease in Percentage |

|
| Solved Examples |

|
| Successive Percentage Increase/Decrease |

|
What are Percentages?
- The term percent comes from the Latin word Centum, which means for every 100 or per hundredth.
- A percentage shows how a number, ratio, fraction, or decimal relates to 100.
- To find the percentage of a number, you need to divide it by the total amount and then multiply the result by 100.
- The term proportion refers to a part of something in relation to one hundred.
How to Calculate a Percentage
1. Number: To represent a number ‘N’ into a percentage, just simply multiply the number with 100.
Example: Convert ‘4’ into a percentage.
Sol: Representation of 4 in Percentage will be
= 4 × 100 = 400%
2. Fraction: To represent a fraction into a percentage, just simply multiply the fraction with 100 and to convert the percentage into fraction just simply divide the percentage by 100.
Percentage = (Part / Whole) × 100
Example: Convert the fraction 3/5 into percentage.
Sol: Representation of 3/5 into Percentage will be
3/5 x 100 = 60%
3. Ratio: To convert the ratio into percentage, first convert the ratio into a fraction and then multiply the fraction with 100.
Example: Convert the ratio 2 : 5 into percentage.
Sol: Converting ratio into fraction we get
Now, the representation of 2/5 into percentage will be
4. Decimal: To represent a decimal into percentage, just simply multiply the decimal by 100 and to convert the percentage into a decimal just simply divide the percentage by 100.
Example: Convert 0.773 into percentage
Sol: Representation of 0.773 into percentage will be
=0.773 × 100=77.3%
Concept of Percentage Change
Whenever the value of measured quantity changes, the change can be captured through the following methods:
a. Absolute Value Change
It refers to the actual change in the measured quantity. For instance, if sales in year one is ₹5000 crore and the sales in year two is ₹6000 crore, then the absolute value of the change is ₹1000 croreb. Percentage Change
It is the percentage change obtained by the formulaPercentage change = (Absolute value change/ Original quantity) x 100
= 1000/5000 * 100 = 20%
Important Formulas
- Percentage Change of A from B = (A-B)/B x 100
- Percentage Change from A to B = (B-A)/A x 100
- How much more is A relative to B = (A-B)/B x 100
Example : The population of a city grew from 20 lac to 22 lac. Find the
(a) percentage change
(b) percentage change based on the final value of population
Sol: (a) percentage change = (Absolute Change/ Original Quantity) x 100
= (2/20) × 100 = 10%
(b) percentage change on the final value = (Absolute Change/ Original Quantity) x 100
= (2/22) x 100 = 9.09%
Increase/Decrease in Percentage
Percentage Increase
When comparing the increase in a quantity over a period of time, we first find the difference between the original value and the increased value. We then use this difference to find the relative increase against the original value and express it in terms of percentage. The formula for percentage increase is given by:
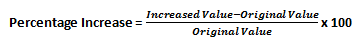
and
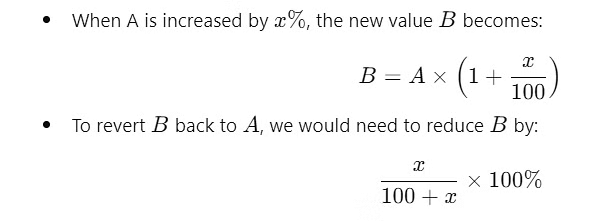
Percentage Decrease
When comparing the decrease in a quantity over a period of time, we first find the difference between the original value and the decreased value. We then use this difference to find the relative decrease against the original value and express it in the form of a percentage. The formula for percentage decrease is given by:
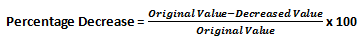
and
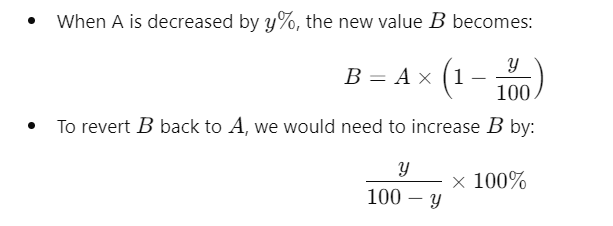
Solved Examples
Example 1: Reema has 25% more pens than Amit. How much percent fewer pens does Amit have as compared to Reema?
Sol:
Reema has 25% more pens than Amit, i.e., x = 25.
So, the percentage of pens Amit has as compared to Reema =
= (x / (100 + x)) × 100
= (25 / 125) × 100 = 20%
Thus, Amit has 20% fewer pens compared to Reema.
Example 2: The price of a notebook increased from ₹80 to ₹100. Find the percentage increase in price.
Sol:
Old price = ₹80
New price = ₹100
Increase = 100 - 80 = ₹20
Percentage increase = (20 / 80) × 100 = 25%
So, the price increased by 25%.
Example 3: A bag was ₹500 earlier and now costs ₹400. Determine the percentage decrease in price.
Sol:
Old price = ₹500
New price = ₹400
Decrease = 500 - 400 = ₹100
Percentage decrease = (100 / 500) × 100 = 20%
So, the price decreased by 20%.
Example 4: Mohan’s salary increased from ₹20,000 to ₹24,000. Find the percentage increase in salary.
Sol:
Original salary = ₹20,000
New salary = ₹24,000
Increase = ₹24,000 - ₹20,000 = ₹4,000
Percentage increase = (4000 / 20000) × 100 = 20%
So, salary increased by 20%.
Example 5: A mobile was sold for ₹15,000 last year and now it is sold for ₹12,000. Find the percentage decrease in price.
Sol:
Old price = ₹15,000
New price = ₹12,000
Decrease = ₹15,000 - ₹12,000 = ₹3,000
Percentage decrease = (3000 / 15000) × 100 = 20%
So, the price decreased by 20%.
Concept of Product Constancy
- Product constancy means the consistency of the product even though there is an increase or decrease in its price.
You can also call product constancy as inverse proportionality. - In product constancy, you have to reduce the consumption when there is an increase in price so that your budget remains the same.
- Product constancy involves the problems with two dependent quantities whose product is the third quantity. Thus, if there is an increase in the first quantity, then to make the third quantity constant, we have to increase/decrease the second quantity.
- Some relations from which questions on product constancy are asked are:
- Expenditure = Price * Consumption
- Price = Rate * Quantity
- Distance = Speed * Time
- Work done = Time taken * Efficiency
- Area of Rectangle = Length * Breadth
Successive Percentage Increase/Decrease
When two or more percentage changes are applied to a quantity consecutively, the percentage change is called a “successive percentage change.” Here, the final change is not the simple addition of two or more percentages. In a successive percentage change, a quantity is changed by some percentage, and the obtained new quantity is changed by another percentage, i.e., both the percentages are not applied to the same actual value.
1. Successive Increment Percentage Change
- When two or more increased percentage changes are applied to a quantity consecutively, the percentage change is called a “successive increment percentage change.”
- If the population of the town increased by a% and then by b%, we now have to apply the first percentage, i.e.
a%, to the initial value, then we have to apply the b%, to the resultant value obtained from the first percentage change. - Example: Let the population of the town be z. Suppose that there is a% increase in the population, then b% and then c% . What is the net percentage increase?
- Sol: Now, the first percentage increase = z + (z × a/100) = z (1 + a/100) = X
Second percentage increase = X + (X × b/100) = {z (1 + a/100) + [z × (1 + a/100) × (b/100)]}
= z (1 + a/100) (1 + b/100) = Y
Therefore, the net percentage change in the population of the town after two successive increments = {(Y – z)/z} × 100
If the value of an object x is successively increased by a%, b%, and then by c% then the final value is
x (1 + a/100) (1 + b/100) (1 + c/100)
2. Successive Decrement Percentage Change
- When two or more decreased percentage changes are applied to a quantity consecutively, the percentage change is called a “successive decrement percentage change.”
- If the price of a product is decreased by a% and then by b%, we now have to apply the first percentage, i.e., a%, to the initial value of the product. Then we have to apply the second percentage, i.e., b%, to the resultant value obtained from the first percentage change.
- Example: Let the price of the product be z. If there is decrease of a% initially then of b% and of c % afterwards, then calculate the final value?
- Sol: Now, the first percentage decrease = z – (z × a/100) = z (1 – a/100) = X
Second percentage decrease = X – (X × b/100) = {z (1 – a/100) – [z × (1 – a/100) × (b/100)]}
= z (1- a/100) (1 – b/100) = Y
Therefore, the net percentage change in the price of the product after two successive decrements = {(Y – z)/z} × 100
If the value of an object x is successively decreased by a%, b%, and then by c% the final value is
x (1 – a/100) (1 – b/100) (1 – c/100).
3. Other Successive Percentage Change
- Both percentage increment and percentage decrement can also be applied to the initial value of an object successively, and the percentage changes can also be used multiple times.
- If the value of an object x is successively changed by a%, b%, and then by c%, the final value is x (1 ± a/100) (1 ± b/100) (1 ± c/100), where the positive sign indicates an increment while the negative sign indicates a decrement.
4. Application of Percentage on Population Increase/Decrease:
- If there is an r% increase/decrease in population of a place in a year, then
- The Population of a place n years later will be
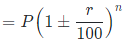
And, population of a place n years ago will be 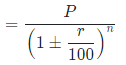
Solved Examples
Example 1: The price of a mobile increased by 10% in January and again by 20% in February. Find the total percentage increase in price.
Sol:
Let the original price = ₹100
After 10% increase:
= 100 × (1 + 10/100) = 100 × 1.10 = ₹110
Now, increase of 20% on ₹110:
= 110 × (1 + 20/100) = 110 × 1.20 = ₹132
Total increase = ₹132 - ₹100 = ₹32
Percentage increase = (32 / 100) × 100 = 32%
Example 2: The price of a fan was decreased by 10% and then again by 20%. Find the total percentage decrease in price.
Sol:
Let the original price = ₹100
After 10% decrease:
= 100 × (1 - 10/100) = 100 × 0.90 = ₹90
Now, 20% decrease on ₹90:
= 90 × (1 - 20/100) = 90 × 0.80 = ₹72
Total decrease = ₹100 - ₹72 = ₹28
Percentage decrease = (28 / 100) × 100 = 28%
Example 3: The value of an item is increased by 20% and then decreased by 10%. Find the net percentage change.
Sol:
Let the original value = ₹100
After 20% increase:
= 100 × 1.20 = ₹120
Now, 10% decrease on ₹120:
= 120 × 0.90 = ₹108
Final value = ₹108
Net change = ₹108 - ₹100 = ₹8 increase
Net percentage change = (8 / 100) × 100 = 8% increase
Example 4: The population of a town increases by 5% every year. If the current population is 20,000, what will be the population after 2 years?
Sol:
Population after 2 years =
= 20000 × (1 + 5/100) × (1 + 5/100)
= 20000 × 1.05 × 1.05 = ₹22050
So, population after 2 years = 22,050
Example 5: In an election, 80% of the voters voted. 60% of the votes cast were in favour of the winning candidate. If 24,000 people voted for the winner, how many registered voters were there?
Sol:
Let total voters = x
80% of x voted = 0.8x
60% of 0.8x = 0.48x = 24000
x = 24000 / 0.48 = 50,000 voters
|
66 videos|253 docs
|
FAQs on Percentage Tips and Tricks for Government Exams
| 1. What is percentage? |  |
| 2. How do you calculate percentage increase or decrease? |  |
| 3. How can I calculate a percentage of a number? |  |
| 4. What is the difference between percentage and percentile? |  |
| 5. How do you calculate the percentage of increase or decrease between two numbers? |  |