Trends in Kinetic Lability | Chemistry Optional Notes for UPSC PDF Download
| Table of contents |

|
| Introduction |

|
| Reaction Coordinate Diagrams |

|
| Self Exchange Reactions |

|
| Factors that Affect Rates of Substitution Reactions |

|
| Taube's Observations of Substitution Rates |

|
Introduction
Kinetics is a branch of chemistry that is concerned with the rates of chemical reactions. In this section, we will discuss the rates of metal-ligand (M-L) substitution reactions.
Let's start with some examples. In the table below are three examples of ligand substitution reactions of hexaaquo metal complexes to form hexaammine complexes. These reactions are nearly identical with the exception of the metal ion. The products are thermodynamically favored in all cases.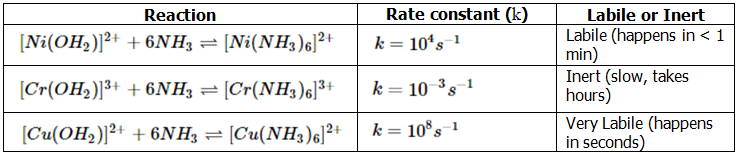
Definitions
- Labile: Metal complexes that undergo "kinetically fast" substitution reactions are labile. These reactions usually happen in less than one minute.
- Inert: Metal complexes that undergo "kinetically slow" substitution reactions are inert or non-labile. These reactions usually take hours.
- Intermediate: Metal complexes that undergo "kinetically intermediate" substitution reactions are intermediate.
A common pitfall is to confuse the meaning of kinetic terms, like labile and inert, with thermodynamic terms, like stable and unstable. It is important to distinguish between kinetics and thermodynamics. For example, the complex [Fe(H2O)Cl]2+ has a large formation constant and is thermodynamically stable; yet it is also labile. On the other hand, the complex [Co(NH3)6]3+ is unstable in acidic aqueous solution and decomposes spontaneously to [Co(H2O)6]3+; yet it decomposes slowly because it is inert. It is good practice to distinguish between meanings by using terms such as "kinetically labile" or "kinetically inert" and "thermodynamically stable" and "thermodynamically unstable".
Reaction Coordinate Diagrams
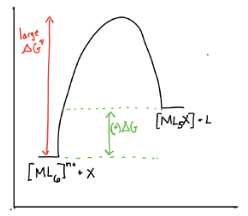
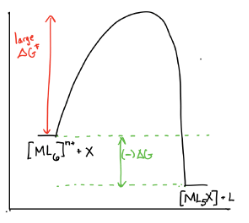
One way to visualize chemical reactions is through reaction coordinate diagrams (Figure 10.2.1). These diagrams illustrate the relative free energy of the reactants and products, when the free energy of the products is lower than the reactants ΔGrxn is negative and the reaction is spontaneous. This is what determines thermodynamic stability. These diagrams also illustrate the changes in free energy as the reaction progresses from reactants to products. In the case of a single step, concerted reaction the reactants go through a higher energy transition state before (potentially) forming products. The free energy difference between the reactants and the transition state is the free energy of activation (ΔG‡), which determines the rate of the reaction. A larger ΔG‡ yields a slower reaction rate because fewer reactants have sufficient energy to form products. In cases where the reaction occurs in 2 steps an intermediate is formed. The step with the largest ΔG‡ will be the slowest, rate determining step. The magnitude of ΔG‡ is what determines kinetic lability.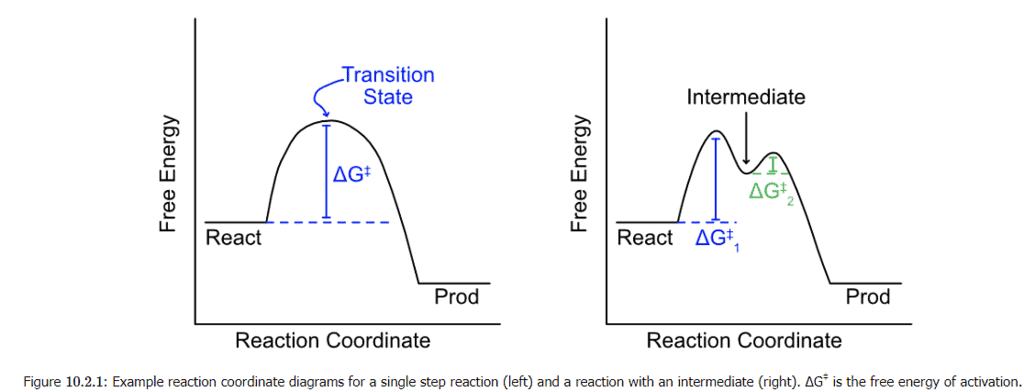
Solved Example
Example: Draw the reaction coordinate diagrams for a reaction of the form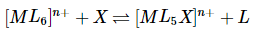 in the following scenarios:
in the following scenarios:
- [ML6]n+ is thermodynamically stable and kinetically inert.
- [ML6]n+ is thermodynamically unstable and kinetically inert.
- [ML6]n+ is thermodynamically stable and kinetically labile.
- [ML6]n+ is thermodynamically unstable and kinetically labile.
Ans:
Self Exchange Reactions

The rate of a chemical reaction depends on both thermodynamic and kinetic factors. One way to isolate the kinetic factors is to study self exchange reactions. In a self exchange reaction the incoming and outgoing ligand are the same and the ΔGrxn of the reaction is 0. In practice this is often done by dissolving hexaaqua metal complexes in isotopically labeled water and monitoring the ligand exchange reaction by NMR. These experiments have found reaction rates for different metals that span more than 12 orders of magnitude as shown in Figure 10.2.2.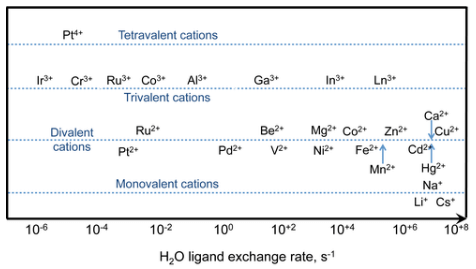 Figure 10.2.2: Water exchange rates measured by NMR for a series of metal ions.
Figure 10.2.2: Water exchange rates measured by NMR for a series of metal ions.
Factors that Affect Rates of Substitution Reactions
- Some of the factors that affect rates of ligand substitution reactions are those that also affect thermodynamic stability. It is important to remember that kinetic and thermodynamic factors are related, yet separate. The same factors that make a complex stable can also make it more inert. However, it is incorrect to assume that stability is always correlated with reaction rates.
- Why are kinetic factors related to thermodynamic stability? For a complex to react or exchange one ligand for another, it must change its geometry to form an intermediate or transition state. For example, inert octahedral complexes often have stable electron configurations. When the reactant electron configuration is particularly stable, it can result in a higher activation energy associated with moving away from the stable configuration.
Factors to consider
- Electron configuration and LFSE: Electron configurations that place electrons in higher-energy orbitals (particularly antibonding orbitals) result in more labile complexes. As long as there are not electrons in higher-energy orbitals, the lability correlates roughly with LFSE. The more negative the LFSE, the more inert.
- Size and charge of the metal ion: In general, higher charge density on the metal ion leads to stronger electrostatic attraction between metal and ligand; higher charge density generally correlates with slower rates of ligand substitution.
Taube's Observations of Substitution Rates
- Henry Taube (Nobel Prize, 1983) tried to understand lability by comparing the factors that govern bond strengths in ionic complexes to observations about the rates of reaction of coordination complexes. He saw some things that were unsurprising. He also drew some new conclusions based on ligand field theory. Taube observed that many M+1 ions are more labile than many M+3 ions, in general. That isn't too surprising, since metal ions function as electrophiles or Lewis acids and ligands function as nucleophiles or Lewis bases in forming coordination complexes. In other words, metals with higher charges ought to be stronger Lewis acids, and so they should bind ligands more tightly. However, there were exceptions to that general rule. For example, Taube also observed that Mo+5 compounds are more labile than Mo+3 compounds. So, there must be more going on here than just the effects of electrostatic attraction.
- Another factor that governs ionic bond strengths is the size of the ion. Typically, ions with smaller atomic radii form stronger bonds than ions with larger radii. Taube observed that Al3+, V3+, Fe3+ and Ga3+ ions are all about the same size. All these ions exchange ligands at about the same rate. That isn't surprising, because they have the same charge and the same radius. However, Cr3+ is also about the same size as those ions and it also has the same charge, but it is much less labile. Once again, there are exceptions to our regular expectations based on simple electrostatic considerations. Furthermore, 4d and 5d transition metals (Y→Cd, and Ac→Hg) are much more inert than 3d transition metals (Sc→Zn). This is unexpected when we consider size; the 4d and 5d metals are much larger than the 3d metals. This unexpected behavior tells us that electrostatics alone cannot predict lability.

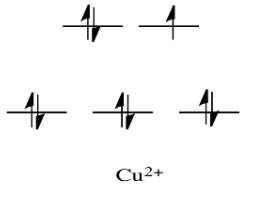
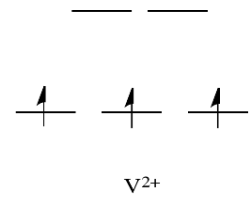
- Taube came up with a hypothesis that could explain the seeming contradictory observations described above: kinetic lability must be affected by d-electron configuration. This idea forms the basis of Taube's rules about lability. For example, metals like Ni2+ and Cu2+ are very labile. The d orbital splitting diagrams for those compounds would have d electrons in the eg set. Remember, the eg set arises from interaction with the ligand donor orbitals; this set corresponds to a σ antibonding level. By comparison, V2+ is rather inert. The d orbital splitting diagram in this case has electrons in the t2g set, but none in the eg set. So, having electrons in the higher energy, antibonding eg level weakens the bond to the ligand, so the ligand can be replaced more easily. In the absence of those higher energy electrons, the bond to the ligand is stronger, and the ligand isn't replaced as easily.

- On the other hand, metals like Ca2+, Sc3+ and Ti4+ are pretty labile. The d orbital splitting diagrams in those cases are pretty simple: there are no d-electrons at all in these ions. That means having no electrons in these mostly non-bonding levels leaves the complex susceptible to ligand replacement. But it's hard to see why population of an orbital that is mostly non-bonding would have an effect on ligand bond strength. Instead, this factor probably has something to do with the part of ligand substitution that we have ignored so far. Not only does one ligand need to leave, but a second one needs to bond in its place. So, having an empty orbital for the ligand to donate electrons into (or, put another way, not having electrons in the way that may complicate donation from the ligand) makes that part of the reaction easier.
- Electron configurations can be generally classified as inert or labile based on crystal field stabilization energy. Configurations with high CFSE will be inert and configurations with low CFSE will be labile. Configurations that are most susceptible to Jahn-Teller distortions will be exceptionally labile, as the elongated bonds will be weaker and those ligands more easily exchanged.
Solved Example
Example: In which compound from each pair would you expect the strongest ionic bonds? Why?
(a) LiF vs KBr
(b) CaCl2 vs. KCl
Ans: (a) The ions in LiF are both smaller than in KBr, so the force of attraction between the ions in LiF is greater because of the smaller separation between the charges.
(b) Calcium has a 2+ charge in CaCl2, whereas potassium has only a + charge, so the chloride ions are more strongly attracted to the calcium than to the potassium.
Labile and inert electron configurations
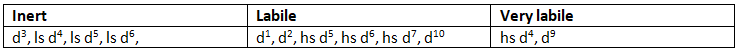
Solved Examples
Example 1: Put the metal ions in order of decreasing reaction rate (from labile to inert):
(a) Al3+, Na+, Mg2+
(b) Ca2+, Mg2+, Sr2+
Ans: (a) Most labile to most inert: Na+ > Mg2+ > Al3+
These are metal ions with similar size and varying charge. They are in order of increasing charge and increasing density from left to right.
(b) Most labile to most inert: Sr2+ > Ca2+ > Mg2+
These metal ions have the same charge, and vary in size. They are in order of decreasing charge and increasing charge density from left to right.
Example 2: Predict whether octahedral complexes of the following metals are labile or inert
(a) Co3+ (high spin)
(b) Co3+ (low spin)
(c) Fe2+ (low spin)
(d) Fe2+ (high spin)
(e) Zn2+
Ans: (a) hs d6 labile (electrons in higher energy d orbital set)
(b) ls d6 inert (all electrons in lower energy d orbitals)
(c) ls d6 inert (all electrons in lower energy d orbitals)
(d) hs d5 labile (electrons in higher energy d orbital set, CFSE = 0)
(e) d10 labile (electrons in higher energy d orbital set, CFSE = 0)
Overall Generalizations for Kinetics
- s-block metals are very labile, except for those with very high charge density (eg. Mg2+ is inert)
- d10 metals are labile (eg: Zn2+, Cu+, Hg2+)
- Other ions with a full shell are labile (eg: Ln3+ of f-block)
- 3d M2+, when high spin, are generally labile (eg. Cu2+ is very labile)
- 4d and 5d are usually inert due to higher CFSE (low spin, high CFSE)
- M2+ is more labile than the same metal as M3+
- d3 and low spin d6 are inert (eg. Cr3+, Co3+, low spin Fe2+)
FAQs on Trends in Kinetic Lability - Chemistry Optional Notes for UPSC
| 1. What are reaction coordinate diagrams? |  |
| 2. What are self exchange reactions? |  |
| 3. What factors affect the rates of substitution reactions? |  |
| 4. What were Taube's observations of substitution rates? |  |
| 5. What are the trends in kinetic lability observed in substitution reactions? |  |

|
Explore Courses for UPSC exam
|

|