NEET Previous Year Questions (2014-2024): Current Electricity | Physics Class 12 PDF Download
2024
Q1: The terminal voltage of the battery, whose emf is 10V and internal resistance 1Ω, when connected through an external resistance of 4Ω as shown in the figure is: [2024]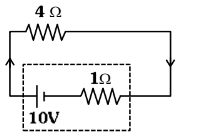 (a) 4 V
(a) 4 V
(b) 6 V
(c) 8 V
(d) 10 V
Ans: (c)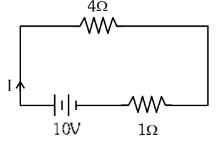 Current in circuit
Current in circuit 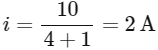
Terminal voltage = E - iR
= 10 - 2 x 1 = 8 V
Q2: A wire of length 'l' and resistance 100Ω is divided into 10 equal parts. The first 5 parts are connected in series while the next 5 parts are connected in parallel. The two combinations are again connected in series. The resistance of this final combination is: [2024]
(a) 26Ω
(b) 52Ω
(c) 55Ω
(d) 60Ω
Ans: (b)
To solve this problem, we first need to understand how the resistance changes when we cut the wire into equal parts and how it behaves when connected in different configurations (series and parallel).
Given:
Original length of the wire, l.
Total resistance of the wire R = 100Ω.
The wire is divided into 10 equal parts.
Resistance of each part:
Since the wire is divided into 10 equal parts, the length of each part is l/10. Resistance is proportional to length (as long as the cross-sectional area and material of the wire remain constant). Therefore, the resistance of each part, denoted as r, is 1/10th of the total resistance:
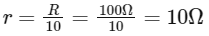
First 5 parts in series:
When resistors are connected in series, the total resistance is the sum of the individual resistances:
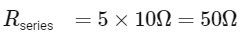
Next 5 parts in parallel:
When resistors are connected in parallel, the total resistance parallel Rparallel can be calculated using the reciprocal formula: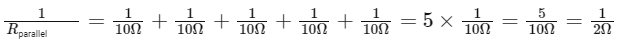
Thus,
Rparallel = 2Ω
Final combination in series:
The total resistance of the combination, where the series and parallel groups are again connected in series, will be: Thus, the correct answer to the resistance of the final combination is:
Thus, the correct answer to the resistance of the final combination is:
Option B: 52Ω
Q3: Choose the correct circuit which can achieve the bridge balance.
(a) 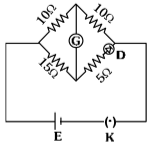
(b) 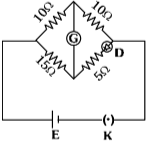
(c) 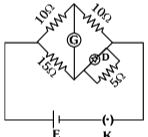
(d) 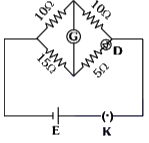 [2024]
[2024]
Ans: (a)
In option (a),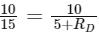
The diode can conduct and have resistance RD = 10Ω because diode have dynamic resistance. In that case bridge will be balanced.
Q4: Two heaters A and B have power rating of 1 kW and 2 kW, respectively. Those two are first connected in series and then in parallel to a fixed power source. The ratio of power outputs for these two cases is:
(a) 1 : 1
(b) 2 : 9
(c) 1 : 2
(d) 2 : 3 [2024]
Ans: (b)
To find the ratio of power outputs when two heaters with different power ratings are connected first in series and then in parallel, we need to understand how the total power output varies based on the type of connection.
Heater Specifications:
- Power of heater A
kWW(PA) - Power of heater B
kWW(PB)
Scenario 1: Series Connection
When resistors (or heaters in this case) are connected in series, the total resistance (Rseries
Using the formula for electrical power: P = V2 / R,
where P is power, V is voltage, and R is resistance, we can express the resistance of each heater as:
Substitute the given power values: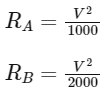
Then the total resistance for the series connection is: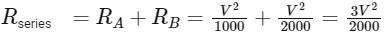
The total power output in series (Pseries) is:
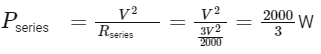
Scenario 2: Parallel Connection
For parallel connections, the total resistance (Rparallel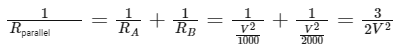
Reformulate to find Rparallel
And the total power output in parallel (P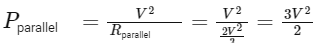
However, simplifying,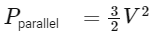
The ratio of powers is then:
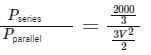
Solving and simplifying,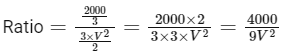
Given that we know one ratio of the actual power values, we simplify further. For calculating power in simple terms, consider voltage to be normalized (taken out of the fraction):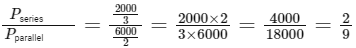
Therefore, the ratio of the power outputs when the heaters are connected first in series and then in parallel is 2:9, which corresponds to Option B.
2023
Q1: If the galvanometer G does not show any deflection in the circuit shown, the value of R is given by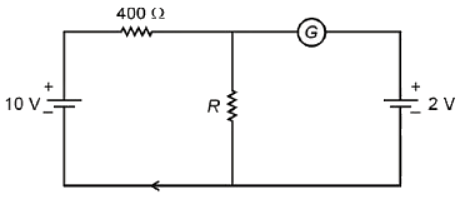 (a) 200 Ω
(a) 200 Ω(b) 50 Ω
(c) 100 Ω
(d) 400 Ω
Ans: (c)
Since the galvanometer does not show any deflection, the potential of each point can be found easily as shown in the image below:
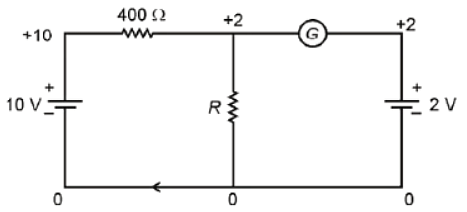 i400=(10-2)/400 =8/400= 1/50 A
i400=(10-2)/400 =8/400= 1/50 AThe current through R will also be the same as no current is flowing through the other side.
iR=1/50 A
R=VR/IR = (2-0)/(1/50)=100Ω
Q2: Resistance of a carbon resistor determined from color codes is (22000 ± 5%) Ω. The colour of the third band must be
(a) Red
(b) Green
(c) Orange
(d) Yellow
Ans: (c)
Resistance = (22 × 103) Ω ± 5%
The third band corresponds to the decimal multiplier.
Decimal multiplier = 103
⇒ Colour → Orange
Q3: The magnitude and direction of the current in the following circuit is
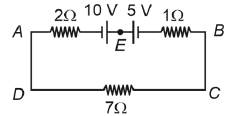
(a) 0.2 A from B to A through E
(b) 0.5 A from A to B through E
(c) 5/9 A from A to B through E
(d) 1.5 A from B to A through E
Ans: (b)
In the given image, the net current will flow from A to B because 10V > 5V.
Applying KVL from A to B to C to D we get,
-2i+10-5-1i-7i=0
5-10i=0
i=1/2 A
i=0.5 A
In clockwise direction (from A to B)
Q4: 10 resistors, each of resistance R are connected in series to a battery of emf E and negligible internal resistance. Then those are connected in parallel to the same battery, the current is increased n times. The value of n is
(a) 10
(b) 100
(c) 1
(d) 1000
Ans: (b)
In series combination
Req = 10R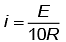
In parallel combination
Q5: The resistance of platinum wire at 0°C is 2 Ω and 6.8 Ω at 80°C. The temperature coefficient of resistance of the wire is
(a) 3 × 10–4 °C–1
(b) 3 × 10–3 °C–1
(c) 3 × 10–2 °C–1
(d) 3 × 10–1 °C–1
Ans: (c)
Using R = R0(1 + αΔT)
where a is the thermal coefficient of resistance
6.8 = 2{1 + α(80 - 0)}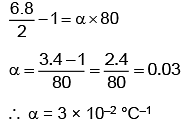
Q6: The equivalent capacitance of the arrangement shown in the figure is: 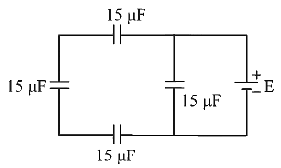
(a) 30μF
(b) 15μF
(c) 25μF
(d) 20μF
Ans: (d)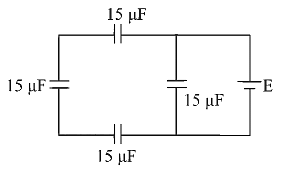
In the diagram below, the capacitors, B, C, and D are receiving the same amount of current, so they are in series as the current flow is the same. The equivalent capacitance of three of these in series is: 1/[(1/15)+(1/15)+(1/15)]= 5 μF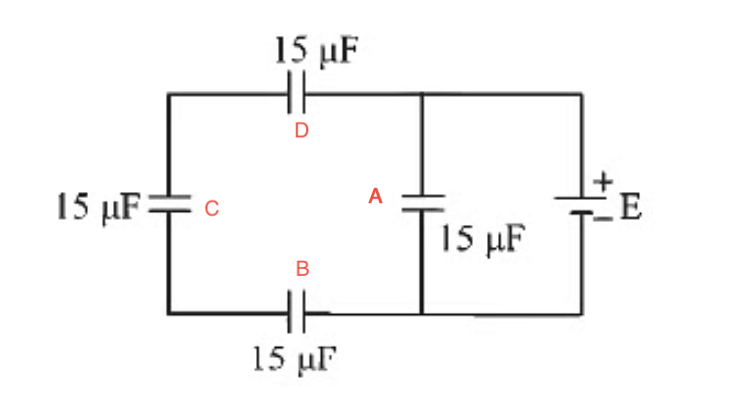
Therefore,
Ceq = 5 + 15 = 20μF
Q7: A certain wire A has a resistance of 81 Ω. The resistance of another wire B of the same material and equal length but of diameter thrice the diameter of A will be :
(a) 81 Ω
(b) 9 Ω
(c) 729 Ω
(d) 243 Ω
Ans: (b)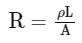
If the diameter becomes thrice then the cross-section area will become 9 times so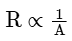
Resistance will become 1/9 times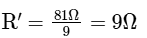
Q8: To produce an instantaneous displacement current of 2 mA in the space between the parallel plates of a capacitor of capacitance 4μF, the rate of change of applied variable potential difference (dv/dt) must be
(a) 800 V/s
(b) 500 V/s
(c) 200 V/s
(d) 400 V/s
Ans: (b)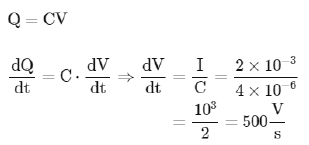
2022
Q1: The reciprocal of resistance is :
(a) conductance
(b) reactance
(c) mobility
(d) conductivity
Ans: (a)
R = 1/G
Thus reciprocal of resistance (R) is conductance (G)
Q2: The distance between the two plates of a parallel plate capacitor is doubled and the area of each plate is halved. If C is its initial capacitance, its final capacitance is equal to
(a) C/4
(b) 2C
(c) C/2
(d) 4C
Ans: (a)

Q3: A cell of emf 4 V and internal resistance 0.5 Ω is connected to a 7.5 Ω external resistance. The terminal potential difference of the cell is
(a) 0.375 V
(b) 3.75 V
(c) 4.25 V
(d) 4V
Ans: (b)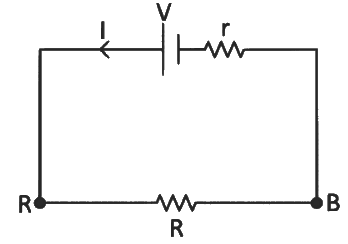 From Kirchhoff's loop law :
From Kirchhoff's loop law :
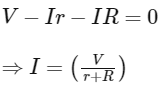
Terminal potential difference across cell,
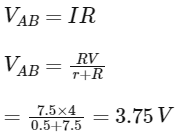
Q4: The equivalent resistance of the infinite network given below is :
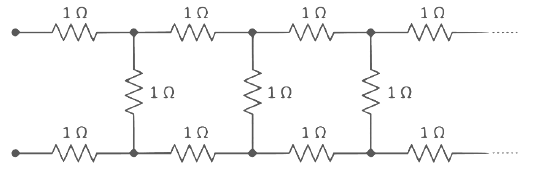
(a) 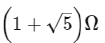
(b) 2 Ω
(c) 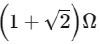
(d) 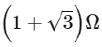
Ans: (d)
Let net resistance of the given infinite network be 'R'
Now, the circuit can be modified as
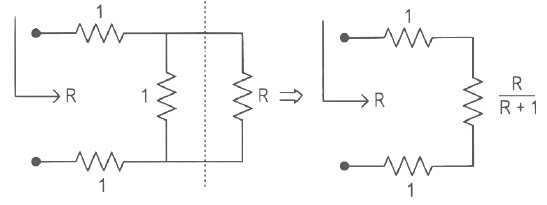
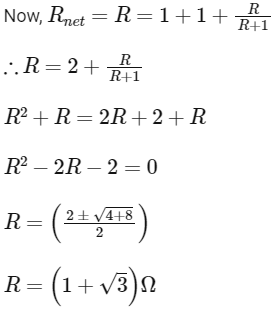
Q5: The effective capacitances of two capacitors are 3 μF and 16 μF, when they are connected in series and parallel respectively. The capacitance of two capacitors are :
(a) 1.2 μF, 1.8 μF
(b) 10μF, 6 μF
(c) 8 μF, 8 μF
(d) 12 μF, 4 μF
Ans: (d)
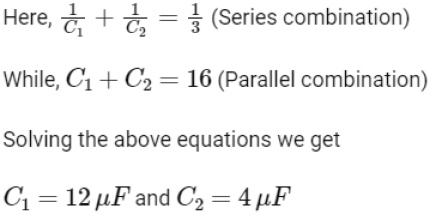
Q6: The sliding contact C is at one fourth of the length of the potentiometer wire (AB) from A as shown in the circuit diagram. If the resistance of the wire AB is R0, then the potential drop (V) across the resistor R is
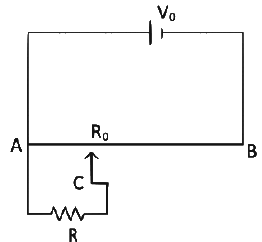 (a)
(a) 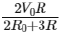
(b) 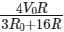
(c) 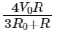
(d) 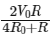
Ans: (b)
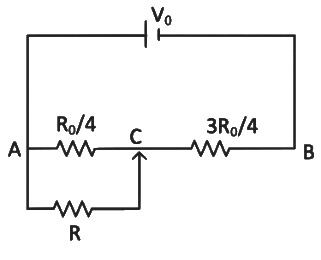
Equivalent resistance across point AC
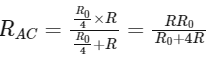
From voltage divider rule
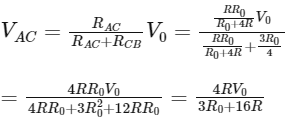
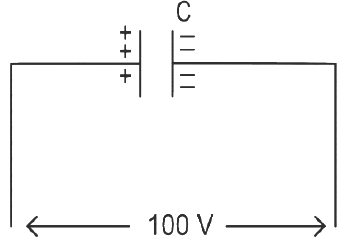
Q7: A capacitor of capacitance C = 900 pF is charged fully by 100 V battery B as shown in figure (a). Then it is disconnected from the battery and connected to another uncharged capacitor of capacitance C = 900 pF as shown in figure (b). The electrostatic energy stored by the system (b) is
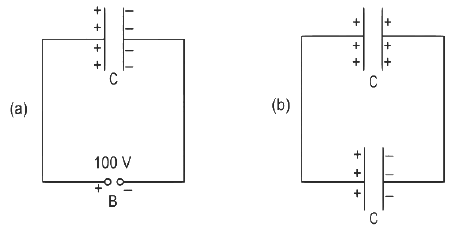
(a) 4.5 x 10-6 J
(b) 3.25 x 10-6 J
(c) 2.25 x 10-6 J
(d) 1.5 x 10-6 J
Ans: (c)
q1 = CV
= 900 x 10-12 x 100
= 9 x 10-8 J
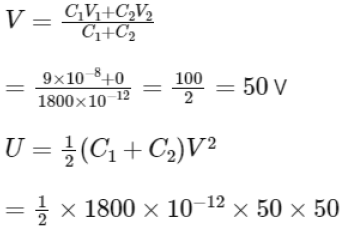
= 225 x 10-8
U = 2.25 x 10-6 J
Q10: A copper wire of length 10 m and radius (10−2/√π) m has an electrical resistance of 10 Ω. The current density in the wire for an electric field strength of 10 (V/m) is:
(a) 106 A/m2
(b) 10–5 A/m2
(c) 105 A/m2
(d) 104 A/m2
Ans: (c)
Q11: A Wheatstone bridge is used to determine the value of unknown resistance X by adjusting the variable resistance Y as shown in the figure. For the most precise measurement of X, the resistances P, and Q 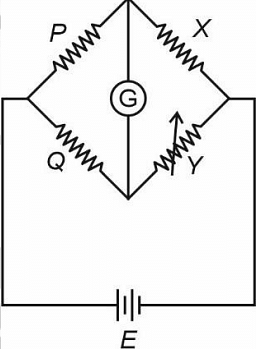 (a) Should be approximately equal and are small
(a) Should be approximately equal and are small
(b) Should be very large and unequal
(c) Do not play any significant role
(d) Should be approximately equal to 2X
Ans: (a)
We know, a Wheatstone bridge is said to be most precise when it is most sensitive. This can be done by making the ratio of arms equal. Thus (1) is the correct option.
Q12: Two resistors of resistance, 100 Ω and 200 Ω are connected in parallel in an electrical circuit. The ratio of the thermal energy developed in 100 Ω to that in 200 Ω in a given time is
(a) 2 : 1
(b) 1 : 4
(c) 4 : 1
(d) 1 : 2
Ans: (a)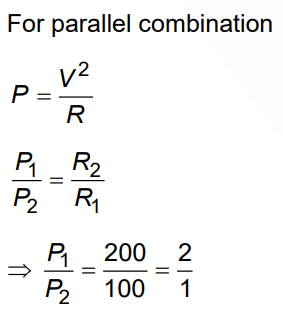
Q13: As the temperature increases, the electrical resistance
(a) Decreases for both conductors and semiconductors
(b) Increases for conductors but decreases for semiconductors
(c) Decreases for conductors but increases for semiconductors
(d) Increases for both conductors and semiconductors
Ans: (b)
As the temperature increases, the resistivity of the conductor increases hence the electrical resistance increases. However, for semiconductors, the resistivity decreases with the temperature. Hence the electrical resistance of semiconductors decreases.
2021
Q1: Column I gives certain physical terms associated with the flow of current through a metallic conductor.
Column II gives some mathematical relations involving electrical quantities.
Match Column-I and Column-II with appropriate relations. (2021)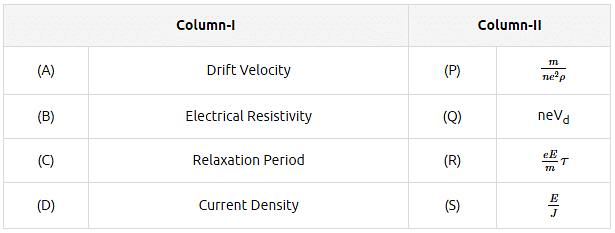
(a) (A)-(R), (B)-(P), (C)-(S), (D)-(Q)
(b) (A)-(R), (B)-(Q), (C)-(S), (D)-(P)
(c) (A)-(R), (B)-(S), (C)-(P), (D)-(Q)
(d) (A)-(R), (B)-(S), (C)-(Q), (D)-(P)
Ans: (c)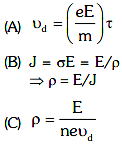
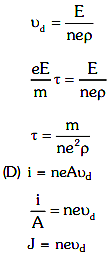
Q2: In a potentiometer circuit, a cell of EMF 1.5 V gives a balance point at 36 cm length of wire. If another cell of EMF 2.5 V replaces the first cell, then at what length of the wire, does the balance point occur?
(a) 64 cm
(b) 62 cm
(c) 60 cm
(d) 21.6 cm
Ans: (c)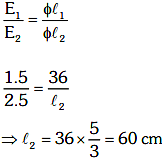
Q3: The effective resistance of a parallel connection that consists of four wires of equal length, equal area of cross-section, and the same material is 0.25Ω. What will be the effective resistance if they are connected in series?
(a) 1Ω
(b) 4Ω
(c) 0.25Ω
(d) 0.5Ω
Ans: (b)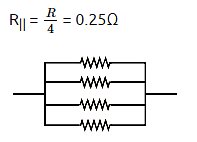
R = 1Ω
Rseries = 4R
= 4(1)
= 4Ω.
Q4: Three resistors having resistances r1, r2, and r3 are connected as shown in the given circuit. The ratio i3/i1of currents in terms of resistances used in the circuit are: 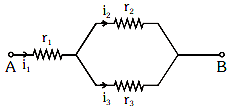
(a) 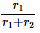
(b) 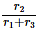
(c) 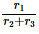
(d) 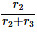
Ans: (d)
v = ir
i = v/r
i ∝ i/r
[ v is same for r2 & r3]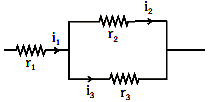
2020
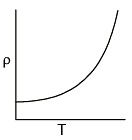
Q1: Which of the following graphs represents the variation of resistivity (r) with temperature (T) For copper? (a)
(b)
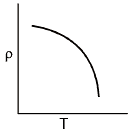
(c)
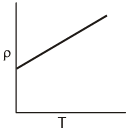
(d)
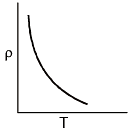
Ans: (a)
The relation between resistivity and temperature is given by ρ = ρ0e-αT
Q2: A charged particle having drift velocity of 7.5×10-4 m s-1 in an electric field of 3×10-10 Vm-1 has a mobility in m2 V-1 s-1 of :
(a) 2.5×10-6
(b) 2.25×10-15
(c) 2.25×1015
(d) 2.5×106
Ans: (d)
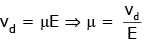
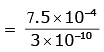
μ = 2.5 × 106
(μ = mobility)
Q3: The color code of resistance is given below:
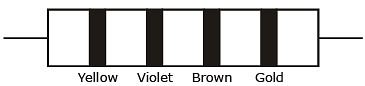
The values of resistance and tolerance, respectively, are :
(a) 4.7 kΩ, 5%
(b) 470Ω, 5%
(c) 470 kΩ, 5%
(d) 47 kΩ, 10%
Ans: (b)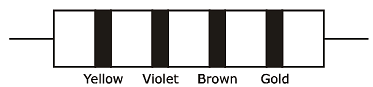
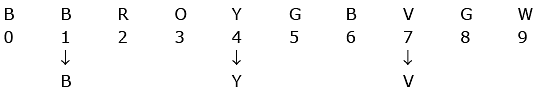 R = 47 × 101Ω ± 5%
R = 47 × 101Ω ± 5%
= 470 Ω ± 5%
So, 470, 5%
Q4: The solids which have the negative temperature coefficient of resistance are :
(a) semiconductors only
(b) insulators and semiconductors
(c) metals
(d) insulators only
Ans: (b)
Insulators have a negative temperature coefficient because as temperature increases, the resistance of insulators decreases. The resistivity of the semiconductor material decreases with the rise in temperature, resulting in a negative temperature coefficient of resistance.
Q5: Two solid conductors are made up of same material, have same length and same resistance. One of them has a circular cross-section of area A1 and the other one has a square cross-section of area A2 .
The ratio A1 / A2
(a) 1.5
(b) 1
(c) 0.8
(d) 2
Ans: (b)
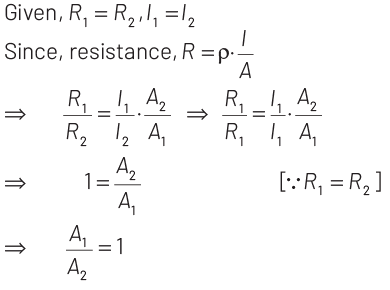
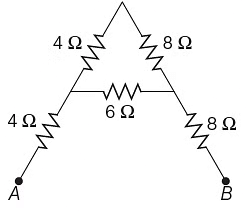
Q6: The equivalent resistance between A and B for the mesh shown in the figure is
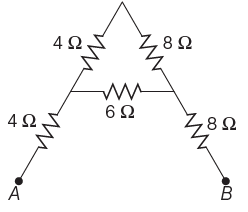 (a) 7.2 Ω
(a) 7.2 Ω
(b) 16 Ω
(c) 30 Ω
(d) 4.8 Ω
Ans: (b)
Equivalent resistance between points A and B is given as
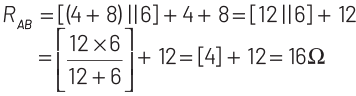
Q7: For the circuit shown in the figure, the current I will be
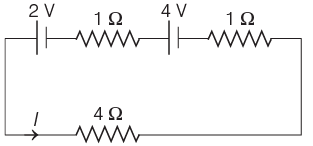 (a) 0.75 A
(a) 0.75 A
(b) 1 A
(c) 1.5 A
(d) 0.5 A
Ans: (b)
The circuit diagram is shown below
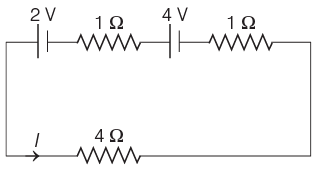
Applying KVL in the loop, we get
4I + I⋅1 −4+ I⋅1 −2 =0
⇒ 6I = 6
⇒ I = 1 A
Q8: For the circuit given below, the Kirchhoff’s loop rule for the loop BCDEB is given by the equation
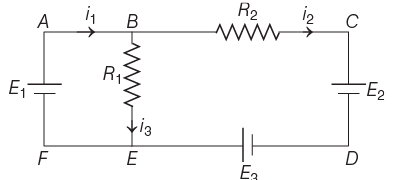
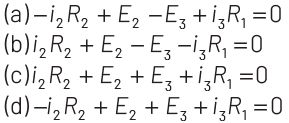
Ans: (b)
The circuit diagram is given below
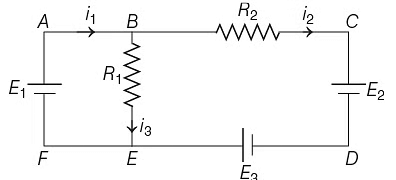
Applying KVL rule in loop BCDEB,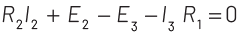
Q9: A resistance wire connected in the left gap of a metre bridge balances a 10 Ω resistance in the right gap at a point which divides the bridge wire in the ratio 3 : 2. If the length of the resistance wire is 1.5 m, then the length of 1 Ω of the resistance wire is
(a) 1.0 × 10−1 m
(b) 1.5 × 10−1 m
(c) 1.5 × 10−2 m
(d) 1.0 × 10−2 m
Ans: (a)
According to the question, the metre bridge is shown below,
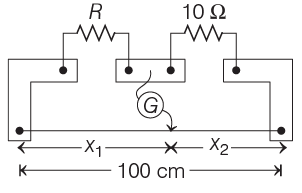
Given, 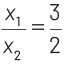
At balance condition in metre bridge,
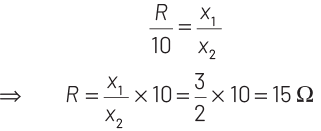
Now, length of given wire whose resistance 15 Ω is 1.5 m.
Therefore, length of 1Ω resistance wire is
= 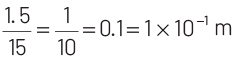
Hence, correct option is (a).
2019
Q1: Six similar bulbs are connected as shown in the figure with a DC source of emf E and zero internal resistance.
The ratio of power consumption by the bulbs when (i) all are glowing and (ii) in the situation when two from section A and one from section B are glowing, will be : 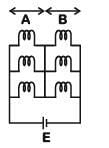
(a) 4 : 9
(b) 9 : 4
(c) 1 : 2
(d) 2 : 1
Ans: (b)
Solution:
(i) All bulbs are glowing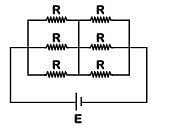
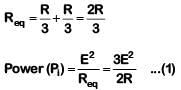
(ii) Two from section A and one from section B are glowing.

Q2: In the circuits shown below, the readings of the voltmeters and the ammeters will be 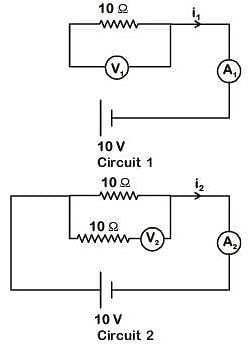
(a) V2 > V1 and i1 = i2
(b) V1 = V2 and i1 > i2
(c) V1 = V2 and i1 = i2
(d) V2 > V1 and i1 > i2
Ans: (c)
Solution:
For ideal voltmeter, resistance is infinite and for the ideal ammeter, resistance is zero.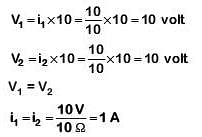
Q3: The meter bridge shown in the balance position with P/Q = l1 / l2. If we now interchange the positions of galvanometer and cell, will the bridge work? If yes, that will be balanced condition?
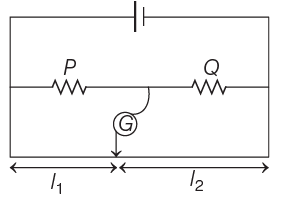
(a) 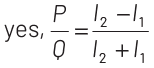
(b) no, no null point
(c) 
(d) 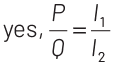
Ans: (d)
For balanced position in a meterbridge
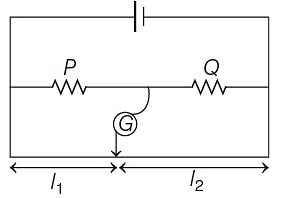
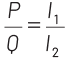
Now, if position of G and cell is interchanged,
The balance condition still remains the same if the jockey points as the same point as given in the initial condition, for which there is no deflection in the galvanometer or no current will be drawn from the cell. Thus, the bridge will work as usual and balance condition is same,
P / Q = l1 /l2
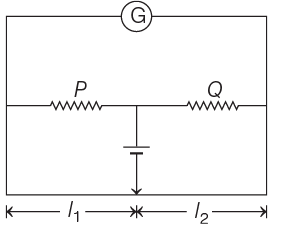
Q4: The reading of an ideal voltmeter in the circuit shown is
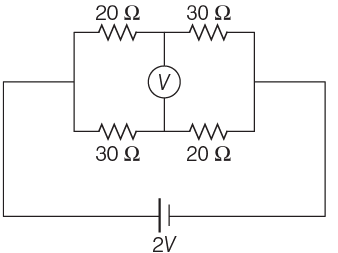
(a) 0.6 V
(b) 0 V
(c) 0.5 V
(d) 0.4 V
Ans: (d)
The given circuit diagram can be drawn as shown below
The equivalent resistance of circuit is given by
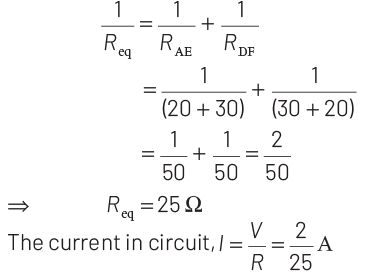
As the resistance of two branches is same i.e. 50 Ω.
So, the current I1 = I2
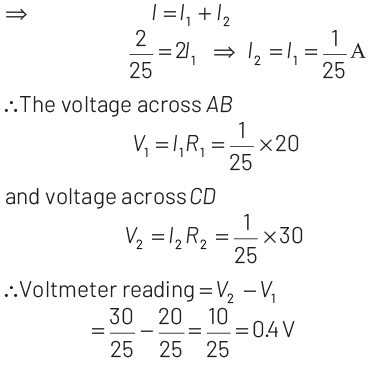
2018
Q1: A set of 'n' equal resistors, of value 'R' each, are connected in series to a battery of emf 'E' and internal resistance 'R'. The current drawn is I. Now, the 'n' resistors are connected in parallel to the same battery. Then the current drawn from the battery becomes 10 I. The value of 'n' is:
(a) 10
(b) 11
(c) 20
(d) 9
Ans: (a)
Solution: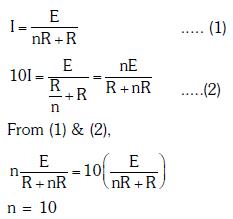
Q2: A battery consists of a variable number 'n' of identical cells (having internal resistance 'r' each) which are connected in series. The terminals of the battery are short-circuited and the current I is measured. Which of the graphs shows the correct relationship between I and n?
(a)
(b)
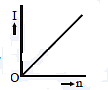
(c)
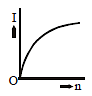
(d)
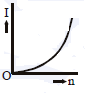
Ans: (a)
Solution: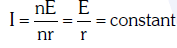
Q3: Current sensitivity of a moving coil galvanometer is 5 div/mA and its voltage sensitivity (angular deflection per unit voltage applied) is 20 div/V. The resistance of the galvanometer is
(a) 40 Ω
(b) 25 Ω
(c) 250 Ω
(d) 500 Ω
Ans: (c)
Solution:
Q4: A carbon resistor of (47 ± 4.7) kΩ is to be marked with rings of different colours for its identification. The colour code sequence will be
(a) Yellow - Green - Violet - Gold
(b) Yellow - Violet - Orange - Silver
(c) Violet - Yellow - Orange - Silver
(d) Green - Orange - Violet - Gold
Ans: (b)
Given, R = (47 ± 4.7) kΩ
= 47 × 103 ± 10% Ω
As per the colour code for carbon resistors, the colour assigned to numbers.
4 – Yellow
7 – Violet
3 – Orange
For ±10% accuracy, the colour is silver.
Hence, the bands of colours on carbon resistor in sequence are yellow, violet, orange and silver.
Note To remember the colour code sequence for carbon resistor, the following sentence should be kept in memory. B B Roy of Great Britain has a Very Good Wife.
2017
Q1: The resistance of a wire is 'R' ohm. If it is melted and stretched to 'n' times its original length, its new resistance will be :
(a) R/n
(b) n2R
(c) R/n2
(d) nR
Ans: (b)
Solution:
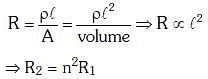
Q2: A potentiometer is an accurate and versatile device to make electrical measurements of E.M.F. because the method involves:
(a) Potential gradients
(b) A condition of no current flow through the galvanometer
(c) A combination of cells, galvanometer, and resistances
(d) Cells
Ans: (b)
Solution:
In zero deflection conditions, the potentiometer draws no current.
Q3: A potentiometer is an accurate and versatile device to make electrical measurement of EMF because the method involves
(a) cells
(b) potential gradients
(c) a condition of no current flow through the galvanometer
(d) a combination of cells, galvanometer and resistances
Ans: (c)
When a cell is balanced against potential drop across a certain length of potentiometer wire, no current flows through the cell
∴ emf of cell = potential drop across balance length of potentiometer wire.
So, potentiometer is a more accurate device for measuring emf of a cell or no current flows through the cell during measurement of emf.
2016
Q1: A potentiometer wire is 100 cm long and a constant potential difference is maintained across it. Two cells are connected in series first to support one another and then in opposite directions. The balance points are obtained at 50 cm and 10 cm from the positive end of the wire in the two cases. The ratio of emf's is :
(a) 3:2
(b) 5:1
(c) 5:4
(d) 3:4
Ans: (a)
Solution: According to the question, the emf of the cell is directly proportional to the balancing length.
i.e., E∝ ℓ ... (i)
Now, in the first case, cells are connected in series
That is,
Net EMF = E1 + E2
From equation (i),
E1 + E2 = 50 cm (given) ... (ii)
Now, the cells are connected in series in the opposite direction,
Net emf = E1 - E2
From equation (i)
E1 - E2 = 10 ... (iii)
From equation (ii) and (iii),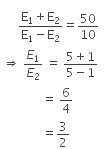
Q2: The charge flowing through a resistance R varies with time t as Q = at - bt2, where a and b are positive constants. The total heat produced in R is :
(a) a3R/b
(b) a3R/6b
(c) a3R/3b
(d) a3R/2b
Ans: (b)
Solution:
Given,
Charge, Q = at - bt2 ... (i)
We know that,
Current, I = dθ/dt
So, equation (i) can be written as,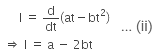
For maximum value of t, the current is given by,
a-2bt = 0
Therefore, t = a/2b ..(iii)
Total heat produced (H) can be given as,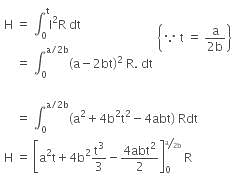
On solving the above equation, we get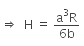
Given,
Charge, Q = at - bt2 ... (i)
We know that,
Current, I = dθ/dt
So, equation (i) can be written as,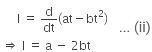
For maximum value of t, the current is given by,
a-2bt = 0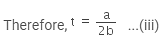
Total heat produced (H) can be given as,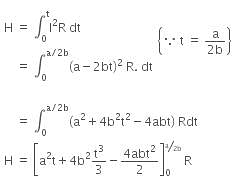
On solving the above equation, we get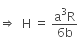
Q3: The potential difference (VA - VB )between the points A and B in the given figure is
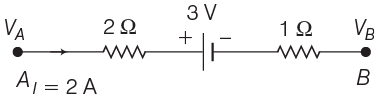
(a) –3 V
(b) +3 V
(c) +6 V
(d) +9 V
Ans: (d)
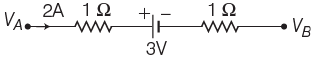
Applying KVL,
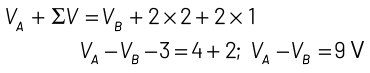
Q4: A filament bulb (500 W, 100 V) is to be used in a 230 V main supply. When a resistance R is connected in series, it works perfectly and the bulb consumes 500 W. The value of R is
(a) 230 Ω
(b) 46 Ω
(c) 26 Ω
(d) 13 Ω
Ans: (c)
If a rated voltage and power are given, then 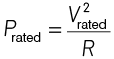
∴ Current in the bulb, i = P/V (∵ P = Vi)
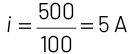
∴ Resistance of bulb,
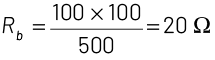
∴ Resistance R is connected in series.
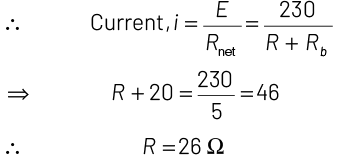
2015
Q1: A, B, and C are voltmeters of resistance R, 1.5 R and 3R respectively as shown in the figure. When some potential difference is applied between X and Y, the voltmeter readings are VA, VB and VC respectively.
Then :
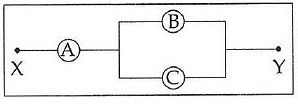
(a)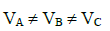
(b)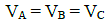
(c)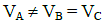
(d)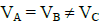
Ans: (b)
Solution: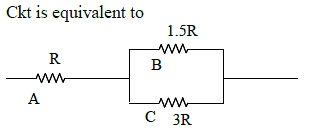
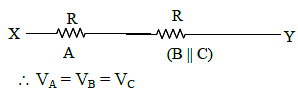
Q2: A potentiometer wire has a length of 4 m and resistance 8 Ω. The resistance must be connected in series with the wire and an accumulator of e.m.f. 2V, so as to get a potential gradient of 1 mV per cm on the wire is :
(a) 48 Ω
(b) 32 Ω
(c) 40 Ω
(d) 44 Ω
Ans: (b)
Solution:
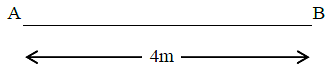
Figure alongside shows a potentiometer wire of length L = 4m and resistance RAB = 8Ω. Resistance connected in series is R.
When an accumulator of emf ε = 2V is used, we have current I given by,
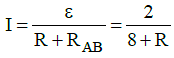
The resistance per unit length of the potentiometer wire is given by,
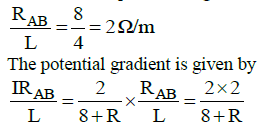

Q3: Across a metallic conductor of non-uniform cross-section, a constant potential difference is applied. The quantity which remains constant along the conductor is :
(a) electric filed
(b) current density
(c) Current
(d) drift velocity
Ans: (c)
Solution:
As the cross-sectional area of the conductor is non-uniform so current density will be different.
As, I = JA
It is clear from Eq. (i) that when an area increases the current density decreases so the number of the flow of electrons will be same and thus the current will be constant.
Q4: Two metal wires of identical dimensions are connected in series. If σ1 and σ2 are the conductivities of the metal wires respectively, the effective conductivity of the combination is
(a) 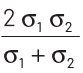
(b) 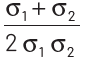
(c) 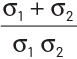
(d) 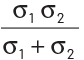
Ans: (a)
Net resistance of a metal wire having resistivity ρ, we have
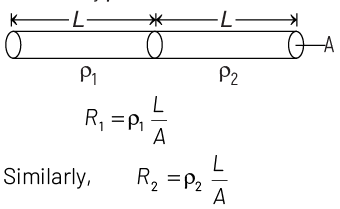
Then, net effective resistance of two metal wires,
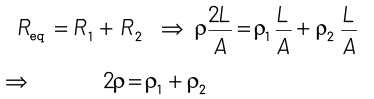
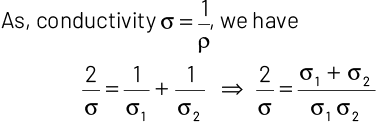
⇒ Net effective conductivity of combined wires,
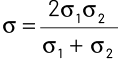
Q5: A circuit contains an ammeter, a battery of 30 V and a resistance 40.8 Ω all connected in series. If the ammeter has a coil of resistance 480 Ω and a shunt of 20 Ω, then reading in the ammeter will be
(a) 0.5 A
(b) 0.25 A
(c) 2 A
(d) 1 A
Ans: (a)
Effective resistance of a circuit,
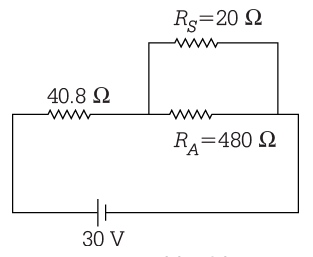
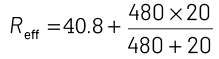
= 40.8 + 19.2 = 60 Ω
So, current flowing across ammeter,
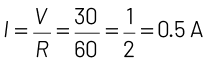
Hence, reading of ammeter = 0.5 A
Q6: A potentiometer wire of length L and a resistance r are connected in series with a battery of e.m.f. E0 and a resistance r1 . An unknown e.m.f. is balanced at a length l of the potentiometer wire.
The e.m.f. E will be given by
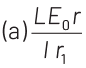
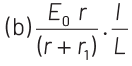
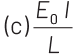
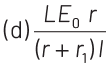
Ans: (b)
Consider a potentiometer wire of length L and a resistance r are connected in series with a battery of emf E0 and a resistance r1 as shown in figure. Current in wire 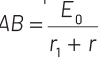
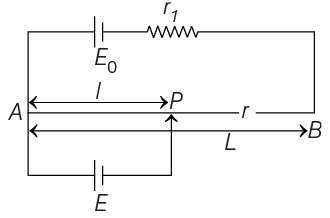
Potential gradient,
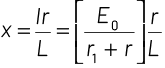
emf produced across E will be given by

2014
Q1: In an ammeter, 0.2% of the main current passes through the galvanometer. If the resistance of the galvanometer is G, the resistance of the ammeter will be:
(a) 1/500 G
(b) 500/499 G
(c) 1/499 G
(d) 499/500 G
Ans: (a)
Solution:
Let the shunt resistance be S.
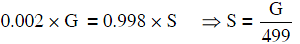
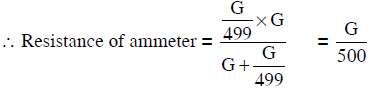
Q2: The resistance in the two arms of the meter bridge is 5 Ω and R Ω, respectively. When the resistance R is shunted with an equal resistance, the new balance point is at 1.6ℓ1. The resistance ‘R’ is :
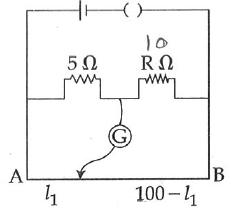
(a) 20 Ω
(b) 25 Ω
(c) 10 Ω
(d) 15 Ω
Ans: (b)
Solution:
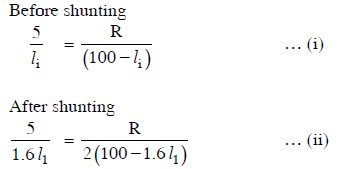
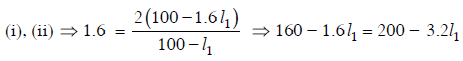
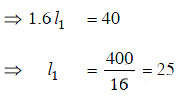
Q3: Two cities are 150 km apart. Electric power is sent from one city to another city through copper wires. The fall of potential per km is 8 volts and the average resistance per km is 0.5 Ω. The power loss in the wire is :
(a) 19.2 J
(b) 12.2 kW
(c) 19.2 W
(d) 19.2 kW
Ans: (d)
Solution:
Total voltage drop across wire = 150 x 8
= 1200 volt
Total resistance of wire
= 150 x 0.5 = 75 Ω
∴ current through wire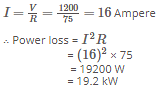
Q4: A potentiometer circuit has been set up for finding the internal resistance of a given cell. The main battery, used across the potentiometer wire, has an EMF of 2.0 V and a negligible internal resistance. The potentiometer wire itself is 4 m long. When the resistance, R connected across the given cell, has a value of:
(i) infinity
(ii) 9.5 Ω, the ‘balancing lengths’, on the potentiometer wire are found to be 3m and 2.85m, respectively.
The value of internal resistance of the cell is:
(a) 0.5 Ω
(b) 0.75 Ω
(c) 0.25 Ω
(d) 0.95 Ω
Ans: (a)
Solution:
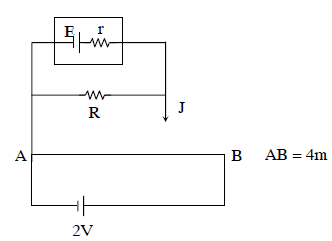
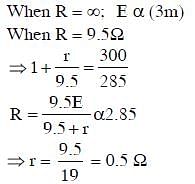
|
97 videos|334 docs|102 tests
|
FAQs on NEET Previous Year Questions (2014-2024): Current Electricity - Physics Class 12
| 1. What is the formula to calculate resistance in a circuit? |  |
| 2. How does the resistance of a conductor change with temperature? |  |
| 3. What is Ohm's Law and how is it applied in circuits? |  |
| 4. How do you calculate power in an electrical circuit? |  |
| 5. What is the difference between series and parallel circuits? |  |

|
Explore Courses for NEET exam
|

|


















