NEET Previous Year Questions (2014-2024): Dual Nature of Radiation & Matter | Physics Class 12 PDF Download
2024
Q1: If c is the velocity of light in free space, the correct statements about photon among the following are:A. The energy of a photon is E = hv.
B. The velocity of a photon is c.
C. The momentum of a photon, p = hv / c.
D. In a photon-electron collision, both total energy and total momentum are conserved.
E. Photon possesses positive charge.
Choose the correct answer from the options given below:
(a) A and B only
(b) A, B, C and D only
(c) A, C and D only
(d) A, B, D and E only [2024]
Ans: (b)
A. The energy of a photon is E = hv.
This statement is correct. The energy of a photon is given by the equation:
E = hv
where
E is the energy, h is Planck's constant, and v (also written as ν) is the frequency of the photon.
B. The velocity of a photon is c.
This statement is also correct. In free space (vacuum), the velocity of light, and hence the velocity of a photon, is constant and is denoted by c.
C. The momentum of a photon, p = hv / c.
This statement is correct as well. The momentum p of a photon can be expressed as:
p = E / c = hv / c
D. In a photon-electron collision, both total energy and total momentum are conserved.
This statement is correct. During collisions involving photons and electrons, such as Compton scattering, both energy and momentum are conserved.
E. Photon possesses positive charge.
This statement is incorrect. Photons are electrically neutral particles and do not possess any charge.
Based on the analysis, the correct statements are A, B, C, and D. Therefore, the correct answer is:
Option B
A, B, C, and D only
Q2: The graph which shows the variation of (1 / λ2) and its kinetic energy, E is (where λ is de Broglie wavelength of a free particle):
(a) 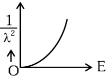
(b) 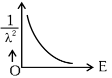
(c) 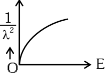
(d) 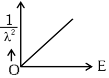 [2024]
[2024]
Ans: (d)
de-Broglie wavelength 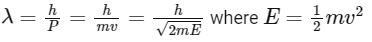
Squaring both sides,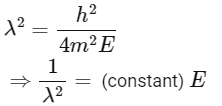
Graph passes through origin with constant slope.
2023
Q1: The work functions of Caesium (Cs), Potassium (K), and Sodium (Na) are 2.14 eV, 2.30 eV, and 2.75 eV respectively. If incident electromagnetic radiation has an incident energy of 2.20 eV, which of these photosensitive surfaces may emit photoelectrons?
A: Cs only
B: Both Na and K
C: K only
D: Na only
Ans: A
Solution: Energy of incident radiation = 2.80 eV
The work function of Cs → 2.14 eV
The work function of K → 2.30 eV
The work function of Na → 2.75 eV
Since the work functions of potassium and sodium are more than the energy of incident radiation hence photons may be emitted from cesium.
Q2: The minimum wavelength of X-rays produced by an electron accelerated through a potential difference of V volts is proportional to :
(a) 1/V
(b) 1/√V
(c) V2
(d) √V
Ans: (a)
Minimum wavelength of X-Rays is
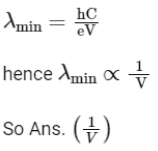
2022
Q1: The light rays having photons of energy 4.2 eV are falling on a metal surface having a work function of 2.2 eV. The stopping potential of the surface is
(a) 6.4 V
(b) 2 eV
(c) 2 V
(d) 1.1 V
Ans: (c)
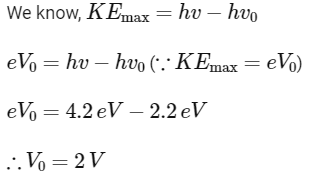
Q2: The threshold frequency of a photoelectric metal is v0. If light of frequency 4v0 is incident on this metal, then the maximum kinetic energy of emitted electrons will be :
(a) 4 hv0
(b) hv0
(c) 2 hv0
(d) 3 hv0
Ans: (d)
According to Einstein's photoelectric equation
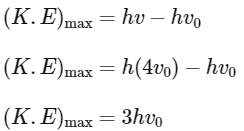
Q3: When two monochromatic lights of frequency, ν and ν/2 are incident on a photoelectric metal, their stopping potential becomes Vs/2 and Vs respectively. The threshold frequency for this metal is
A: 3ν
B: 2/3ν
C: 3/2ν
D: 2ν
Ans: (c)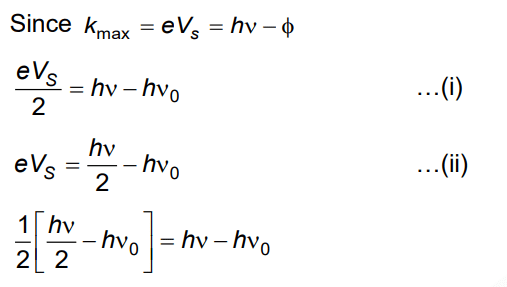
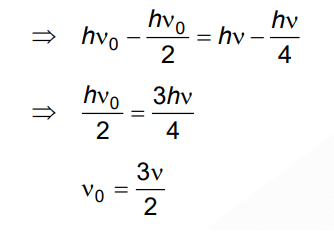
2021
Q1: The number of photons per second on average emitted by the source of monochromatic light of wavelength 600 nm, when it delivers the power of 3.3 × 10–3 watts will be : (h = 6.6 × 10–34 Js)
A: 1016
B: 1015
C: 1018
D: 1017
Ans: A
Solution: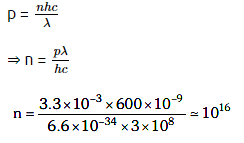
Q2: An electromagnetic wave of wavelength 'λ' is incident on a photosensitive surface of negligible work function. If 'm' is the mass of photoelectron emitted from the surface that has de-Broglie wavelength λd, then:
A: 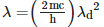
B: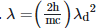
C: 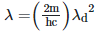
D: 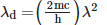
Ans: A
Solution: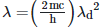
2020
Q1: Light of frequency 1.5 times the threshold frequency is incident on a photosensitive material. What will be the photoelectric current if the frequency is halved and the intensity is doubled?
A: one-fourth
B: Zero
C: doubled
D: four times
Ans: B
Solution:
f0 < f1 = 1.5 f0
∴ f2 = 0.75 f0
for given condition
fincident < fthreshold
so no photo electron emission
i = 0
Q2: The de-Broglie wavelength of an electron moving with kinetic energy of 144 eV is nearly
(a) 102 × 10−3 nm
(b) 102 × 10−4 nm
(c) 102 × 10−5 nm
(d) 102 × 10−2 nm
Ans: (d)
Kinetic energy of electron, K = 144 eV
⇒ eV = 144 eV
⇒ V = 144 V
∴ de-Broglie wavelength
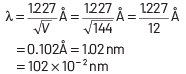
Q3: The wave nature of electrons was experimentally verified by
(a) de-Broglie
(b) Hertz
(c) Einstein
(d) Davisson and Germer
Ans: (a)
The wave nature of electrons was experimentally verified by de-Broglie.
2019
Q1: An electron is accelerated through a potential difference of 10,000 V. Its de-Broglie wavelength is, (nearly) : (me = 9 × 10–31 kg)
A: 12.2 × 10–13 m
B: 12.2 × 10–12 m
C: 12.2 × 10–14 m
D: 12.2 nm
Ans: B
For an electron accelerated through a potential V
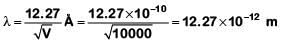
Q2: The work function of a photosensitive material is 4.0 eV.
This longest wavelength of light that can cause photon emission from the substance is (approximately)
(a) 3100 nm
(b) 966 nm
(c) 31 nm
(d) 310 nm
Ans: (d)
The work function of material is given by
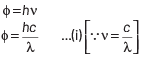
where, h = Planck’s constant = 6.63 × 10−34 J-s
c = speed of length = 3 × 108 ms−1
and λ = wavelength of light
Here, φ = 4 eV = 4 × 1.6 × 10 −19 J
Substituting the given values in Eq. (i), we get
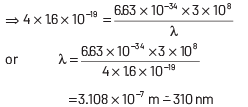
Q3: An electron is accelerated through a potential difference of 10,000 V.
Its de-Broglie wavelength is, (nearly) : (m e = 9 × 10−31 kg)
(a) 12.2 × 10−12 m
(b) 12.2 × 10−14m
(c) 12.2 nm
(d) 12.2 × 10−13m
Ans: (a)
Given, potential difference, V = 10000 V If electron is accelerated through a potential of V volt, then the wavelength associated with it is given by
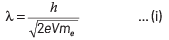
where, h = Planck’s constant = 6.63 × 10−34 J-s,
e = electronic charge = 1.6 × 10−19 C a
nd me = mass of electron = 9 × 10−31 kg Substituting these values in Eq. (i),
we get
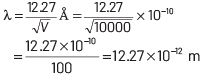
2018
Q1: An electron of mass m with an initial velocity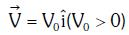 enters an electric field
enters an electric field 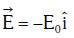 E0 = constant > 0) at t = 0. If λ0 is its de-Broglie wavelength initially, then its de-Broglie wavelength at time t is:
E0 = constant > 0) at t = 0. If λ0 is its de-Broglie wavelength initially, then its de-Broglie wavelength at time t is:
A: 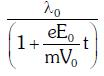
B: 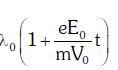
C: λ0t
D: λ0
Ans: A
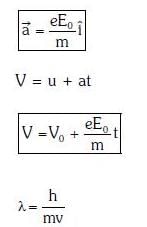
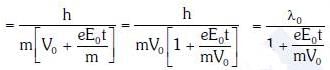
Q2: When the light of frequency 2v0 (where v0 is threshold frequency), is incident on a metal plate, the maximum velocity of electrons emitted is v1. When the frequency of the incident radiation is increased to 5 v0, the maximum velocity of electrons emitted from the same plate is v2. The ratio of v1 to v2 is :-
A: 1 : 2
B: 1 : 4
C: 4 : 1
D: 2 : 1
Ans: A
Solution:
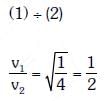
Q3: When the light of frequency 2ν0 (where, ν0 is threshold frequency), is incident on a metal plate, the maximum velocity of electrons emitted is v1 . When the frequency of the incident radiation is increased to 5ν0 , the maximum velocity of electrons emitted from the same plate is v2 . The ratio of v1 to v2 is
(a) 4 : 1
(b) 1 : 4
(c) 1 : 2
(d) 2 : 1
Ans: (c)
According to the Einstein’s photoelectric equation,
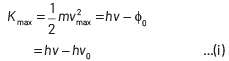
where, Kmax is the maximum kinetic energy of photoelectrons having maximum velocity vmax. When incident frequency of light, v = 2v0 Substituting the value of ν in Eq. (i), we get
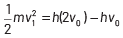
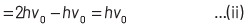
If incident frequency of radiation, ν = 5ν0 Substituting the value of ν in Eq. (i), we get
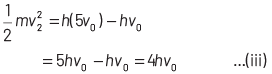
On dividing Eq. (ii) by Eq (iii), we get
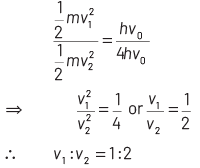
2017
Q1: The photoelectric threshold wavelength of silver is 3250 × 10–10m. The velocity of the electron ejected from a silver surface by ultraviolet light of wavelength 2536 × 10–10 m is:
(Given h = 4.14 × 10–15 eVs and c = 3 × 108 ms–1)
A: ≈ 0.6 × 106 ms–1
B: ≈ 61 × 103 ms–1
C: ≈ 0.3 × 106 ms–1
D: ≈ 6 × 105 ms–1
Ans: A
Solution:
Q2: The de-Broglie wavelength of a neutron in thermal equilibrium with heavy water at a temperature T (Kelvin) and mass m, is
(a) 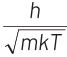
(b) 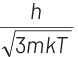
(c) 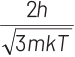
(d) 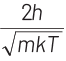
Ans: (b)
Thinking Process de-Broglie wavelength associated with a moving particle can be given as
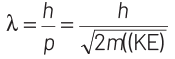
At thermal equilibrium, temperature of neutron and heavy water will be same. This common temperature is given as, T. Also, we know that, kinetic energy of a particle
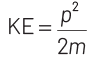
where, p = momentum of the particle m = mass of the particle Kinetic energy of the neutron is
K.E. = 3/2 kT
∴ de-Broglie wavelength of the neutron
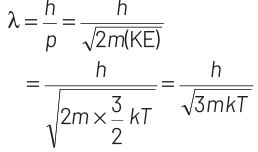
2016
Q1: When a metallic surface is illuminated with radiation of wavelength λ the stopping potential is V. If the same surface is illuminated with radiation of wavelength 2λ, the stopping potential is V/4. The threshold wavelength for the metallic surface is :
A: 3λ
B: 4λ
C: 5λ
D: 5/2λ
Ans: A
When a metallic surface is illuminated with radiation of wavelength λ, the stopping potential is V.
The photoelectric equation can be written as,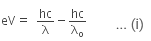
Now, when the same surface is illuminated with radiation of wavelength 2λ, the stopping potential is V/4. So, the photoelectric equation can be written as,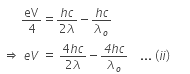
From equations (i) and (ii), we get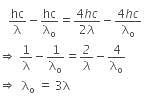
When a metallic surface is illuminated with radiation of wavelength λ stopping potential is V.
The photoelectric equation can be written as,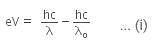
Now, when the same surface is illuminated with radiation of wavelength 2λ, the stopping potential is V/4. So, the photoelectric equation can be written as,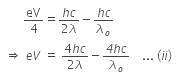
From equations (i) and (ii), we get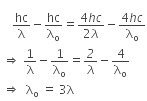
Q2: An electron of mass m and a photon have the same energy E. The ratio of de-Broglie wavelengths associated with them is:
A:
B:
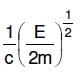
C:
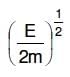
D: c(2mE)1/2
Ans: B
Given that an electron has a mass of m.
De-Broglie wavelength for an electron will be given as,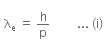
where,
h is the Planck's constant, and
p is the linear momentum of the electron
The kinetic energy of the electron is given by, 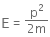
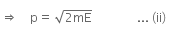
From equation (i) and (ii), we have
The energy of a photon can be given as,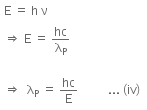
Hence, λP is the de-Broglie wavelength of the photon.
Now, dividing equation (iii) by (iv), we get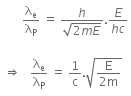
Q3: Photons with energy 5 eV are incident on a cathode C in a photoelectric cell. The maximum energy of emitted photoelectrons is 2 eV. When photons of energy 6 eV are incident on C, no photoelectrons will reach the anode A, if the stopping potential of A relative to C is
(a) + 3 V
(b) + 4 V
(c) – 1 V
(d) – 3 V
Ans: (d)
Key Idea Use Einstein’s photoelectric equation.
We know that, E = (KE)max + Work function (φ)
where, φ = hν0 E = hν
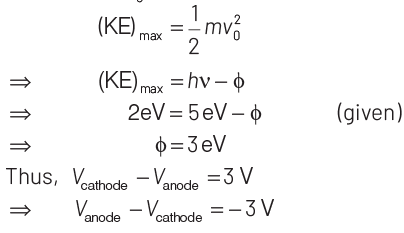
Q4: Electrons of mass m with de-Broglie wavelength λ fall on the target in an X-ray tube. The cut-off wavelength (λ 0 ) of the emitted X-ray is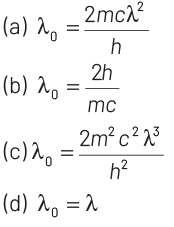
Ans: (a)
Cut-off wavelength occurs when incoming electron looses its complete energy in collision. This energy appears in the form of X-rays.
Given, mass of electrons = m
de-Broglie wavelength = λ
So, kinetic energy of electron = p2/2m
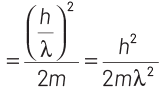
Now, maximum energy of photon can be given by
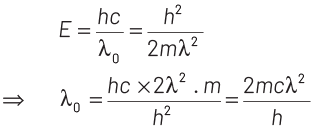
2015
Q1: A certain metallic surface is illuminated with monochromatic light of wavelength, λ. The stopping potential for photo-electric current for this light is 3V0. If the same surface is illuminated with light of wavelength 2λ, the stopping potential is V0. The threshold wavelength for this surface for the photoelectric effect is :
A: λ/6
B: 6λ
C: 4λ
D: λ/4
Ans: C
We have,
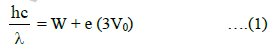
where W is the work function and (3V0) is the stopping potential when monochromatic light of wavelength λ is used.
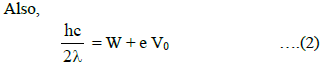
where V0 is the stopping potential when monochromatic light of wavelength 2λ is used.
Subtracting equation (2) from equation (1)
We get,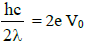
∴ 
Substituting in equation (2) we get,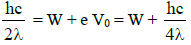
∴ 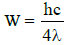
The threshold wavelength is therefore 4λ.
Q2: Which of the following figures represents the variation of the particle momentum and the associated de-Broglie wavelength?
A:
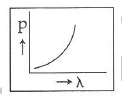
B:
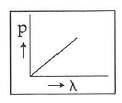
C: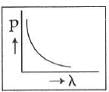
D:
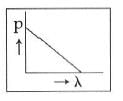
Ans: C
The de-Broglie wavelength is given by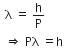
This equation is in the form of yx = c, which is the equation of a rectangular hyperbola.
The de-Broglie wavelength is given by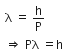
This equation is in the form of yx = c, which is the equation of a rectangular hyperbola.
(a)
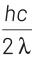
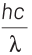
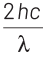
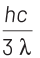
According to Einstein’s photoelectric equation,
E = Kmax + φ
where, Kmax is maximum kinetic energy of emitted electron and φ is work function of an electron.

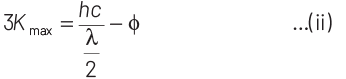
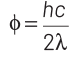
2014
Q1: When the energy of the incident radiation is increased by 20%, the kinetic energy of the photoelectrons emitted from a metal surface increases from 0.5 eV to 0.8 eV. The work function of the metal is :
A: 1.3 eV
B: 1.5 eV
C: 0.65 eV
D: 1.0 eV
Ans: D
Solution:
The original energy of the photon is E0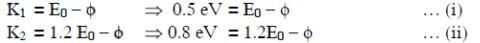
From equation (i) and (ii)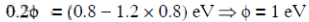
Q2: If the kinetic energy of the particle is increased to 16 times its previous value, the percentage change in the de−Broglie wavelength of the particle is :
A: 60
B: 50
C: 25
D: 75
Ans: D
Solution: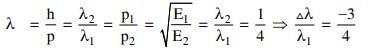
Q3: Light of wavelength 500 nm is incident on a metal with work function 2.28 eV. The de-Broglie wavelength of the emitted electron is
(a) < 2.8 × 10− 10 m
(b) < 2.8 × 10− 9 m
(c) ≥ 2.8 × 10− 9 m
(d) ≤ 2.8 × 10− 12 m
Ans: (c)
As, energy of photon, E = hν
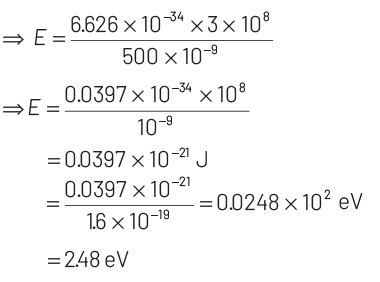
According to Einstein’s photoelectric emission, we have
KEmax = E − W = 2.48 − 2.28 = 0.2 eV
For de-Broglie wavelength of the emitted electron,
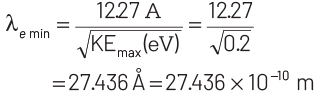
Thus, minimum wavelength of the emitted electron is
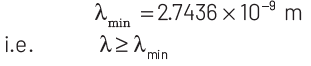
|
97 videos|336 docs|104 tests
|
FAQs on NEET Previous Year Questions (2014-2024): Dual Nature of Radiation & Matter - Physics Class 12
| 1. What is the photoelectric effect? |  |
| 2. How does the photoelectric effect support the wave-particle duality of light? |  |
| 3. What is the threshold frequency in the context of the photoelectric effect? |  |
| 4. How does the kinetic energy of emitted electrons in the photoelectric effect depend on the frequency of light? |  |
| 5. What is the significance of the work function in the photoelectric effect? |  |

|
Explore Courses for NEET exam
|

|













