Filter Characteristics of Linear Systems | Signals and Systems - Electronics and Communication Engineering (ECE) PDF Download
A system for which the principle of superposition and the principle of homogeneity is valid is called a linear system.
Filter Characteristics of Linear Systems
- For a given linear system, an input signal 𝑥(𝑡) produces a response signal 𝑦(𝑡). Therefore, the system processes the input signal 𝑥(𝑡) according to the characteristics of system.
- The spectral density function of the input signal 𝑥(𝑡) is given by 𝑋(𝑠) in s-domain or 𝑋(𝜔) in frequency domain.
- Also, the spectral density function of the response signal 𝑦(𝑡) is given by 𝑌(𝑠) in s-domain and 𝑌(𝜔) in frequency domain. Therefore,

Or,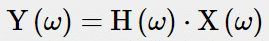
Where, 𝐻(𝑠) or 𝐻(𝜔) is the transfer function of the system. - Thus, the system modifies the spectral density function of the input signal. The linear system acts as a filter for various frequency components, i.e., some frequency components are amplified and some frequency components are attenuated. Also, some frequency components may remain unaffected.
- Similarly, each frequency component suffers a different amount of phase shift in the process of transmission. Hence, the system modifies the spectral density function of the input signal according to its filter characteristics.
- This modification is performed according to the transfer function 𝐻(𝑠) or 𝐻(𝜔) of the system.
- The transfer function represents the response of the system for various frequency components.
- The transfer function 𝐻(𝜔) acts as a weighted function or spectral shaping function to the different frequency components in the input signal. Therefore, an LTI system acts as a filter.
Depending upon the response of the system for various frequency components of the input signal, i.e., filter characteristics, a given linear system can act as following types of filter −
Types of Filters
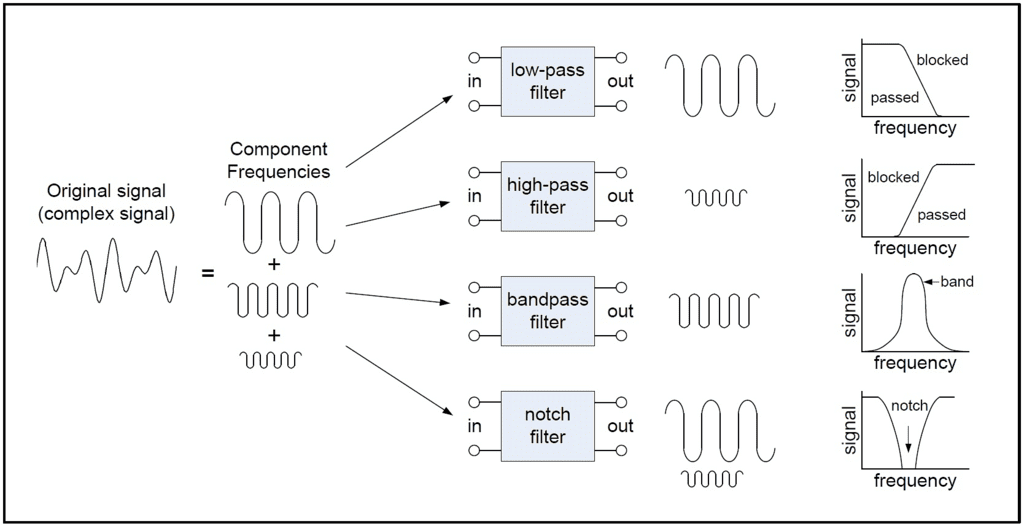 Types of Filters
Types of Filters
- When the LTI system allows the transmission of only low frequency components and blocks all the high frequency components. Then, the system is called the low-pass filter (LPF).
- When the LTI system allows the transmission of only high frequency components and blocks all the low frequency components, the system is called the high-pass filter (HPF).
- When the system allows the transmission of only a particular band of frequencies and blocks all other frequency components. Then, the system is called the band-pass filter (BPF).
- When the LTI system rejects only a particular band of frequencies and allows all other frequency components. The system is called band rejection filter (BRF).
The band of frequency components which is allowed by the filter is called passband and the band of frequency components which is not allowed to pass through the filter is called stop-band or rejection-band.
Response Curves of Filters
- Response curves are utilized to depict the behavior of a filter. A response curve is essentially a graphical representation that illustrates the relationship between the attenuation ratio (VOUT / VIN) and frequency (as depicted in Figure below).
- Attenuation is frequently quantified in decibels (dB), while frequency can be represented in two ways: angular form (ω) measured in radians per second (rad/s) or the more widely used form, denoted as 'f,' which is measured in Hertz (Hz) or cycles per second. These two representations are linked by the equation ω = 2πf.
- Ultimately, filter response curves can be presented in three formats: linear-linear, log-linear, or log-log. The most commonly adopted approach is to display decibels on the vertical (y) axis and logarithmic frequency on the horizontal (x) axis.
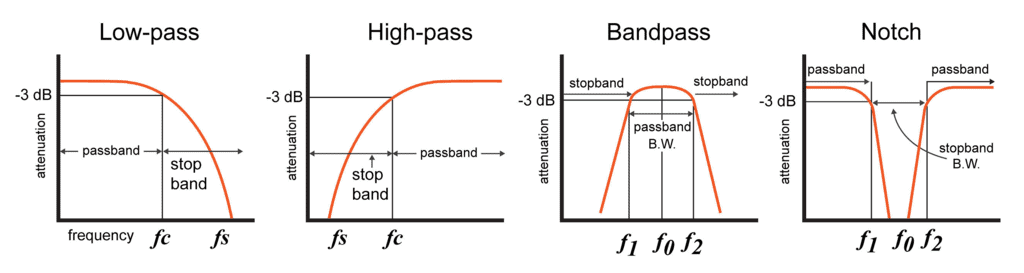
Please note: A notch filter is a type of bandstop filter characterized by a narrow bandstop bandwidth. Notch filters are employed to reduce the amplitude of a specific range of frequencies.
Here are some common technical terms used in describing filter response curves:
- -3dB Frequency (f3dB): This term, pronounced as "minus 3dB frequency," corresponds to the input frequency at which the output signal's amplitude decreases by -3dB compared to the input signal. The -3dB frequency is also known as the cutoff frequency. It's the point where the output power is reduced by half, often called the "half-power frequency." This frequency is where the output voltage equals the input voltage multiplied by 1/√2. In low-pass and high-pass filters, there is a single -3dB frequency. However, band-pass and notch filters have two -3dB frequencies, typically referred to as f1 and f2.
- Center frequency (f0): The center frequency, used in band-pass and notch filters, is a central frequency situated between the upper and lower cutoff frequencies. The center frequency is typically calculated as the arithmetic mean or geometric mean of the lower and upper cutoff frequencies.
- Bandwidth (β or B.W.): Bandwidth refers to the width of the passband, which is the range of frequencies that experience minimal attenuation when passing through the filter from input to output.
- Stopband frequency (fs): This denotes a specific frequency at which the attenuation reaches a predefined level.
For low-pass and high-pass filters, frequencies beyond the stopband frequency are termed the stopband.
For band-pass and notch filters, two stopband frequencies exist, and the frequencies in between are also known as the stopband. - Quality factor (Q): The quality factor of a filter describes its damping characteristics. In the time domain, damping corresponds to the amount of oscillation in the system's step response. In the frequency domain, higher Q values result in more prominent peaking (either positive or negative) in the system's magnitude response. For band-pass or notch filters, Q represents the ratio between the center frequency and the -3dB bandwidth (the difference between f1 and f2).
For both band-pass and notch filters:
Q = f0 / (f2 - f1)
|
36 videos|117 docs|63 tests
|
FAQs on Filter Characteristics of Linear Systems - Signals and Systems - Electronics and Communication Engineering (ECE)
| 1. What are the different types of filters used in electrical engineering? |  |
| 2. What are the characteristics of linear systems in filter design? |  |
| 3. How do low-pass filters work in electrical engineering? |  |
| 4. What is the purpose of a band-pass filter in electrical engineering? |  |
| 5. What is the significance of stability in filter design? |  |
















