JEE Advanced (Subjective Type Questions): Complex Numbers | Chapter-wise Tests for JEE Main & Advanced PDF Download
Q. 1. Express 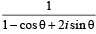 in the form x + iy. (1978)
in the form x + iy. (1978)
Ans. 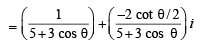
Sol.
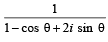
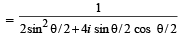 =
= 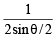
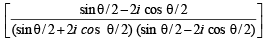
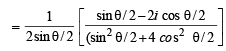
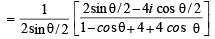
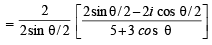
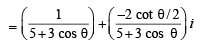
which is of the form X + iY.
Q. 2. If x = a + b, y = aγ + bβ and z = aβ + bγ where γ and b are the complex cube roots of unity, show that xyz = a3 + b3. (1978)
Ans. Sol. As b and γ are the complex cube roots of unity therefore,
let β = ω and γ = ω2
so that ω + ω2 + 1= 0 and ω3 =1.
Then xyz = (a + b) (aω2 + bω) (aω + bω2)
= (a + b) (a2ω3 + abω4 + abω2 + b2ω3)
= (a + b) (a2 + abω + abω2 + b2) (using ω3 = 1)
= (a + b) (a2 + ab(ω + ω2 ) + b2)
= (a + b) (a2 – ab + b2 ) (using ω+ ω2 = –1)
= a3 + b3 Hence proved.
Q. 3. If x + iy = 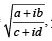 prove that (x2 + y2)2 =
prove that (x2 + y2)2 = 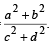 (1979)
(1979)
Ans.
Sol. Given x + iy =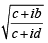
⇒ (x + iy)2 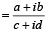 ....(1)
....(1)
Taking conjugate on both sides, we get
(x - iy)2 = 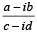 ....(2)
....(2)
Multiply (1) and (2), we get
(x2 + y2)2 = 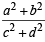
Q. 4. Find the real values of x and y for which the following equation is satisfied 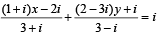 (1980)
(1980)
Ans.
Sol. 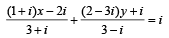
⇒ (4 + 2i) x – 6i – 2 + (9 – 7i) y + 3i – 1= 10i
⇒ (4x + 9y – 3) + (2x – 7y – 3) i = 10i
⇒ 4x + 9y – 3 = 0 and 2x – 7y – 3 = 10
On solving these two, we get x = 3, y = – 1
Q. 5. Let the complex number z1, z2 and z3 be the vertices of an equilateral triangle. Let z0 be the circumcentre of the triangle.
Then prove that z12 + z22 + z32 = 3z02. (1981 - 4 Marks)
Ans.
Sol.
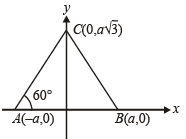
Let us consider the equilateral Δ with each side of length 2a and having two of its vertices on x-axis namely A (–a,0) and B (a, 0), then third vertex C will clearly lie on y-axis s.t.
OC = 2a sin 60°=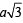 ∴ C has the co-ordinates (0,
∴ C has the co-ordinates (0,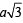 ).
).
Now in the form of complex numbers if A, B and C are represented by z1, z2, z3 then z1 = - a ; z2 = a ; z3= a3i As in an equilateral Δ, centriod and circumcentre coincide, we get
Circumcentre, 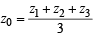
⇒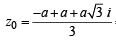
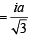
Now, 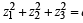
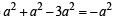
and 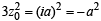
∴ Clearly 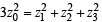
Q. 6. Prove that the complex numbers z1, z2 and the origin form an equilateral triangle only if z12 + z22 –z1z2 = 0. (1983 - 3 Marks)
Ans. Sol. We know that if z1, z2, z3 are vertices of an equilateral Δ then
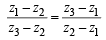
Here
We get 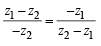
⇒ - ( z1 - z2)= z1z2
⇒ - z12 - z22 + 2 z1 z2= z1z2 ⇒ z12 + z22 - z1z2= 0.
Q. 7. If 1, a1, a2 ......, an – 1 are the n roots of unity, then show that (1 – a1)(1 – a2) (1 – a3) ....(1 – an – 1) = n (1984 - 2 Marks)
Ans.
Sol. 1, a1, a2, .... an – 1 are the n roots of unity. Clearly above n values are roots of eq. xn – 1 = 0
Therefore we must have (by factor theorem)
xn – 1 = (x – 1) (x – a1) (x – a2) ....(x – an – 1) ....(1)
⇒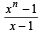 = (x – a1) (x – a2) ....(x – an – 1) ....(2)
= (x – a1) (x – a2) ....(x – an – 1) ....(2)
Differentiating both sides of eq. (1), we get
nxn – 1 = (x – a1) (x – a2) ... (x – an – 1) + (x – 1) (x – a2) ....(x – an – 1) +....+ (x – 1) (x – a1) ... (x – an – 2)
For x = 1, we get n = (1– a1) (1– a2) .... (1 – an – 1)
[All the terms except first contain (x – 1) and hence become zero for x = 1] Proved.
Q. 8. Show that the area of the triangle on the Argand diagram formed by the complex numbers z, iz and z + iz is 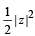 (1986 - 2½ Marks)
(1986 - 2½ Marks)
Ans.
Sol. Let A = z = x + iy, B = iz = – y + ix,
C = z + iz = (x – y) + i (x + y)
Now, area of ΔABC =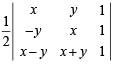
Operating R2 – R1 , R3 – R1, we get
Δ = 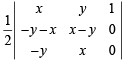
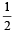 |x(-y - x) +y (x - y)|
|x(-y - x) +y (x - y)|
= 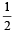 |- xy -x2 + xy-y2| =
|- xy -x2 + xy-y2| = 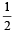 |-x2-y2|
|-x2-y2|
= 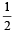 |x2+y2|=
|x2+y2|= 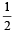 |z2| Hence Proved.
|z2| Hence Proved.
Q. 9. Let Z1 = 10 + 6i and Z2 = 4 + 6i. If Z is any complex number such that the argument of 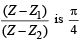 , then prove that |Z – 7 – 9i| =
, then prove that |Z – 7 – 9i| = 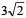 . (1990 - 4 Marks)
. (1990 - 4 Marks)
Ans. Sol. We are given that z1 = 10 + 6i and z2 = 4 + 6i
Also arg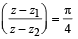
⇒ arg (z – z1) – arg (z – z2) =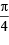 = NOTE THIS STEP
= NOTE THIS STEP
⇒ arg ((x+iy) - (10 + 6i)) -arg((x+iy) - (4 + 6i)) =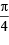
⇒ arg [(x - 10) +i (y - 6)] -arg [( x - 4) + i(y - 6)] =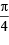
⇒ tan-1 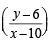 - tan-1
- tan-1 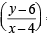 =
=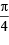
⇒ tan-1  =
=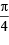
⇒ 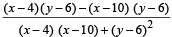 = tan
= tan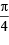
⇒ (x – 4 – x + 10) (y – 6) = (x – 4) (x – 10) + (y – 6)2
⇒ 6y – 36 = x2 + y2 –14x – 12y + 40 + 36
⇒ x2 + y2 – 14x – 18y + 112 = 0
⇒ (x2 – 14x + 49) + (y2 – 18y + 81) =18
⇒ ( x - 7)2 + (y - 9)2= (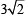 )2
)2
⇒ ( x + iy) - (7 + 9i)= 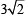
⇒ z - (7 + 9i)= 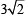 . Hence Proved.
. Hence Proved.
Q. 10. If iz3 + z2 – z + i = 0, then show that | z | = 1. (1995 - 5 Marks)
Ans.
Sol. Dividing through out by i and knowing that 1/i = -i we get i =-
z3 – iz2 + iz + 1= 0
or z2(z – i) + i (z – i) = 0
as 1= – i2 or (z – i) (z2 + i) = 0 ∴z = i
or z2 = – i
∴ | z | = | i | = 1 or | z2 | = | z |2 = | – i | = 1
⇒ | z | =1
Hence in either case | z | = 1
Q. 11. If |Z|≤ 1, |W |≤ 1, show that
|Z -W|2 ≤ (|Z|- |W|)2 +( Arg Z- ArgW)2 (1995 - 5 Marks)
Ans. Sol. Let Z = r1 (cos θ1 +i sinθ1)
and W = r2 (cos θ2 +i sinθ2)
We have | Z | = r1, | W | = r2, Arg Z= θ1 and
Arg W = θ2
Since | Z | ≤ 1, |W |≤ 1, it follows that r1 ≤ and r2≤ 1
We have Z - W = (rl cos θ1 -r2 cosθ2)
+i(rl sin θ1 -r2 sinθ2)
|Z - W|2 = (r1 cos θ1 -r2 cosθ2) + (r1 sin θ1 -r2 sinθ2)2
= r12 cos2 θ1 + r22 cos2 θ2 - 2 r1r2 cos θ1 cos θ2 +r12 sin2θ1
+ r2 sin θ2 - 2 r1r2 sin θ1 sinθ2
= r12 (cos2 θ1 + sin2 θ1) +r22 (cos2 θ2 + sin2θ2)
- 2 r1r2 (cos θ1 cos θ2 + sin θ1 sinθ2)
= = r12 + r22 - 2 r1r2 cos ( θ1 -θ2)
= (r1 - r2)2 + 2r1r2[1 - cos ( θ1 -θ2)]
= (r1 - r2) 2 + 4 r1r2 sin2 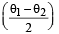
= |r1 - r2|2 + 4 r1r2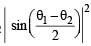
≤ |r1 - r2|2 + 4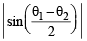 [ ∵ r1, r2 ≤ 1]
[ ∵ r1, r2 ≤ 1]
But | sin θ| ≤| θ| ∀ θ∈R
NOTE THIS STEP
Therefore,
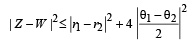
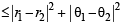
Thus 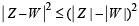 + (Arg Z- ArgW)2
+ (Arg Z- ArgW)2
12. Find all non-zero complex numbers Z satisfying 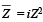 (1996 - 2 Marks)
(1996 - 2 Marks)
Ans.
Sol. Let z = x + iy then 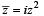
⇒ x – iy = i(x2 – y2 + 2ixy)
⇒ x – iy = i(x2 – y2) – 2xy
⇒ x (1 + 2y) = 0 ; x2 – y2 + y = 0
⇒ x = 0 or y = -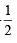 ⇒ x = 0, y = 0, 1
⇒ x = 0, y = 0, 1
or 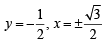
For non zero complex number z
x = 0, y = 1;
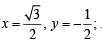
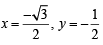
∴ 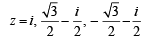
Q. 13. Let z1 and z2 be roots of the equation z2+pz+q = 0, where the coefficients p and q may be complex numbers. Let A and B represent z1 and z2 in the complex plane. If ∠AOB = a ≠ 0 and OA = OB, where O is the origin, prove that
p2 = 4q cos2 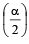 (1997 - 5 Marks)
(1997 - 5 Marks)
Ans. Sol. z2 + pz + q = 0
z1+ z2= – p, z1z2 = q
By rotation through a in anticlockwise direction
z2 = z1 eiα ....(1)
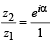
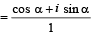
Add 1 in both sides to get z1 + z2 = – p
∴ 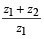
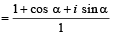
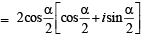
or 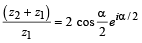
On squaring (z1 + z2)2 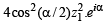
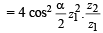 =
=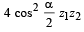
or p2 = 4q cos2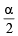
Q. 14. For complex numbers z and w, prove that |z|2 w-|w|2 z = z –w if and only if z = w or z 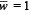 . (1999 - 10 Marks)
. (1999 - 10 Marks)
Ans.
Sol. Given that z and w are two complex numbers.
To prove |z|2 w –|w|2 z = z – w ⇔ z = w or z 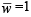
First let us consider
|z|2 w –|w|2 z = z – w ....(1)
⇒ z (1 +|w|2 = w ( 1 +|z|2)
⇒ 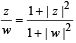 = a real number
= a real number
⇒ 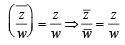
⇒ 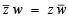 ....(2)
....(2)
Again from equation (1),
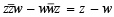
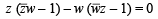
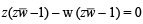 (Using equation (2))
(Using equation (2))
⇒ 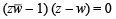 ⇒
⇒ 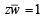 or z = w
or z = w
Conversely if z = w then
L.H.S. of (1) =|w|2 w –|w|2 w = 0.
R.H.S. of (1) = w – w = 0
∴ (1) holds
Also if z 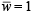 then
then
L.H.S. of (1) 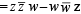
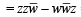 = z – w = R .H.S. Hence proved.
= z – w = R .H.S. Hence proved.
Q. 15. Let a complex number α, α ≠ 1 , be a root of the equation zp+q - zp - zq + 1 = 0, where p, q are distinct primes. Show that either 1 + α + α2 + .... + αp - 1 = 0 or 1 + α + α2 + ... + αq - 1 = 0, but not both together. (2002 - 5 Marks)
Ans.
Sol. The given equation can be written as
(zp –1) (zq – 1) = 0
∴ z = (1)1/p or (1)1/q ....(1)
where p and q are distinct prime numbers.
Hence both the equations will have distinct roots and as
z ≠ 1, both will not be simultaneously zero for any value of z given by equations in (1)
NOTE THIS STEP
Also 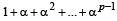
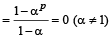
or 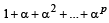
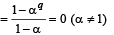
Because of (1) either αp = 1 and if αq = 1 but not both simultaneously as p and q are distinct primes.
Q. 16. If z1 and z2 are two complex numbers such taht |z1| < 1< |z2| then prove that 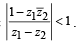 (2003 - 2 Marks)
(2003 - 2 Marks)
Ans.
Sol. Given that|z1|<1<|z2|
Then 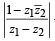 < 1 is true
< 1 is true
if 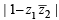 <|z1– z2|is true
<|z1– z2|is true
if 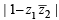 < |z1– z2|2 is true
< |z1– z2|2 is true
if 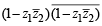 < (z1 - z2)
< (z1 - z2) 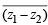 is true
is true
if 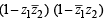 < (z1 - z2)
< (z1 - z2)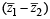
if 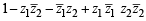
- 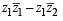 is true
is true
if 1+|z1|2|z2|2<|z1|2+|z2|2 is true
if (1 –|z1|2 ) (1–|z2|2 ) < 0 is true.
which is obviously true as|z1|< 1<|z2|
⇒|z1|2 < 1<|z2|2
⇒|1–|z1|2 > 0 and (1–|z2|2) < 0 Hence proved.
Q. 17. Prove that there exists no complex number z such that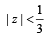 and
and 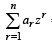 = 1 where |ar| < 2. (2003 - 2 Marks)
= 1 where |ar| < 2. (2003 - 2 Marks)
Ans.
Sol. Let us consider, 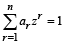 where | ar |< 2
where | ar |< 2
⇒ a1 z + a2z2 + a3z3 + ... + anzn = 1
⇒ |a1z + a2z2 + a3z3 + ...+ anzn| = 1 ....(1)
But we know that | z1 + z2 | ≤ | z1 |+ |z2|
∴ Using its generalised form, we get
| a1 z + a2 z2 + a3 z3 + ... + an zn |
≤ | a1 z | + | a2 z2 | + ... + | an zn |
⇒ 1 ≤ | a1| | z | + | a2 | |z2 | + | a3 | | z3 | + ... + | an | |zn | (Using eqn (1))
But given that | ar | < 2 ∀ r= 1(1)n
∴ 1< 2 [ | z | + | z |2 + | z |3 + ... + | z |n ] [Using | zn | = | z |n ]
⇒ 1< 2 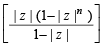 ⇒
⇒ 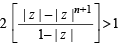
⇒ 2 [ | z | – | z |n+1] > 1– | z | (∵ 1– | z | > 0 as | z | < 1/3)
⇒ [|z| -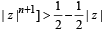 ⇒
⇒ 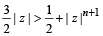
⇒ [|z| 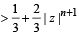 ⇒ [|z| >
⇒ [|z| >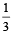
which is a contradiction as given that [|z| <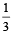
∴ There exist no such complex number.
Q. 18. Fin d the centre an d radius of circle given by 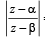 = k, k ≠ 1
= k, k ≠ 1
where, z = x + iy, α = α1 + iα2, β = β1 + iβ2 (2004 - 2 Marks)
Ans. Sol. We are given that
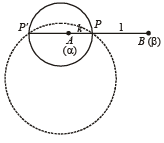
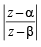 = k ⇒ | z -a|= k |z -b|
= k ⇒ | z -a|= k |z -b|
Let pt. A represents complex number α and B that of β, and P represents z. then | z – α | = k | z – β |
⇒ z is the complex number whose distance from A is k times its distance from B. i.e. PA = k PB
⇒ P divides AB in the ratio k : 1 internally or externally (at P').
Then 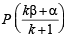 and
and 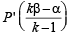
Now through PP' there can pass a number of circles, but with given data we can find radius and centre of that circle for which PP' is diameter.
And hence then centre = mid. point of PP'
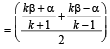
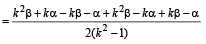
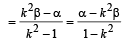
Also radius
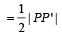
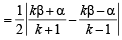
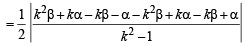
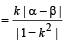
Q. 19. If one the vertices of the square circumscribing the circle |z – 1| = 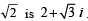 . Find the other vertices of thesquare. (2005 - 4 Marks)
. Find the other vertices of thesquare. (2005 - 4 Marks)
Ans. Sol. The given circle is |z - 1|=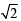 where z0=1 is the centre and
where z0=1 is the centre and 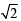 is radius of circle. z1 is one of the vertex of square inscribed in the given circle.
is radius of circle. z1 is one of the vertex of square inscribed in the given circle.
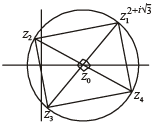
Clearly z2 can be obtained by rotating z1 by an ∠90° in anticlockwise sense, about centre z0 Thus, z2 – z0 = (z1 – z0) eiπ/2
or z2 - 1 = (2 + i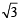 - 1)i ⇒ z2 =i -
- 1)i ⇒ z2 =i -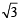 +1
+1
z2 = (1 - 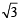 )+i
)+i
Again rotating z2 by 90° about z0 we get
z3 – z0 = (z2 – z0) i
⇒ z3 -1= [(1- 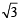 ) + i -1] i = -
) + i -1] i = - 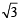 i-1 ⇒ z3 =-i
i-1 ⇒ z3 =-i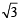
and similarly 1 = (- i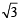 - 1) i =
- 1) i =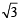 -i
-i
⇒ z4 = ( 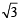 + 1)-i
+ 1)-i
Thus the remaining vertices are
(1 - 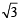 ) + i, - i
) + i, - i 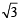 , (
, (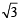 + 1)-i
+ 1)-i
|
481 docs|964 tests
|
















