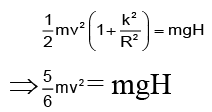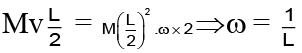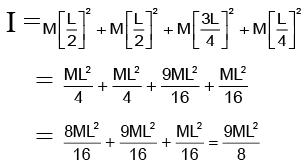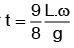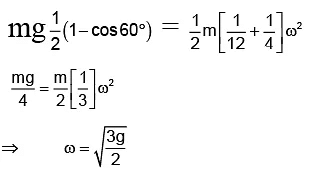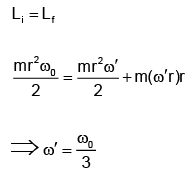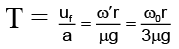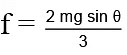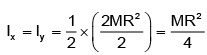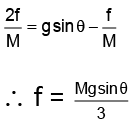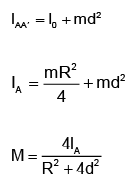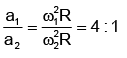JEE Advanced (Single Correct Type): Rotational Motion | Chapter-wise Tests for JEE Main & Advanced PDF Download

Q.1. A thin spherical shell of mass m and radius R rolls down a parabolic path PQR from a height H without slipping. Part PQ is sufficiently rough while part QR is smooth. If the height reached by the shell on the part QR be h, then h/H is given by (assume R << H)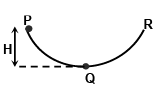
 (a) 1
(b) 0.75
(c) 0.6
(d) 0.5

Correct Answer is  option (c)

For pure rolling on part PQ, mechanical energy is conserved. Hence

For part QR, the translational K.E. = 1/2 mv² will BE converted  into the potential energy. Hence 1/2 mv² = mgh. Hence h = 3/5 H
Hence (C) is correct.

Q.2. Two particles each of mass M, are connected by a rigid rod of negligible mass and length L. The system is lying on a horizontal frictionless surface. An impulse Mv, perpendicular to the rod, is given at one end of the rod as shown in the figure. The angular velocity acquired by the rod is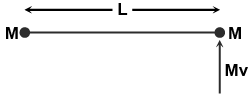
(a) 4v/L
(b) 2v/L
(c) v/L
(d) v/4L

Correct Answer is  option (c)
Apply COAM about centre of mass,

Hence (C) is correct.

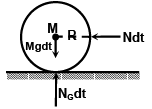
Q.3. A slender rod of mass M and length L hinged at O is kept horizontal and then released. The other end of the rod strikes a solid sphere of mass M and radius R (at point P) kept on a smooth horizontal surface. The points O and P are on the same vertical line. After the collision, the rod comes to rest. The angular speed of the sphere after the collision is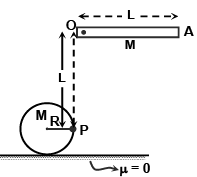
(a)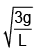
(b)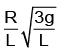
(c)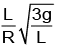
(d) zero

Correct Answer is  option (d)
Draw the F.B.D of sphere.

Since all the impulses acting on the sphere are passing through the centre of the sphere, the angular velocity remains zero.

Q.4. Four particles are attached to the ends of two massless rods. The rods are connected at point O as shown in the figure. The system can rotate about an axis perpendicular to the plane of the paper and passing through O. If, starting from rest under the action of a constant net external torque having magnitude MgL, the system rotates about that axis, then at what time will its angular speed become ω?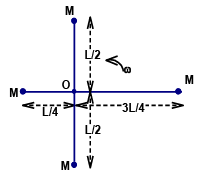
(a) 5ωL/8g
(b) 2ωL/3g
(c) 9ωL/8g
(d) 5ωL/3g

Correct Answer is  option (c)

Angular momentum = 9/8 ML²ω = MgL x t
⇒

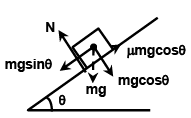
Q.5. When a body rolls without sliding up an inclined plane, the frictional force is
(a) directed up the plane
(b) directed down the plane
(c) zero
(d) dependent on its velocity

Correct Answer is  option (a)
Since, the body rolls without sliding,

tangential acceleration a_p = 0  & tangential speed v_p = 0
⇒ v - rω  = 0  &  a – r α = 0
v = rω & a = r α
Since the body's acceleration a is downwards parallel to the plane, therefore acceleration of the body must be in anticlockwise sense.
That means the friction must act up the plane to produce an anticlockwise torque to produce anticlockwise angular acceleration.
Hence (A) is correct.

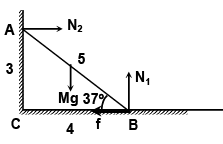
Q.6. A 5 m long pole of 3 kg mass is placed against a smooth vertical wall as shown in the figure. Under equilibrium condition, if the pole makes an angle of 37° with the horizontal, the frictional force between the pole and horizontal surface is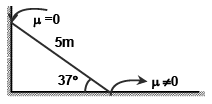
(a) 20 N
(b) 30 N
(c) 20 μN
(d) 30 μN

Correct Answer is  option (a)

Along horizontal f = N₂ . . . (1)

Finding torque about ‘B’
Mg(2) + N1(0) = f(0) + (N2) (3)
⇒ N₂ = 20

∴ From (1) f = 20 N

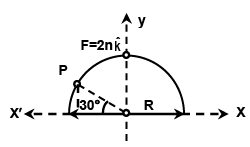
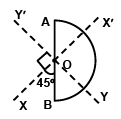
Q.7. A semi-circular disc of radius 1 m and mass = 4 kg is lying on the horizontal x-y plane. If XX' is the axis of rotation of the body and a force  = 2 N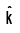is applied at point P, the magnitude of angular acceleration is equal to (the region is gravity free)
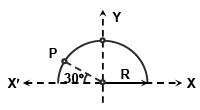(a) 2 rad/s²
(b) 3 rad/s²
(c) 1 rad/s²
(d) 4 rad/s²

Correct Answer is option (c)

I = MR²/4 = 4.1²/4 = 1 kg-m²
Torque = 2 N x 1/2 m = 1N-m
Angular acceleration = T/I = 1 rad/s²

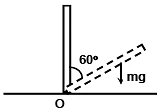
Q.8. A uniform meter stick of mass m is pivoted about a horizontal axis through its lower end O. Initially, it is held vertical and is allowed to fall freely. Its angular velocity at the instant when it makes an angle of 60° with the vertical is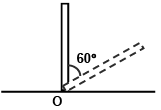
(a)
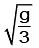
(b)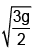
(c)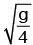
(d)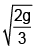

Correct Answer is option (b)

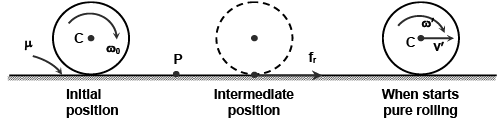
Q.9. A disc of radius r is rotating about its centre with an angular speed ω₀. It is gently placed on a rough horizontal surface. After what time it will be in pure rolling?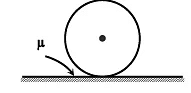
(a) w₀r/2μg
(b) w₀r/3μg
(c) w₀r/μg
(d) 3w₀r/2 μg

Correct Answer is option (b)
About point P, we can apply angular momentum theorem,


Apply v_i = 0 , u_i = w'R, a = μg, time = t

Hence (B) is correct.

Q.10. There is a uniform circular ring of mass m. A particle of mass m is placed at its centre and is connected to the rim by rigid light spokes. If this assembly is placed on a rough inclined surface, then frictional force acting on the assembly, if it undergoes pure rolling, is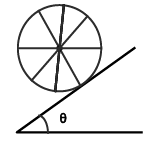
(a) 1/3 mg sin θ
(b) 2/3 mg sin θ
(c) 1/6 mg sin θ
(d) 1/2 mg sin θ

Correct Answer is option (b)

2mg sin θ- f = 2ma
fr = mr² a/r ⇒ f = ma
∴ 2mg sin θ - f = 2f
⇒

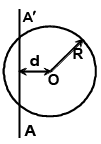
Q.11. A semicircular disc has mass m and radius R, as shown in the figure. The moment of inertia of the disc about an axis XX' in the plane of the disc passing through O (mid-point of the diameter) will be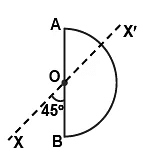
(a) MR²/2
(b) MR²/√2
(c) MR²/4
(d) none of these

Correct Answer is option (c)

Let I₁ and I₂ are MI about X-X' and Y-Y' axes respectively.
Due to symmetry,

Q.12. A block with a square base having area (A = axa) measuring axa, and height h is placed on an inclined plane. The coefficient of friction between the block and the inclined plane is μ. The angle of inclination θ of the plane is gradually increased. The block will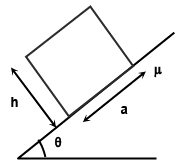
(a) topple before sliding if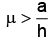
(b) topple before sliding if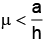
(c) slide before toppling if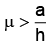
(d) none of these

Correct Answer is option (a)

It will topple before sliding if
mg sin θ x h/2 > mg cos θ x a/2
or tan θ > a/h
or μ > a/h

Q.13. Let I be the moment of inertia of a uniform square plate about an axis AB that passes though its centre and is parallel to two of its sides.  CD is a line in the plane of the plate that passes through the centre of the plate & makes an angle q with AB.  The moment of inertia of the plate about the axis CD is then equal to
(a) I
(b) I cos²θ
(c) I sin²θ
(d) I cos²(θ/2)

Correct Answer is option (a)

According to the perpendicular axis theorem
I_Z = I_x + I_y
Since the plate is quite  symmetrical about x' and y',  I_x' = I_y'
⇒ I_z = I_'x + I_'y =  2I_'x = 2I_'y

⇒ I_'x = I_'y = I_z/2
Similarly I_x = I_y = I_z/2
The required moment of inertia = I, where I_z/2 = I
Hence (A) is correct.

Q.14. A solid homogeneous sphere is moving on a rough horizontal surface, partly rolling and partly sliding.  During this kind of motion of this sphere
(a) total kinetic energy is conserved
(b) angular momentum of the sphere about the point of contact with the plane is conserved
(c) only the rotational kinetic energy about the centre of mass is conserved.
(d) angular momentum about the centre of mass is conserved.

Correct Answer is option (b)
When sphere partly rolls & partly slides, frictional loss is there. Therefore, total mechanical energy cannot be conserved.

Since, all the forces passes through the instantaneous point of contact, their torque about this point is zero
⇒ Angular momentum about that point (not about the mass centre) remains constant.

Q.15. A solid sphere is released from rest on a rough inclined plane as shown in the figure. A block is kept at rest at distance 1 m from the sphere as shown. The velocity with which the sphere strikes the block is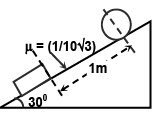
(a) 1 m/s
(b) 2 m/s
(c) 3 m/s
(d) none of these

Correct Answer is option (b)
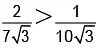
For rolling of sphere,  μ_min = 2/7 tan θ = 2/7 tan 30° =
Hence, the sphere slips on inclined plane.
a = g sin θ-μ g cosθ = 4.5 m/s²
v =  = 3 m/s.

Q.16. A sphere of mass M and radius R is moving on a rough fixed surface, having co-efficient of friction m as shown in figure. It will attain a minimum linear velocity after a time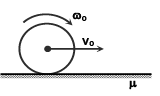
(a) v₀/μg
(b) ω₀R/μg
(c) (v₀-ω₀R)/ μg
(d) 2(v₀-ω₀R)/7μg

Correct Answer is option (d)
Min Linear velocity will be attained when pure rolling will start.
v(t) = ω(t) R
⇒ v_o- μgt = (ω₀ + αt)R
⇒ μmgR = 2/5 mR²α

⇒ α = 5μg/2R
⇒ v_o - μgt = ω_oR + 5μg/2Rt

⇒ t = 2(v_o - ω_oR)/7μg

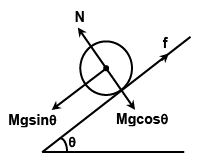
Q.17. A disc of mass ‘M’ and radius ‘R’ is gently placed on a sufficiently rough incline of inclination q with the horizontal and released. The frictional force acting on the ball is [Assume pure rolling of the disc.]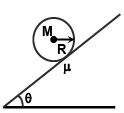
(a) μMg cosθ
(b) 12/3 Mgcos θ
(c) 1/3 Mgcos θ
(d) 1/3 Mg sin θ

Correct Answer is option (d)
rα = a_cm (for pure rolling)

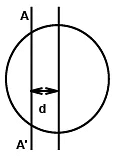
Q.18. Moment of inertia of a uniform circular disc of radius R about axis AA' is I_A. So, mass of the disc is

(a)
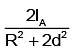
(b)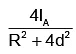
(c)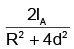
(d)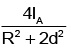

Correct Answer is option (b)

Q.19. A particle of mass m is moving with a uniform speed v in x - y plane along a straight line, y = x + k, the angular momentum of the particle about the origin is
(a) mvk/√2
(b) mvy/√2
(c) mvx/√2
(d) mvk

Correct Answer is option (a)
y = mx + c and y = x + k
⇒ θ = 45° and c = k
∴ Angular momentum, L = mv x k x 1/√2 = mvk/√2

Q.20. A disc and a ring having same mass M and radius R are rotating about their respective axis which are normal to their plane. If both have the same angular momentum, then the normal ratio of acceleration of the particles at the circumference of the disc to the ring is
(a) 1: 2
(b) 2: 1
(c) 1: 4
(d) 4: 1

Correct Answer is option (d)

Angular momentum, L = Iω
= MR²w₂ ⇒ ω₁ = 2ω₂