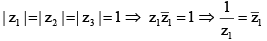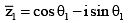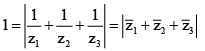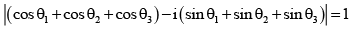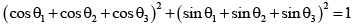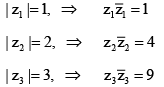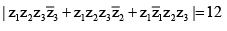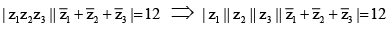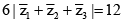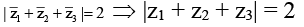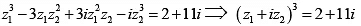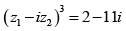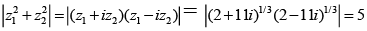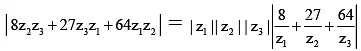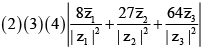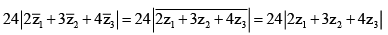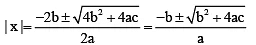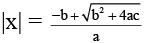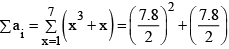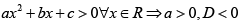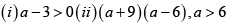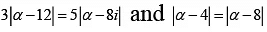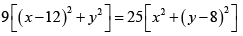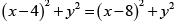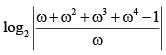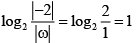Integer Answer Type Questions for JEE: Complex Numbers | Chapter-wise Tests for JEE Main & Advanced PDF Download

Clear all your JEE doubts with EduRev Join for Free

Join for Free

Q.1. If z₁, z₂, z₃ are complex numbers such that |z₁| = |z₂| = |z₃| = 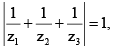 then |z₁ + z₂+ z₃| is 

Ans. 1

Put then
Now,
or

or,   | z₁ + z₂ + z₃ |= 1

Q.2. f |z₁| = 1, |z₂| = 2, |z₃| = 3 and |9z₁z₂ + 4z₁z₃ + z₂z₃| = 12, then the value of |z₁ + z₂ + z₃| is equal to

Ans. 2

|9z₁z₂ + 4z₁z₃ + z₂z₃| = 12
⇒
⇒
⇒
⇒

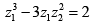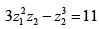Q.3. If z₁ , z₂ are complex numbers such that  and  then  =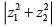

Ans. 5

Similarly,

Q.4. If |z₁| = 2, |z₂| = 3, |z₃| = 4 and | 2z₁ + 3z₂ + 4z₃ |= 4 , then absolute value of 8z₂z₃ + 27z₃z1+ 64z₁z₂ equals 

Ans. 96

=
=
= (24) (4) = 96

Q.5. If a, b, c are three distinct positive real numbers then the number of real roots of ax² + 2b|x| - c = 0 is

Ans. 2
 ∴
a, b, c are positive. So,
∴ x has two real values.

Q.6. The sum of all positive integral values of 'a ', a ∈ [1, 500] for which the equation [ x ]³ + x - a = 0 has solution is  ([.] denote G.I.F)

Ans. 812
a is integer then x must be integer, i.e.,  [x] = x
a = x³ + x


= 812

Q.7. If p, q ∈ {1, 2, 3, 4}, the number of equations of the form px² + qx + 1 = 0 having real roots is

Ans. 7
for the real roots D ≥ 0 ⇒ q² - 4p ≥ 0 ⇒ q² ≥ 4p
If  p = 1  then q² ≥ 4p ⇒ q² ≥ 4 ⇒ q = 2, 3, 4
If p = 2 then q² ≥ 4p ⇒ q² ≥ 8 ⇒ q = 3, 4
If p = 3 then q² ≥ 4p ⇒ q² ≥ 12 ⇒ q = 4
If p = 4 then q² ≥ 4p ⇒ q² ≥ 16 ⇒ q = 4.
∴ there are 7 real roots.

Q.8. The least integral value of ‘a’ such that (a - 3)x² + 12x + a + 6 > 0∀ x ∈ R is 

Ans. 7

⇒

Least integral value of a = 7

Q.9. If α satisfies 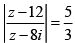 and 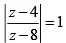 simultaneously, then the real part of α is _____

Ans. 6
Given that
Take a = x+ iy
∴
and
∴ x = 6, y = 8,17
∴ R_e (α )= 6

Q.10. If ω is fifth root of unity, then log₂| 1 + ω + ω² + ω³ - ω^-1| is equal to 

Ans. 1
Since ω = (1)^{1/ 5}  ∴ ω⁵ =1
and 1 + ω + ω² + ω³ + ω⁴ = 0, |ω| = 1

⇒