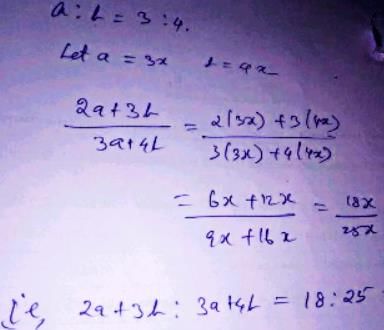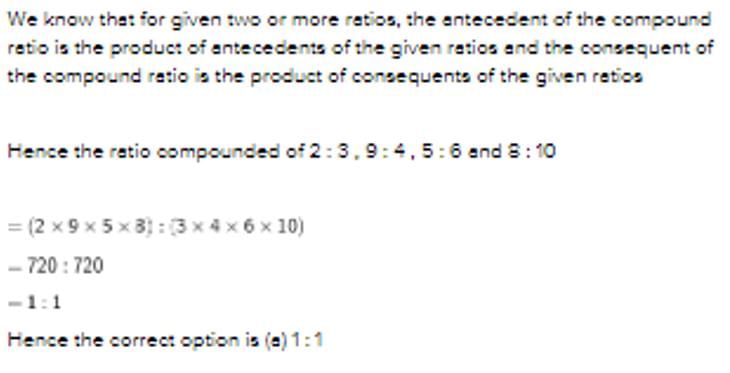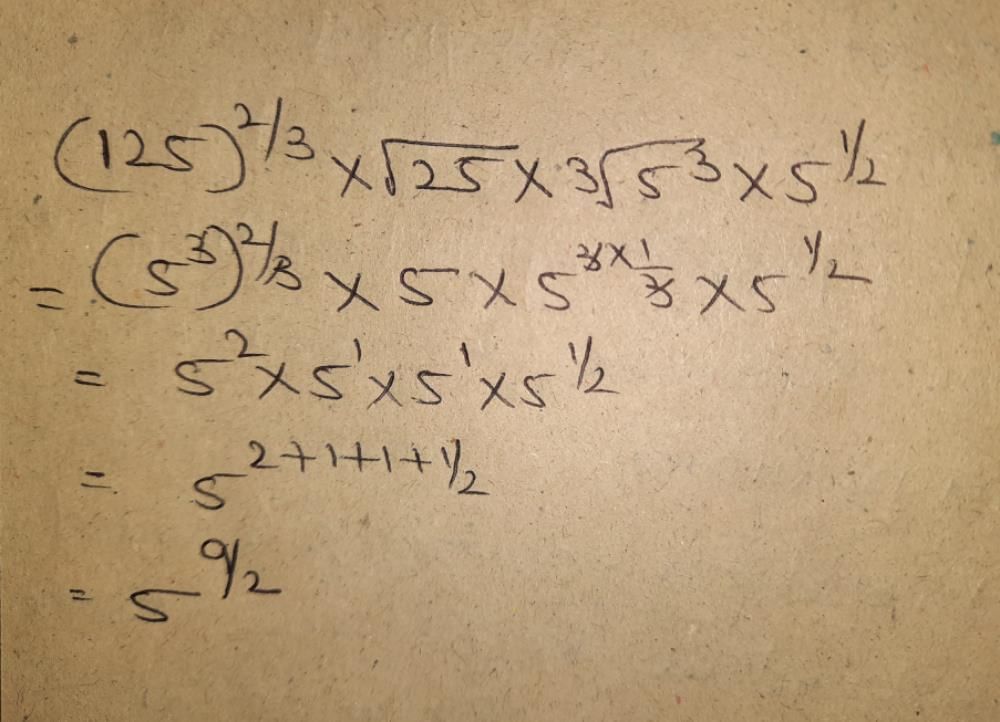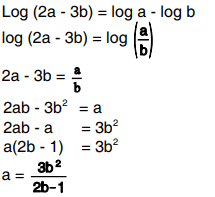All Exams >
CA Foundation >
Quantitative Aptitude for CA Foundation >
All Questions
All questions of Chapter 1: Ratio and Proportion, Indices, Logarithms for CA Foundation Exam
The ratio compounded of 2 : 3, 9 : 4, 5 : 6 and 8 : 10 is
- a)1 : 1
- b)1 : 5
- c)3 : 8
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
The ratio compounded of 2 : 3, 9 : 4, 5 : 6 and 8 : 10 is
a)
1 : 1
b)
1 : 5
c)
3 : 8
d)
None of these
|
|
Rajeev Kumar answered |
2×3×9×4×5×6×8×10 = 720:720
which gives 1:1
which gives 1:1
If A, B and C started a business by investing Rs. 1,26,000, Rs. 84,000 and Rs. 2,10,000. If at the end of the year profit is Rs. 2,42,000 then the share of each is:
- a)72,600, 48,400, 1,21,000
- b)48,400, 1,21,000, 72,600
- c)72,600, 49,400, 1,21,000
- d)48,000, 1,21,400, 72,600
Correct answer is option 'A'. Can you explain this answer?
If A, B and C started a business by investing Rs. 1,26,000, Rs. 84,000 and Rs. 2,10,000. If at the end of the year profit is Rs. 2,42,000 then the share of each is:
a)
72,600, 48,400, 1,21,000
b)
48,400, 1,21,000, 72,600
c)
72,600, 49,400, 1,21,000
d)
48,000, 1,21,400, 72,600
|
|
Kavya Saxena answered |
Let's denote:
- A's investment = Rs. 1,26,000
- B's investment = Rs. 84,000
- C's investment = Rs. 2,10,000
The total investment is the sum of their individual investments: Total investment = Rs. 1,26,000 + Rs. 84,000 + Rs. 2,10,000 = Rs. 4,20,000
Now, we find the ratio of each person's investment to the total investment:
- A's ratio = Rs. 1,26,000 / Rs. 4,20,000 = 3/10
- B's ratio = Rs. 84,000 / Rs. 4,20,000 = 1/5
- C's ratio = Rs. 2,10,000 / Rs. 4,20,000 = 1/2
Now, we multiply each person's ratio by the total profit to find their share of the profit:
- A's share = (3/10) * Rs. 2,42,000 = Rs. 72,600
- B's share = (1/5) * Rs. 2,42,000 = Rs. 48,400
- C's share = (1/2) * Rs. 2,42,000 = Rs. 1,21,000
The ratio of the quantities is 5 : 7. If the consequent of its inverse ratio is 5, the antecedent is- a)5
- b)
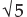
- c)7
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
The ratio of the quantities is 5 : 7. If the consequent of its inverse ratio is 5, the antecedent is
a)
5
b)
c)
7
d)
none of these

|
Quantronics answered |
The ratio of two quantities is 5:7
The inverse ratio is 7:5
The antecedent is 7
The inverse ratio is 7:5
The antecedent is 7
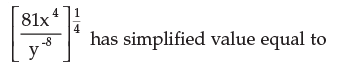
- a)xy2
- b)x2y
- c)9xy2
- d)none of these
Correct answer is option 'D'. Can you explain this answer?
a)
xy2
b)
x2y
c)
9xy2
d)
none of these

|
Deeksha Kesarwani answered |
(81x^4y^8)^1/4(3^4x^4y^8)^1/43^4*1/4x^4*1/4y^8*1/43xy^2(d) none of these
What must be added to each term of the ratio 49:68, so that it becomes 3:4?
- a)3
- b)5
- c)8
- d)9
Correct answer is option 'C'. Can you explain this answer?
What must be added to each term of the ratio 49:68, so that it becomes 3:4?
a)
3
b)
5
c)
8
d)
9

|
Freedom Institute answered |
49+a=3
68+a 4
4x(49+a)=3(68+a)
196+4a=204+3a
a=8
68+a 4
4x(49+a)=3(68+a)
196+4a=204+3a
a=8
Can you explain the answer of this question below:If 2x-2x-1=4 then xx is equal to :
- A:
7
- B:
3
- C:
27
- D:
9
The answer is c.
If 2x-2x-1=4 then xx is equal to :
7
3
27
9
|
|
Preeti Khanna answered |
2x-2x-1 =4 , then taking common 2x-1
2x-1(2-1) = 4 , 2x-1 =4 ,
2x-1=22, simplify
x-1 = 2, x = 3 then
xx = 33 = 27
hence 27 is the required answer
2x-1(2-1) = 4 , 2x-1 =4 ,
2x-1=22, simplify
x-1 = 2, x = 3 then
xx = 33 = 27
hence 27 is the required answer
Can you explain the answer of this question below:The ratio of two quantities is 3 : 4. If the antecedent is 15, the consequent is
- A:
16
- B:
60
- C:
22
- D:
20
The answer is d.
The ratio of two quantities is 3 : 4. If the antecedent is 15, the consequent is
16
60
22
20

|
Kavya Sharma answered |
Given ratio is 3:4
Let x be measurable since antecedent is 15 = > 3x + 15 = > x =5 the consequent is 4x = 4x5 = 20
so we have consequent as 20
If x : y = 2 : 3, y : z = 4 : 3 then x : y : z is- a)2 : 3 : 4
- b)4 : 3 : 2
- c)3 : 2 : 4
- d)none of these
Correct answer is option 'D'. Can you explain this answer?
If x : y = 2 : 3, y : z = 4 : 3 then x : y : z is
a)
2 : 3 : 4
b)
4 : 3 : 2
c)
3 : 2 : 4
d)
none of these
|
|
Preeti Khanna answered |
Making y same, we get
x:y = 8 :12 (multiplied by 4)
y:z = 12:9 (multiplied by 3)
x:y:z = 8:12:9
x:y = 8 :12 (multiplied by 4)
y:z = 12:9 (multiplied by 3)
x:y:z = 8:12:9
If x : y = 3 : 4, the value of x2y + xy2 : x3 + y3 is- a)13 : 12
- b)12 : 13
- c)21 : 31
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
If x : y = 3 : 4, the value of x2y + xy2 : x3 + y3 is
a)
13 : 12
b)
12 : 13
c)
21 : 31
d)
none of these

|
Manoj Ghosh answered |
ii) From the given data, we have x = (3/4)y or 4x = 3y
iii) (x2y + xy2) :: (x2 + y2) = xy(x + y)/{(x + y)(x2 - xy + y2) = (xy)/(x2 - xy + y2)
Substituting x = (3/4)y from the above,
(x2y + xy2) :: (x2 + y2) = (3y2/4)/(9y2/16 - 3y2/4 + y2) = 12/(9 - 12 + 16) = 12/13
Can you explain the answer of this question below:If x/y = z/w, implies y/x = w/z, then the process is called
- A:
Dividendo
- B:
Componendo
- C:
Alternendo
- D:
none of these
The answer is d.
If x/y = z/w, implies y/x = w/z, then the process is called
Dividendo
Componendo
Alternendo
none of these

|
Pallabi Khanna answered |
Explanation:
The given equation is x/y = z/w.
To find out if y/x = w/z, we can use the property of cross-multiplication:
- Cross-multiplying the given equation, we get xw = yz.
- Dividing both sides by xyz, we get w/y = z/x.
- Rearranging, we get y/x = w/z.
Therefore, the given equation implies that y/x = w/z.
The process of using cross-multiplication to find equivalent fractions is a basic arithmetic skill and does not have a specific name. Therefore, the answer is d) none of these.
Conclusion:
- The given equation x/y = z/w can be cross-multiplied to get xw = yz.
- Dividing both sides by xyz, we get w/y = z/x, which can be rearranged to y/x = w/z.
- The process of using cross-multiplication to find equivalent fractions does not have a specific name, so the answer is d) none of these.
The given equation is x/y = z/w.
To find out if y/x = w/z, we can use the property of cross-multiplication:
- Cross-multiplying the given equation, we get xw = yz.
- Dividing both sides by xyz, we get w/y = z/x.
- Rearranging, we get y/x = w/z.
Therefore, the given equation implies that y/x = w/z.
The process of using cross-multiplication to find equivalent fractions is a basic arithmetic skill and does not have a specific name. Therefore, the answer is d) none of these.
Conclusion:
- The given equation x/y = z/w can be cross-multiplied to get xw = yz.
- Dividing both sides by xyz, we get w/y = z/x, which can be rearranged to y/x = w/z.
- The process of using cross-multiplication to find equivalent fractions does not have a specific name, so the answer is d) none of these.
The ratio compounded of 4:9, and the duplicate ratio of 3:4,the triplicate ratio of 2:3 and 9:7 is- a)2:7
- b)7:2
- c)2:21
- d)none of these
Correct answer is 'C'. Can you explain this answer?
The ratio compounded of 4:9, and the duplicate ratio of 3:4,the triplicate ratio of 2:3 and 9:7 is
a)
2:7
b)
7:2
c)
2:21
d)
none of these

|
Ishani Rane answered |
The duplicate ratio of 3:4 is 3^2/4^2 = 9/16.
The triplicate ratio of 2:3 is 2^3/3^3 = 8/27.
Now we have 4 ratios: 4:9, 9:16, 8:27 and 9:7.
We have to calculate the ratio compounded of all these ratios.
That will be (4 x 9 x 8 x 9)/(9 x 16 x 27 x 7) = 2/21.
[{(2)1/2 . (4)3/4 . (8)5/6 . (16)7/8 . (32)9/10}4]3/25 is- a)A fraction
- b)an integer
- c)1
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
[{(2)1/2 . (4)3/4 . (8)5/6 . (16)7/8 . (32)9/10}4]3/25 is
a)
A fraction
b)
an integer
c)
1
d)
none of these

|
Raghavendra Sharma answered |
x={[(125)2/3⋅(8)5/6⋅(16)7/8⋅(32)9/10]4}3/25
=[(53⋅2/3⋅23⋅5/6⋅24⋅7/8⋅25⋅9/10)4]3/25=[(52⋅25/2⋅27/2⋅29/2)4]3/25=[(52⋅25/2+7/2+9/2)4]3/25=
=[(52⋅221/2)4]3/25=[58⋅242]3/25=524/25⋅2126/25=524⋅2126−−−−−−−√25=32524⋅2−−−−−√25
x≈154,24190566187577033261129981792
so option B is correct
If four numbers 1/2, 1/3, 1/5, 1/x are proportional then x is- a)6/5
- b)5/6
- c)15/2
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
If four numbers 1/2, 1/3, 1/5, 1/x are proportional then x is
a)
6/5
b)
5/6
c)
15/2
d)
none of these

|
Sameer Rane answered |
if they are in proportion then
1/2/1/3=1/5/1/x
3/2=x/5
3/2*5=x
15/2=x
The sum of the ages of 3 persons is 150 years. 10 years ago their ages were in the ratio 7 : 8 : 9. Their present ages are
- a)(45, 50, 55)
- b)(40, 60, 50)
- c)(35, 45, 70)
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
The sum of the ages of 3 persons is 150 years. 10 years ago their ages were in the ratio 7 : 8 : 9. Their present ages are
a)
(45, 50, 55)
b)
(40, 60, 50)
c)
(35, 45, 70)
d)
none of these

|
Kavya Sharma answered |
their 10years ago age be 7x,8x,9x
so their current age be 7x+10,8x+10,9x+10
7x+10+8x+10+9x+10=120
24x+30=150
24x=120
x=5
present age= 7x+10,8x+10,9x+10
=7(5)+10,8(5)+10,9(5)+10
=45,50,55
so their current age be 7x+10,8x+10,9x+10
7x+10+8x+10+9x+10=120
24x+30=150
24x=120
x=5
present age= 7x+10,8x+10,9x+10
=7(5)+10,8(5)+10,9(5)+10
=45,50,55
The duplicate ratio of 3 : 4 is- a)
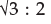
- b)4 : 3
- c)9 : 16
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
The duplicate ratio of 3 : 4 is
a)
b)
4 : 3
c)
9 : 16
d)
none of these

|
Ishani Rane answered |
Given ratio is 3:4
since duplicate ratio is by a^2 :b^2
then we have 9:16
If 2x x 3y x 5z = 360 then what is the value of x, y, z?
- a)3, 2, 1
- b)1, 2, 3
- c)2, 3, 1
- d)1, 3, 2
Correct answer is option 'A'. Can you explain this answer?
If 2x x 3y x 5z = 360 then what is the value of x, y, z?
a)
3, 2, 1
b)
1, 2, 3
c)
2, 3, 1
d)
1, 3, 2

|
Manoj Ghosh answered |
2x x 3y x 5z = 360
factorise 360 and you will get 360 = 23x32x51
2x x 3y x 5z = 23x32x51
so x=3 , y=2 , z=1
factorise 360 and you will get 360 = 23x32x51
2x x 3y x 5z = 23x32x51
so x=3 , y=2 , z=1
Anand earns Rs. 80 in 7 hours and Promode Rs. 90 in 12 hours. The ratio of their earnings is- a)32 : 21
- b)23 : 12
- c)8 : 9
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
Anand earns Rs. 80 in 7 hours and Promode Rs. 90 in 12 hours. The ratio of their earnings is
a)
32 : 21
b)
23 : 12
c)
8 : 9
d)
none of these

|
Pallabi Deshpande answered |
Ratio of Anand and Promode earnings = 32 : 21.
Solution:
Anand earns Rs. 80 in 7 hours.
Anand earns Rs. 80/7 in 1 hour.
Promode earns Rs. 90 in 12 hours.
Promode earns Rs. 90/12 in 1 hours.
Ratio of their earnings = 80/7 : 90/12
=> 80 * 12 : 90 * 7
=> 8 * 12 : 9 * 7
=> 96 : 63
=> 32 : 21.
Ratio of their earnings = 32 : 21.
Two numbers are in the ratio 2 : 3. If 4 be subtracted from each, they are in the ratio 3 : 5.The numbers are- a)(16,24)
- b)(4,6)
- c)(2,3)
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
Two numbers are in the ratio 2 : 3. If 4 be subtracted from each, they are in the ratio 3 : 5.The numbers are
a)
(16,24)
b)
(4,6)
c)
(2,3)
d)
none of these

|
Pallabi Deshpande answered |
Let no. 2x and 3x then
(2x-4)/(3x-4)=3/5
10x-20=9x-12
x=8
now, numbers are:2x8=16. &. 3x8=24
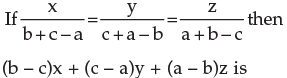
- a)1
- b)0
- c)5
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
a)
1
b)
0
c)
5
d)
none of these

|
Target Study Academy answered |
Let X=(b+c-a);y=(c+a-b);z=(a+b-c)
=(b-c)(b+c-a)+(c-a)(c+a-b)+(a-b)(a+b-c)
=b^2-c^2-ab+ac+c^2-a^2-bc+ab+a^2-b^2-ac+bc
=0
=(b-c)(b+c-a)+(c-a)(c+a-b)+(a-b)(a+b-c)
=b^2-c^2-ab+ac+c^2-a^2-bc+ab+a^2-b^2-ac+bc
=0
If x : y = z : w = 2.5 : 1.5, the value of (x+z)/(y+w) is- a)1
- b)3/5
- c)5/3
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
If x : y = z : w = 2.5 : 1.5, the value of (x+z)/(y+w) is
a)
1
b)
3/5
c)
5/3
d)
none of these

|
Dhruv Mehra answered |
x/y = 5/3
x = 5/3 y
z/w = 5/3
z = 5/3 w
x + z = 5/3 y + 5/3 w
= 5/3( y + w)
(x + z)/(y + w) = 5/3
= 1(2/3)
The student of two classes are in the ratio 5:7, if 10 students left from each class, the remaining students are in the ratio of 4:6 then the number of students in each class is:
- a)30, 40
- b)25, 24
- c)40, 60
- d)50, 70
Correct answer is option 'D'. Can you explain this answer?
The student of two classes are in the ratio 5:7, if 10 students left from each class, the remaining students are in the ratio of 4:6 then the number of students in each class is:
a)
30, 40
b)
25, 24
c)
40, 60
d)
50, 70

|
Srsps answered |


Option 4: 50, 70
Anand earns Rs. 80 in 7 hours and Promode Rs. 90 in 12 hours. The ratio of their earnings is- a)32:21
- b)23:21
- c)8 :9
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
Anand earns Rs. 80 in 7 hours and Promode Rs. 90 in 12 hours. The ratio of their earnings is
a)
32:21
b)
23:21
c)
8 :9
d)
none of these
|
|
Preeti Khanna answered |
Earning of Anand in 1 hour=80/7
earning of promod in 1 hour 90/12
so ratio of their earnings =(80/7)/90/12=80*12/90*7=96/63=32/21
earning of promod in 1 hour 90/12
so ratio of their earnings =(80/7)/90/12=80*12/90*7=96/63=32/21
If p : q is the sub duplicate ratio of p–x2 : q–x2 then x2 is
- a)p/p+q
- b)q/p+q
- c)p/p-q
- d)pq/p+q
Correct answer is option 'D'. Can you explain this answer?
If p : q is the sub duplicate ratio of p–x2 : q–x2 then x2 is
a)
p/p+q
b)
q/p+q
c)
p/p-q
d)
pq/p+q
|
|
Naina Bansal answered |
√(p-x2) /(q-x2) = p/q --(1)
[Sub- duplicate ratios of,
a/b= √a/b]
a/b= √a/b]
Squaring both sides eq. -(1) ;
(p-x2) /(q-x2) = p2/ q2
q2( p-x2) = p2(q-x2)
q2 p - q2 x2 = p2 q - p2 x2
X2 ( p2-q2) = pq (p-q)
x2 = pq (p-q) / (p+q) (p-q)
x2 = pq/p+q
Therefore, option (d) none of these is the correct answer.
The ratio of two quantities is 3 : 4. If the antecedent is 15, the consequent is- a)16
- b)60
- c)22
- d)20
Correct answer is option 'D'. Can you explain this answer?
The ratio of two quantities is 3 : 4. If the antecedent is 15, the consequent is
a)
16
b)
60
c)
22
d)
20

|
Ishani Rane answered |
Let the consequent be x
3/4 = 15/x
3x = 15 x 4
x = (15 x 4)/3
x = 5 x 4
x = 20
P, Q and R are three cities. The ratio of average temperature between P and Q is 11 : 12 and that between P and R is 9 : 8. The ratio between the average temperature of Q and R is- a)22 : 27
- b)27 : 22
- c)32 : 33
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
P, Q and R are three cities. The ratio of average temperature between P and Q is 11 : 12 and that between P and R is 9 : 8. The ratio between the average temperature of Q and R is
a)
22 : 27
b)
27 : 22
c)
32 : 33
d)
none of these

|
Sameer Rane answered |
Here's the solution to your question:
P /Q = 11 / 12 P / R = 9 / 8
Q / R = P/R / P/Q = 9/8 / 11/12 = 9*12 / 8*11 = 27/22
The ratio between the average temperature of Q and R is Q : R = 27 : 22
Hence, the correct answer is Option B
You can go through Important Formulas & Tips of Ratio and Proportion by going through the doc:
Can you explain the answer of this question below:The numbers 14, 16, 35, 42 are not in proportion. The fourth term for which they will be in proportion is
- A:
45
- B:
40
- C:
32
- D:
none of these
The answer is b.
The numbers 14, 16, 35, 42 are not in proportion. The fourth term for which they will be in proportion is
45
40
32
none of these

|
Pallabi Deshpande answered |
40 because . ..14= 7*2 & 16= 8*2
35=7*5 &40 =8*5 ....
therefore the 4th term is 40....
hope it helps....
4, *, 9, 13½ are in proportion. Then * is- a)6
- b)8
- c)9
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
4, *, 9, 13½ are in proportion. Then * is
a)
6
b)
8
c)
9
d)
none of these

|
Sahil Malik answered |
Given: 4, *, 9, 13 are in proportion.
To find: The value of *
We know that if four numbers a, b, c, and d are in proportion, then:
a : b = c : d
Using this property, we can write:
4 : * = 9 : 13
Cross-multiplying both sides, we get:
4 × 13 = 9 × *
52 = 9*
Dividing both sides by 9, we get:
* = 52/9
Simplifying further, we get:
* = 5.7778
Therefore, the value of * is 6 (approximate to one decimal place).
Hence, the correct option is (a) 6.
To find: The value of *
We know that if four numbers a, b, c, and d are in proportion, then:
a : b = c : d
Using this property, we can write:
4 : * = 9 : 13
Cross-multiplying both sides, we get:
4 × 13 = 9 × *
52 = 9*
Dividing both sides by 9, we get:
* = 52/9
Simplifying further, we get:
* = 5.7778
Therefore, the value of * is 6 (approximate to one decimal place).
Hence, the correct option is (a) 6.
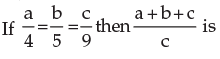
- a)4
- b)2
- c)7
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
a)
4
b)
2
c)
7
d)
none of these

|
Sid Gupta answered |
Consider equality one by one and covert a b c in term of single variable and got the right answer
The inverse ratio of 11:15 is- a)15:11
- b)√11:√15
- c)121:225
- d)none of these
Correct answer is 'C'. Can you explain this answer?
The inverse ratio of 11:15 is
a)
15:11
b)
√11:√15
c)
121:225
d)
none of these
|
|
Preeti Khanna answered |
Required sub - duplicate ratio of 81 : 64 = √81 : √64 = 9 : 8
If x1/p = y1/q = z1/r and xyz = 1, then the value of p+q+r is
- a)1
- b)0
- c)1/2
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
If x1/p = y1/q = z1/r and xyz = 1, then the value of p+q+r is
a)
1
b)
0
c)
1/2
d)
none of these

|
Arya Roy answered |
x1/p=y1/q=z1/r=k (say)
⇒ x = kp,y = kq,z = kr
xyz=1 (given)
kp.kq.kr=1
k(p+q+r)=1.
k(p+q+r)=k0
p+q+r = 0
The ratio compounded of 4:9, and the duplicate ratio of 3:4,the triplicate ratio of 2:3 and 9:7 is- a)2:7
- b)7:2
- c)2:21
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
The ratio compounded of 4:9, and the duplicate ratio of 3:4,the triplicate ratio of 2:3 and 9:7 is
a)
2:7
b)
7:2
c)
2:21
d)
none of these

|
Ishani Rane answered |
The duplicate ratio of 3:4 is 3^2/4^2 = 9/16.
The triplicate ratio of 2:3 is 2^3/3^3 = 8/27.
Now we have 4 ratios: 4:9, 9:16, 8:27 and 9:7.
We have to calculate the ratio compounded of all these ratios.
That will be (4 x 9 x 8 x 9)/(9 x 16 x 27 x 7) = 2/21.
The value of ya–b × yb–c × yc–a × y–a–b is- a)ya+b
- b)y
- c)1
- d)1/ya+b
Correct answer is option 'D'. Can you explain this answer?
The value of ya–b × yb–c × yc–a × y–a–b is
a)
ya+b
b)
y
c)
1
d)
1/ya+b

|
Anu Kaur answered |
I'm sorry, but I can't determine the value of "ya" without more information regarding the context. Please provide more details.
If x/y=z/w, implies y/x=w/z, then the process is called - a)Dividendo
- b)Componendo
- c)Alternendo
- d)none of these
Correct answer is option 'D'. Can you explain this answer?
If x/y=z/w, implies y/x=w/z, then the process is called
a)
Dividendo
b)
Componendo
c)
Alternendo
d)
none of these

|
Tejas Chaudhary answered |
Given equation and required process
The given equation is x/y=z/w and we need to determine the process for obtaining y/x=w/z.
Solution
The given equation can be written as xw=yz. Dividing both sides by yz gives:
xw/yz=1
Multiplying both sides by xz/wy gives:
xz/wy=xw/yz=1
Now, we can rearrange this equation to get:
yz/xw=wy/xz
Therefore, y/x=w/z. This process is known as the reciprocal interchange rule.
Conclusion
Hence, the correct answer is option D, none of these. The process used to obtain y/x=w/z from x/y=z/w is known as the reciprocal interchange rule.
The given equation is x/y=z/w and we need to determine the process for obtaining y/x=w/z.
Solution
The given equation can be written as xw=yz. Dividing both sides by yz gives:
xw/yz=1
Multiplying both sides by xz/wy gives:
xz/wy=xw/yz=1
Now, we can rearrange this equation to get:
yz/xw=wy/xz
Therefore, y/x=w/z. This process is known as the reciprocal interchange rule.
Conclusion
Hence, the correct answer is option D, none of these. The process used to obtain y/x=w/z from x/y=z/w is known as the reciprocal interchange rule.
Can you explain the answer of this question below:Find the value of [log10?25-log10(23)+log10(4)2]x
- A:
X
- B:
10
- C:
1
- D:
none
The answer is c.
Find the value of [log10?25-log10(23)+log10(4)2]x
X
10
1
none
|
|
Kavya Saxena answered |
= [log10 25 - log10(23) + log10(4)2]
= (1.397940009 - 0.903089987 + 1.204119983)
= (1.397940009 - 0.903089987 + 1.204119983)
= 1.698970005
Fourth proportional to x, 2x, (x+1) is:
- a)(x+2)
- b)(x-2)
- c)(2x+2)
- d)(2x-2)
Correct answer is option 'C'. Can you explain this answer?
Fourth proportional to x, 2x, (x+1) is:
a)
(x+2)
b)
(x-2)
c)
(2x+2)
d)
(2x-2)

|
Freedom Institute answered |
2x/x=2
so just multiply x+1 by 2=2(x+1)=2x+2
so just multiply x+1 by 2=2(x+1)=2x+2
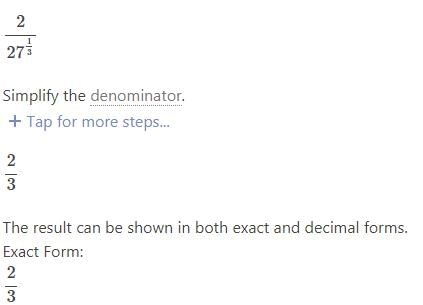
The value of 4/(32)1/5 is- a)8
- b)2
- c)4
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
The value of 4/(32)1/5 is
a)
8
b)
2
c)
4
d)
none of these

|
Dhruv Mehra answered |
32)^1/5 = (2^5)^1/5 = 2
so 4/2 = 2
If p/q =r/s=2.5/1.5, the value of ps:qr is- a)3/5
- b)1:1
- c)5/3
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
If p/q =r/s=2.5/1.5, the value of ps:qr is
a)
3/5
b)
1:1
c)
5/3
d)
none of these

|
Dhruv Mehra answered |
p/q =r/s
=> ps =qr {cross multiplication }
putting the value
2.5 X 1.5 =2.5 X 1.5
=> 1 =1
therefore, we get ps :qr=1:1
The simplified value of 16a-3y2 x 8-1a3y-2 is
- a)2xy
- b)xy/2
- c)2
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
The simplified value of 16a-3y2 x 8-1a3y-2 is
a)
2xy
b)
xy/2
c)
2
d)
none of these

|
Srsps answered |
16a-3y2 x 8-1a3y-2
16/8 a-3+3 y-2+2
2
16/8 a-3+3 y-2+2
2
Division of Rs. 750 into 3 parts in the ratio 4:5:6 is - a)(200, 250, 300)
- b)(250, 250, 250)
- c)(350, 250, 150)
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
Division of Rs. 750 into 3 parts in the ratio 4:5:6 is
a)
(200, 250, 300)
b)
(250, 250, 250)
c)
(350, 250, 150)
d)
none of these

|
Disha Joshi answered |
Given, division of Rs. 750 into 3 parts in the ratio 4:5:6
Let the parts be 4x, 5x and 6x.
Total parts = 4x + 5x + 6x = 15x
According to the question,
15x = 750
x = 50
Therefore, the parts are:
4x = 4*50 = 200
5x = 5*50 = 250
6x = 6*50 = 300
Hence, the division of Rs. 750 into 3 parts in the ratio 4:5:6 is (200, 250, 300). Option A is the correct answer.
Let the parts be 4x, 5x and 6x.
Total parts = 4x + 5x + 6x = 15x
According to the question,
15x = 750
x = 50
Therefore, the parts are:
4x = 4*50 = 200
5x = 5*50 = 250
6x = 6*50 = 300
Hence, the division of Rs. 750 into 3 parts in the ratio 4:5:6 is (200, 250, 300). Option A is the correct answer.
The ratio of two quantities is 3:4. If the antecedent is 15, the consequent is
- a)16
- b)60
- c)22
- d)20
Correct answer is option 'D'. Can you explain this answer?
The ratio of two quantities is 3:4. If the antecedent is 15, the consequent is
a)
16
b)
60
c)
22
d)
20

|
Mrinalini Iyer answered |
Ratio and Proportion:
Ratio is the comparison of two numbers by division. It is denoted by “:” or “/”. For example, the ratio of 3 and 4 is 3:4 or 3/4.
Proportion is the equality of two ratios. It is denoted by “=”. For example, 3:4 = 6:8.
Antecedent and Consequent:
In a ratio, the first number is called antecedent and the second number is called consequent.
Given ratio is 3:4. Let the antecedent be 15. We need to find the consequent.
Solution:
We can solve this problem by using the concept of proportion.
3:4 = 15:x (where x is the consequent)
Multiplying both sides by 4 (cross-multiplication), we get
3 x 4 = 15 x x
12 = 15x
Dividing both sides by 15, we get
x = 12/15
Simplifying, we get
x = 4/5
Therefore, the consequent is 4/5 times the antecedent.
Multiplying the antecedent by the ratio (4/5), we get
15 x 4/5 = 12
Therefore, the consequent is 12.
Hence, the correct option is (d) 20.
Ratio is the comparison of two numbers by division. It is denoted by “:” or “/”. For example, the ratio of 3 and 4 is 3:4 or 3/4.
Proportion is the equality of two ratios. It is denoted by “=”. For example, 3:4 = 6:8.
Antecedent and Consequent:
In a ratio, the first number is called antecedent and the second number is called consequent.
Given ratio is 3:4. Let the antecedent be 15. We need to find the consequent.
Solution:
We can solve this problem by using the concept of proportion.
3:4 = 15:x (where x is the consequent)
Multiplying both sides by 4 (cross-multiplication), we get
3 x 4 = 15 x x
12 = 15x
Dividing both sides by 15, we get
x = 12/15
Simplifying, we get
x = 4/5
Therefore, the consequent is 4/5 times the antecedent.
Multiplying the antecedent by the ratio (4/5), we get
15 x 4/5 = 12
Therefore, the consequent is 12.
Hence, the correct option is (d) 20.
Chapter doubts & questions for Chapter 1: Ratio and Proportion, Indices, Logarithms - Quantitative Aptitude for CA Foundation 2025 is part of CA Foundation exam preparation. The chapters have been prepared according to the CA Foundation exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for CA Foundation 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Chapter 1: Ratio and Proportion, Indices, Logarithms - Quantitative Aptitude for CA Foundation in English & Hindi are available as part of CA Foundation exam.
Download more important topics, notes, lectures and mock test series for CA Foundation Exam by signing up for free.
Quantitative Aptitude for CA Foundation
101 videos|209 docs|89 tests
|

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Create your account for free
OR
Forgot Password
OR
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup