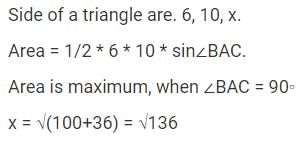All Exams >
UPSC >
CSAT Preparation >
All Questions
All questions of Plane Geometry for UPSC CSE Exam
A rectangular enclosure 40 m x 36 m has a horse tethered to a corner with a rope of 14 m in length. What is the ratio of the respective areas it can graze, if it is outside the enclosure and if it is inside the enclosure?- a)2
- b)2:3
- c)1:4
- d)3:1
Correct answer is option 'D'. Can you explain this answer?
A rectangular enclosure 40 m x 36 m has a horse tethered to a corner with a rope of 14 m in length. What is the ratio of the respective areas it can graze, if it is outside the enclosure and if it is inside the enclosure?
a)
2
b)
2:3
c)
1:4
d)
3:1

|
Talent Skill Learning answered |
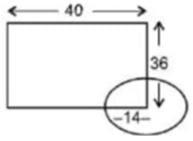
- Imagine a circle on the corner of the rectangle.
- 3 quarters of the circle lie outside the rectangle and 1 quarter lies inside.
- Hence, Required ratio = 3 : 1
Four horses are tethered at four comers of a square plot of side 14 m so that the adjacent horses can just reach one another. There is a small circular pond of area 20 m2 at the centre. Find the ungrazed area.- a)42 m2
- b)22 m2
- c)84 m2
- d)168 m2
Correct answer is option 'B'. Can you explain this answer?
Four horses are tethered at four comers of a square plot of side 14 m so that the adjacent horses can just reach one another. There is a small circular pond of area 20 m2 at the centre. Find the ungrazed area.
a)
42 m2
b)
22 m2
c)
84 m2
d)
168 m2
|
|
Preeti Khanna answered |
Total area of plot = 14 * 14 = 196m2
Horses can graze in quarter circle of radius = 7m
Grazed area = 4 * (pie r2)/4 = 154 m2
Area of plot when horses cannot reach = (196 - 154) = 42m2
Ungrazed area = 42 - 20 = 22m2
Horses can graze in quarter circle of radius = 7m
Grazed area = 4 * (pie r2)/4 = 154 m2
Area of plot when horses cannot reach = (196 - 154) = 42m2
Ungrazed area = 42 - 20 = 22m2
A ball of diameter 15 cm is floating so that the top of the ball is 5 cm above the smooth surface (water) of the pond. What is the circumference in centimeters of the circle formed by the contact of the water surface with the ball?- a)10√2π
- b)50
- c)10 p
- d)5√2π
Correct answer is option 'A'. Can you explain this answer?
A ball of diameter 15 cm is floating so that the top of the ball is 5 cm above the smooth surface (water) of the pond. What is the circumference in centimeters of the circle formed by the contact of the water surface with the ball?
a)
10√2π
b)
50
c)
10 p
d)
5√2π

|
Priyanka Datta answered |
In the given figure, AB and CD are two chords of a circle intersecting at O. If AO = 4 cm and OB = 6 cm and OC = 3 cm. Find OD.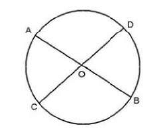
- a)4 cm
- b)6 cm
- c)8 cm
- d)10 cm
Correct answer is option 'C'. Can you explain this answer?
In the given figure, AB and CD are two chords of a circle intersecting at O. If AO = 4 cm and OB = 6 cm and OC = 3 cm. Find OD.
a)
4 cm
b)
6 cm
c)
8 cm
d)
10 cm

|
Pathways Academy answered |
By the rule of chords, cutting externally, we get:
(9 + 6) * 6 = (5 + x) * 5
90 = 25 + 5x
5x = 65
x = 13 cm
90 = 25 + 5x
5x = 65
x = 13 cm
A cyclic quadrilateral is such that two of its adjacent angles are divisible by 6 and 10 respectively. One of the remaining angles will necessarily be divisible by:
- a)3
- b)4
- c)8
- d)None of these
Correct answer is option 'D'. Can you explain this answer?
A cyclic quadrilateral is such that two of its adjacent angles are divisible by 6 and 10 respectively. One of the remaining angles will necessarily be divisible by:
a)
3
b)
4
c)
8
d)
None of these
|
|
Palak Bose answered |
We know that the sum of the opposite angles of a cyclic quadrilateral is 180 degrees. Let the four angles be A, B, C, and D, with A and B being the angles divisible by 6 and 10, respectively.
Since A is divisible by 6 and B is divisible by 10, we know that A = 6m and B = 10n for some integers m and n.
Now, consider the opposite angles. Since the sum of opposite angles is 180 degrees, we have:
C = 180 - B = 180 - 10n
D = 180 - A = 180 - 6m
We want to find which of the given options the angles C or D are necessarily divisible by. Let's examine each option:
1. 3: Since B is divisible by 10, it is possible that B is divisible by 5 but not 3 (e.g. B = 10). In this case, C = 180 - B would not be divisible by 3. Also, A is divisible by 6, so A is always divisible by 3, which means D = 180 - A would never be divisible by 3. So, this option is incorrect.
2. 4: Since A is divisible by 6, it is possible that A is divisible by 2 but not 4 (e.g. A = 6). In this case, D = 180 - A would not be divisible by 4. Also, B is divisible by 10, so B is always divisible by 2, which means C = 180 - B would never be divisible by 4. So, this option is also incorrect.
3. 8: If A is divisible by 6, then it can be even or odd multiples of 6 (e.g. A = 6, 12, 18, ...). D will be 180 - A, which means D can be both even and odd (e.g. D = 180 - 6 = 174, D = 180 - 12 = 168, D = 180 - 18 = 162, ...). Since D can be both even and odd, it is not necessarily divisible by 8. Similarly, C can also be both even and odd, so it is not necessarily divisible by 8. Thus, this option is also incorrect.
4. None of these: Since none of the previous options work, the correct answer is None of these.
So, the correct answer is option 4: None of these.
Since A is divisible by 6 and B is divisible by 10, we know that A = 6m and B = 10n for some integers m and n.
Now, consider the opposite angles. Since the sum of opposite angles is 180 degrees, we have:
C = 180 - B = 180 - 10n
D = 180 - A = 180 - 6m
We want to find which of the given options the angles C or D are necessarily divisible by. Let's examine each option:
1. 3: Since B is divisible by 10, it is possible that B is divisible by 5 but not 3 (e.g. B = 10). In this case, C = 180 - B would not be divisible by 3. Also, A is divisible by 6, so A is always divisible by 3, which means D = 180 - A would never be divisible by 3. So, this option is incorrect.
2. 4: Since A is divisible by 6, it is possible that A is divisible by 2 but not 4 (e.g. A = 6). In this case, D = 180 - A would not be divisible by 4. Also, B is divisible by 10, so B is always divisible by 2, which means C = 180 - B would never be divisible by 4. So, this option is also incorrect.
3. 8: If A is divisible by 6, then it can be even or odd multiples of 6 (e.g. A = 6, 12, 18, ...). D will be 180 - A, which means D can be both even and odd (e.g. D = 180 - 6 = 174, D = 180 - 12 = 168, D = 180 - 18 = 162, ...). Since D can be both even and odd, it is not necessarily divisible by 8. Similarly, C can also be both even and odd, so it is not necessarily divisible by 8. Thus, this option is also incorrect.
4. None of these: Since none of the previous options work, the correct answer is None of these.
So, the correct answer is option 4: None of these.
The ratio of the sides of Δ ABC is 1:2:4. What is the ratio of the altitudes drawn onto these sides?- a)4:2:1
- b)1:2:4
- c)1:4:16
- d)None of these
Correct answer is option 'D'. Can you explain this answer?
The ratio of the sides of Δ ABC is 1:2:4. What is the ratio of the altitudes drawn onto these sides?
a)
4:2:1
b)
1:2:4
c)
1:4:16
d)
None of these
|
|
Aakash Giery answered |
Sum of any two sides should be greater than third side.
here 1+2=3 is not less than 4 ,
1+2<4 ,so="" triangle="" is="" not="" possible.="" ,so="" triangle="" is="" not="">
here 1+2=3 is not less than 4 ,
1+2<4 ,so="" triangle="" is="" not="" possible.="" ,so="" triangle="" is="" not="">
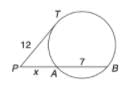
Find the value of x in the given figure.
- a)16 cm
- b)7 cm
- c)12 cm
- d)9 cm
Correct answer is option 'D'. Can you explain this answer?
Find the value of x in the given figure.

a)
16 cm
b)
7 cm
c)
12 cm
d)
9 cm

|
Pooja Sen answered |
Isosceles trapezium is always cyclic The sum of opposite angles of a cyclic quadrilateral is 180°
Read the passage below and solve the questions based on it The area of a square is equal lo the area of a rectangle. Moreover, the perimeter of the square is also equal to the perimeter of the rectangle,Q.The length of the rectangle is equal to the:a)Breadth of the rectangleb)Side of the squarec)Cannot be determinedd)Both [1] and [2]Correct answer is option 'D'. Can you explain this answer?
|
|
Nikita Singh answered |
This is possible only when both the length and breadth of the rectangle are equal to the side of the square.
A chord of length 32 cm is placed inside a circle of radius 20 cm and a point whose distance from the centre of the circle is 13 cm, is marked on the chord. Calculate the lengths of the segment of the chord.- a)21 cm and 11 cm
- b)19 cm and 13 cm
- c)16 cm each
- d)18 cm and 14 cm
Correct answer is option 'A'. Can you explain this answer?
A chord of length 32 cm is placed inside a circle of radius 20 cm and a point whose distance from the centre of the circle is 13 cm, is marked on the chord. Calculate the lengths of the segment of the chord.
a)
21 cm and 11 cm
b)
19 cm and 13 cm
c)
16 cm each
d)
18 cm and 14 cm

|
Arya Mehta answered |
AQ = (16 + 5 = 21) cm and QB = 32 - 21 = 11 cm
The volume of two spheres are in the ratio 27 : 125. The ratio of their surface area is?- a)25 : 9
- b)27 : 11
- c)11 : 27
- d)9 : 25
Correct answer is option 'D'. Can you explain this answer?
The volume of two spheres are in the ratio 27 : 125. The ratio of their surface area is?
a)
25 : 9
b)
27 : 11
c)
11 : 27
d)
9 : 25
|
|
Sagar Sharma answered |
Understanding the Volume and Surface Area of Spheres
The problem states that the volumes of two spheres are in the ratio 27:125. To find the ratio of their surface areas, we need to understand the relationships between the two.
Volume of a Sphere
- The formula for the volume (V) of a sphere is given by V = (4/3)πr^3, where r is the radius.
- If the volumes of two spheres are in the ratio 27:125, we can express this as:
- V1/V2 = 27/125
Finding the Ratio of Radii
- Since volumes are proportional to the cube of the radii, we have:
- (r1^3)/(r2^3) = 27/125
- Taking the cube root on both sides gives us:
- r1/r2 = (27^(1/3))/(125^(1/3)) = 3/5
Surface Area of a Sphere
- The formula for the surface area (A) of a sphere is A = 4πr^2.
- Now, to find the ratio of the surface areas of the two spheres, we have:
- A1/A2 = (4πr1^2)/(4πr2^2) = (r1^2)/(r2^2)
Calculating the Surface Area Ratio
- Substituting the ratio of the radii:
- r1/r2 = 3/5
- Therefore, (r1^2)/(r2^2) = (3^2)/(5^2) = 9/25
Final Answer
- The ratio of the surface areas of the two spheres is 9:25, which corresponds to option 'D'.
The problem states that the volumes of two spheres are in the ratio 27:125. To find the ratio of their surface areas, we need to understand the relationships between the two.
Volume of a Sphere
- The formula for the volume (V) of a sphere is given by V = (4/3)πr^3, where r is the radius.
- If the volumes of two spheres are in the ratio 27:125, we can express this as:
- V1/V2 = 27/125
Finding the Ratio of Radii
- Since volumes are proportional to the cube of the radii, we have:
- (r1^3)/(r2^3) = 27/125
- Taking the cube root on both sides gives us:
- r1/r2 = (27^(1/3))/(125^(1/3)) = 3/5
Surface Area of a Sphere
- The formula for the surface area (A) of a sphere is A = 4πr^2.
- Now, to find the ratio of the surface areas of the two spheres, we have:
- A1/A2 = (4πr1^2)/(4πr2^2) = (r1^2)/(r2^2)
Calculating the Surface Area Ratio
- Substituting the ratio of the radii:
- r1/r2 = 3/5
- Therefore, (r1^2)/(r2^2) = (3^2)/(5^2) = 9/25
Final Answer
- The ratio of the surface areas of the two spheres is 9:25, which corresponds to option 'D'.
The dimensions of a rectangular box are in the ratio of 1:2:4 and the difference between the costs of covering it with the cloth and a sheet at the rate of Rs 20 and Rs 20.5 per sq m respectively is Rs 126. Find the dimensions of the box.- a)2 m, 6 m, 9 m
- b)6 m, 12 m, 24 m
- c)1 m, 2 m, 4 m
- d)None of these
Correct answer is option 'D'. Can you explain this answer?
The dimensions of a rectangular box are in the ratio of 1:2:4 and the difference between the costs of covering it with the cloth and a sheet at the rate of Rs 20 and Rs 20.5 per sq m respectively is Rs 126. Find the dimensions of the box.
a)
2 m, 6 m, 9 m
b)
6 m, 12 m, 24 m
c)
1 m, 2 m, 4 m
d)
None of these

|
Debanshi Rane answered |
Two circles of an equal radii are drawn, without a... moreny overlap, in a semicircle of radius 2 cm. If these are the largest possible circles that the semicircle can accommodate, what is the radius (in cm) of each of the circles?a)0.414b)0.828c)0.172d)0.586Correct answer is option 'B'. Can you explain this answer?

|
Naveen Unni answered |
The biggest possible regular hexagon H is cut out of an equilateral triangle X. The biggest possible equilateral triangle Y is cut out from the hexagon H. What is the ratio of the areas of the equilateral triangles X and Y?- a)5 : 1
- b)6 : 1
- c)8 : 1
- d)3 : 1
Correct answer is option 'D'. Can you explain this answer?
The biggest possible regular hexagon H is cut out of an equilateral triangle X. The biggest possible equilateral triangle Y is cut out from the hexagon H. What is the ratio of the areas of the equilateral triangles X and Y?
a)
5 : 1
b)
6 : 1
c)
8 : 1
d)
3 : 1

|
Srishti Joshi answered |
In the given figure, AD is the bisector of ∠BAC, AB = 6 cm, AC = 5 cm and BD = 3 cm. Find DC. It is given that ∠ABD = ∠ACD.
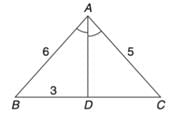
- a)11.3 cm
- b)4 cm
- c)3.5 cm
- d)2.5 cm
Correct answer is option 'D'. Can you explain this answer?
In the given figure, AD is the bisector of ∠BAC, AB = 6 cm, AC = 5 cm and BD = 3 cm. Find DC. It is given that ∠ABD = ∠ACD.

a)
11.3 cm
b)
4 cm
c)
3.5 cm
d)
2.5 cm
|
|
Pooja Shah answered |
We know that the internal bisector of angle of a triangle divides the opposite side internally in the ratio of the sides containing the angle.
Hence:
Hence:
In triangle ABD and ACD
Angle BAD = CAD (Given AD is the bisector)
Angle ABD = ACD (GIven)
there fore they are similar (AAA Property)
AB/BD = AC/CD
6/3 = 5/CD
CD = 2.5 cm
6/3 = 5/CD
CD = 2.5 cm
A pond 100 m in diameter is surrounded by a circular grass walk-way 2 m wide. How many square metres of grass is the on the walk-way?- a)98 π
- b)100 π
- c)204 π
- d)202 π
Correct answer is option 'C'. Can you explain this answer?
A pond 100 m in diameter is surrounded by a circular grass walk-way 2 m wide. How many square metres of grass is the on the walk-way?
a)
98 π
b)
100 π
c)
204 π
d)
202 π
|
|
Aarav Sharma answered |
The radius of the pond is 100/2 = <100 =50="">>50 m.
The radius of the grass walkway is 50+2 = <50+2=52>>52 m.
The area of the grass walkway is pi*(52^2 - 50^2) = pi*(2704 - 2500) = pi*204
≈ 204*pi
≈ 204*3.14
≈ <204*3.14=640.56>>640.56 m^2.
So, the answer is a) 640.
The radius of the grass walkway is 50+2 = <50+2=52>>52 m.
The area of the grass walkway is pi*(52^2 - 50^2) = pi*(2704 - 2500) = pi*204
≈ 204*pi
≈ 204*3.14
≈ <204*3.14=640.56>>640.56 m^2.
So, the answer is a) 640.
A square is inscribed in a semi circle of radius 10 cm. What is the area of the inscribed square? (Given that the side of the square is along the diameter of the semicircle.)- a)70 cm2
- b)50 cm2
- c)25 cm2
- d)80 cm2
Correct answer is option 'D'. Can you explain this answer?
A square is inscribed in a semi circle of radius 10 cm. What is the area of the inscribed square? (Given that the side of the square is along the diameter of the semicircle.)
a)
70 cm2
b)
50 cm2
c)
25 cm2
d)
80 cm2
|
|
Aarav Sharma answered |
Given:
Radius of the semicircle = 10 cm
Side of the square is along the diameter of the semicircle
To find:
Area of the inscribed square
Solution:
Let's draw the diagram and try to solve the problem.
[Insert Image]
1. Draw a semicircle of radius 10 cm.
[Insert Image]
2. Draw a diameter of the semicircle. Let's call it AB.
[Insert Image]
3. Draw a square ABCD with AB as one of its sides.
[Insert Image]
4. Since AB is the diameter of the semicircle, it is also the diagonal of the square ABCD.
[Insert Image]
5. Let's find the length of the side of the square.
Using Pythagoras theorem,
AB² = BC² + AC²
AB² = 10² + BC²
Since ABCD is a square, BC = CD = DA
AB² = 10² + BC² + BC²
AB² = 10² + 2BC²
BC² = (AB² - 10²)/2
BC = (AB² - 10²)/2√2
But AB = side of the square
Side of the square = (AB² - 10²)/2√2
Side of the square = (20² - 10²)/2√2
Side of the square = 10√2 cm
6. Now, we can find the area of the square.
Area of the square = (Side of the square)²
Area of the square = (10√2)²
Area of the square = 100 x 2
Area of the square = 200 cm²
Therefore, the area of the inscribed square is 200 cm².
Hence, the correct option is (D) 80 cm².
Radius of the semicircle = 10 cm
Side of the square is along the diameter of the semicircle
To find:
Area of the inscribed square
Solution:
Let's draw the diagram and try to solve the problem.
[Insert Image]
1. Draw a semicircle of radius 10 cm.
[Insert Image]
2. Draw a diameter of the semicircle. Let's call it AB.
[Insert Image]
3. Draw a square ABCD with AB as one of its sides.
[Insert Image]
4. Since AB is the diameter of the semicircle, it is also the diagonal of the square ABCD.
[Insert Image]
5. Let's find the length of the side of the square.
Using Pythagoras theorem,
AB² = BC² + AC²
AB² = 10² + BC²
Since ABCD is a square, BC = CD = DA
AB² = 10² + BC² + BC²
AB² = 10² + 2BC²
BC² = (AB² - 10²)/2
BC = (AB² - 10²)/2√2
But AB = side of the square
Side of the square = (AB² - 10²)/2√2
Side of the square = (20² - 10²)/2√2
Side of the square = 10√2 cm
6. Now, we can find the area of the square.
Area of the square = (Side of the square)²
Area of the square = (10√2)²
Area of the square = 100 x 2
Area of the square = 200 cm²
Therefore, the area of the inscribed square is 200 cm².
Hence, the correct option is (D) 80 cm².
One of the angles of a parallelogram is of 150°. Altitudes are drawn from the vertex of this angle. If these altitudes measure 6 cm and 8 cm, then find the perimeter of the parallelogram.
- a)28 cm
- b)42 cm
- c)56 cm
- d)64 cm
Correct answer is option 'C'. Can you explain this answer?
One of the angles of a parallelogram is of 150°. Altitudes are drawn from the vertex of this angle. If these altitudes measure 6 cm and 8 cm, then find the perimeter of the parallelogram.
a)
28 cm
b)
42 cm
c)
56 cm
d)
64 cm

|
Debolina Roy answered |

Two sides of a triangle are 4 and 5. Then, for the area of the triangle, which one of the following bounds is the sharpest?- a)<10.
- b)≤ 10.
- c)< 8.
- d)> 5.
Correct answer is option 'B'. Can you explain this answer?
Two sides of a triangle are 4 and 5. Then, for the area of the triangle, which one of the following bounds is the sharpest?
a)
<10.
b)
≤ 10.
c)
< 8.
d)
> 5.

|
Prerna Choudhary answered |
Let AB = 4 and BC = 5 and AB is perpendicular to BC
then Area = 1/2 AB . AC = 1/2 . 4.5 = 10
For a regular octagon inscribed in a circle of radius 1 cm, the product of the distance from a fixed vertex to the other seven vertices is- a)4
- b)8
- c)12
- d)16
Correct answer is option 'B'. Can you explain this answer?
For a regular octagon inscribed in a circle of radius 1 cm, the product of the distance from a fixed vertex to the other seven vertices is
a)
4
b)
8
c)
12
d)
16

|
Subham Basu answered |
We have to calculate AH x AG x AF x AE x AD x AC x AB
AE = 2
A gain by C osine form ula get A G (= A C) and A F (= AD)
A ring of diameter 10 cm is suspended from a point 12 cm vertically above the centre by six equal strings. The strings are attached to the circumference of the ring at equal intervals, thus keeping the ring in a horizontal plane. The value of cosine of the angle between the two adjacent strings lies in between- a)0 and 1/2
- b)1/2 and 1
- c)- 1 / 2 to 0
- d)- 1 t o - 1 / 2
Correct answer is option 'B'. Can you explain this answer?
A ring of diameter 10 cm is suspended from a point 12 cm vertically above the centre by six equal strings. The strings are attached to the circumference of the ring at equal intervals, thus keeping the ring in a horizontal plane. The value of cosine of the angle between the two adjacent strings lies in between
a)
0 and 1/2
b)
1/2 and 1
c)
- 1 / 2 to 0
d)
- 1 t o - 1 / 2

|
Srishti Joshi answered |
Consider the problem as a pyramid to the base of a regular hexagon.
Now in A POR
Now in A POR
OP = 12 cm
OR = 5 cm
PR = 13 cm
Now cosine formula will give the answer.
The line AB is 6 m, in length and is tangent to the inner of the two concentric circles at point C. It is known that the radii of the two circles are integers. The radiusof the outer circle is-------, where A and B are points on the outer circle.- a)5 m
- b)4 m
- c)6 m
- d)3 m
Correct answer is option 'A'. Can you explain this answer?
The line AB is 6 m, in length and is tangent to the inner of the two concentric circles at point C. It is known that the radii of the two circles are integers. The radiusof the outer circle is-------, where A and B are points on the outer circle.
a)
5 m
b)
4 m
c)
6 m
d)
3 m
|
|
Aarav Sharma answered |
Given Information
- The line AB is 6 m in length and is tangent to the inner of the two concentric circles at point C.
- The radii of the two circles are integers.
To Find
- The radius of the outer circle.
Solution
Let's consider the diagram below:
We can see that the radius of the inner circle is equal to the length of the perpendicular from the center of the circles to the line AB.
Let O be the center of the circles, and let OC be x. Then, by the Pythagorean theorem, we have:
OA^2 = OC^2 + AC^2
OB^2 = OC^2 + BC^2
Since OA = OB (both A and B are on the outer circle), we can subtract the two equations to get:
AC^2 - BC^2 = 0
(AC + BC)(AC - BC) = 0
Since AC and BC are both positive, we have AC = BC.
Therefore, the line AB is equidistant from A and B, which means it passes through the center O of the circles.
Let r be the radius of the outer circle. Then, we have:
OC = r - x (since x is the radius of the inner circle)
By the Pythagorean theorem, we also have:
AC^2 = r^2 - OC^2 = r^2 - (r - x)^2 = 2rx - x^2
Since AC = BC, we have:
2rx - x^2 = (6/2)^2 = 9
Simplifying, we get:
x(2r - x) = 9
Since x is an integer, and 2r - x is also an integer, we can see that x must be a factor of 9. The possible values of x are:
x = 1, 3, or 9
If x = 1, then 2r - x = 2r - 1 is odd, which means r is not an integer.
If x = 3, then 2r - x = 2r - 3 is odd, which means r is not an integer.
Therefore, we must have x = 9, which gives:
2r - x = 2r - 9
x(2r - x) = 9
Substituting x = 9, we get:
r = (x^2 + 9)/2x = (81 + 9)/18 = 5
Therefore, the radius of the outer circle is 5 m.
Answer: (a) 5 m
- The line AB is 6 m in length and is tangent to the inner of the two concentric circles at point C.
- The radii of the two circles are integers.
To Find
- The radius of the outer circle.
Solution
Let's consider the diagram below:
We can see that the radius of the inner circle is equal to the length of the perpendicular from the center of the circles to the line AB.
Let O be the center of the circles, and let OC be x. Then, by the Pythagorean theorem, we have:
OA^2 = OC^2 + AC^2
OB^2 = OC^2 + BC^2
Since OA = OB (both A and B are on the outer circle), we can subtract the two equations to get:
AC^2 - BC^2 = 0
(AC + BC)(AC - BC) = 0
Since AC and BC are both positive, we have AC = BC.
Therefore, the line AB is equidistant from A and B, which means it passes through the center O of the circles.
Let r be the radius of the outer circle. Then, we have:
OC = r - x (since x is the radius of the inner circle)
By the Pythagorean theorem, we also have:
AC^2 = r^2 - OC^2 = r^2 - (r - x)^2 = 2rx - x^2
Since AC = BC, we have:
2rx - x^2 = (6/2)^2 = 9
Simplifying, we get:
x(2r - x) = 9
Since x is an integer, and 2r - x is also an integer, we can see that x must be a factor of 9. The possible values of x are:
x = 1, 3, or 9
If x = 1, then 2r - x = 2r - 1 is odd, which means r is not an integer.
If x = 3, then 2r - x = 2r - 3 is odd, which means r is not an integer.
Therefore, we must have x = 9, which gives:
2r - x = 2r - 9
x(2r - x) = 9
Substituting x = 9, we get:
r = (x^2 + 9)/2x = (81 + 9)/18 = 5
Therefore, the radius of the outer circle is 5 m.
Answer: (a) 5 m
In any quadrilateral ABCD, the diagonal AC and BD intersect at a point X. If E, F, G and H are the midpoints of AX, BX, CX and DX respectively, then what is the ratio of (EF + FG + GH + GE) to (AD + DC + CB + BA)?- a)1/2
- b)2/3
- c)3/4
- d)Data insufficient
Correct answer is option 'A'. Can you explain this answer?
In any quadrilateral ABCD, the diagonal AC and BD intersect at a point X. If E, F, G and H are the midpoints of AX, BX, CX and DX respectively, then what is the ratio of (EF + FG + GH + GE) to (AD + DC + CB + BA)?
a)
1/2
b)
2/3
c)
3/4
d)
Data insufficient

|
Priyanka Datta answered |
This problem is based on mid-point theorem.
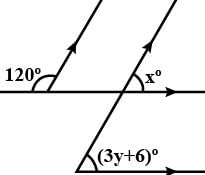
In the given figure, straight line AB and CD intersect at O. IF ∠δ =3∠v, then ∠v = ?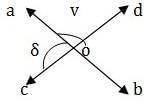
- a)40⁰
- b)50⁰
- c)55⁰
- d)45⁰
Correct answer is option 'D'. Can you explain this answer?
In the given figure, straight line AB and CD intersect at O. IF ∠δ =3∠v, then ∠v = ?

a)
40⁰
b)
50⁰
c)
55⁰
d)
45⁰
|
|
Meera Kapoor answered |
COD is a straight line
∴ ∠δ + ∠v =180⁰ ⇒ 3v +v =180 ⇒ 4v = 180 ⇒ v =45⁰.
∴ ∠δ + ∠v =180⁰ ⇒ 3v +v =180 ⇒ 4v = 180 ⇒ v =45⁰.
Two circles APQC and PBDQ intersect each other at the points P and Q and APB and CQD are two parallel straight lines. Then only one of the following statements is always true. Which one is it?- a)ABDC is a cyclic quadrilateral
- b)AC is parallel to BD
- c)ABDC is a rectangle
- d)AACQ is a right angle
Correct answer is option 'B'. Can you explain this answer?
Two circles APQC and PBDQ intersect each other at the points P and Q and APB and CQD are two parallel straight lines. Then only one of the following statements is always true. Which one is it?
a)
ABDC is a cyclic quadrilateral
b)
AC is parallel to BD
c)
ABDC is a rectangle
d)
AACQ is a right angle

|
Debolina Roy answered |
AB is the diameter of the circle and ∠PAB=40∘
what is the value of ∠PCA?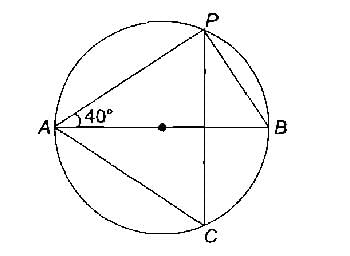
- a)50∘
- b)55°
- c)70°
- d)45°
Correct answer is option 'A'. Can you explain this answer?
AB is the diameter of the circle and ∠PAB=40∘
what is the value of ∠PCA?
what is the value of ∠PCA?

a)
50∘
b)
55°
c)
70°
d)
45°

|
KS Coaching Center answered |
- In △PAB⇒ ∠PAB=40o [ Given ]⇒ ∠BPA=90o [ angle inscribed in a semi-circle ]⇒ ∠PAB+∠PBA+∠BPA=180o∴ 40o+∠PBA+90o=180o∴ ∠PBA=180o−130o∴ ∠PBA=50o⇒ ∠PBA=∠PCA=50o [ angles inscribed in a same arc PA ]∴ ∠PCA=50o
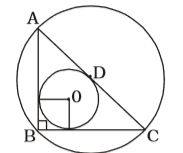
Three horses are grazing within a semi-circular field. In the diagram given below, AB is the diameter of the semi-circular field with centre at O. Horses are tied up at P, R and S such that PO and RO are the radii of semi-circles with centres at P and R respectively and S is the centre of the circle touching the two semi-circles with diameters AO and OB. The horses tied at P and R can graze within the respective semi-circle and the horse tied at S can graze within the circle centered at S. The percentage of the area of the semi-circles with diameter AB that cannot be grazed by the horses is nearest to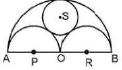
- a)20
- b)28
- c)36
- d)40
Correct answer is option 'B'. Can you explain this answer?
Three horses are grazing within a semi-circular field. In the diagram given below, AB is the diameter of the semi-circular field with centre at O. Horses are tied up at P, R and S such that PO and RO are the radii of semi-circles with centres at P and R respectively and S is the centre of the circle touching the two semi-circles with diameters AO and OB. The horses tied at P and R can graze within the respective semi-circle and the horse tied at S can graze within the circle centered at S. The percentage of the area of the semi-circles with diameter AB that cannot be grazed by the horses is nearest to
a)
20
b)
28
c)
36
d)
40

|
Uday Nambiar answered |
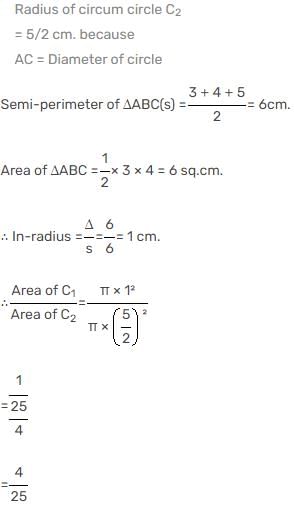
Consider the following figure: AB = 10 cm, AC = 17 cm, BC = 21 cm and EHFD is a square. Find the length of the side of square (in cm).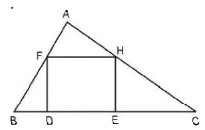
- a)10.5
- b)12
- c)13.5
- d)None of these
Correct answer is option 'D'. Can you explain this answer?
Consider the following figure: AB = 10 cm, AC = 17 cm, BC = 21 cm and EHFD is a square. Find the length of the side of square (in cm).
a)
10.5
b)
12
c)
13.5
d)
None of these

|
Sahil Kumar answered |
Side of the square should always be less than the attitude of the triangle .
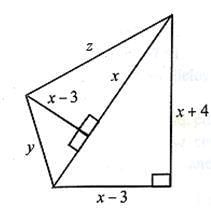
Based on the figure below, what is the value of x, if y=10.
- a)10
- b)11
- c)12
- d)None of these
Correct answer is option 'D'. Can you explain this answer?
Based on the figure below, what is the value of x, if y=10.

a)
10
b)
11
c)
12
d)
None of these

|
Priyanka Datta answered |
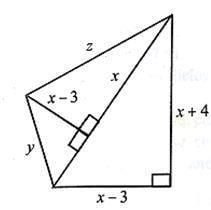
AB2 = (x+4)2 + (x−3)2 = 2x2 + 25 + 2x
Since solving this equation is very difficult. So, it is a better approach (Time saving) to put the values given in the options and try to find out a solution.
Hence, trying out we get 11 as the value of x .
Since solving this equation is very difficult. So, it is a better approach (Time saving) to put the values given in the options and try to find out a solution.
Hence, trying out we get 11 as the value of x .
What is the area of the triangle below?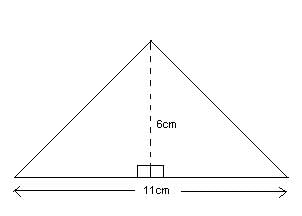
- a)22 cm2
- b)33 cm2
- c)44 cm2
- d)50 cm2
Correct answer is option 'B'. Can you explain this answer?
What is the area of the triangle below?

a)
22 cm2
b)
33 cm2
c)
44 cm2
d)
50 cm2

|
Pritam Saha answered |
The area of a triangle may be found by using the formula, A=1/2bh, where brepresents the base and h represents the height. Thus, the area may be written as A=1/2(11)(6), or A = 33. The area of the triangle is 33 cm'.
Chapter doubts & questions for Plane Geometry - CSAT Preparation 2025 is part of UPSC CSE exam preparation. The chapters have been prepared according to the UPSC CSE exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for UPSC CSE 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Plane Geometry - CSAT Preparation in English & Hindi are available as part of UPSC CSE exam.
Download more important topics, notes, lectures and mock test series for UPSC CSE Exam by signing up for free.

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Create your account for free
OR
Forgot Password
OR
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup