All Exams >
UPSC >
CSAT Preparation >
All Questions
All questions of Permutation & Combination for UPSC CSE Exam
A coin is tossed 3 times. Find out the number of possible outcomes.
- a)1
- b)8
- c)2
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
A coin is tossed 3 times. Find out the number of possible outcomes.
a)
1
b)
8
c)
2
d)
None of these

|
Ishani Rane answered |
For any multiple independent event, there are nm
total possible outcomes, where n is the number of outcomes per event, and m is the number of such events.
So for a coin, discounting the unlikely event of landing on its side, there are two possible outcomes per event, heads or tails. And it is stated that there are 3 such events. So nm=23=8
.
2a + 5b = 103. How many pairs of positive integer values can a, b take such that a > b?
- a)12
- b)9
- c)7
- d)8
Correct answer is option 'C'. Can you explain this answer?
2a + 5b = 103. How many pairs of positive integer values can a, b take such that a > b?
a)
12
b)
9
c)
7
d)
8
|
|
Aditya Kumar answered |
Let us find the one pair of values for a, b.
a = 4, b = 19 satisfies this equation.
2*4 + 5*19 = 103.
Now, if we increase ‘a’ by 5 and decrease ‘b’ by 2 we should get the next set of numbers. We can keep repeating this to get all values.
Let us think about why we increase ‘a’ by 5 and decrease b by 2.
a = 4, b = 19 works.
Let us say, we increase ‘a’ by n, then the increase would be 2n.
This has to be offset by a corresponding decrease in b.
Let us say we decrease b by ‘m’.
This would result in a net drop of 5m.
In order for the total to be same, 2n should be equal to 5m.
The smallest value of m, n for this to work would be 2, 5.
a = 4, b = 19
a = 9, b = 17
a = 14, b = 15
..
And so on till
a = 49, b = 1
We are also told that ‘a’ should be greater than ‘b’, then we have all combinations from (19, 13) … (49, 1).
7 pairs totally.
a = 4, b = 19 satisfies this equation.
2*4 + 5*19 = 103.
Now, if we increase ‘a’ by 5 and decrease ‘b’ by 2 we should get the next set of numbers. We can keep repeating this to get all values.
Let us think about why we increase ‘a’ by 5 and decrease b by 2.
a = 4, b = 19 works.
Let us say, we increase ‘a’ by n, then the increase would be 2n.
This has to be offset by a corresponding decrease in b.
Let us say we decrease b by ‘m’.
This would result in a net drop of 5m.
In order for the total to be same, 2n should be equal to 5m.
The smallest value of m, n for this to work would be 2, 5.
a = 4, b = 19
a = 9, b = 17
a = 14, b = 15
..
And so on till
a = 49, b = 1
We are also told that ‘a’ should be greater than ‘b’, then we have all combinations from (19, 13) … (49, 1).
7 pairs totally.
Hence the answer is "7"
Choice C is the correct answer.
How many 3 digit numbers can be formed from the digits 2, 3, 5, 6, 7 and 9 which are divisible by 5 and none of the digits is repeated?
- a)20
- b)16
- c)8
- d)24
Correct answer is option 'A'. Can you explain this answer?
How many 3 digit numbers can be formed from the digits 2, 3, 5, 6, 7 and 9 which are divisible by 5 and none of the digits is repeated?
a)
20
b)
16
c)
8
d)
24
|
|
Upasana Dey answered |
Since each desired number is divisible by 5, so we must have 5 at the unit place. So, there is 1 way of doing it.
The tens place can now be filled by any of the remaining 5 digits (2, 3, 6, 7, 9). So, there are 5 ways of filling the tens place.
The hundreds place can now be filled by any of the remaining 4 digits. So, there are 4 ways of filling it.
∴ Required number of numbers = (1 x 5 x 4) = 20.
The tens place can now be filled by any of the remaining 5 digits (2, 3, 6, 7, 9). So, there are 5 ways of filling the tens place.
The hundreds place can now be filled by any of the remaining 4 digits. So, there are 4 ways of filling it.
∴ Required number of numbers = (1 x 5 x 4) = 20.
MCQ (Multiple Choice Questions) with solution are available for Practice, which would help you prepare for Permutation and Combination under Quantitative Aptitude. You can practice these practice quizzes as per your speed and improvise the topic. The same topic is covered under various competitive examinations like - CAT, GMAT, Bank PO, SSC and other competitive examinations.
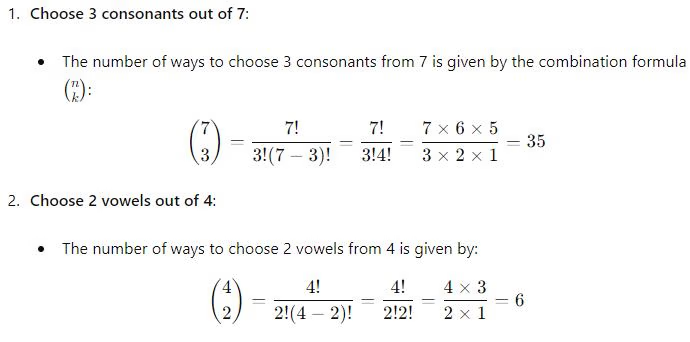
Q. Out of 7 consonants and 4 vowels, how many words of 3 consonants and 2 vowels can be formed?
- a)24400
- b)21300
- c)210
- d)25200
Correct answer is option 'D'. Can you explain this answer?
MCQ (Multiple Choice Questions) with solution are available for Practice, which would help you prepare for Permutation and Combination under Quantitative Aptitude. You can practice these practice quizzes as per your speed and improvise the topic. The same topic is covered under various competitive examinations like - CAT, GMAT, Bank PO, SSC and other competitive examinations.
Q. Out of 7 consonants and 4 vowels, how many words of 3 consonants and 2 vowels can be formed?
a)
24400
b)
21300
c)
210
d)
25200

|
Manoj Ghosh answered |

How many arrangements can be made out of the letters of the word 'ENGINEERING' ?
- a)924000
- b)277200
- c)182000
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
How many arrangements can be made out of the letters of the word 'ENGINEERING' ?
a)
924000
b)
277200
c)
182000
d)
None of these
|
|
Aditya Kumar answered |
The number of arrangements of the word ENGINEERING is 277200.
ENGINEERING word has 3 times of 3, three times of N, 2 times of G and 2 times of I. Then, the total letter is 11.
So, the number of arrangements of the word ENGINEERING = 11!/[3! * 3! * 2! * 2!] = 39916800/[6 * 6 * 2 * 2] = 277200
Hence, the number of arrangements of the word ENGINEERING is 277200.
ENGINEERING word has 3 times of 3, three times of N, 2 times of G and 2 times of I. Then, the total letter is 11.
So, the number of arrangements of the word ENGINEERING = 11!/[3! * 3! * 2! * 2!] = 39916800/[6 * 6 * 2 * 2] = 277200
Hence, the number of arrangements of the word ENGINEERING is 277200.
In how many different ways can the letters of the word 'MATHEMATICS' be arranged such that the vowels must always come together?- a)9800
- b)100020
- c)120960
- d)140020
Correct answer is option 'C'. Can you explain this answer?
a)
9800
b)
100020
c)
120960
d)
140020

|
Gowri Chakraborty answered |
In the word 'MATHEMATICS', we'll consider all the vowels AEAI together as one letter.
Thus, we have MTHMTCS (AEAI).
Now, we have to arrange 8 letters, out of which M occurs twice, T occurs twice
Number of ways of arranging these letters =8! / ((2!)(2!))= 10080.
Now, AEAI has 4 letters in which A occurs 2 times and the rest are different.
Number of ways of arranging these letters =4! / 2!= 12.
Required number of words = (10080 x 12) = 120960
A bag contains 2 white balls, 3 black balls and 4 red balls. In how many ways can 3 balls be drawn from the bag, if at least one black ball is to be included in the draw?
- a)64
- b)128
- c)32
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
A bag contains 2 white balls, 3 black balls and 4 red balls. In how many ways can 3 balls be drawn from the bag, if at least one black ball is to be included in the draw?
a)
64
b)
128
c)
32
d)
None of these

|
Gowri Chakraborty answered |
The bag contains 2 White, 3 Black and 4 Red balls.
So, total 9 balls are there in the bag; among them 3 are Black and 6 are non-Black balls.
Three balls can randomly be drawn in (9C3) = 84 ways.
1 Black and 2 non-Black balls can be drawn in (3C1)*(6C2) = 45 ways.
1 non-Black and 2 Black balls can be drawn in (6C1)*(3C2) = 18 ways.
3 Black balls can be drawn in (3C3) = 1 way.
So, three balls drawn in (45 + 18 + 1) = 64 ways will have at least one Black ball among the drawn ones.
How many 3-letter words with or without meaning, can be formed out of the letters of the word, 'LOGARITHMS', if repetition of letters is not allowed? - a)720
- b)420
- c)5040
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
a)
720
b)
420
c)
5040
d)
None of these

|
Gowri Chakraborty answered |
The word 'LOGARITHMS' has 10 different letters.
Hence, the number of 3-letter words(with or without meaning) formed by using these letters
= 10P3
= 10 * 9 * 8
= 720
In how many different ways can the letters of the word 'DETAIL' be arranged such that the vowels must occupy only the odd positions?
- a)36
- b)64
- c)120
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
In how many different ways can the letters of the word 'DETAIL' be arranged such that the vowels must occupy only the odd positions?
a)
36
b)
64
c)
120
d)
None of these

|
Naroj Boda answered |
There are 6 letters in the given word, out of which there are 3 vowels and 3 consonants.
Let us mark these positions as under:
(1) (2) (3) (4) (5) (6)
Now, 3 vowels can be placed at any of the three places out 4, marked 1, 3, 5.
Number of ways of arranging the vowels = 3P3
= 3! = 6.
Also, the 3 consonants can be arranged at the remaining 3 positions.
Number of ways of these arrangements = 3P3
= 3! = 6.
Total number of ways = (6 x 6) = 36.
In how many different ways can the letters of the word 'JUDGE' be arranged such that the vowels always come together?
- a)64
- b)48
- c)32
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
In how many different ways can the letters of the word 'JUDGE' be arranged such that the vowels always come together?
a)
64
b)
48
c)
32
d)
None of these
|
|
Anaya Patel answered |
The given word contains 5 different letters.
Keeping the vowels UE together, we suppose them as 1 letter.
Then, we have to arrange the letters JDG (UE).
Now, we have to arrange in 4! = 24 ways.
The vowels (UE) can be arranged among themselves in 2 ways.
∴ Required number of ways = (24 × 2) = 48
Keeping the vowels UE together, we suppose them as 1 letter.
Then, we have to arrange the letters JDG (UE).
Now, we have to arrange in 4! = 24 ways.
The vowels (UE) can be arranged among themselves in 2 ways.
∴ Required number of ways = (24 × 2) = 48
In how many different ways can the letters of the word 'LEADING' be arranged such that the vowels should always come together? - a)122
- b)720
- c)420
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
a)
122
b)
720
c)
420
d)
None of these

|
Arya Roy answered |
The word 'LEADING' has 7 different letters.
When the vowels EAI are always together, they can be supposed to form one letter.
Then, we have to arrange the letters LNDG (EAI).
Now, 5 (4 + 1) letters can be arranged in 5! = 120 ways.
The vowels (EAI) can be arranged among themselves in 3! = 6 ways.
Required number of ways = (120 * 6) = 720.
In how many different ways can the letters of the word 'OPTICAL' be arranged so that the vowels always come together? - a)610
- b)720
- c)825
- d)920
Correct answer is option 'B'. Can you explain this answer?
a)
610
b)
720
c)
825
d)
920
|
|
Nidhi Mukherjee answered |
The word 'OPTICAL' contains 7 different letters. When the vowels OIA are always together, they can be supposed to form one letter. Then, we have to arrange the letters PTCL (OIA). Now, 5 letters can be arranged in 5!=120 ways. The vowels (OIA) can be arranged among themselves in 3!=6 ways. Required number of ways =(120∗6)=720.
In how many different ways can the letters of the word 'CORPORATION' be arranged so that the vowels always come together? - a)47200
- b)48000
- c)42000
- d)50400
Correct answer is option 'D'. Can you explain this answer?
a)
47200
b)
48000
c)
42000
d)
50400

|
Gowri Chakraborty answered |
Vowels in the word "CORPORATION" are O,O,A,I,O
Lets make it as CRPRTN(OOAIO)
This has 7 lettes, where R is twice so value = 7!/2!
= 2520
Vowel O is 3 times, so vowels can be arranged = 5!/3!
= 20
Total number of words = 2520 * 20 = 50400
In a group of 6 boys and 4 girls, four children are to be selected. In how many different ways can they be selected such that at least one boy should be there? - a)159
- b)209
- c)201
- d)212
Correct answer is 'B'. Can you explain this answer?
a)
159
b)
209
c)
201
d)
212

|
Sameer Rane answered |
We may have (1 boy and 3 girls) or (2 boys and 2 girls) or (3 boys and 1 girl) or (4 boys).
Required number of ways = (6C1*4C3)+(6C2*4C2)+(6C3*4C1)+6C4
= (6C1*4C1)+(6C2*4C2)+(6C3*4C1)+6C2 = 209.
How many words with or without meaning, can be formed by using all the letters of the word, 'DELHI' using each letter exactly once?
- a)720
- b)24
- c)120
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
How many words with or without meaning, can be formed by using all the letters of the word, 'DELHI' using each letter exactly once?
a)
720
b)
24
c)
120
d)
None of these
|
|
Aditya Kumar answered |
Explanation :
The word 'DELHI' has 5 letters and all these letters are different.
Total words (with or without meaning) formed by using all these
5 letters using each letter exactly once
= Number of arrangements of 5 letters taken all at a time
= 5P5 = 5! = 5 x 4 x 3 x 2 x 1 = 120
Total words (with or without meaning) formed by using all these
5 letters using each letter exactly once
= Number of arrangements of 5 letters taken all at a time
= 5P5 = 5! = 5 x 4 x 3 x 2 x 1 = 120
A seven-digit number comprises of only 2's and 3's. How many of these are multiples of 12?
- a)10
- b)22
- c)12
- d)11
Correct answer is option 'D'. Can you explain this answer?
A seven-digit number comprises of only 2's and 3's. How many of these are multiples of 12?
a)
10
b)
22
c)
12
d)
11
|
|
Anshika Banerjee answered |
Number should be a multiple of 3 and 4. So, the sum of the digits should be a multiple of 3. We can either have all seven digits as 3, or have three 2's and four 3's, or six 2's and a 3.
(The number of 2's should be a multiple of 3).
For the number to be a multiple of 4, the last 2 digits should be 32. Now, let us combine these two.
All seven 3's - No possibility.
Three 2's and four 3's - The first 5 digits should have two 2's and three 3's in some order.
No of possibilities = 5!3!2!5!3!2! = 10
Six 2's and one 3 - The first 5 digits should all be 2's. So, there is only one number 2222232.
So, there are a total of 10 + 1 = 11 solutions.
(The number of 2's should be a multiple of 3).
For the number to be a multiple of 4, the last 2 digits should be 32. Now, let us combine these two.
All seven 3's - No possibility.
Three 2's and four 3's - The first 5 digits should have two 2's and three 3's in some order.
No of possibilities = 5!3!2!5!3!2! = 10
Six 2's and one 3 - The first 5 digits should all be 2's. So, there is only one number 2222232.
So, there are a total of 10 + 1 = 11 solutions.
Hence the answer is "11"
Choice D is the correct answer.
If we listed all numbers from 100 to 10,000, how many times would the digit 3 be printed?
- a)3600
- b)3768
- c)3980
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
If we listed all numbers from 100 to 10,000, how many times would the digit 3 be printed?
a)
3600
b)
3768
c)
3980
d)
None of these

|
Ishani Rane answered |
Three-digit numbers: A B C. 3 can be printed in the 100’s place or 10’s place or units place.
=> 100’s place: 3 B C. B can take values 0 to 9, C can take values 0 to 9. So, 3 gets printed in the 100’s place 100 times
=> 10’s place: A 3 C. A can take values 1 to 9, C can take values 0 to 9. So, 3 gets printed in the 10’s place 90 times
=> Unit’s place: A B 3. A can take values 1 to 9, B can take values 0 to 9. So, 3 gets printed in the unit’s place 90 times
So, 3 gets printed 280 times in 3-digit numbers
Four-digit numbers: A B C D. 3 can be printed in the 1000’s place, 100’s place or 10’s place or units place.
=> 1000’s place: 3 B C D. B can take values 0 to 9, C can take values 0 to 9, D can take values 0 to 9. So, 3 gets printed in the 100’s place 1000 times.
=> 100’s place: A 3 C D. A can take values 1 to 9, C & D can take values 0 to 9. So, 3 gets printed in the 100’s place 900 times.
=> 10’s place: A B 3 D. A can take values 1 to 9, B & D can take values 0 to 9. So, 3 gets printed in the 10’s place 900 times.
=> Unit’s place: A B C 3. A can take values 1 to 9, B & C can take values 0 to 9. So, 3 gets printed in the unit’s place 900 times.
3 gets printed 3700 times in 4-digit numbers.
So, there are totally 3700 + 280 = 3980 numbers.
=> 100’s place: 3 B C. B can take values 0 to 9, C can take values 0 to 9. So, 3 gets printed in the 100’s place 100 times
=> 10’s place: A 3 C. A can take values 1 to 9, C can take values 0 to 9. So, 3 gets printed in the 10’s place 90 times
=> Unit’s place: A B 3. A can take values 1 to 9, B can take values 0 to 9. So, 3 gets printed in the unit’s place 90 times
So, 3 gets printed 280 times in 3-digit numbers
Four-digit numbers: A B C D. 3 can be printed in the 1000’s place, 100’s place or 10’s place or units place.
=> 1000’s place: 3 B C D. B can take values 0 to 9, C can take values 0 to 9, D can take values 0 to 9. So, 3 gets printed in the 100’s place 1000 times.
=> 100’s place: A 3 C D. A can take values 1 to 9, C & D can take values 0 to 9. So, 3 gets printed in the 100’s place 900 times.
=> 10’s place: A B 3 D. A can take values 1 to 9, B & D can take values 0 to 9. So, 3 gets printed in the 10’s place 900 times.
=> Unit’s place: A B C 3. A can take values 1 to 9, B & C can take values 0 to 9. So, 3 gets printed in the unit’s place 900 times.
3 gets printed 3700 times in 4-digit numbers.
So, there are totally 3700 + 280 = 3980 numbers.
Hence the answer is "3980", Choice C is the correct answer.
In how many ways can a group of 5 men and 2 women be made out of a total of 7 men and 3 women? - a)1
- b)126
- c)63
- d)64
Correct answer is option 'C'. Can you explain this answer?
a)
1
b)
126
c)
63
d)
64

|
Ishani Rane answered |
Required number of ways = (7C5*3C2) = (7C2*3C1) = 63
Chapter doubts & questions for Permutation & Combination - CSAT Preparation 2025 is part of UPSC CSE exam preparation. The chapters have been prepared according to the UPSC CSE exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for UPSC CSE 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Permutation & Combination - CSAT Preparation in English & Hindi are available as part of UPSC CSE exam.
Download more important topics, notes, lectures and mock test series for UPSC CSE Exam by signing up for free.

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Create your account for free
OR
Forgot Password
OR
Signup on EduRev and stay on top of your study goals
10M+ students crushing their study goals daily














