All Exams >
JEE >
Chapter-wise Tests for JEE Main & Advanced >
All Questions
All questions of Permutations and Combinations for JEE Exam
A lady wants to select one cotton saree and one polyster saree from a textile shop. If there are 15 cotton and 13 polyster varieties in that shop, in how many ways can she pick up two sarees?- a)125
- b)215
- c)345
- d)195
Correct answer is option 'D'. Can you explain this answer?
A lady wants to select one cotton saree and one polyster saree from a textile shop. If there are 15 cotton and 13 polyster varieties in that shop, in how many ways can she pick up two sarees?
a)
125
b)
215
c)
345
d)
195
|
|
Suresh Reddy answered |
The lady can select one cotton saree out of 15 cotton varieties in 15 ways since
any of 15 varieties can be selected. Corresponding to each selection of a cotton saree, she can
choose a polyester saree in 13 ways. Hence the two sarees (one cotton and one polyester), by
multiplication principle of counting, can be selected in 15 x 13= 195 ways
any of 15 varieties can be selected. Corresponding to each selection of a cotton saree, she can
choose a polyester saree in 13 ways. Hence the two sarees (one cotton and one polyester), by
multiplication principle of counting, can be selected in 15 x 13= 195 ways
The number of ways in which the 6 faces of a cube can be painted with 6 different colours is- a)6
- b)12
- c)6!
- d)none of these
Correct answer is option 'D'. Can you explain this answer?
The number of ways in which the 6 faces of a cube can be painted with 6 different colours is
a)
6
b)
12
c)
6!
d)
none of these
|
|
Preeti Iyer answered |
Consider the face that has red color. There are fi... moreve face
Four alphabets A, M, P, O are purchased from a warehouse. How many ordered pairs of initials can be formed using these?- a)10
- b)16
- c)18
- d)12
Correct answer is option 'D'. Can you explain this answer?
Four alphabets A, M, P, O are purchased from a warehouse. How many ordered pairs of initials can be formed using these?
a)
10
b)
16
c)
18
d)
12
|
|
Riya Banerjee answered |
Total number of letters = 4
Number of ordered pairs of letters that can be formed like (A, M) or (P, O) etc = 4P2 = 4!/2!
= 24/2
= 12
Number of ordered pairs of letters that can be formed like (A, M) or (P, O) etc = 4P2 = 4!/2!
= 24/2
= 12
In how many ways can a cricket team of 11 players be chosen out from a squad of 14 players, if 5 particular players are always chosen?- a)84
- b)144
- c)1001
- d)1365
Correct answer is option 'A'. Can you explain this answer?
In how many ways can a cricket team of 11 players be chosen out from a squad of 14 players, if 5 particular players are always chosen?
a)
84
b)
144
c)
1001
d)
1365
|
|
Aryan Khanna answered |
Total no of players = 14 out of which 5 are fixed.
So, 11-5 = 6
Remaining players = 14 - 6
= 9 players
9C6 = 9!/(3!*6!)
= 84
So, 11-5 = 6
Remaining players = 14 - 6
= 9 players
9C6 = 9!/(3!*6!)
= 84
How many three digit odd numbers can be formed by using the digits 5,6,7,8 if the repetition of digits is allowed?- a)64
- b)32
- c)24
- d)None of these
Correct answer is 'B'. Can you explain this answer?
How many three digit odd numbers can be formed by using the digits 5,6,7,8 if the repetition of digits is allowed?
a)
64
b)
32
c)
24
d)
None of these
|
|
Pooja Shah answered |
The unit place can be filled in 2 ways because question asked to find 3 digit odd numbers from 5,6,7,8 and the other two places can be filled in 4 ways as repetition is allowed.
So, Unit's place can be filled in 2 ways. Decimal place can be filled in 4 ways. Hundred's place can be filled in 4 ways.
=> 4*4*2 = 32.
So, Unit's place can be filled in 2 ways. Decimal place can be filled in 4 ways. Hundred's place can be filled in 4 ways.
=> 4*4*2 = 32.
In how many ways can 3 letters be posted in 4 letter boxes?- a)27
- b)4!
- c)64
- d)3!
Correct answer is option 'C'. Can you explain this answer?
In how many ways can 3 letters be posted in 4 letter boxes?
a)
27
b)
4!
c)
64
d)
3!
|
|
Preeti Iyer answered |
The first letter can be posted in 4 ways. So, total outcomes about the first letter = 4.
For every outcome about the first letter, the second letter can be posted in 4 ways. So, total outcomes about the first and the second letters= (4*4) = 16.
Therefore, following the same route, we can say, total possible outcomes about the first and the second letters = (4*4*4) = 64.
In how many ways 2 directors and 3 executives can be arranged for a meeting? If there are 6 chairs available two on one side and remaining four on the other side of the table and the two directors has to be together on one side and the executives on the other side.- a)24
- b)48
- c)720
- d)120
Correct answer is option 'B'. Can you explain this answer?
In how many ways 2 directors and 3 executives can be arranged for a meeting? If there are 6 chairs available two on one side and remaining four on the other side of the table and the two directors has to be together on one side and the executives on the other side.
a)
24
b)
48
c)
720
d)
120
|
|
Hansa Sharma answered |
3 executives in 4 chairs = 4P3 ways
Total no. of ways = 2! * 4P3
=> 2*1*(4!/1!)
= 2*24
= 48
Total no. of ways = 2! * 4P3
=> 2*1*(4!/1!)
= 2*24
= 48
How many three digit odd numbers can be formed by using the digits 1,2,3,4,5,6 if the repetition of digit is not allowed:- a)216
- b)120
- c)60
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
How many three digit odd numbers can be formed by using the digits 1,2,3,4,5,6 if the repetition of digit is not allowed:
a)
216
b)
120
c)
60
d)
None of these
|
|
Lavanya Menon answered |
There can be only 3 digits at the last digit (1,3,5) the middle digit should have 5 No's. out of 6, because one digit is used in last digit similarly 1st digit will have 4 No's.
Therefore : 4×5×3 => 60
A man has 3 jackets, 10 shirts, and 5 pairs of slacks. If an outfit consists of a jacket, a shirt, and a pair of slacks, how many different outfits can the man make ?Correct answer is '150'. Can you explain this answer?
A man has 3 jackets, 10 shirts, and 5 pairs of slacks. If an outfit consists of a jacket, a shirt, and a pair of slacks, how many different outfits can the man make ?
|
|
Vijay Kumar answered |
Total number of jackets=3
Total number of shirts=10
Total number of pair of slacks=5
Ways of selecting one jacket =3C1=3
Ways of selecting one shirt =10C1=10
Ways of selecting one pair of slack = 5C1=5
Number of different outfits that can be made=3×10×5=150
Number of different outfits that can be made=150
Total number of shirts=10
Total number of pair of slacks=5
Ways of selecting one jacket =3C1=3
Ways of selecting one shirt =10C1=10
Ways of selecting one pair of slack = 5C1=5
Number of different outfits that can be made=3×10×5=150
Number of different outfits that can be made=150
Total number of four digit odd numbers that can be formed using 0, 1, 2, 3, 5, 7 (using repetition allowed) are [2002]- a)216
- b)375
- c)400
- d)720
Correct answer is option 'D'. Can you explain this answer?
Total number of four digit odd numbers that can be formed using 0, 1, 2, 3, 5, 7 (using repetition allowed) are [2002]
a)
216
b)
375
c)
400
d)
720
|
|
Dishani Dey answered |
Required number of numbers = 5 x 6 x 6 x 4 = 36 x 20 = 720.
How many numbers are there between 100 and 1000 , in which all the digits are distinct?- a)729
- b)648
- c)858
- d)546
Correct answer is option 'B'. Can you explain this answer?
How many numbers are there between 100 and 1000 , in which all the digits are distinct?
a)
729
b)
648
c)
858
d)
546
|
|
Nandini Patel answered |
H T U
9.9.8 = 9 x 9 x 8 = 648 numbers
No. of digits in hundreds place should be filled first as the zero should be excluded
(1,2,3,4,5,6,7,8,9) = 9 ways
No. of digits in tens place (now the zero can be included but one digit has been used at hundreds place) so, no. of ways we can fill ten's place is = 9 ways
No of digits in units place is any one among the remaining 8 no. = 8 ways
so, 9 x 9 x 8 = 648
The number of different ways in which a man can invite one or more of his 6 friends to dinner is?- a)30
- b)63
- c)120
- d)15
Correct answer is option 'B'. Can you explain this answer?
The number of different ways in which a man can invite one or more of his 6 friends to dinner is?
a)
30
b)
63
c)
120
d)
15

|
Infinity Academy answered |
He can invite any one friend in 6C1 ways = 6 ways:
He can invite any two friends in 6C2 ways = 15 ways
He can invite any three friends in 6C3 ways = 20 ways
He can invite any 4 friends in 6C4ways = 15 ways
He can invite any 5 friends in 6C5 ways = 6 ways
He can invite all the 6 friends in 6C6 ways= 1 way.
Since any one of these could happen total possibilities are, 6+15+20+15+6+1 = 63.
He can invite any two friends in 6C2 ways = 15 ways
He can invite any three friends in 6C3 ways = 20 ways
He can invite any 4 friends in 6C4ways = 15 ways
He can invite any 5 friends in 6C5 ways = 6 ways
He can invite all the 6 friends in 6C6 ways= 1 way.
Since any one of these could happen total possibilities are, 6+15+20+15+6+1 = 63.
In how many ways can a cricket team be selected out of 15 players if a particular player has to be included?- a)C(14,11)
- b)C(14,10)
- c)C(15,11)
- d)C(15,10)
Correct answer is option 'A'. Can you explain this answer?
In how many ways can a cricket team be selected out of 15 players if a particular player has to be included?
a)
C(14,11)
b)
C(14,10)
c)
C(15,11)
d)
C(15,10)
|
|
Pooja Shah answered |
11 players can be selected out of 16 in 16C11 ways = 16!/(11! 5!) = 4368 ways. Now, If two particular players is to be included and one particular player is to be rejected, then we have to select 9 more players out of 13 in 13C9 ways.
= 13!/(9! 4!) = 715 ways.
= 13!/(9! 4!) = 715 ways.
A room has 8 doors. In how many ways, a man can enter in the room through one door and exit through a different door?- a)5040
- b)40320
- c)56
- d)8
Correct answer is option 'C'. Can you explain this answer?
A room has 8 doors. In how many ways, a man can enter in the room through one door and exit through a different door?
a)
5040
b)
40320
c)
56
d)
8
|
|
Naina Sharma answered |
The person has 8 options to enter the hall. For each of these 8 options, he has 7 options to exit the hall. Thus, he has 8 × 7 = 56 ways to enter and exit from different doors.
There are 7 chairs in a row. In how many ways can 3 persons occupy any three of them- a)240
- b)210
- c)260
- d)120
Correct answer is option 'B'. Can you explain this answer?
There are 7 chairs in a row. In how many ways can 3 persons occupy any three of them
a)
240
b)
210
c)
260
d)
120
|
|
Neha Joshi answered |
First person can sit on any of the 7 seats, second person can sit on other 6 vacant seats except one occupied by the first one. Similarly, the third can do sit on 5 so, total no. of ways = 7*6*5 = 210
How many triangles can be formed by joining four points on a circle?- a)8
- b)6
- c)4
- d)2
Correct answer is option 'C'. Can you explain this answer?
How many triangles can be formed by joining four points on a circle?
a)
8
b)
6
c)
4
d)
2
|
|
Preeti Khanna answered |
Triangle formed = mC3
Therefore, 4 points on circle, m = 4
4C3 = 4C1
⇒ 4
Therefore, 4 points on circle, m = 4
4C3 = 4C1
⇒ 4
Nidhi has 6 friends. In how many ways can she invite one or more of them to a party at her home?- a)64
- b)63
- c)25
- d)24
Correct answer is option 'B'. Can you explain this answer?
Nidhi has 6 friends. In how many ways can she invite one or more of them to a party at her home?
a)
64
b)
63
c)
25
d)
24
|
|
Lavanya Menon answered |
She has 6 friends and he wants to invite one or more. That is the same as saying he wants to invite at least 1 of his friends.
So, the number of ways he could do this is:
Invite only one friend
Invite any two friends
Invite any three friends
Invite any four friends
Invite any five friends
Invite all six friends
This can be thought of in terms of combinations. Inviting r friends out of n is same as choosing r friends out of n . So, we can write the possibilities as:
6C1 + 6C2 + 6C3 + 6C4 + 6C5 + 6C6
= 6 + 15 + 20 + 15 + 6 + 1
= 63
So, the number of ways he could do this is:
Invite only one friend
Invite any two friends
Invite any three friends
Invite any four friends
Invite any five friends
Invite all six friends
This can be thought of in terms of combinations. Inviting r friends out of n is same as choosing r friends out of n . So, we can write the possibilities as:
6C1 + 6C2 + 6C3 + 6C4 + 6C5 + 6C6
= 6 + 15 + 20 + 15 + 6 + 1
= 63
The number of ways in which 6 “ + “ and 4 “ – “ signs can be arranged in a line such that no two “ – “ signs occur together is- a)P(10,4)
- b)C(7,4)
- c)C(10,4)
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
The number of ways in which 6 “ + “ and 4 “ – “ signs can be arranged in a line such that no two “ – “ signs occur together is
a)
P(10,4)
b)
C(7,4)
c)
C(10,4)
d)
none of these

|
Sushil Kumar answered |
′+′ signs can be put in a row in one way creating seven gaps shown as arrows:
Now 4′−′ signs must be kept in these gaps. So, no tow ′−′ signs should be together.
Out of these 7 gaps 4 can be chosen in 7C4 ways.
Now 4′−′ signs must be kept in these gaps. So, no tow ′−′ signs should be together.
Out of these 7 gaps 4 can be chosen in 7C4 ways.
A lady arranges a dinner party for 6 guests .The number of ways in which they may be selected from among 10 friends if 2 of the friends will not attend the party together is- a)164
- b)140
- c)112
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
A lady arranges a dinner party for 6 guests .The number of ways in which they may be selected from among 10 friends if 2 of the friends will not attend the party together is
a)
164
b)
140
c)
112
d)
none of these
|
|
Neha Joshi answered |
Let us say that the two particular friends are A and B.
If A is invited among six guests and B is not, then: number of combinations to select 5 more guests from the remaining 8 friends:
C(8, 5) = 8 ! / (5! 3!) = 56
If B is invited among the six guests and A is not , then the number of ways of selecting the remaining 5 guests = C(8, 5) = 56
Suppose both A and B are not included in the six guests list : then the number of such combinations = C(8, 6) = 7 * 8 /2 = 28
So the total number of sets of guests that can be selected = 140.
If A is invited among six guests and B is not, then: number of combinations to select 5 more guests from the remaining 8 friends:
C(8, 5) = 8 ! / (5! 3!) = 56
If B is invited among the six guests and A is not , then the number of ways of selecting the remaining 5 guests = C(8, 5) = 56
Suppose both A and B are not included in the six guests list : then the number of such combinations = C(8, 6) = 7 * 8 /2 = 28
So the total number of sets of guests that can be selected = 140.
Number of signals that can be made using given 4 flags of which 3 are blue and 1 is red?- a)3i
- b)12
- c)4i
- d)4
Correct answer is option 'D'. Can you explain this answer?
Number of signals that can be made using given 4 flags of which 3 are blue and 1 is red?
a)
3i
b)
12
c)
4i
d)
4

|
Shiksha Academy answered |
Total flags = 4
Blue = 3
red = 1
Total no. of signals = (4!*3!)/3!
= 4
Blue = 3
red = 1
Total no. of signals = (4!*3!)/3!
= 4
How many number of four digits can be formed with the digits 1,2,3,4,5 if the digit can be repeated in any number of times?- a)55
- b)5!
- c)625
- d)9000
Correct answer is option 'C'. Can you explain this answer?
How many number of four digits can be formed with the digits 1,2,3,4,5 if the digit can be repeated in any number of times?
a)
55
b)
5!
c)
625
d)
9000
|
|
Om Desai answered |
There are 5 digits 1,2,3,4 & 5. the 4 digit number can be formed with the digits 1,2,3,4,5 where digits can be repeated =5*5*5*5=625.
The total number of ways of answering 5 objective questions, each question having four choices are- a)2880
- b)120
- c)1024
- d)None of the above
Correct answer is option 'C'. Can you explain this answer?
The total number of ways of answering 5 objective questions, each question having four choices are
a)
2880
b)
120
c)
1024
d)
None of the above
|
|
Preeti Iyer answered |
The total ways of answering would be. 4x4x4x4x4=4^5 that will be 1024.
In how many ways can 4 red, 3 yellow and 2 green chairs be arranged in a row if the chairs of the same colour are indistinguishable?- a)15120
- b)1260
- c)362880
- d)None of the above
Correct answer is option 'B'. Can you explain this answer?
In how many ways can 4 red, 3 yellow and 2 green chairs be arranged in a row if the chairs of the same colour are indistinguishable?
a)
15120
b)
1260
c)
362880
d)
None of the above
|
|
Hansa Sharma answered |
Total no of balls = 9
red balls = 4
yellow balls = 3
green balls = 2
Total no. of arrangements = 9!/(4!*3!*2!)
= 1260
red balls = 4
yellow balls = 3
green balls = 2
Total no. of arrangements = 9!/(4!*3!*2!)
= 1260
Among 7 flags 4 are of red colour and the rest are all different colours.How mant different signals can be generated using these flags?- a)240
- b)210
- c)180
- d)120
Correct answer is option 'B'. Can you explain this answer?
Among 7 flags 4 are of red colour and the rest are all different colours.How mant different signals can be generated using these flags?
a)
240
b)
210
c)
180
d)
120
|
|
Poonam Reddy answered |
Total flags = 4
Different colour = 3
red = 4
Total no. of signals = 7P3
= 7!/(4!)
= 210
Different colour = 3
red = 4
Total no. of signals = 7P3
= 7!/(4!)
= 210
How many 4 digit numbers are there, when a digit may be repeated any number of times?- a)3×43
- b)9×103
- c)104
- d)410
Correct answer is option 'B'. Can you explain this answer?
How many 4 digit numbers are there, when a digit may be repeated any number of times?
a)
3×43
b)
9×103
c)
104
d)
410
|
|
Nandini Patel answered |
If we assume the leading digit can not be zero, then the first 4-digit number is 1000.
The last 4-digit number is 9999.
—
The number of 4-digit numbers is therefore:
9999 - 1000 + 1 = 9000
——
Another way to calculate this:
1..9 are available for the first digit —> 9 choices
0..9 are available for the 2nd, 3rd, and 4th digits —> 10 choices for each: 10*10*10 = 1000 choices total
and 9*1000 = 9000
A coin is tossed 6 times, in how many throws can 4 heads and 2 tails be obtained?- a)24
- b)18
- c)15
- d)10
Correct answer is option 'C'. Can you explain this answer?
A coin is tossed 6 times, in how many throws can 4 heads and 2 tails be obtained?
a)
24
b)
18
c)
15
d)
10
|
|
Naina Bansal answered |
Whether we toss a coin 6 times or six coins one time the number of arrangement will remain same .
As to find number of ways we get 4 heads and 2 tails out of 6 times
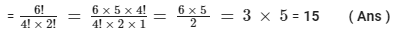
In how many ways, a party of 5 men and 5 women be seated at a circular table, so that no two women are adjacent?- a)720
- b)14400
- c)2880
- d)1440
Correct answer is option 'C'. Can you explain this answer?
In how many ways, a party of 5 men and 5 women be seated at a circular table, so that no two women are adjacent?
a)
720
b)
14400
c)
2880
d)
1440

|
Ayush Joshi answered |
Lets first place the men (M). '*' here indicates the linker of round table
* M -M - M - M - M *
which is in (5-1)! ways
So we have to place the women in between the men which is on the 5 empty seats ( 4 -'s and 1 linker i.e * )
SO 5 women can sit on 5 seats in (5)! ways or
1st seat in 5 ways
2nd seat 4
3rd seat 3
4th seat 2
5th seat 1
i.e 5*4*3*2*1 ways
So the answer is 5! * 4! = 2880
Four visitors A, B, C & D arrive at a town which has 5 hotels. In how many ways can they disperse themselves among 5 hotels, if 4 hotels are used to accommodate them.Correct answer is '120'. Can you explain this answer?
Four visitors A, B, C & D arrive at a town which has 5 hotels. In how many ways can they disperse themselves among 5 hotels, if 4 hotels are used to accommodate them.
|
|
Krishna Iyer answered |
Given,
Number of visitors= 4
Number of hotels= 5
So, A can disperse among 5 hotels,
B can disperse among 4 hotels,
C can disperse among 3 hotels,
D can disperse among 2 hotels.
∴ They can disperse in the hotels in 5 × 4 × 3 × 2 = 120 ways
Number of visitors= 4
Number of hotels= 5
So, A can disperse among 5 hotels,
B can disperse among 4 hotels,
C can disperse among 3 hotels,
D can disperse among 2 hotels.
∴ They can disperse in the hotels in 5 × 4 × 3 × 2 = 120 ways
Sonia has 10 balloons out of which 5 are red, 2 white, 2 blue and 1pink, which she wants to use for the decoration. Her favourite pink colour balloon should be filled with toffees and should be put at the centre of the room above the cake table and remaining 9 at the wall behind the cake table. How many ways she can arrange the balloons?- a)956
- b)756
- c)9!
- d)7560
Correct answer is option 'B'. Can you explain this answer?
Sonia has 10 balloons out of which 5 are red, 2 white, 2 blue and 1pink, which she wants to use for the decoration. Her favourite pink colour balloon should be filled with toffees and should be put at the centre of the room above the cake table and remaining 9 at the wall behind the cake table. How many ways she can arrange the balloons?
a)
956
b)
756
c)
9!
d)
7560
|
|
Pooja Shah answered |
1 balloon is fixed that is to be placed above the cake
Now she have to arrange 9 balloons = 9!/(5!*2!*2!)
(9*8*7*6*5!)/((5!*2*2)
= (9*8*7*6)/(2*2)
= 756
Now she have to arrange 9 balloons = 9!/(5!*2!*2!)
(9*8*7*6*5!)/((5!*2*2)
= (9*8*7*6)/(2*2)
= 756
The number of ways in which three different rings can be worn in four fingers with at most one in each finger, are- a)12
- b)64
- c)24
- d)6
Correct answer is 'C'. Can you explain this answer?
The number of ways in which three different rings can be worn in four fingers with at most one in each finger, are
a)
12
b)
64
c)
24
d)
6
|
|
Gaurav Kumar answered |
The total number of ways is same as the number of arrangements of 4 fingers, taken 3 at a time.
So, required number of ways = 4P3
= 4!/(4-3)!
= 4!/1!
= 4! => 24
So, required number of ways = 4P3
= 4!/(4-3)!
= 4!/1!
= 4! => 24
In a college there are 20 professors including the principal and the vice principal. A committee of 5 is to be formed. In how many ways it can be formed so that neither the principal nor the vice principal is included?- a)C(18,2)
- b)C(20,2)
- c)C(20,18)
- d)C(18,5)
Correct answer is option 'D'. Can you explain this answer?
In a college there are 20 professors including the principal and the vice principal. A committee of 5 is to be formed. In how many ways it can be formed so that neither the principal nor the vice principal is included?
a)
C(18,2)
b)
C(20,2)
c)
C(20,18)
d)
C(18,5)
|
|
Preeti Iyer answered |
Out of 20 professors, excluding the principal and vice-principal, 18 professors are left.
No. of ways of selecting 5 professors out of 18 = 18C5
No. of ways of selecting 5 professors out of 18 = 18C5
In a class, there are 30 boys and 18 girls. The teacher wants to select one boy and one girl to represent the class for quiz competition. In how many ways can the teacher make this selection?- a)625
- b)125
- c)25
- d)540
Correct answer is option 'D'. Can you explain this answer?
In a class, there are 30 boys and 18 girls. The teacher wants to select one boy and one girl to represent the class for quiz competition. In how many ways can the teacher make this selection?
a)
625
b)
125
c)
25
d)
540
|
|
Vijay Kumar answered |
Out of 30 Boys,
No. of ways to select 1 boy = 30C1 = 30
Out of 18 Girls,
No. of ways to select 1 boy = 18C1 = 18
No. of ways of selecting 1 boy and 1 girl =
30*18 = 540
No. of ways to select 1 boy = 30C1 = 30
Out of 18 Girls,
No. of ways to select 1 boy = 18C1 = 18
No. of ways of selecting 1 boy and 1 girl =
30*18 = 540
A coin is tossed n times, the number of all the possible outcomes is- a)C(n , 2)
- b)P(n , 2)
- c)2n
- d)2n
Correct answer is option 'D'. Can you explain this answer?
A coin is tossed n times, the number of all the possible outcomes is
a)
C(n , 2)
b)
P(n , 2)
c)
2n
d)
2n
|
|
Krishna Iyer answered |
If coin is tossed n times then possible number of outcomes = 2n
8Cr = 8Cp. So- a)r = p or r = 8p
- b)r = p or r + p = 8
- c)r = p or r – p = 8
- d)r = p or r = 2p
Correct answer is option 'B'. Can you explain this answer?
8Cr = 8Cp. So
a)
r = p or r = 8p
b)
r = p or r + p = 8
c)
r = p or r – p = 8
d)
r = p or r = 2p
|
|
Aryan Khanna answered |
8Cr = 8Cp = 8C8-p
So, either r = p or r = 8 – p
i.e. r = p or r + p = 8
So, either r = p or r = 8 – p
i.e. r = p or r + p = 8
How many of the 900 three digit numbers have at least one even digit ?- a)775
- b)875
- c)450
- d)750
Correct answer is option 'A'. Can you explain this answer?
How many of the 900 three digit numbers have at least one even digit ?
a)
775
b)
875
c)
450
d)
750
|
|
Krishna Iyer answered |
Total 3 digit numbers = 900
There are only 5 odd numbers (1,3,5,7,9)
So 3 digit numbers having containing only odd digits = 5*5*5 = 125
So, 3 digit numbers containing at least one even digit = 900–125 = 775
There are only 5 odd numbers (1,3,5,7,9)
So 3 digit numbers having containing only odd digits = 5*5*5 = 125
So, 3 digit numbers containing at least one even digit = 900–125 = 775
The number of ways in which 5 boys and 3 girls can sit around a round table so that all the girls are not sit together is- a)4020
- b)4120
- c)4220
- d)4320
Correct answer is option 'D'. Can you explain this answer?
The number of ways in which 5 boys and 3 girls can sit around a round table so that all the girls are not sit together is
a)
4020
b)
4120
c)
4220
d)
4320

|
Sai Kulkarni answered |
The correct option is D.
Number of ways in which 8 people can sit around a round table
= (8 — 1)! = 5040 ways
If three girls sit together, we consider it as 1 group and these
three girls can sit in 3! ways.
So, number of ways in which 3 girls sit together in a
the circular table of 8 people is 3!5! = 720.
Required number of ways in which no three girls sit together =
5040 -720 = 4320
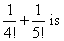
- a)4/5!
- b)5/5!
- c)6/5!
- d)1/5!
Correct answer is option 'C'. Can you explain this answer?
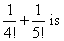
a)
4/5!
b)
5/5!
c)
6/5!
d)
1/5!
|
|
Pooja Shah answered |
1/4! + 1/5!
= 1/4![1 + 1/5]
1/4![6/5]
=> 6/5!
= 1/4![1 + 1/5]
1/4![6/5]
=> 6/5!
The number of different ways in which a man can invite one or more of his 6 friends to dinner is- a)63
- b)15
- c)30
- d)120
Correct answer is option 'A'. Can you explain this answer?
The number of different ways in which a man can invite one or more of his 6 friends to dinner is
a)
63
b)
15
c)
30
d)
120
|
|
Om Desai answered |
He can invite one or more friends by inviting 1 friend, or 2 friends or 3 friends, or all the 6 friends.
1 friend can be selected out of 6 in 6C1 = 6 ways
2 friends can be selected out of 6 in 6C2 = 15 ways
3 friends can be selected out of 6 in 6C3 = 20 ways
4 friends can be selected out of 6 in 6C4 = 15 ways
5 friends can be selected out of 6 in 6C5 = 6 ways
6 friends can be selected out of 6 in 6C6 = 1 ways
Therefore the required number of ways (combinations) = 6 + 15 + 20 + 15 + 6 + 1 = 63
1 friend can be selected out of 6 in 6C1 = 6 ways
2 friends can be selected out of 6 in 6C2 = 15 ways
3 friends can be selected out of 6 in 6C3 = 20 ways
4 friends can be selected out of 6 in 6C4 = 15 ways
5 friends can be selected out of 6 in 6C5 = 6 ways
6 friends can be selected out of 6 in 6C6 = 1 ways
Therefore the required number of ways (combinations) = 6 + 15 + 20 + 15 + 6 + 1 = 63
If nC8 = nC2, then n is- a)8
- b)12
- c)10
- d)2
Correct answer is option 'C'. Can you explain this answer?
If nC8 = nC2, then n is
a)
8
b)
12
c)
10
d)
2
|
|
Shadow answered |
NCr =nCs-->n=r+s ,n=8+2=10
The number of three digit numbers having atleast one digit as 5 is- a)225
- b)246
- c)648
- d)252
Correct answer is option 'D'. Can you explain this answer?
The number of three digit numbers having atleast one digit as 5 is
a)
225
b)
246
c)
648
d)
252
|
|
Ravi Sharma answered |
These digit number without digit 5 →100....999
→ these are 900 three-digit number
→ from 100 to 199 → 19 number with 5.
200−299→19
300−399→19
400−499→19
600−699→19
700−799→19
800−899→19
900−999→19
500−599→100
total number with 5=19×8+100 for (500-599)
=152+100
=252
→ these are 900 three-digit number
→ from 100 to 199 → 19 number with 5.
200−299→19
300−399→19
400−499→19
600−699→19
700−799→19
800−899→19
900−999→19
500−599→100
total number with 5=19×8+100 for (500-599)
=152+100
=252
On a railway track, there are 20 stations. The number of tickets required in order that it may be possible to book a passenger from every station to every other is- a)C(20,2)
- b)P(20,2)
- c)400
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
On a railway track, there are 20 stations. The number of tickets required in order that it may be possible to book a passenger from every station to every other is
a)
C(20,2)
b)
P(20,2)
c)
400
d)
none of these
|
|
Milan Shah answered |
Solution:
- There are 20 stations in total.
- Number of ways to select 2 stations out of 20 is given by C(20,2).
- So, the number of tickets required to book a passenger from every station to every other station is P(20,2).
- Therefore, the correct answer is option B.
The number of seven digit i ntegers, with sum of the digits equal to 10 and formed by using the digits 1, 2 and 3 only, is (2009)- a)55
- b)66
- c)77
- d)88
Correct answer is option 'C'. Can you explain this answer?
The number of seven digit i ntegers, with sum of the digits equal to 10 and formed by using the digits 1, 2 and 3 only, is (2009)
a)
55
b)
66
c)
77
d)
88
|
|
Lavanya Menon answered |
We h ave to for m 7 digit n umber s, using th e digits 1, 2 and 3 only, such that the sum of the digits in a number = 10.
This can be done by taking 2, 2, 2, 1, 1, 1, 1, or by taking 2, 3, 1, 1, 1, 1, 1.
∴ Number of ways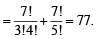
This can be done by taking 2, 2, 2, 1, 1, 1, 1, or by taking 2, 3, 1, 1, 1, 1, 1.
∴ Number of ways
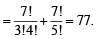
The number of all numbers that can be formed by using some or all of the digits 1, 3, 5, 7, 9 (without repetitions) is- a)325
- b)120
- c)32
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
The number of all numbers that can be formed by using some or all of the digits 1, 3, 5, 7, 9 (without repetitions) is
a)
325
b)
120
c)
32
d)
none of these
|
|
Pooja Shah answered |
Out of 1, 3, 5, 7, 9
No. of 1-digit numbers = 5
No. of 2-digit numbers = 5*4 = 20
No. of 3-digit numbers = 5*4*3 = 60
No. of 4-digit numbers = 5*4*3*2 = 120
No. of 5-digit numbers = 5*4*3*2*1 = 120
Total no. of numbers = 5 + 20 + 60 + 120 + 120 = 325
No. of 1-digit numbers = 5
No. of 2-digit numbers = 5*4 = 20
No. of 3-digit numbers = 5*4*3 = 60
No. of 4-digit numbers = 5*4*3*2 = 120
No. of 5-digit numbers = 5*4*3*2*1 = 120
Total no. of numbers = 5 + 20 + 60 + 120 + 120 = 325
A committee of 7 has to be formed from 9 boys and 4 girls.In how many ways can this be done when the committee consists of Exactly 3 girls?

|
Shiksha Academy answered |
out of 7 persons , we have to select exactly 3 girls . hence, it's clear that remaining 4 persons will be boys .
Now, number of ways taken 4 boys from 9 boys = 9C4
and number of ways taken 3 girls from 4 girls = 4C3
hence, by fundamental principle of counting ,
total number of ways for making the committee = 9C4 × 4C3
= 9!/5!.4! × 4!/3!
= 9×8×7×6/3×2×1
= 9×8×7
= 504 ways
Now, number of ways taken 4 boys from 9 boys = 9C4
and number of ways taken 3 girls from 4 girls = 4C3
hence, by fundamental principle of counting ,
total number of ways for making the committee = 9C4 × 4C3
= 9!/5!.4! × 4!/3!
= 9×8×7×6/3×2×1
= 9×8×7
= 504 ways
If (n + 1)! = 20(n – 1)!, then n is equal to- a)20
- b)5
- c)-5
- d)4
Correct answer is option 'D'. Can you explain this answer?
If (n + 1)! = 20(n – 1)!, then n is equal to
a)
20
b)
5
c)
-5
d)
4
|
|
Vikas Kapoor answered |
(n + 1)! = 20 (n – 1)!
n (n + 1) = 20
(n – 4) (n + 5) = 0
Since, (n – 1)! exists, n ≥ 1
So, n = 4
n (n + 1) = 20
(n – 4) (n + 5) = 0
Since, (n – 1)! exists, n ≥ 1
So, n = 4
The figures 4, 5, 6, 7, 8 are written in every possible order. The number of numbers greater than 56000 is- a)98
- b)72
- c)90
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
The figures 4, 5, 6, 7, 8 are written in every possible order. The number of numbers greater than 56000 is
a)
98
b)
72
c)
90
d)
none of these
|
|
Riya Banerjee answered |
There are in total 6 numbers, 4,5,6,7,8.
Now consider the number 56000
Consider the numbers of the form
56−−−.
Considering no repetitions we get
3×2×1 = 6 numbers.
Similarly for 57−−− and 58−−−.
Hence 3×6 = 18 numbers.
Now consider the numbers starting with 6.
6−−−−
We get 4×3×2×1 = 24.
Similarly for the numbers starting with 7 and 8 we get in total 24 numbers each.
Hence total number of numbers greater than 56000 will be
= (24×3)+18
= 72+18 = 90
Now consider the number 56000
Consider the numbers of the form
56−−−.
Considering no repetitions we get
3×2×1 = 6 numbers.
Similarly for 57−−− and 58−−−.
Hence 3×6 = 18 numbers.
Now consider the numbers starting with 6.
6−−−−
We get 4×3×2×1 = 24.
Similarly for the numbers starting with 7 and 8 we get in total 24 numbers each.
Hence total number of numbers greater than 56000 will be
= (24×3)+18
= 72+18 = 90
Total number of four digit odd numbers that can be formed using 0, 1, 2, 3, 4, 5, 7 are- a)216
- b)375
- c)1176
- d)720
Correct answer is option 'C'. Can you explain this answer?
Total number of four digit odd numbers that can be formed using 0, 1, 2, 3, 4, 5, 7 are
a)
216
b)
375
c)
1176
d)
720
|
|
Om Desai answered |
Total number of four digit odd numbers = 6 × 7 × 7 × 4 = 1176.
Chapter doubts & questions for Permutations and Combinations - Chapter-wise Tests for JEE Main & Advanced 2025 is part of JEE exam preparation. The chapters have been prepared according to the JEE exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for JEE 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Permutations and Combinations - Chapter-wise Tests for JEE Main & Advanced in English & Hindi are available as part of JEE exam.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Create your account for free
OR
Forgot Password
OR
Signup on EduRev and stay on top of your study goals
10M+ students crushing their study goals daily

 ?
?