Class 10 Exam > Class 10 Questions > Obtain all the zeroes of x4+7x-17x2-17x=6 if...
Start Learning for Free
Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1?
Verified Answer
Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 ...
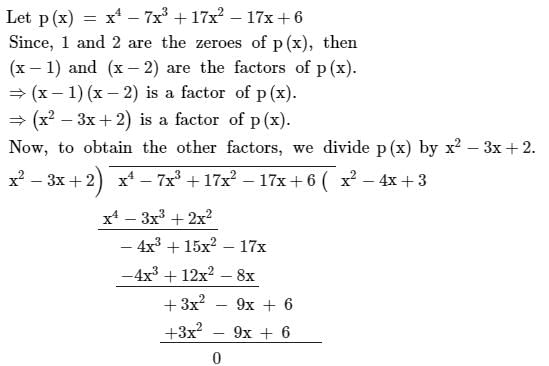
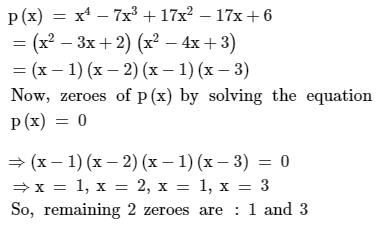
 This question is part of UPSC exam. View all Class 10 courses
This question is part of UPSC exam. View all Class 10 courses
Most Upvoted Answer
Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 ...
Solution:
To find the remaining zeroes of the given polynomial equation, we can use the fact that if two of its zeroes are 3 and 1, then the polynomial must be divisible by (x-3) and (x-1).
Let's factorize the polynomial equation using these two known zeroes:
x^4 + 7x - 17x^2 - 17x = 6
Rearranging the terms, we get:
x^4 - 17x^2 + 7x - 17x = 6
x(x^3 - 17x + 7 - 17) = 6
x(x^3 - 17x - 10) = 6
Since (x-3) and (x-1) are factors of the polynomial, we can write:
x^3 - 17x - 10 = 6/(x-3)(x-1)
Now, let's find the remaining zeroes using the factor theorem:
1. Synthetic Division:
Using synthetic division, we can divide the polynomial (x^3 - 17x - 10) by (x-3).
Using synthetic division, we get:
3 | 1 0 -17 -10
| 3 9 -24
-----------------------
1 3 -8 -34
The quotient is x^2 + 3x - 8 with a remainder of -34.
Hence, we have reduced the polynomial to:
(x-3)(x^2 + 3x - 8) = 6/(x-3)(x-1)
2. Quadratic Equation:
Now, let's solve the quadratic equation x^2 + 3x - 8 = 6/(x-1) to find the other two zeroes.
Multiplying both sides by (x-1), we get:
(x-1)(x^2 + 3x - 8) = 6
Expanding the equation, we have:
x^3 + 3x^2 - 8x - x^2 - 3x + 8 = 6
Simplifying further, we get:
x^3 + 2x^2 - 11x + 2 = 0
Now, we have reduced the polynomial to a cubic equation. To solve this equation and find the remaining zeroes, we can use numerical methods such as the Newton-Raphson method or graphical methods like plotting the graph and finding the roots.
3. Additional Methods:
Other methods like factoring, completing the square, or using the cubic formula can also be used to find the remaining zeroes of the cubic equation.
In conclusion, by using the known zeroes and applying the factor theorem, we were able to reduce the given polynomial equation and find a cubic equation. The remaining zeroes can be found by solving the cubic equation using numerical or graphical methods.
To find the remaining zeroes of the given polynomial equation, we can use the fact that if two of its zeroes are 3 and 1, then the polynomial must be divisible by (x-3) and (x-1).
Let's factorize the polynomial equation using these two known zeroes:
x^4 + 7x - 17x^2 - 17x = 6
Rearranging the terms, we get:
x^4 - 17x^2 + 7x - 17x = 6
x(x^3 - 17x + 7 - 17) = 6
x(x^3 - 17x - 10) = 6
Since (x-3) and (x-1) are factors of the polynomial, we can write:
x^3 - 17x - 10 = 6/(x-3)(x-1)
Now, let's find the remaining zeroes using the factor theorem:
1. Synthetic Division:
Using synthetic division, we can divide the polynomial (x^3 - 17x - 10) by (x-3).
Using synthetic division, we get:
3 | 1 0 -17 -10
| 3 9 -24
-----------------------
1 3 -8 -34
The quotient is x^2 + 3x - 8 with a remainder of -34.
Hence, we have reduced the polynomial to:
(x-3)(x^2 + 3x - 8) = 6/(x-3)(x-1)
2. Quadratic Equation:
Now, let's solve the quadratic equation x^2 + 3x - 8 = 6/(x-1) to find the other two zeroes.
Multiplying both sides by (x-1), we get:
(x-1)(x^2 + 3x - 8) = 6
Expanding the equation, we have:
x^3 + 3x^2 - 8x - x^2 - 3x + 8 = 6
Simplifying further, we get:
x^3 + 2x^2 - 11x + 2 = 0
Now, we have reduced the polynomial to a cubic equation. To solve this equation and find the remaining zeroes, we can use numerical methods such as the Newton-Raphson method or graphical methods like plotting the graph and finding the roots.
3. Additional Methods:
Other methods like factoring, completing the square, or using the cubic formula can also be used to find the remaining zeroes of the cubic equation.
In conclusion, by using the known zeroes and applying the factor theorem, we were able to reduce the given polynomial equation and find a cubic equation. The remaining zeroes can be found by solving the cubic equation using numerical or graphical methods.

|
Explore Courses for Class 10 exam
|

|
Similar Class 10 Doubts
Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1?
Question Description
Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1?.
Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1?.
Solutions for Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? in English & in Hindi are available as part of our courses for Class 10.
Download more important topics, notes, lectures and mock test series for Class 10 Exam by signing up for free.
Here you can find the meaning of Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? defined & explained in the simplest way possible. Besides giving the explanation of
Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1?, a detailed solution for Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? has been provided alongside types of Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? theory, EduRev gives you an
ample number of questions to practice Obtain all the zeroes of x4+7x-17x2-17x=6 if two of its zeroes are 3 and 1? tests, examples and also practice Class 10 tests.

|
Explore Courses for Class 10 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























