NEET Exam > NEET Questions > The correction factor 'a' to the idea...
Start Learning for Free
The correction factor 'a' to the ideal gas equationcorresponds to
- a)density of the gas molecules
- b)volume of the gas molecules
- c)electric field present between the gas molecules
- d)forces of attraction between the gas molecules
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The correction factor 'a' to the ideal gas equationcorresponds...
Vanderwaal constant (a)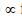 
forces of attraction.
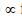forces of attraction.Most Upvoted Answer
The correction factor 'a' to the ideal gas equationcorresponds...
The correction factor, denoted by 'a', in the ideal gas equation corresponds to the forces of attraction between the gas molecules. The ideal gas equation, also known as the equation of state for an ideal gas, is given by:
PV = nRT
Where:
P = pressure of the gas
V = volume of the gas
n = number of moles of the gas
R = ideal gas constant
T = temperature of the gas
Explanation:
The ideal gas equation assumes that gas molecules do not interact with each other and occupy no volume. However, in reality, gas molecules do interact with each other and occupy some volume due to intermolecular forces.
The correction factor 'a' is introduced to account for these deviations from ideal behavior. It represents the attractive forces between gas molecules. When the gas molecules are close to each other, these attractive forces come into play and reduce the pressure of the gas compared to what would be expected based on the ideal gas equation.
Key Points:
- The correction factor 'a' in the ideal gas equation accounts for the forces of attraction between gas molecules.
- In reality, gas molecules do interact with each other and occupy some volume due to intermolecular forces.
- The correction factor 'a' corrects for these deviations from ideal behavior by reducing the pressure of the gas.
- The value of 'a' depends on the nature and strength of the intermolecular forces present in the gas.
- Gases with stronger intermolecular forces will have higher values of 'a' compared to gases with weaker intermolecular forces.
Overall, the correction factor 'a' in the ideal gas equation is introduced to account for the forces of attraction between gas molecules, which deviate from the assumptions of an ideal gas. It helps to provide a more accurate description of the behavior of real gases by incorporating the effects of intermolecular forces.
PV = nRT
Where:
P = pressure of the gas
V = volume of the gas
n = number of moles of the gas
R = ideal gas constant
T = temperature of the gas
Explanation:
The ideal gas equation assumes that gas molecules do not interact with each other and occupy no volume. However, in reality, gas molecules do interact with each other and occupy some volume due to intermolecular forces.
The correction factor 'a' is introduced to account for these deviations from ideal behavior. It represents the attractive forces between gas molecules. When the gas molecules are close to each other, these attractive forces come into play and reduce the pressure of the gas compared to what would be expected based on the ideal gas equation.
Key Points:
- The correction factor 'a' in the ideal gas equation accounts for the forces of attraction between gas molecules.
- In reality, gas molecules do interact with each other and occupy some volume due to intermolecular forces.
- The correction factor 'a' corrects for these deviations from ideal behavior by reducing the pressure of the gas.
- The value of 'a' depends on the nature and strength of the intermolecular forces present in the gas.
- Gases with stronger intermolecular forces will have higher values of 'a' compared to gases with weaker intermolecular forces.
Overall, the correction factor 'a' in the ideal gas equation is introduced to account for the forces of attraction between gas molecules, which deviate from the assumptions of an ideal gas. It helps to provide a more accurate description of the behavior of real gases by incorporating the effects of intermolecular forces.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.
|
Explore Courses for NEET exam
|
|
Similar NEET Doubts
Top Courses for NEETView all
The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer?
Question Description
The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer?.
The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer?.
Solutions for The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The correction factor 'a' to the ideal gas equationcorresponds toa)density of the gas moleculesb)volume of the gas moleculesc)electric field present between the gas moleculesd)forces of attraction between the gas moleculesCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice NEET tests.
|
|
Explore Courses for NEET exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup