Electrical Engineering (EE) Exam > Electrical Engineering (EE) Questions > How many complex multiplications are performe...
Start Learning for Free
How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?
- a)N(L+M+2)
- b)N(L+M-2)
- c)N(L+M-1)
- d)N(L+M+1)
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
How many complex multiplications are performed in computing the N-poin...
Explanation: The expression for N point DFT is given as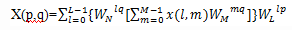
The first step involves L DFTs, each of M points. Hence this step requires LM2 complex multiplications, second require LM and finally third requires ML2. So, Total complex multiplications= N(L+M+1)
The first step involves L DFTs, each of M points. Hence this step requires LM2 complex multiplications, second require LM and finally third requires ML2. So, Total complex multiplications= N(L+M+1)
Most Upvoted Answer
How many complex multiplications are performed in computing the N-poin...
Complex Multiplications in Computing the N-point DFT using Divide-and-Conquer Method
The divide-and-conquer method is a popular technique used to compute the Discrete Fourier Transform (DFT) of a sequence. In this method, the sequence is divided into smaller subsequences, and the DFT of each subsequence is computed separately. These smaller DFTs are then combined to obtain the DFT of the original sequence.
In the divide-and-conquer method, the sequence is typically divided into two halves recursively until the base case is reached. The base case occurs when the sequence length is 1, in which case the DFT of the sequence is simply the sequence itself.
To compute the DFT of a sequence of length N using the divide-and-conquer method, the sequence is divided into two subsequences of length N/2. Each subsequence is then further divided into two subsequences of length N/4, and so on, until the base case is reached.
Complex Multiplications in Each Level
At each level of the divide-and-conquer method, complex multiplications are performed to compute the DFT of the subsequences. Let's consider a level where the sequence length is N/(2^k), where k is the level number.
At this level, there are N/(2^k) subsequences, each of length N/(2^(k+1)). To compute the DFT of each subsequence, N/(2^(k+1)) complex multiplications are required. Therefore, at this level, the total number of complex multiplications is N/(2^k) * N/(2^(k+1)).
Total Number of Complex Multiplications
To compute the DFT of a sequence of length N using the divide-and-conquer method, the sequence is divided into log2(N) levels, starting from the top-level where the sequence length is N, and reaching the base case where the sequence length is 1.
The total number of complex multiplications can be calculated by summing up the number of complex multiplications at each level. Let's consider the case where N = L * M, where L and M are integers.
- At the top-level, the number of complex multiplications is N * 1 = N.
- At the next level, the number of complex multiplications is (N/2) * (N/2^2) = N/2.
- At the next level, the number of complex multiplications is (N/4) * (N/2^3) = N/4.
- ...
- At the base case, the number of complex multiplications is 1 * (N/2^log2(N)) = N/(2^log2(N)) = N.
The total number of complex multiplications is the sum of the number of complex multiplications at each level, which can be simplified as:
N + N/2 + N/4 + ... + N/(2^log2(N)) = N * (1 + 1/2 + 1/4 + ... + 1/(2^log2(N))) = N * (1 - (1/2)^log2(N)) = N * (1 - 1/N) = N * (N-1)/N = N * (L * M - 1)/(L * M) = N * (L * M - 1)/(N) = L * M
The divide-and-conquer method is a popular technique used to compute the Discrete Fourier Transform (DFT) of a sequence. In this method, the sequence is divided into smaller subsequences, and the DFT of each subsequence is computed separately. These smaller DFTs are then combined to obtain the DFT of the original sequence.
In the divide-and-conquer method, the sequence is typically divided into two halves recursively until the base case is reached. The base case occurs when the sequence length is 1, in which case the DFT of the sequence is simply the sequence itself.
To compute the DFT of a sequence of length N using the divide-and-conquer method, the sequence is divided into two subsequences of length N/2. Each subsequence is then further divided into two subsequences of length N/4, and so on, until the base case is reached.
Complex Multiplications in Each Level
At each level of the divide-and-conquer method, complex multiplications are performed to compute the DFT of the subsequences. Let's consider a level where the sequence length is N/(2^k), where k is the level number.
At this level, there are N/(2^k) subsequences, each of length N/(2^(k+1)). To compute the DFT of each subsequence, N/(2^(k+1)) complex multiplications are required. Therefore, at this level, the total number of complex multiplications is N/(2^k) * N/(2^(k+1)).
Total Number of Complex Multiplications
To compute the DFT of a sequence of length N using the divide-and-conquer method, the sequence is divided into log2(N) levels, starting from the top-level where the sequence length is N, and reaching the base case where the sequence length is 1.
The total number of complex multiplications can be calculated by summing up the number of complex multiplications at each level. Let's consider the case where N = L * M, where L and M are integers.
- At the top-level, the number of complex multiplications is N * 1 = N.
- At the next level, the number of complex multiplications is (N/2) * (N/2^2) = N/2.
- At the next level, the number of complex multiplications is (N/4) * (N/2^3) = N/4.
- ...
- At the base case, the number of complex multiplications is 1 * (N/2^log2(N)) = N/(2^log2(N)) = N.
The total number of complex multiplications is the sum of the number of complex multiplications at each level, which can be simplified as:
N + N/2 + N/4 + ... + N/(2^log2(N)) = N * (1 + 1/2 + 1/4 + ... + 1/(2^log2(N))) = N * (1 - (1/2)^log2(N)) = N * (1 - 1/N) = N * (N-1)/N = N * (L * M - 1)/(L * M) = N * (L * M - 1)/(N) = L * M
Attention Electrical Engineering (EE) Students!
To make sure you are not studying endlessly, EduRev has designed Electrical Engineering (EE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Electrical Engineering (EE).

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Similar Electrical Engineering (EE) Doubts
How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer?
Question Description
How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? for Electrical Engineering (EE) 2024 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer?.
How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? for Electrical Engineering (EE) 2024 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer?.
Solutions for How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electrical Engineering (EE).
Download more important topics, notes, lectures and mock test series for Electrical Engineering (EE) Exam by signing up for free.
Here you can find the meaning of How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer?, a detailed solution for How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? has been provided alongside types of How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice How many complex multiplications are performed in computing the N-point DFT of a sequence using divide-and-conquer method if N=LM?a)N(L+M+2)b)N(L+M-2)c)N(L+M-1)d)N(L+M+1)Correct answer is option 'D'. Can you explain this answer? tests, examples and also practice Electrical Engineering (EE) tests.

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























