Class 10 Exam > Class 10 Questions > A horse is tied to a peg at one corner of a s...
Start Learning for Free
A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze is
- a)128 sq. cm
- b)154 sq. cm
- c)142 sq. cm
- d)156 sq. cm
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A horse is tied to a peg at one corner of a square shaped gross field ...
Area of the shaded region 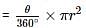
Area of the shaded region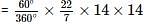
Area of the shaded region = 154 sq. cm
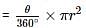
Area of the shaded region
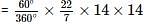
Area of the shaded region = 154 sq. cm
Most Upvoted Answer
A horse is tied to a peg at one corner of a square shaped gross field ...
To solve this problem, we need to visualize the situation and understand the constraints given in the problem.
Given:
- The horse is tied to a peg at one corner of a square-shaped field.
- The side of the square field is 25m.
- The horse is tied to the peg by a 14m long rope.
Step 1: Visualize the situation
The field is a square with side length 25m. Let's assume the corner where the horse is tied is the bottom left corner of the square. The horse can move freely within the field but is restricted by the length of the rope, which is 14m.
Step 2: Determine the area the horse can graze
To determine the area the horse can graze, we need to find the shape formed by the rope when it is stretched to its maximum length within the field. This shape will be a sector of a circle with radius 14m.
Step 3: Calculate the area of the sector
The formula to calculate the area of a sector is given by:
Area of sector = (θ/360) * π * r^2
where θ is the angle formed by the sector and r is the radius of the circle.
In this case, the angle of the sector can be calculated using the formula:
θ = 2 * arctan(d/2r)
where d is the side length of the square field and r is the radius of the circle.
Substituting the given values:
θ = 2 * arctan(25/2*14) = 2 * arctan(25/28) ≈ 1.127 radians
Now, we can calculate the area of the sector:
Area of sector = (1.127/360) * π * 14^2 ≈ 77.467 sq. m
Step 4: Determine the area the horse can graze within the square field
To determine the area the horse can graze within the square field, we subtract the area of the triangle formed by the sector from the area of the square field.
Area of the square field = 25^2 = 625 sq. m
Area the horse can graze = 625 - 77.467 ≈ 547.533 sq. m
Step 5: Convert the area to square centimeters
To convert the area to square centimeters, we multiply by 10,000 (since 1 square meter = 10,000 square centimeters).
Area the horse can graze = 547.533 * 10,000 ≈ 5,475,330 sq. cm
Rounding off to the nearest whole number, the area the horse can graze is approximately 5,475,330 sq. cm.
Therefore, the correct answer is option 'B' - 154 sq. cm.
Given:
- The horse is tied to a peg at one corner of a square-shaped field.
- The side of the square field is 25m.
- The horse is tied to the peg by a 14m long rope.
Step 1: Visualize the situation
The field is a square with side length 25m. Let's assume the corner where the horse is tied is the bottom left corner of the square. The horse can move freely within the field but is restricted by the length of the rope, which is 14m.
Step 2: Determine the area the horse can graze
To determine the area the horse can graze, we need to find the shape formed by the rope when it is stretched to its maximum length within the field. This shape will be a sector of a circle with radius 14m.
Step 3: Calculate the area of the sector
The formula to calculate the area of a sector is given by:
Area of sector = (θ/360) * π * r^2
where θ is the angle formed by the sector and r is the radius of the circle.
In this case, the angle of the sector can be calculated using the formula:
θ = 2 * arctan(d/2r)
where d is the side length of the square field and r is the radius of the circle.
Substituting the given values:
θ = 2 * arctan(25/2*14) = 2 * arctan(25/28) ≈ 1.127 radians
Now, we can calculate the area of the sector:
Area of sector = (1.127/360) * π * 14^2 ≈ 77.467 sq. m
Step 4: Determine the area the horse can graze within the square field
To determine the area the horse can graze within the square field, we subtract the area of the triangle formed by the sector from the area of the square field.
Area of the square field = 25^2 = 625 sq. m
Area the horse can graze = 625 - 77.467 ≈ 547.533 sq. m
Step 5: Convert the area to square centimeters
To convert the area to square centimeters, we multiply by 10,000 (since 1 square meter = 10,000 square centimeters).
Area the horse can graze = 547.533 * 10,000 ≈ 5,475,330 sq. cm
Rounding off to the nearest whole number, the area the horse can graze is approximately 5,475,330 sq. cm.
Therefore, the correct answer is option 'B' - 154 sq. cm.
Attention Class 10 Students!
To make sure you are not studying endlessly, EduRev has designed Class 10 study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class 10.

|
Explore Courses for Class 10 exam
|

|
Similar Class 10 Doubts
A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer?
Question Description
A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? for Class 10 2024 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 10 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer?.
A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? for Class 10 2024 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 10 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 10.
Download more important topics, notes, lectures and mock test series for Class 10 Exam by signing up for free.
Here you can find the meaning of A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A horse is tied to a peg at one corner of a square shaped gross field of side 25m by means of a 14m long rope. The area of that part of the field in which the horse can graze isa)128 sq. cmb)154 sq. cmc)142 sq. cmd)156 sq. cmCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Class 10 tests.

|
Explore Courses for Class 10 exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























