Physics Exam > Physics Questions > If the time period of oscillation of mass M s...
Start Learning for Free
If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :
Correct answer is '2'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
If the time period of oscillation of mass M suspended from a spring is...
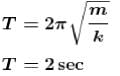
The correct answer is: 2
Most Upvoted Answer
If the time period of oscillation of mass M suspended from a spring is...
Explanation:
To understand why the time period of oscillation for a mass 4M is 2 seconds, we need to first understand the relationship between the time period of oscillation and the mass of the object.
Time Period of Oscillation:
The time period of oscillation is the time taken for one complete cycle of oscillation. It is denoted by the symbol T and is measured in seconds.
Hooke's Law and the Time Period:
The time period of oscillation for a mass-spring system can be determined using Hooke's law, which states that the force exerted by a spring is directly proportional to the displacement of the spring from its equilibrium position.
Mathematically, Hooke's law can be expressed as:
F = -kx
Where F is the force exerted by the spring, k is the spring constant, and x is the displacement from the equilibrium position.
The force exerted by the spring can also be expressed as:
F = ma
Where m is the mass of the object and a is the acceleration.
Equating the two expressions for F, we get:
ma = -kx
This equation can be rearranged to:
a = -(k/m)x
This equation represents the acceleration of the object as a function of its displacement.
Simple Harmonic Motion:
When an object is subjected to a restoring force that is proportional to its displacement and acts in the opposite direction, it undergoes simple harmonic motion.
In the case of a mass-spring system, the object oscillates back and forth around its equilibrium position with a constant amplitude.
The equation of motion for simple harmonic motion is given by:
x(t) = A cos(ωt + φ)
Where x(t) is the displacement of the object at time t, A is the amplitude of the motion, ω is the angular frequency, and φ is the phase angle.
Angular Frequency and Time Period:
The angular frequency of the oscillation is given by:
ω = √(k/m)
And the time period is given by:
T = 2π/ω
Substituting the value of ω in terms of k and m, we get:
T = 2π√(m/k)
From the above equation, we can see that the time period is inversely proportional to the square root of the mass.
Relation between Mass and Time Period:
If the time period of oscillation of a mass M is one second, then we can write:
1 = 2π√(M/k)
Squaring both sides of the equation, we get:
1 = 4π^2(M/k)
Simplifying the equation further, we get:
k = 4π^2M
Now, we need to find the time period for a mass 4M. Let's denote it as T'.
Using the equation for time period, we have:
T' = 2π√(4M/k)
Substituting the value of k, we get:
T' = 2π√(4M/4π^2M)
Simplifying the equation further, we get:
T' = 2
Therefore, the time period of oscillation for a mass 4M is 2 seconds.
To understand why the time period of oscillation for a mass 4M is 2 seconds, we need to first understand the relationship between the time period of oscillation and the mass of the object.
Time Period of Oscillation:
The time period of oscillation is the time taken for one complete cycle of oscillation. It is denoted by the symbol T and is measured in seconds.
Hooke's Law and the Time Period:
The time period of oscillation for a mass-spring system can be determined using Hooke's law, which states that the force exerted by a spring is directly proportional to the displacement of the spring from its equilibrium position.
Mathematically, Hooke's law can be expressed as:
F = -kx
Where F is the force exerted by the spring, k is the spring constant, and x is the displacement from the equilibrium position.
The force exerted by the spring can also be expressed as:
F = ma
Where m is the mass of the object and a is the acceleration.
Equating the two expressions for F, we get:
ma = -kx
This equation can be rearranged to:
a = -(k/m)x
This equation represents the acceleration of the object as a function of its displacement.
Simple Harmonic Motion:
When an object is subjected to a restoring force that is proportional to its displacement and acts in the opposite direction, it undergoes simple harmonic motion.
In the case of a mass-spring system, the object oscillates back and forth around its equilibrium position with a constant amplitude.
The equation of motion for simple harmonic motion is given by:
x(t) = A cos(ωt + φ)
Where x(t) is the displacement of the object at time t, A is the amplitude of the motion, ω is the angular frequency, and φ is the phase angle.
Angular Frequency and Time Period:
The angular frequency of the oscillation is given by:
ω = √(k/m)
And the time period is given by:
T = 2π/ω
Substituting the value of ω in terms of k and m, we get:
T = 2π√(m/k)
From the above equation, we can see that the time period is inversely proportional to the square root of the mass.
Relation between Mass and Time Period:
If the time period of oscillation of a mass M is one second, then we can write:
1 = 2π√(M/k)
Squaring both sides of the equation, we get:
1 = 4π^2(M/k)
Simplifying the equation further, we get:
k = 4π^2M
Now, we need to find the time period for a mass 4M. Let's denote it as T'.
Using the equation for time period, we have:
T' = 2π√(4M/k)
Substituting the value of k, we get:
T' = 2π√(4M/4π^2M)
Simplifying the equation further, we get:
T' = 2
Therefore, the time period of oscillation for a mass 4M is 2 seconds.
Free Test
FREE
| Start Free Test |
Community Answer
If the time period of oscillation of mass M suspended from a spring is...
T=2π√(m/k) =1sec then .
for mass 4m time period will be
T=2π√(4m/k) =2π.2.√(m/k)=2.2π.√(m/k) =2.t
since t=1sec.
therefore T=2.t=2.1sec =2sec.
for mass 4m time period will be
T=2π√(4m/k) =2π.2.√(m/k)=2.2π.√(m/k) =2.t
since t=1sec.
therefore T=2.t=2.1sec =2sec.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer?
Question Description
If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer?.
If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer?.
Solutions for If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer?, a detailed solution for If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? has been provided alongside types of If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :Correct answer is '2'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















