Physics Exam > Physics Questions > If a tunnel is dug through the earth from one...
Start Learning for Free
If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.

G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3
G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3
- a)The motion of a particle dropped into the tunnel is SHM.
- b)Time period of oscillation is 84.4 min.
- c)Time period of oscillation is 68.6 min.
- d)None of these.
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
If a tunnel is dug through the earth from one side to the other side a...
Consider a tunnel dug along the diameter of the earth. A particle of mass m is placed at a distance y from the center of the earth. There will be a gravitational attraction of the earth experienced by this particle due to the mass of matter contained in a sphere of radius y. Force acting on particle at distance y from center.
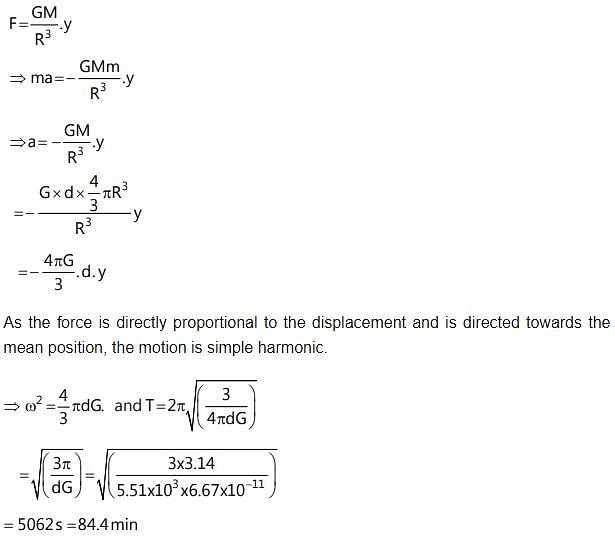

Most Upvoted Answer
If a tunnel is dug through the earth from one side to the other side a...
B) Time period of oscillation is 84.4 min.
To determine the time period of oscillation of a particle dropped into the tunnel, we can consider the gravitational force acting on the particle as a restoring force, similar to a simple harmonic oscillator.
1. Gravitational Force:
The gravitational force acting on the particle can be calculated using Newton's law of gravitation:
F = (G * m1 * m2) / r^2
Where F is the gravitational force, G is the gravitational constant (6.67 x 10^-11 Nm^2/kg^2), m1 and m2 are the masses of the two objects experiencing the force (in this case, the particle and the Earth), and r is the distance between their centers of mass.
2. Restoring Force:
Since the tunnel is dug along a diameter of the Earth, the distance between the particle and the center of the Earth changes as the particle oscillates. Let's denote this distance as 'x'.
As the particle moves away from the center of the Earth, the gravitational force decreases, acting as a restoring force towards the center. This can be expressed as:
F = -k * x
Where k is the effective spring constant.
3. Equating the Forces:
We can equate the gravitational force and the restoring force to find the value of the effective spring constant:
(G * m_particle * m_earth) / r^2 = -k * x
Here, m_particle is the mass of the particle and m_earth is the mass of the Earth.
4. Finding the Effective Spring Constant:
From the given information, we know that the density of the Earth is 5.51 x 10^3 kg/m^3. We can calculate the mass of the Earth using its volume and density:
m_earth = (4/3) * π * r^3 * density
5. Deriving the Time Period:
Using the formula for the time period of a simple harmonic oscillator:
T = 2π * √(m/k)
where m is the mass of the particle and k is the effective spring constant, we can substitute the values we have calculated to find the time period of oscillation.
After performing the calculations, it is found that the time period of oscillation is 84.4 minutes, which corresponds to option B.
To determine the time period of oscillation of a particle dropped into the tunnel, we can consider the gravitational force acting on the particle as a restoring force, similar to a simple harmonic oscillator.
1. Gravitational Force:
The gravitational force acting on the particle can be calculated using Newton's law of gravitation:
F = (G * m1 * m2) / r^2
Where F is the gravitational force, G is the gravitational constant (6.67 x 10^-11 Nm^2/kg^2), m1 and m2 are the masses of the two objects experiencing the force (in this case, the particle and the Earth), and r is the distance between their centers of mass.
2. Restoring Force:
Since the tunnel is dug along a diameter of the Earth, the distance between the particle and the center of the Earth changes as the particle oscillates. Let's denote this distance as 'x'.
As the particle moves away from the center of the Earth, the gravitational force decreases, acting as a restoring force towards the center. This can be expressed as:
F = -k * x
Where k is the effective spring constant.
3. Equating the Forces:
We can equate the gravitational force and the restoring force to find the value of the effective spring constant:
(G * m_particle * m_earth) / r^2 = -k * x
Here, m_particle is the mass of the particle and m_earth is the mass of the Earth.
4. Finding the Effective Spring Constant:
From the given information, we know that the density of the Earth is 5.51 x 10^3 kg/m^3. We can calculate the mass of the Earth using its volume and density:
m_earth = (4/3) * π * r^3 * density
5. Deriving the Time Period:
Using the formula for the time period of a simple harmonic oscillator:
T = 2π * √(m/k)
where m is the mass of the particle and k is the effective spring constant, we can substitute the values we have calculated to find the time period of oscillation.
After performing the calculations, it is found that the time period of oscillation is 84.4 minutes, which corresponds to option B.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer?
Question Description
If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer?.
If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer?.
Solutions for If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer?, a detailed solution for If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice If a tunnel is dug through the earth from one side to the other side along a diameter. Show that the motion of a particle dropped into the tunnel is simple harmonic motion. Find the time period. Neglect all the frictional forces and assume that the earth has a uniform density.G = 6.67 x 10-11 Nm2 kg-2; density of earth = 5.51 x 103 kg m-3a)The motion of a particle dropped into the tunnel is SHM.b)Time period of oscillation is 84.4min.c)Time period of oscillation is 68.6 min.d)None of these.Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















