Physics Exam > Physics Questions > The wavelength of a photon and the de-Broglie...
Start Learning for Free
The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energy
- a)Electron
- b)U-atom
- c)Nothing can be predicted
- d)Photon
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The wavelength of a photon and the de-Broglie wavelength of an electro...
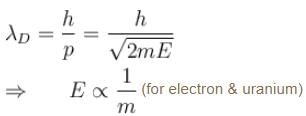
for photon & electron
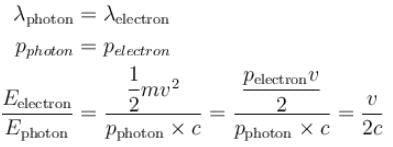
The correct answer is: Photon
Most Upvoted Answer
The wavelength of a photon and the de-Broglie wavelength of an electro...
Explanation:
The de-Broglie wavelength of a particle is given by the equation:
λ = h / p
Where λ is the wavelength, h is the Planck's constant, and p is the momentum of the particle.
The wavelength of a photon is given by the equation:
λ = c / f
Where λ is the wavelength, c is the speed of light, and f is the frequency of the photon.
Comparison between the wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom:
1. Photon:
The wavelength of a photon is determined by its frequency, and it does not have any mass. Therefore, the momentum of a photon is given by:
p = h / λ = hf / c
Since the speed of light is a constant, the momentum of a photon is directly proportional to its frequency. Higher frequency photons have higher momentum and shorter wavelengths.
2. Electron:
The de-Broglie wavelength of an electron is determined by its momentum, which is given by its mass and velocity. The kinetic energy of an electron can be calculated using the equation:
K.E. = (1/2)mv²
Where m is the mass of the electron and v is its velocity.
3. Uranium Atom:
Similarly, the de-Broglie wavelength of a uranium atom is determined by its momentum, which is given by its mass and velocity. The kinetic energy of a uranium atom can be calculated using the equation:
K.E. = (1/2)Mv²
Where M is the mass of the uranium atom and v is its velocity.
Determining the particle with the highest kinetic energy:
Since the wavelength of the photon and the de-Broglie wavelength of the electron and uranium atom are identical, it means that their momenta are also equal. However, the kinetic energy of a particle is determined by its mass and velocity.
1. Photon:
Since the photon does not have any mass, its kinetic energy is zero.
2. Electron:
The electron has a much smaller mass compared to a uranium atom. Therefore, for the same momentum, the electron will have a higher velocity and thus a higher kinetic energy compared to the uranium atom.
3. Uranium Atom:
The uranium atom has a much larger mass compared to an electron. Therefore, for the same momentum, the uranium atom will have a lower velocity and thus a lower kinetic energy compared to the electron.
Therefore, the electron will have the highest kinetic energy among the given options.
The de-Broglie wavelength of a particle is given by the equation:
λ = h / p
Where λ is the wavelength, h is the Planck's constant, and p is the momentum of the particle.
The wavelength of a photon is given by the equation:
λ = c / f
Where λ is the wavelength, c is the speed of light, and f is the frequency of the photon.
Comparison between the wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom:
1. Photon:
The wavelength of a photon is determined by its frequency, and it does not have any mass. Therefore, the momentum of a photon is given by:
p = h / λ = hf / c
Since the speed of light is a constant, the momentum of a photon is directly proportional to its frequency. Higher frequency photons have higher momentum and shorter wavelengths.
2. Electron:
The de-Broglie wavelength of an electron is determined by its momentum, which is given by its mass and velocity. The kinetic energy of an electron can be calculated using the equation:
K.E. = (1/2)mv²
Where m is the mass of the electron and v is its velocity.
3. Uranium Atom:
Similarly, the de-Broglie wavelength of a uranium atom is determined by its momentum, which is given by its mass and velocity. The kinetic energy of a uranium atom can be calculated using the equation:
K.E. = (1/2)Mv²
Where M is the mass of the uranium atom and v is its velocity.
Determining the particle with the highest kinetic energy:
Since the wavelength of the photon and the de-Broglie wavelength of the electron and uranium atom are identical, it means that their momenta are also equal. However, the kinetic energy of a particle is determined by its mass and velocity.
1. Photon:
Since the photon does not have any mass, its kinetic energy is zero.
2. Electron:
The electron has a much smaller mass compared to a uranium atom. Therefore, for the same momentum, the electron will have a higher velocity and thus a higher kinetic energy compared to the uranium atom.
3. Uranium Atom:
The uranium atom has a much larger mass compared to an electron. Therefore, for the same momentum, the uranium atom will have a lower velocity and thus a lower kinetic energy compared to the electron.
Therefore, the electron will have the highest kinetic energy among the given options.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer?
Question Description
The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer?.
The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer?.
Solutions for The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The wavelength of a photon and the de-Broglie wavelength of an electron and uranium atom are identical. Which one of them will have highest kinetic energya)Electronb)U-atomc)Nothing can be predictedd)PhotonCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















