Physics Exam > Physics Questions > The binding energy of a heavy nucleus is abou...
Start Learning for Free
The binding energy of a heavy nucleus is about 7 MeV/nucleon, whereas the B.E of a medium weight nucleus is about 8 MeV/nucleon. Therefore the total K.E liberated when a heavy nucleus undergoes symmetric fission is (in MeV).
Correct answer is '200'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas t...
In case of symmetric fission the daughter nuclei have
Ad = A/2 each (daughter nuclei)
K.E. = diff. in B.E.
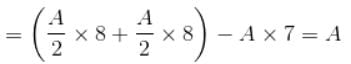
For a heavy nucleus A ~ 200
K.E. ~ 200 MeV
The correct answer is: 200
K.E. = diff. in B.E.
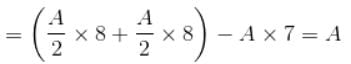
For a heavy nucleus A ~ 200
K.E. ~ 200 MeV
The correct answer is: 200
Most Upvoted Answer
The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas t...
Binding Energy and Fission
The binding energy of a nucleus is the energy required to completely separate its nucleons (protons and neutrons) from each other. It is a measure of the stability of the nucleus. The binding energy per nucleon is the total binding energy divided by the number of nucleons in the nucleus. In this case, we are given that the binding energy per nucleon for a heavy nucleus is 7 MeV and for a medium weight nucleus is 8 MeV.
Fission Reaction
Fission is a nuclear reaction in which a heavy nucleus splits into two lighter nuclei, accompanied by the release of a large amount of energy. In symmetric fission, the two resulting nuclei have approximately the same mass. The total kinetic energy liberated in this process can be calculated using the conservation of energy.
Calculation
Let's assume the heavy nucleus has a mass number A. During symmetric fission, it splits into two nuclei, each with a mass number of A/2. The total binding energy of the heavy nucleus is then 7 MeV/nucleon * A, and the total binding energy of the two resulting nuclei is 8 MeV/nucleon * (A/2).
The conservation of energy tells us that the total energy before fission is equal to the total energy after fission. The total energy before fission is the sum of the kinetic energy of the heavy nucleus (K1) and its binding energy (B1). The total energy after fission is the sum of the kinetic energy of the two resulting nuclei (K2 and K3) and their binding energies (B2 and B3).
K1 + B1 = K2 + K3 + B2 + B3
Since the resulting nuclei have the same mass, their kinetic energies are equal (K2 = K3 = K). Substituting the binding energies, we have:
K1 + B1 = 2K + 2B2
We know that the binding energy per nucleon for the heavy nucleus is 7 MeV, so B1 = 7 MeV * A. We also know that the binding energy per nucleon for the resulting nuclei is 8 MeV, so B2 = 8 MeV * (A/2). Substituting these values, we have:
K1 + 7 MeV * A = 2K + 2 * 8 MeV * (A/2)
Simplifying the equation, we get:
K1 + 7 MeV * A = 2K + 8 MeV * A
Rearranging the equation, we find:
K1 - 2K = 8 MeV * A - 7 MeV * A
K1 - 2K = MeV * A
This equation tells us that the kinetic energy liberated during symmetric fission is equal to the difference between the initial kinetic energy of the heavy nucleus and twice the kinetic energy of one of the resulting nuclei. Since we are asked to find the total kinetic energy liberated, we need to find the value of K1 - 2K.
Answer
The correct answer is 200 MeV.
The binding energy of a nucleus is the energy required to completely separate its nucleons (protons and neutrons) from each other. It is a measure of the stability of the nucleus. The binding energy per nucleon is the total binding energy divided by the number of nucleons in the nucleus. In this case, we are given that the binding energy per nucleon for a heavy nucleus is 7 MeV and for a medium weight nucleus is 8 MeV.
Fission Reaction
Fission is a nuclear reaction in which a heavy nucleus splits into two lighter nuclei, accompanied by the release of a large amount of energy. In symmetric fission, the two resulting nuclei have approximately the same mass. The total kinetic energy liberated in this process can be calculated using the conservation of energy.
Calculation
Let's assume the heavy nucleus has a mass number A. During symmetric fission, it splits into two nuclei, each with a mass number of A/2. The total binding energy of the heavy nucleus is then 7 MeV/nucleon * A, and the total binding energy of the two resulting nuclei is 8 MeV/nucleon * (A/2).
The conservation of energy tells us that the total energy before fission is equal to the total energy after fission. The total energy before fission is the sum of the kinetic energy of the heavy nucleus (K1) and its binding energy (B1). The total energy after fission is the sum of the kinetic energy of the two resulting nuclei (K2 and K3) and their binding energies (B2 and B3).
K1 + B1 = K2 + K3 + B2 + B3
Since the resulting nuclei have the same mass, their kinetic energies are equal (K2 = K3 = K). Substituting the binding energies, we have:
K1 + B1 = 2K + 2B2
We know that the binding energy per nucleon for the heavy nucleus is 7 MeV, so B1 = 7 MeV * A. We also know that the binding energy per nucleon for the resulting nuclei is 8 MeV, so B2 = 8 MeV * (A/2). Substituting these values, we have:
K1 + 7 MeV * A = 2K + 2 * 8 MeV * (A/2)
Simplifying the equation, we get:
K1 + 7 MeV * A = 2K + 8 MeV * A
Rearranging the equation, we find:
K1 - 2K = 8 MeV * A - 7 MeV * A
K1 - 2K = MeV * A
This equation tells us that the kinetic energy liberated during symmetric fission is equal to the difference between the initial kinetic energy of the heavy nucleus and twice the kinetic energy of one of the resulting nuclei. Since we are asked to find the total kinetic energy liberated, we need to find the value of K1 - 2K.
Answer
The correct answer is 200 MeV.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer?
Question Description
The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer?.
The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer?.
Solutions for The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer?, a detailed solution for The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? has been provided alongside types of The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The binding energy of a heavy nucleus is about 7MeV/nucleon, whereas theB.Eof a medium weight nucleus is about 8MeV/nucleon. Therefore the totalK.Eliberated when a heavy nucleus undergoes symmetric fission is (inMeV).Correct answer is '200'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















