Electrical Engineering (EE) Exam > Electrical Engineering (EE) Questions > The dielectric strength of rubber is 30000 V/...
Start Learning for Free
The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?
- a)0.9 cm
- b)0.8 mm
- c)1.0 cm
- d)1.1 mm
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The dielectric strength of rubber is 30000 V/mm at a frequency of 50 H...
Given that,
Electric field (E) = 30000 V/mm
Breakdown voltage (V) = 33 kV
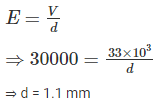
Most Upvoted Answer
The dielectric strength of rubber is 30000 V/mm at a frequency of 50 H...
Dielectric Strength and Breakdown Voltage
Dielectric strength is a measure of the electrical insulation capability of a material. It represents the maximum electric field that a material can withstand before it breaks down and allows current to flow through it. For insulating materials like rubber, dielectric strength is an important property to consider in electrical applications.
In this case, the dielectric strength of rubber is given as 30,000 V/mm at a frequency of 50 Hz. This means that the rubber insulation can withstand an electric field of 30,000 volts per millimeter before it breaks down.
Calculating the Required Insulation Thickness
To determine the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown, we can use the formula:
Insulation thickness (mm) = Voltage (kV) / Electric field strength (V/mm)
Here, the voltage is given as 33 kV. We need to convert this to volts by multiplying it by 1,000 to get 33,000 V. The electric field strength is given as 30,000 V/mm.
Substituting these values into the formula, we get:
Insulation thickness (mm) = 33,000 V / 30,000 V/mm
Simplifying the equation, we find:
Insulation thickness (mm) = 1.1 mm
Therefore, the required insulation thickness on the electrical conductor to sustain the breakdown at 33 kV is 1.1 mm.
Conclusion
In summary, to determine the insulation thickness required on an electrical conductor to sustain the breakdown at a given voltage, we need to know the dielectric strength of the insulating material. By using the formula for insulation thickness calculation and substituting the given values, we can calculate the required thickness. In this case, the correct answer is option D, 1.1 mm.
Dielectric strength is a measure of the electrical insulation capability of a material. It represents the maximum electric field that a material can withstand before it breaks down and allows current to flow through it. For insulating materials like rubber, dielectric strength is an important property to consider in electrical applications.
In this case, the dielectric strength of rubber is given as 30,000 V/mm at a frequency of 50 Hz. This means that the rubber insulation can withstand an electric field of 30,000 volts per millimeter before it breaks down.
Calculating the Required Insulation Thickness
To determine the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown, we can use the formula:
Insulation thickness (mm) = Voltage (kV) / Electric field strength (V/mm)
Here, the voltage is given as 33 kV. We need to convert this to volts by multiplying it by 1,000 to get 33,000 V. The electric field strength is given as 30,000 V/mm.
Substituting these values into the formula, we get:
Insulation thickness (mm) = 33,000 V / 30,000 V/mm
Simplifying the equation, we find:
Insulation thickness (mm) = 1.1 mm
Therefore, the required insulation thickness on the electrical conductor to sustain the breakdown at 33 kV is 1.1 mm.
Conclusion
In summary, to determine the insulation thickness required on an electrical conductor to sustain the breakdown at a given voltage, we need to know the dielectric strength of the insulating material. By using the formula for insulation thickness calculation and substituting the given values, we can calculate the required thickness. In this case, the correct answer is option D, 1.1 mm.
Attention Electrical Engineering (EE) Students!
To make sure you are not studying endlessly, EduRev has designed Electrical Engineering (EE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Electrical Engineering (EE).
|
Explore Courses for Electrical Engineering (EE) exam
|
|
Similar Electrical Engineering (EE) Doubts
Top Courses for Electrical Engineering (EE)View all
The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer?
Question Description
The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? for Electrical Engineering (EE) 2024 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer?.
The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? for Electrical Engineering (EE) 2024 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer?.
Solutions for The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electrical Engineering (EE).
Download more important topics, notes, lectures and mock test series for Electrical Engineering (EE) Exam by signing up for free.
Here you can find the meaning of The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The dielectric strength of rubber is 30000 V/mm at a frequency of 50 Hz. What is the thickness of insulation required on an electrical conductor at 33 kV to sustain the breakdown?a)0.9 cmb)0.8 mmc)1.0 cmd)1.1 mmCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice Electrical Engineering (EE) tests.
|
|
Explore Courses for Electrical Engineering (EE) exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup