Physics Exam > Physics Questions > A string under a tension of 129.6 N produces ...
Start Learning for Free
A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.
- a)50
- b)100
- c)150
- d)200
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
A string under a tension of 129.6 N produces 10 beats per sec when is ...
As here the tension in the wire in charged, so its fundamental frequency
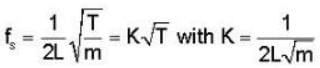
Now with increase in tension, K√T will increase and beats and decreasing to zero when T = 160 N (as unison means the frequencies are equal).

Substituting the value of K from second equation in first,
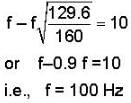
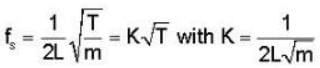
Now with increase in tension, K√T will increase and beats and decreasing to zero when T = 160 N (as unison means the frequencies are equal).

Substituting the value of K from second equation in first,
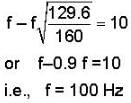
Most Upvoted Answer
A string under a tension of 129.6 N produces 10 beats per sec when is ...
As here the tension in the wire in charged, so its fundamental frequency
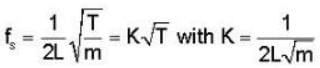
Now with increase in tension, K√T will increase and beats and decreasing to zero when T = 160 N (as unison means the frequencies are equal).

Substituting the value of K from second equation in first,
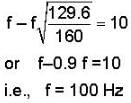
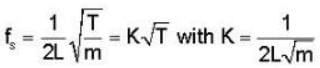
Now with increase in tension, K√T will increase and beats and decreasing to zero when T = 160 N (as unison means the frequencies are equal).

Substituting the value of K from second equation in first,
Free Test
FREE
| Start Free Test |
Community Answer
A string under a tension of 129.6 N produces 10 beats per sec when is ...
Understanding the Problem
The problem involves a string under tension producing different frequencies when the tension is varied. When the tension is 129.6 N, the string produces 10 beats per second, and when the tension is increased to 160 N, it sounds in unison with a tuning fork.
Key Formulas
- The frequency of a vibrating string is given by the formula:
Frequency (f) = (1/2L) * √(T/μ)
Where:
- T = tension in the string
- μ = linear mass density of the string
- L = length of the string
Calculating Frequencies
1. Initial Tension (129.6 N)
- The string produces 10 beats per second, meaning its frequency (f1) is 10 Hz away from the tuning fork's frequency (f). Thus, we have:
f1 = f ± 10
2. Increased Tension (160 N)
- At this tension, the string resonates in unison with the tuning fork, meaning:
f2 = f
Finding the Relation Between Frequencies
- As the tension increases, the frequency of the string also increases.
- The ratio of the square roots of the tensions gives us the relationship between the frequencies:
f2/f1 = √(T2/T1)
- Substituting T1 = 129.6 N and T2 = 160 N:
f2/f1 = √(160/129.6)
Calculation Steps
- Since f2 = f and f1 = f ± 10, we can infer that:
f2 = f1 + 10 or f1 - 10
- Solving these equations will lead you to find that:
f2 = 100 Hz.
Conclusion
The fundamental frequency of the tuning fork is therefore 100 Hz, confirming that option 'B' is correct.
The problem involves a string under tension producing different frequencies when the tension is varied. When the tension is 129.6 N, the string produces 10 beats per second, and when the tension is increased to 160 N, it sounds in unison with a tuning fork.
Key Formulas
- The frequency of a vibrating string is given by the formula:
Frequency (f) = (1/2L) * √(T/μ)
Where:
- T = tension in the string
- μ = linear mass density of the string
- L = length of the string
Calculating Frequencies
1. Initial Tension (129.6 N)
- The string produces 10 beats per second, meaning its frequency (f1) is 10 Hz away from the tuning fork's frequency (f). Thus, we have:
f1 = f ± 10
2. Increased Tension (160 N)
- At this tension, the string resonates in unison with the tuning fork, meaning:
f2 = f
Finding the Relation Between Frequencies
- As the tension increases, the frequency of the string also increases.
- The ratio of the square roots of the tensions gives us the relationship between the frequencies:
f2/f1 = √(T2/T1)
- Substituting T1 = 129.6 N and T2 = 160 N:
f2/f1 = √(160/129.6)
Calculation Steps
- Since f2 = f and f1 = f ± 10, we can infer that:
f2 = f1 + 10 or f1 - 10
- Solving these equations will lead you to find that:
f2 = 100 Hz.
Conclusion
The fundamental frequency of the tuning fork is therefore 100 Hz, confirming that option 'B' is correct.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
Question Description
A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer?.
A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer?.
Solutions for A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer?, a detailed solution for A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A string under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning fork. When the tension in the string is increased to 160 N, it sounds in unison with the same tuning fork. Calculate the fundamental frequency of the tuning fork.a)50b)100c)150d)200Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















