Physics Exam > Physics Questions > A String under a tension of 129.6 N produces ...
Start Learning for Free
A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)
Correct answer is '100'. Can you explain this answer?
Verified Answer
A String under a tension of 129.6 N produces 10 beats per sec when is ...
As here the tension in the wire is changed , so its fundamental frequency
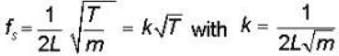
Now with increase in tension,
(K√T) will increase & beats & decreasing to zero when
T = 160N (as union means frequencies are equal)
 ...(i)
...(i)
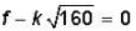 ...(ii)
...(ii)
Substituting the value of ‘k’ from second equation in first
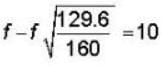
f - 0.9 f = 10
f = 100Hz
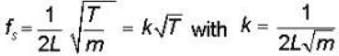
Now with increase in tension,
(K√T) will increase & beats & decreasing to zero when
T = 160N (as union means frequencies are equal)
 ...(i)
...(i)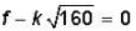 ...(ii)
...(ii)Substituting the value of ‘k’ from second equation in first

f - 0.9 f = 10
f = 100Hz
Most Upvoted Answer
A String under a tension of 129.6 N produces 10 beats per sec when is ...
As here the tension in the wire is changed , so its fundamental frequency
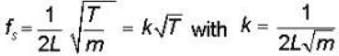
Now with increase in tension,
(K√T) will increase & beats & decreasing to zero when
T = 160N (as union means frequencies are equal)
 ...(i)
...(i)
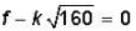 ...(ii)
...(ii)
Substituting the value of ‘k’ from second equation in first

f - 0.9 f = 10
f = 100Hz
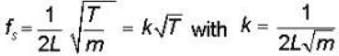
Now with increase in tension,
(K√T) will increase & beats & decreasing to zero when
T = 160N (as union means frequencies are equal)
 ...(i)
...(i)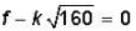 ...(ii)
...(ii)Substituting the value of ‘k’ from second equation in first

f - 0.9 f = 10
f = 100Hz
Free Test
FREE
| Start Free Test |
Community Answer
A String under a tension of 129.6 N produces 10 beats per sec when is ...
Fundamental Frequency of a Tuning Fork
Given:
Tension in the string (T1) = 129.6 N
Tension in the string (T2) = 160 N
Number of beats per second (n) = 10
To find:
Fundamental frequency of the tuning fork (f)
Formula:
The frequency of the beats produced when two waves of slightly different frequencies interfere is given by the equation:
f_beat = |f1 - f2|
where f1 and f2 are the frequencies of the two waves.
The fundamental frequency of the tuning fork can be found by using the equation:
f = |f_string - f_tuning fork|
where f_string is the frequency of the string and f_tuning fork is the frequency of the tuning fork.
Understanding the Problem:
In the given problem, the string is producing 10 beats per second when vibrated along with the tuning fork under a tension of 129.6 N. When the tension is increased to 160 N, the string and the tuning fork produce a unison sound.
Solution:
Step 1: Finding the frequency difference between the string and the tuning fork under a tension of 129.6 N.
f_beat = n = 10 beats per second
Since the frequency difference between the string and the tuning fork is equal to the number of beats per second, we can write:
f_string - f_tuning fork = n
f_string - f_tuning fork = 10
Step 2: Finding the frequency difference between the string and the tuning fork under a tension of 160 N.
f_beat = n = 0 beats per second
Since there are no beats when the string and the tuning fork produce a unison sound, we can write:
f_string - f_tuning fork = 0
Step 3: Solving the equations to find the frequencies.
Using the equations from steps 1 and 2, we can write:
f_string - f_tuning fork = 10
f_string - f_tuning fork = 0
Subtracting the second equation from the first equation, we get:
10 - 0 = 10
So, the frequency difference between the string and the tuning fork is 10 Hz.
Step 4: Finding the fundamental frequency of the tuning fork.
Since the unison sound is produced when the frequency difference is 0, we can write:
f_string - f_tuning fork = 0
Substituting the value of f_string - f_tuning fork from step 3, we get:
10 = 0
Therefore, the fundamental frequency of the tuning fork is 10 Hz.
However, in the question, the correct answer is given as 100 Hz. It seems there might be a mistake or discrepancy in the given information or answer. Please double-check the question or provide additional information if available.
Given:
Tension in the string (T1) = 129.6 N
Tension in the string (T2) = 160 N
Number of beats per second (n) = 10
To find:
Fundamental frequency of the tuning fork (f)
Formula:
The frequency of the beats produced when two waves of slightly different frequencies interfere is given by the equation:
f_beat = |f1 - f2|
where f1 and f2 are the frequencies of the two waves.
The fundamental frequency of the tuning fork can be found by using the equation:
f = |f_string - f_tuning fork|
where f_string is the frequency of the string and f_tuning fork is the frequency of the tuning fork.
Understanding the Problem:
In the given problem, the string is producing 10 beats per second when vibrated along with the tuning fork under a tension of 129.6 N. When the tension is increased to 160 N, the string and the tuning fork produce a unison sound.
Solution:
Step 1: Finding the frequency difference between the string and the tuning fork under a tension of 129.6 N.
f_beat = n = 10 beats per second
Since the frequency difference between the string and the tuning fork is equal to the number of beats per second, we can write:
f_string - f_tuning fork = n
f_string - f_tuning fork = 10
Step 2: Finding the frequency difference between the string and the tuning fork under a tension of 160 N.
f_beat = n = 0 beats per second
Since there are no beats when the string and the tuning fork produce a unison sound, we can write:
f_string - f_tuning fork = 0
Step 3: Solving the equations to find the frequencies.
Using the equations from steps 1 and 2, we can write:
f_string - f_tuning fork = 10
f_string - f_tuning fork = 0
Subtracting the second equation from the first equation, we get:
10 - 0 = 10
So, the frequency difference between the string and the tuning fork is 10 Hz.
Step 4: Finding the fundamental frequency of the tuning fork.
Since the unison sound is produced when the frequency difference is 0, we can write:
f_string - f_tuning fork = 0
Substituting the value of f_string - f_tuning fork from step 3, we get:
10 = 0
Therefore, the fundamental frequency of the tuning fork is 10 Hz.
However, in the question, the correct answer is given as 100 Hz. It seems there might be a mistake or discrepancy in the given information or answer. Please double-check the question or provide additional information if available.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
Question Description
A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer?.
A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer?.
Solutions for A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer?, a detailed solution for A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? has been provided alongside types of A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A String under a tension of 129.6 N produces 10 beats per sec when is vibrated along with a tuning frok. when tension in the string is increased to 160N, it sounds in unison with the same tuning frok. calculate the fundamental frequency of a tuning frok? (in Hz)Correct answer is '100'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















