Physics Exam > Physics Questions > A simple pendulum is set - up in a trolley wh...
Start Learning for Free
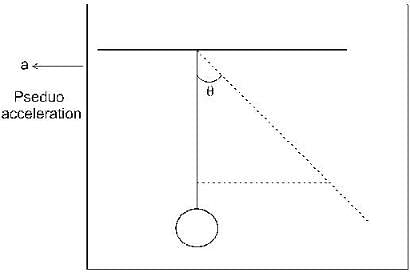
A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the vertical
- a)tan-1 (a/g) in the forward direction
- b)tan-1 (a/g) in the backward direction
- c)tan-1 (g/a) in the backward direction
- d)tan-1 (g/a) in the forward direction
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A simple pendulum is set - up in a trolley which moves to the right wi...
Most Upvoted Answer
A simple pendulum is set - up in a trolley which moves to the right wi...
With the vertical. Let's call this angle θ.
When the trolley is at rest (a=0), the pendulum hangs vertically downwards and θ=0.
When the trolley is moving with acceleration a, the pendulum is no longer vertical and θ is non-zero.
To analyze the motion of the pendulum in this situation, we need to consider the forces acting on it. There are two main forces: the tension in the thread and the gravitational force.
The tension in the thread can be decomposed into two components: one along the thread (Tcosθ) and one perpendicular to the thread (Tsinθ). The gravitational force acts downwards with a magnitude of mg, where m is the mass of the pendulum bob and g is the acceleration due to gravity.
In the mean position, the net force acting on the pendulum bob is the sum of the tension component along the thread and the gravitational force. Since the bob is not moving vertically, the net force acting on it must be zero.
Therefore, we can write the following equation:
Tcosθ - mg = 0
This equation tells us that the tension component along the thread is equal to the weight of the pendulum bob (mg). From this equation, we can solve for T:
T = mg/cosθ
Now, let's consider the forces acting along the horizontal direction. The only force in this direction is the tension component perpendicular to the thread (Tsinθ). This force is responsible for providing the necessary centripetal force to keep the bob moving in a circular path.
The centripetal force is given by the equation:
Tsinθ = m * (a - g)
This equation tells us that the tension component perpendicular to the thread is equal to the mass of the bob times the difference between the acceleration of the trolley and the acceleration due to gravity (a - g).
Combining these two equations, we can eliminate T and solve for θ:
mg/cosθ * sinθ = m * (a - g)
Simplifying this equation, we get:
tanθ = (a - g)/g
This equation relates the angle θ with the acceleration of the trolley and the acceleration due to gravity. By knowing the values of a and g, we can determine the angle θ.
When the trolley is at rest (a=0), the pendulum hangs vertically downwards and θ=0.
When the trolley is moving with acceleration a, the pendulum is no longer vertical and θ is non-zero.
To analyze the motion of the pendulum in this situation, we need to consider the forces acting on it. There are two main forces: the tension in the thread and the gravitational force.
The tension in the thread can be decomposed into two components: one along the thread (Tcosθ) and one perpendicular to the thread (Tsinθ). The gravitational force acts downwards with a magnitude of mg, where m is the mass of the pendulum bob and g is the acceleration due to gravity.
In the mean position, the net force acting on the pendulum bob is the sum of the tension component along the thread and the gravitational force. Since the bob is not moving vertically, the net force acting on it must be zero.
Therefore, we can write the following equation:
Tcosθ - mg = 0
This equation tells us that the tension component along the thread is equal to the weight of the pendulum bob (mg). From this equation, we can solve for T:
T = mg/cosθ
Now, let's consider the forces acting along the horizontal direction. The only force in this direction is the tension component perpendicular to the thread (Tsinθ). This force is responsible for providing the necessary centripetal force to keep the bob moving in a circular path.
The centripetal force is given by the equation:
Tsinθ = m * (a - g)
This equation tells us that the tension component perpendicular to the thread is equal to the mass of the bob times the difference between the acceleration of the trolley and the acceleration due to gravity (a - g).
Combining these two equations, we can eliminate T and solve for θ:
mg/cosθ * sinθ = m * (a - g)
Simplifying this equation, we get:
tanθ = (a - g)/g
This equation relates the angle θ with the acceleration of the trolley and the acceleration due to gravity. By knowing the values of a and g, we can determine the angle θ.
Free Test
FREE
| Start Free Test |
Community Answer
A simple pendulum is set - up in a trolley which moves to the right wi...
|
Explore Courses for Physics exam
|
|
Similar Physics Doubts
A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer?
Question Description
A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer?.
A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A simple pendulum is set - up in a trolley which moves to the right with an acceleration 'a' on a horizontal plane, then the thread of the pendulum in the mean position makes an angle θ with the verticala)tan-1(a/g)in the forward directionb)tan-1(a/g)in the backward directionc)tan-1(g/a)in the backward directiond)tan-1(g/a)in the forward directionCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Physics tests.
|
|
Explore Courses for Physics exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup