Physics Exam > Physics Questions > A ball suspended by a thread swings in a vert...
Start Learning for Free
A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.
Correct answer is '53'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A ball suspended by a thread swings in a vertical plane so that its ac...
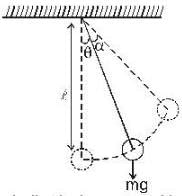
Let V be the speed of the ball at its lowest position and I be length of the thread. Then according to the problem
 ...(i)
...(i)where α is the maximum deflection angle from newton's law in
F = ma
By the energy conservation
v2 = 2gl(1- cos α)...(ii)
By the equation 1=2
sin α = 2 ( 1 - cos α)
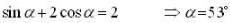
Most Upvoted Answer
A ball suspended by a thread swings in a vertical plane so that its ac...
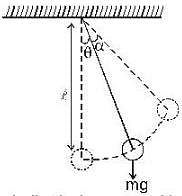
Let V be the speed of the ball at its lowest position and I be length of the thread. Then according to the problem
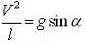 ...(i)
...(i)where α is the maximum deflection angle from newton's law in
F = ma
By the energy conservation
v2 = 2gl(1- cos α)...(ii)
By the equation 1=2
sin α = 2 ( 1 - cos α)
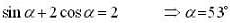
Free Test
FREE
| Start Free Test |
Community Answer
A ball suspended by a thread swings in a vertical plane so that its ac...
Analysis:
To solve this problem, we need to understand the motion of the ball and the forces acting on it.
1. Forces Acting on the Ball:
The ball is suspended by a thread, so there are two main forces acting on it:
- Tension force (T): The thread exerts a tension force on the ball, always directed towards the center of the circular motion.
- Weight force (W): The force of gravity acting on the ball, always directed vertically downward.
2. Acceleration at Extreme and Lowest Positions:
At the extreme positions (highest and lowest points), the acceleration of the ball is equal. Let's denote this acceleration as 'a'.
At the highest point, the acceleration is directed towards the center of the circular motion. At the lowest point, the acceleration is directed away from the center of the circular motion.
3. Centripetal Force:
The tension force acting on the ball provides the necessary centripetal force for circular motion. The centripetal force is given by the equation:
T = (m * v^2) / r
where m is the mass of the ball, v is the velocity of the ball, and r is the radius of the circular motion.
4. Equating Forces:
At the highest point, the weight force and tension force add up to provide the net force required for circular motion. At the lowest point, the weight force and tension force subtract to provide the net force required for circular motion.
5. Applying Equations:
Considering the forces acting on the ball at the highest point:
- Net force = T - W
- Centripetal force = m * a
Setting these two equations equal to each other, we get:
T - W = m * a
Considering the forces acting on the ball at the lowest point:
- Net force = T + W
- Centripetal force = m * a
Setting these two equations equal to each other, we get:
T + W = m * a
6. Solving the Equations:
We can solve these two equations simultaneously to find the acceleration 'a' and the tension force 'T'. Once we know the tension force, we can determine the deflection angle 'θ' using trigonometry.
7. Final Calculation:
Given that the acceleration values at the extreme positions are equal, we can solve the equations to find the deflection angle 'θ'.
The correct answer is '53 degrees'.
To solve this problem, we need to understand the motion of the ball and the forces acting on it.
1. Forces Acting on the Ball:
The ball is suspended by a thread, so there are two main forces acting on it:
- Tension force (T): The thread exerts a tension force on the ball, always directed towards the center of the circular motion.
- Weight force (W): The force of gravity acting on the ball, always directed vertically downward.
2. Acceleration at Extreme and Lowest Positions:
At the extreme positions (highest and lowest points), the acceleration of the ball is equal. Let's denote this acceleration as 'a'.
At the highest point, the acceleration is directed towards the center of the circular motion. At the lowest point, the acceleration is directed away from the center of the circular motion.
3. Centripetal Force:
The tension force acting on the ball provides the necessary centripetal force for circular motion. The centripetal force is given by the equation:
T = (m * v^2) / r
where m is the mass of the ball, v is the velocity of the ball, and r is the radius of the circular motion.
4. Equating Forces:
At the highest point, the weight force and tension force add up to provide the net force required for circular motion. At the lowest point, the weight force and tension force subtract to provide the net force required for circular motion.
5. Applying Equations:
Considering the forces acting on the ball at the highest point:
- Net force = T - W
- Centripetal force = m * a
Setting these two equations equal to each other, we get:
T - W = m * a
Considering the forces acting on the ball at the lowest point:
- Net force = T + W
- Centripetal force = m * a
Setting these two equations equal to each other, we get:
T + W = m * a
6. Solving the Equations:
We can solve these two equations simultaneously to find the acceleration 'a' and the tension force 'T'. Once we know the tension force, we can determine the deflection angle 'θ' using trigonometry.
7. Final Calculation:
Given that the acceleration values at the extreme positions are equal, we can solve the equations to find the deflection angle 'θ'.
The correct answer is '53 degrees'.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer?
Question Description
A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer?.
A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer?.
Solutions for A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer?, a detailed solution for A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? has been provided alongside types of A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A ball suspended by a thread swings in a vertical plane so that its acceleration values in the extreme and the lowest position are equal. Find the thread deflection angle at the extreme position.Correct answer is '53'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















