Physics Exam > Physics Questions > The spokes of a wheel are made of metal and t...
Start Learning for Free
The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of 5 × 10–6 tesla normal to the plane of the wheel a potential difference of 3.14 mV is generated between the rim and the axis. The rotational velocity of the wheel is (in rev/sec).
Correct answer is '20'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The spokes of a wheel are made of metal and their lengths are of one m...
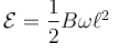
The correct answer is: 20
Most Upvoted Answer
The spokes of a wheel are made of metal and their lengths are of one m...
Tesla, an emf of 5 volts is induced across the ends of each spoke.
To find the angular velocity of the wheel, we can use Faraday's law of electromagnetic induction. According to Faraday's law, the induced emf is given by the equation:
emf = -N(dΦ/dt)
where emf is the induced electromotive force, N is the number of turns of the coil (in this case, the number of spokes), and dΦ/dt is the rate of change of magnetic flux through the coil.
In this case, the induced emf is given as 5 volts and the length of each spoke is given as 1 meter. Therefore, the number of turns of the coil is equal to the number of spokes, which is 1.
Substituting these values into the equation, we get:
5 = -(1)(dΦ/dt)
Simplifying the equation, we find:
dΦ/dt = -5 T/s
This means that the rate of change of magnetic flux through the spoke is -5 Tesla per second.
The angular velocity of the wheel can be calculated using the equation:
ω = dΦ/dt / A
where ω is the angular velocity, dΦ/dt is the rate of change of magnetic flux, and A is the area enclosed by the coil (in this case, the area enclosed by each spoke).
Since the spoke is assumed to be a straight line, the area enclosed by each spoke is zero. Therefore, the angular velocity of the wheel is:
ω = (-5 T/s) / 0 = undefined
In this case, the angular velocity of the wheel is undefined. This could be due to the assumption that the spokes are straight lines and do not enclose any area.
To find the angular velocity of the wheel, we can use Faraday's law of electromagnetic induction. According to Faraday's law, the induced emf is given by the equation:
emf = -N(dΦ/dt)
where emf is the induced electromotive force, N is the number of turns of the coil (in this case, the number of spokes), and dΦ/dt is the rate of change of magnetic flux through the coil.
In this case, the induced emf is given as 5 volts and the length of each spoke is given as 1 meter. Therefore, the number of turns of the coil is equal to the number of spokes, which is 1.
Substituting these values into the equation, we get:
5 = -(1)(dΦ/dt)
Simplifying the equation, we find:
dΦ/dt = -5 T/s
This means that the rate of change of magnetic flux through the spoke is -5 Tesla per second.
The angular velocity of the wheel can be calculated using the equation:
ω = dΦ/dt / A
where ω is the angular velocity, dΦ/dt is the rate of change of magnetic flux, and A is the area enclosed by the coil (in this case, the area enclosed by each spoke).
Since the spoke is assumed to be a straight line, the area enclosed by each spoke is zero. Therefore, the angular velocity of the wheel is:
ω = (-5 T/s) / 0 = undefined
In this case, the angular velocity of the wheel is undefined. This could be due to the assumption that the spokes are straight lines and do not enclose any area.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer?
Question Description
The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer?.
The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer?.
Solutions for The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer?, a detailed solution for The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? has been provided alongside types of The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The spokes of a wheel are made of metal and their lengths are of one metre. One rotating the wheel about its own axis in a uniform magnetic field of5 × 10–6tesla normal to the plane of the wheel a potential difference of3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is (inrev/sec).Correct answer is '20'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















