Physics Exam > Physics Questions > A charged particle is projected with velocity...
Start Learning for Free
A charged particle is projected with velocity v0 along positive x-axis. The magnetic field B is directed along negative z-axis between x = 0 and x = L. The particle emerges out (at x = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (at x = 0) along positive x-axis so that when it emerges out (at x = L), the angle made by it is 30° with the direction of projection is nv0. Find the value of n.
Correct answer is '1.732'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A charged particle is projected with velocityv0along positive x-axis. ...
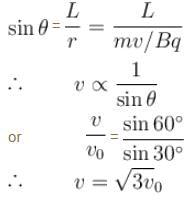
The correct answer is: 1.732
Most Upvoted Answer
A charged particle is projected with velocityv0along positive x-axis. ...
To find the velocity of the charged particle at the point of emergence, we can use the principles of electromagnetic force.
The Lorentz force on a charged particle moving in a magnetic field is given by:
F = q(v x B)
where F is the force, q is the charge of the particle, v is the velocity vector of the particle, and B is the magnetic field vector.
In this case, the force is acting in the negative y-direction (since the particle emerges at an angle of 60 degrees), so we can write:
F = -q(v x B)
The magnitude of the force is given by:
|F| = q|v||B|sinθ
where θ is the angle between the velocity vector and the magnetic field vector.
Since the particle is projected along the positive x-axis, the velocity vector can be written as:
v = v0i
where v0 is the magnitude of the initial velocity and i is the unit vector along the x-axis.
The magnetic field vector is given to be directed along the negative z-axis, so we can write:
B = -Bk
where B is the magnitude of the magnetic field and k is the unit vector along the z-axis.
The angle θ between the velocity vector and the magnetic field vector is 90 degrees, since they are perpendicular to each other.
Plugging these values into the equation for the magnitude of the force, we get:
|F| = q|v0||B|sin90 = qv0B
The force is acting in the negative y-direction, so we can write:
F = -Fj
where j is the unit vector along the y-axis.
Since F = q(v x B), we can equate the corresponding components to get:
-F = q(v0B)j
Comparing the y-components, we get:
-qv0B = -F
Solving for v0, we find:
v0 = F/(qB)
So, the magnitude of the initial velocity is given by:
|v0| = |F|/(q|B|)
Now, we can substitute the given values:
|F| = mg (where m is the mass of the particle and g is the acceleration due to gravity)
q = charge of the particle
B = magnitude of the magnetic field
Plugging these values into the equation, we get:
|v0| = mg/(q|B|)
So, the magnitude of the initial velocity is mg/(q|B|).
Note: This calculation assumes that the only force acting on the particle is the electromagnetic force due to the magnetic field. Other forces such as gravitational force or electric force may also be present, depending on the specific scenario.
The Lorentz force on a charged particle moving in a magnetic field is given by:
F = q(v x B)
where F is the force, q is the charge of the particle, v is the velocity vector of the particle, and B is the magnetic field vector.
In this case, the force is acting in the negative y-direction (since the particle emerges at an angle of 60 degrees), so we can write:
F = -q(v x B)
The magnitude of the force is given by:
|F| = q|v||B|sinθ
where θ is the angle between the velocity vector and the magnetic field vector.
Since the particle is projected along the positive x-axis, the velocity vector can be written as:
v = v0i
where v0 is the magnitude of the initial velocity and i is the unit vector along the x-axis.
The magnetic field vector is given to be directed along the negative z-axis, so we can write:
B = -Bk
where B is the magnitude of the magnetic field and k is the unit vector along the z-axis.
The angle θ between the velocity vector and the magnetic field vector is 90 degrees, since they are perpendicular to each other.
Plugging these values into the equation for the magnitude of the force, we get:
|F| = q|v0||B|sin90 = qv0B
The force is acting in the negative y-direction, so we can write:
F = -Fj
where j is the unit vector along the y-axis.
Since F = q(v x B), we can equate the corresponding components to get:
-F = q(v0B)j
Comparing the y-components, we get:
-qv0B = -F
Solving for v0, we find:
v0 = F/(qB)
So, the magnitude of the initial velocity is given by:
|v0| = |F|/(q|B|)
Now, we can substitute the given values:
|F| = mg (where m is the mass of the particle and g is the acceleration due to gravity)
q = charge of the particle
B = magnitude of the magnetic field
Plugging these values into the equation, we get:
|v0| = mg/(q|B|)
So, the magnitude of the initial velocity is mg/(q|B|).
Note: This calculation assumes that the only force acting on the particle is the electromagnetic force due to the magnetic field. Other forces such as gravitational force or electric force may also be present, depending on the specific scenario.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer?
Question Description
A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer?.
A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer?.
Solutions for A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer?, a detailed solution for A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? has been provided alongside types of A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A charged particle is projected with velocityv0along positive x-axis. The magnetic fieldB is directed along negativez-axis betweenx= 0andx = L. The particle emerges out (atx = L) at an angle of 60° with the direction of projection. Find the velocity with which the same particle is projected (atx= 0)along positivex-axis so that when it emerges out (atx = L), the angle made by it is 30° with the direction of projection isnv0. Find the value ofn.Correct answer is '1.732'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















