Physics Exam > Physics Questions > What is the reciprocal lattice to simple cubi...
Start Learning for Free
What is the reciprocal lattice to simple cubic lattice?
- a)fcc
- b)simple cubic
- c)hcp
- d)bcc
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
What is the reciprocal lattice to simple cubic lattice?a)fccb)simple c...
Primitive translation Vectors of simple cubic lattice may be written as :
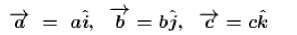
Then primitive translation vector of reciprocal lattice is
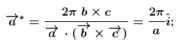
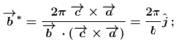
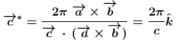
Hence, the reciprocal lattice is itself a simple cubic lattice with lattice constant
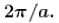
The correct answer is: simple cubic
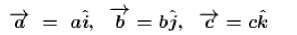
Then primitive translation vector of reciprocal lattice is
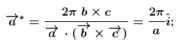
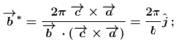
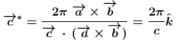
Hence, the reciprocal lattice is itself a simple cubic lattice with lattice constant
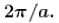
The correct answer is: simple cubic
Most Upvoted Answer
What is the reciprocal lattice to simple cubic lattice?a)fccb)simple c...
Reciprocal lattice is a concept used in solid state physics to describe the periodicity of a crystal lattice in reciprocal space. It is a mathematical construct that provides a convenient way to analyze the diffraction patterns produced by crystals.
The reciprocal lattice is derived from the direct lattice, which represents the arrangement of atoms in the crystal. In the case of a simple cubic lattice, the direct lattice consists of an infinite array of points arranged in a simple cubic structure, with lattice points located at the corners of the unit cell.
To determine the reciprocal lattice for a given direct lattice, we need to define a set of reciprocal lattice vectors. These vectors are chosen such that their dot products with the direct lattice vectors are all integers.
In the case of a simple cubic lattice, the direct lattice vectors are given by:
a1 = a * x̂
a2 = a * ȳ̂
a3 = a * ẑ
where a is the lattice constant and x̂, ȳ̂, and ẑ are the unit vectors along the x, y, and z axes, respectively.
To find the reciprocal lattice vectors, we use the formula:
b1 = 2π(a2 × a3) / (a1 · (a2 × a3))
b2 = 2π(a3 × a1) / (a1 · (a2 × a3))
b3 = 2π(a1 × a2) / (a1 · (a2 × a3))
where × denotes the cross product and · denotes the dot product.
In the case of a simple cubic lattice, the reciprocal lattice vectors are given by:
b1 = 2πȳ̂ × ẑ / (a^3)
b2 = 2πẑ × x̂ / (a^3)
b3 = 2πx̂ × ȳ̂ / (a^3)
These reciprocal lattice vectors represent the periodicity of the simple cubic lattice in reciprocal space. The reciprocal lattice itself is also a simple cubic lattice, with lattice points located at the corners of the unit cell. Therefore, the correct answer is option B - simple cubic.
The reciprocal lattice is derived from the direct lattice, which represents the arrangement of atoms in the crystal. In the case of a simple cubic lattice, the direct lattice consists of an infinite array of points arranged in a simple cubic structure, with lattice points located at the corners of the unit cell.
To determine the reciprocal lattice for a given direct lattice, we need to define a set of reciprocal lattice vectors. These vectors are chosen such that their dot products with the direct lattice vectors are all integers.
In the case of a simple cubic lattice, the direct lattice vectors are given by:
a1 = a * x̂
a2 = a * ȳ̂
a3 = a * ẑ
where a is the lattice constant and x̂, ȳ̂, and ẑ are the unit vectors along the x, y, and z axes, respectively.
To find the reciprocal lattice vectors, we use the formula:
b1 = 2π(a2 × a3) / (a1 · (a2 × a3))
b2 = 2π(a3 × a1) / (a1 · (a2 × a3))
b3 = 2π(a1 × a2) / (a1 · (a2 × a3))
where × denotes the cross product and · denotes the dot product.
In the case of a simple cubic lattice, the reciprocal lattice vectors are given by:
b1 = 2πȳ̂ × ẑ / (a^3)
b2 = 2πẑ × x̂ / (a^3)
b3 = 2πx̂ × ȳ̂ / (a^3)
These reciprocal lattice vectors represent the periodicity of the simple cubic lattice in reciprocal space. The reciprocal lattice itself is also a simple cubic lattice, with lattice points located at the corners of the unit cell. Therefore, the correct answer is option B - simple cubic.
Free Test
FREE
| Start Free Test |
Community Answer
What is the reciprocal lattice to simple cubic lattice?a)fccb)simple c...
Primitive translation Vectors of simple cubic lattice may be written as :
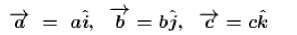
Then primitive translation vector of reciprocal lattice is
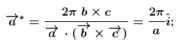
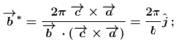
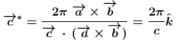
Hence, the reciprocal lattice is itself a simple cubic lattice with lattice constant
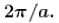
The correct answer is: simple cubic
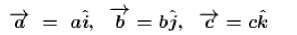
Then primitive translation vector of reciprocal lattice is
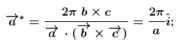
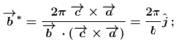
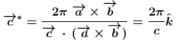
Hence, the reciprocal lattice is itself a simple cubic lattice with lattice constant
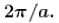
The correct answer is: simple cubic

|
Explore Courses for Physics exam
|

|
Question Description
What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer?.
What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer?.
Solutions for What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice What is the reciprocal lattice to simple cubic lattice?a)fccb)simple cubicc)hcpd)bccCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.























