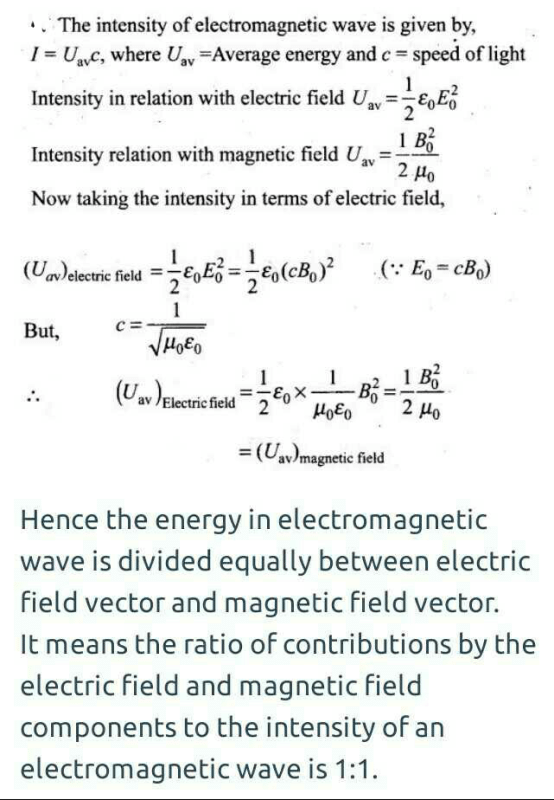
The ratio of contributions made by the electric field and magnetic field components to the intensity of an electromagnetic wave is 1:1.

Explanation:
An electromagnetic wave is composed of both electric and magnetic field components that are mutually perpendicular to each other and propagate perpendicular to the direction of wave propagation.

Intensity of an Electromagnetic Wave:
The intensity of an electromagnetic wave is the amount of energy passing through a unit area perpendicular to the direction of wave propagation per unit time. It is a measure of the wave's power per unit area.

Contribution of Electric and Magnetic Fields:
Both the electric and magnetic field components contribute to the intensity of an electromagnetic wave.

- The electric field component of an electromagnetic wave is responsible for the displacement of charged particles and the creation of electric currents. It can exert forces on charged particles and cause them to move. The energy associated with the electric field is proportional to the square of the electric field strength.

- The magnetic field component of an electromagnetic wave is responsible for the creation of magnetic fields and the induction of electric currents. It can exert forces on moving charged particles. The energy associated with the magnetic field is proportional to the square of the magnetic field strength.

Ratio of Contributions:
The intensity of an electromagnetic wave is proportional to the sum of the energies associated with its electric and magnetic field components. Since the energy associated with both components is proportional to the square of their respective field strengths, the ratio of their contributions to the intensity can be determined.

Let the electric field contribution be E and the magnetic field contribution be B.

- The energy associated with the electric field component is proportional to E^2.
- The energy associated with the magnetic field component is proportional to B^2.

The ratio of contributions is therefore (E^2) : (B^2).

Since the electric and magnetic field components are mutually perpendicular and propagate perpendicular to the direction of wave propagation, their field strengths are related by the speed of the electromagnetic wave (c), such that E = cB.

Substituting this relationship into the ratio, we have:
(E^2) : (B^2) = (cB)^2 : B^2 = c^2 : 1.

Therefore, the ratio of contributions made by the electric field and magnetic field components to the intensity of an electromagnetic wave is 1 : 1.

Hence, the correct answer is option D, 1 : 1.