Chemical Engineering Exam > Chemical Engineering Questions > Heat is generated in a 3-cm-diameter spheric...
Start Learning for Free
Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation is
- a)56°C
- b)84°C
- c)494°C
- d)650°C
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Heat is generated in a 3-cm-diameter spherical radio-active material ...
At steady state,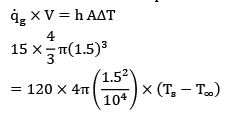
View all questions of this test
Rate of heat generation inside the sphere
= Rate of heat dissipation from the surface
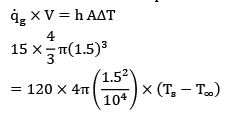
T8 = 650°C
Note:
In L.H.S, radius is put in cm, because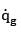 
is given in W/cm3 but in R.H.S, radius is put in m because h is given in W\m2-k.
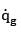is given in W/cm3 but in R.H.S, radius is put in m because h is given in W\m2-k.Most Upvoted Answer
Heat is generated in a 3-cm-diameter spherical radio-active material ...
Given:
- Diameter of the spherical radioactive material = 3 cm
- Heat generation rate = 15 W/cm3
- Heat transfer coefficient = 12 W/m2-K
- Surrounding medium temperature = 25°C
To find:
Surface temperature of the material in steady operation
Assumptions:
- Steady-state conditions exist
- Heat transfer is one-dimensional
- Thermal conductivity of the material is constant
- Conduction and convection are the only modes of heat transfer
Solution:
Step 1: Calculate the volume of the spherical radioactive material
The volume of a sphere is given by the formula:
Volume = (4/3) * π * r^3
where r is the radius of the sphere.
Given that the diameter of the sphere is 3 cm, the radius is half of the diameter:
r = 3 cm / 2 = 1.5 cm = 0.015 m
Substituting the values into the formula, we get:
Volume = (4/3) * π * (0.015 m)^3
Step 2: Calculate the heat generation rate
The total heat generation rate is given by the formula:
Heat generation = Heat generation rate * Volume
Substituting the values, we get:
Heat generation = 15 W/cm3 * (4/3) * π * (0.015 m)^3
Step 3: Calculate the surface area of the sphere
The surface area of a sphere is given by the formula:
Surface area = 4 * π * r^2
Substituting the value of the radius, we get:
Surface area = 4 * π * (0.015 m)^2
Step 4: Calculate the heat transfer rate
The heat transfer rate from the surface of the sphere to the surrounding medium is given by the formula:
Heat transfer rate = Heat transfer coefficient * Surface area * (Surface temperature - Surrounding temperature)
Substituting the given values, we get:
Heat transfer rate = 12 W/m2-K * [4 * π * (0.015 m)^2] * (Surface temperature - 25°C)
Since the system is in steady state, the heat generation rate must be equal to the heat transfer rate:
Heat generation = Heat transfer rate
Substituting the respective formulas, we get:
15 W/cm3 * (4/3) * π * (0.015 m)^3 = 12 W/m2-K * [4 * π * (0.015 m)^2] * (Surface temperature - 25°C)
Step 5: Solve for the surface temperature
Simplifying the equation, we get:
Surface temperature - 25°C = (15 W/cm3 * (4/3) * π * (0.015 m)^3) / (12 W/m2-K * [4 * π * (0.015 m)^2])
Canceling out the common terms and converting units, we get:
Surface temperature - 25°C = 0.625°C
Adding 25°C to both sides of the equation, we get:
Surface temperature = 25°C + 0.625°C = 25.625°C
Step 6: Convert the surface
- Diameter of the spherical radioactive material = 3 cm
- Heat generation rate = 15 W/cm3
- Heat transfer coefficient = 12 W/m2-K
- Surrounding medium temperature = 25°C
To find:
Surface temperature of the material in steady operation
Assumptions:
- Steady-state conditions exist
- Heat transfer is one-dimensional
- Thermal conductivity of the material is constant
- Conduction and convection are the only modes of heat transfer
Solution:
Step 1: Calculate the volume of the spherical radioactive material
The volume of a sphere is given by the formula:
Volume = (4/3) * π * r^3
where r is the radius of the sphere.
Given that the diameter of the sphere is 3 cm, the radius is half of the diameter:
r = 3 cm / 2 = 1.5 cm = 0.015 m
Substituting the values into the formula, we get:
Volume = (4/3) * π * (0.015 m)^3
Step 2: Calculate the heat generation rate
The total heat generation rate is given by the formula:
Heat generation = Heat generation rate * Volume
Substituting the values, we get:
Heat generation = 15 W/cm3 * (4/3) * π * (0.015 m)^3
Step 3: Calculate the surface area of the sphere
The surface area of a sphere is given by the formula:
Surface area = 4 * π * r^2
Substituting the value of the radius, we get:
Surface area = 4 * π * (0.015 m)^2
Step 4: Calculate the heat transfer rate
The heat transfer rate from the surface of the sphere to the surrounding medium is given by the formula:
Heat transfer rate = Heat transfer coefficient * Surface area * (Surface temperature - Surrounding temperature)
Substituting the given values, we get:
Heat transfer rate = 12 W/m2-K * [4 * π * (0.015 m)^2] * (Surface temperature - 25°C)
Since the system is in steady state, the heat generation rate must be equal to the heat transfer rate:
Heat generation = Heat transfer rate
Substituting the respective formulas, we get:
15 W/cm3 * (4/3) * π * (0.015 m)^3 = 12 W/m2-K * [4 * π * (0.015 m)^2] * (Surface temperature - 25°C)
Step 5: Solve for the surface temperature
Simplifying the equation, we get:
Surface temperature - 25°C = (15 W/cm3 * (4/3) * π * (0.015 m)^3) / (12 W/m2-K * [4 * π * (0.015 m)^2])
Canceling out the common terms and converting units, we get:
Surface temperature - 25°C = 0.625°C
Adding 25°C to both sides of the equation, we get:
Surface temperature = 25°C + 0.625°C = 25.625°C
Step 6: Convert the surface
|
Explore Courses for Chemical Engineering exam
|
|
Similar Chemical Engineering Doubts
Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer?
Question Description
Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? for Chemical Engineering 2024 is part of Chemical Engineering preparation. The Question and answers have been prepared according to the Chemical Engineering exam syllabus. Information about Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Chemical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer?.
Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? for Chemical Engineering 2024 is part of Chemical Engineering preparation. The Question and answers have been prepared according to the Chemical Engineering exam syllabus. Information about Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Chemical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer?.
Solutions for Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Chemical Engineering.
Download more important topics, notes, lectures and mock test series for Chemical Engineering Exam by signing up for free.
Here you can find the meaning of Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Heat is generated in a 3-cm-diameter spherical radio-active material uniformly at a rate of 15 W/cm3. Heat is dissipated to the surrounding medium at 25°C with a heat transfer coefficient of 12 W/m2-K. The surface temperature of the material in steady operation isa) 56°Cb) 84°Cc) 494°Cd) 650°CCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice Chemical Engineering tests.
|
|
Explore Courses for Chemical Engineering exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup