Chemical Engineering Exam > Chemical Engineering Questions > If thermal conductivity of a material of wal...
Start Learning for Free
If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)
- a)Depends on other factors
- b)More than that in case of constant thermal conductivity
- c)Less than that in case of constant thermal conductivity
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
If thermal conductivity of a material of wall varies as k0 (1 - ∝T), ...
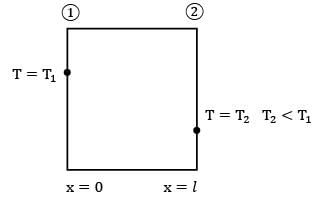
if this material is at steady state with no internal heat generation
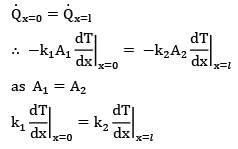
If k1 = k2 i.e. k does not depend upon temperature
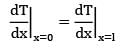
Thus temperature profile is a straight line
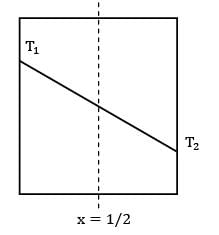
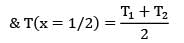
But given k = k0(1 - ∝T), where ∝ is +Ve.
∴ k1 < />2
∵ T1 > T2
∴ 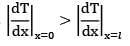
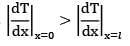
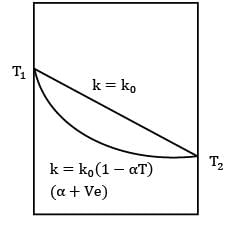
Thus temperature profile is as given
Most Upvoted Answer
If thermal conductivity of a material of wall varies as k0 (1 - ∝T), ...
Thermal Conductivity and Temperature at the Center of the Wall
Thermal conductivity is the property of a material that describes its ability to conduct heat. It is represented by the symbol k and is measured in units of W/mK. The higher the thermal conductivity of a material, the more quickly it can transfer heat.
The given equation k0 (1 - ∝T) shows that the thermal conductivity of the material of the wall varies with temperature. Here, k0 is the thermal conductivity at a reference temperature, and ∝ is a constant that determines the rate of change of thermal conductivity with temperature.
When a temperature gradient is applied across a wall made of this material, the rate of heat transfer through the wall will also vary with temperature. As a result, the temperature at the center of the wall will be affected by the variation in thermal conductivity.
Effect of Varying Thermal Conductivity on Temperature at the Center of the Wall
The temperature at the center of the wall can be calculated using the heat transfer equation. If the wall is assumed to be steady-state and one-dimensional, the temperature profile can be expressed as:
T(x) = T1 + (T2 - T1) * (x/L)
Here, T1 and T2 are the temperatures at the two surfaces of the wall, x is the distance from the surface, and L is the thickness of the wall.
The temperature gradient dT/dx can be calculated as:
dT/dx = (T2 - T1)/L
The rate of heat transfer through the wall can be expressed as:
Q = k * A * dT/dx
Here, A is the cross-sectional area of the wall.
Substituting the given equation for k, we get:
Q = k0 (1 - ∝T) * A * (T2 - T1)/L
This equation shows that the rate of heat transfer through the wall is affected by the temperature-dependent thermal conductivity.
Now, as the thermal conductivity decreases with temperature, the rate of heat transfer will also decrease. This means that the temperature at the center of the wall will be less than that in case of constant thermal conductivity. Therefore, option C is the correct answer.
Thermal conductivity is the property of a material that describes its ability to conduct heat. It is represented by the symbol k and is measured in units of W/mK. The higher the thermal conductivity of a material, the more quickly it can transfer heat.
The given equation k0 (1 - ∝T) shows that the thermal conductivity of the material of the wall varies with temperature. Here, k0 is the thermal conductivity at a reference temperature, and ∝ is a constant that determines the rate of change of thermal conductivity with temperature.
When a temperature gradient is applied across a wall made of this material, the rate of heat transfer through the wall will also vary with temperature. As a result, the temperature at the center of the wall will be affected by the variation in thermal conductivity.
Effect of Varying Thermal Conductivity on Temperature at the Center of the Wall
The temperature at the center of the wall can be calculated using the heat transfer equation. If the wall is assumed to be steady-state and one-dimensional, the temperature profile can be expressed as:
T(x) = T1 + (T2 - T1) * (x/L)
Here, T1 and T2 are the temperatures at the two surfaces of the wall, x is the distance from the surface, and L is the thickness of the wall.
The temperature gradient dT/dx can be calculated as:
dT/dx = (T2 - T1)/L
The rate of heat transfer through the wall can be expressed as:
Q = k * A * dT/dx
Here, A is the cross-sectional area of the wall.
Substituting the given equation for k, we get:
Q = k0 (1 - ∝T) * A * (T2 - T1)/L
This equation shows that the rate of heat transfer through the wall is affected by the temperature-dependent thermal conductivity.
Now, as the thermal conductivity decreases with temperature, the rate of heat transfer will also decrease. This means that the temperature at the center of the wall will be less than that in case of constant thermal conductivity. Therefore, option C is the correct answer.

|
Explore Courses for Chemical Engineering exam
|

|
Similar Chemical Engineering Doubts
If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer?
Question Description
If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? for Chemical Engineering 2024 is part of Chemical Engineering preparation. The Question and answers have been prepared according to the Chemical Engineering exam syllabus. Information about If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Chemical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer?.
If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? for Chemical Engineering 2024 is part of Chemical Engineering preparation. The Question and answers have been prepared according to the Chemical Engineering exam syllabus. Information about If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Chemical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer?.
Solutions for If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Chemical Engineering.
Download more important topics, notes, lectures and mock test series for Chemical Engineering Exam by signing up for free.
Here you can find the meaning of If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice If thermal conductivity of a material of wall varies as k0 (1 - ∝T), the temperature at the center of the wall will be (∝ is + ve)a) Depends on other factorsb) More than that in case of constant thermal conductivityc) Less than that in case of constant thermal conductivityd) None of theseCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Chemical Engineering tests.

|
Explore Courses for Chemical Engineering exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















